Chapter Twelve
Radiation and scattering of sound by the boundary value method
Abstract
This chapter applies the boundary value method to solutions of the wave equation. Those treated are radiation from a pulsating infinite cylinder; radiation from an infinite line source; scattering from a rigid sphere by a plane wave and by point sources; radiation from a point source on a sphere; a spherical cap on a sphere; a rectangular cap on a sphere; a piston in a sphere; and radiation from an oscillating convex dome and from an oscillating concave dome in an infinite baffle.
Keywords
Boundary value method; Far-field pressure; Huygens–Fresnel principle; Legendre functions; Pulsating cylinder; Wave equation
In this chapter and the next, we will derive results that were used in previous chapters to study transducers and their radiation characteristics. The aim is to provide insight into how the shape of a transducer determines its behavior as well as an understanding of how to solve acoustical problems analytically. In each problem, a new concept or method is introduced so that each problem is slightly more complicated than the previous one. Formulas are given which the interested reader may use as part of his or her own simulations. In this chapter, we will take the wave equation solutions of Chapter 2 and apply the appropriate boundary conditions to them to determine the unknown coefficients. This is known as the boundary value method. In fact, we have already used this method to solve for the reflection of a plane wave from a plane in Section 4.9, radiation from a pulsating sphere in Section 4.10, and an oscillating sphere Section 4.15. In Chapter 13, we will treat sound sources as arrays of point sources, which are integrated using the boundary integral method.
Part XXXIII: Radiation in cylindrical coordinates
12.1. Radiation from a pulsating infinite cylinder
The infinitely long pulsating cylinder is a useful model for vertical loudspeaker arrays. If the height of the array is much greater than the wavelength of the sound being radiated, then we can use a two-dimensional model of infinite extent. Because of axial symmetry, it can be treated as a one-dimensional problem with just a single radial ordinate w.
Pressure field
Because the cylinder is radiating into free space, where there are no reflections, we take the outward-going part of the solution to the cylindrical wave Eq. (2.125) given by Eq. (2.129), where
 is an unknown coefficient to be determined from the
boundary conditions. Let us now impose a boundary condition at the surface of the cylinder whereby the particle velocity normal to the surface, given by Eq. (2.130), is equal to the uniform surface velocity
is an unknown coefficient to be determined from the
boundary conditions. Let us now impose a boundary condition at the surface of the cylinder whereby the particle velocity normal to the surface, given by Eq. (2.130), is equal to the uniform surface velocity
 so that
so that
 , where a is the radius, which gives
, where a is the radius, which gives
Inserting this into Eq. (2.129) and substituting
 , where
, where
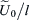 is the total volume velocity per unit length, yields
is the total volume velocity per unit length, yields
 (12.2)
(12.2)
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
