12
COOPERATIVE GAME THEORY APPLIED TO IEAS: A COMPARISON OF SOLUTION CONCEPTS
Marco Rogna
University of Trento
1. Introduction
Game theory (GT) is the study of mathematical modelling of rational players interacting with each other and it has been extensively applied in the environmental context, ranging from fisheries management to natural resources extraction and waste treatment (Zara et al., 2006a2006a, 2006a2006b). A subtopic that has received special attention is environmental pollution. As soon as the potential negative impact of anthropogenic emissions on the earth's ecosystem was widely recognized by the scientific and international community, different models have been envisaged in order to represent the possible interactions of countries to coordinate their efforts in abating emissions. Based on earlier models of transboundary pollution, a full class of new models has born to depict the ‘global warming game’ (Maler, 1989).
Although the solution concepts that have been adopted are usually already present in the game theoretical literature, it is still important to note that the ‘global warming game’ has its own specificities; indeed it is now possible to speak, without the risk of adding unjustified emphasis, of a new strand in that literature. Three elements, and their specific combination, can be considered the distinctive features of this particular game: the character of the players, the strategies at their disposal and the effects produced by their cooperative behaviour. Starting with the first, countries are the common unit of analysis (players, for game theory) and the game consists of constituting an international environmental treaty (IEA). Although this may appear quite natural, it is not trivial if examined more carefully. Countries, in fact, are neither the direct producers of emissions and pollution nor the direct recipients of their negative consequences. They act, therefore, as mediators between polluters (firms, consumers) and pollutees (people in general, for an anthropocentric perspective). According to the Coase Theorem (Coase, 1960), the problem can be solved by the market alone, through the law of demand and supply, but this would imply that pollution – or its complement, environmental quality – is a normal, ‘priceable’ good. Given that pollution is an externality and environmental quality a public, rather than a private, good, polluters and pollutees cannot use the market as an exchange platform unless a superior authority has previously defined appropriate property rights.
This leads to the second specificity, that is the strategy space defining this environmental game. It is possible to differentiate at least two distinct, although interconnected, classes of choices faced by countries. First of all, an IEA is a treaty and therefore a country has a dichotomous choice between being or not a signatory. This decision can be additionally divided into two steps, that of signing and then ratifying the treaty, as recognized, for example, in the model of Köke and Lange (2013). In general, however, this possibility is simply disregarded and the simplifying assumption of perfect coincidence between the two steps is adopted.1 The second strategic choice regards the optimal level of emissions or abatement to adopt. From a modelling perspective, speaking of emissions or abatement is equivalent since one is the complement of the other, so their use depends simply on author's preferences.2 Three further clarifications are required. First, since countries bargain as representatives of their population, a model presenting a country's unique utility function implicitly relies on the assumption that the intra-state bargaining processes have already been solved. This constitutes a parallel with the role played by the representative agent in several models, where this role is here taken by the state itself. The second point regards the enforceability of state decisions. Countries are supposed to ‘play’ as rational actors. Therefore, besides having a single utility function, they are also supposed to possess full enforceability of their decisions. If this happens through a tax or a marketable quota mechanism (in the dedicated literature it has been largely demonstrated that the two mechanisms, under certain assumptions, are equivalent (Perman et al., 2003)) or through other instruments, is not important, but the central point to stress is that a country has a mechanism to perfectly implement the decisionstrategy chosen. The third specificity concerns the outcome of cooperation which always generates positive externalities given that it reduces pollution.3
To sum up, in this paper IEAs are conceived to be games in which players are countries, supposed to be rational, utility maximizing agents. They have a double, interconnected, strategy space constituted by the choice of cooperate (being part of the agreement) or not cooperate (remaining outside) and a continuous choice on the level of emissionsabatement to adopt. The constitution of an IEA always generates positive externalities. Bearing these distinguishing characteristics in mind, this paper analyses the cooperative approach, describing the main solution concepts adopted in the literature, applied to the ‘global warming game’.
2. Cooperative versus Non-Cooperative Approach
Since this paper deals with cooperative GT (CGT), it is opportune to give its definition and to stress the difference with the non-cooperative approach (NCGT). First of all, however, it is useful to recall a general definition of GT. This discipline can be described as the mathematical modelling of situations of conflict and cooperation that, starting from assumptions about the strategic behavioural patterns of players, provides their resulting pay-offs according to the adopted solution concepts (Zara et al., 2006a2006). Bearing this definition in mind, the words of Osborne and Rubinstein (1994) clearly explain the difference between the two approaches:
A coalitional model (a cooperative model, ndr) is distinguished from a non-cooperative model primarily by its focus on what groups of players can achieve rather than on what individual players can do and by the fact that it does not consider the details of how groups of players function internally.
Another way to explain this difference refers to the enforceability of agreements. NCGT models situations where this enforceability is absent, so that players are free to strategically pursue their own objectives, whereas CGT mainly considers the allocation of cooperative gains resulting from binding agreements (Zara et al., 2006a2006a). Adopting this last view and considering the structure of the international community, based on the principle of state sovereignty, it could seem more appropriate to adopt an NCGT approach to model the ‘global warming game’. However, two arguments can be used to contradict this thesis.
The first stems from the words of Osborne and Rubinstein (1994). The authors point that the two approaches differ on their focus. It is therefore a matter of perspective, a matter of what the researcher wants to investigate and which questions she wants to answer. The problem of agreement enforceability is simply disregarded. Criticizing a coalitional model on the ground that it assumes enforceability in a situation where it does not exist, although usually rewarding, could actually cause a simple deviation from the focal point. In choosing this approach, in fact, the researcher already knows its limits and this criticism becomes a mere pleonasm. The second point should be limited to the case of IEAs and derives from a peculiar development of the literature in this field. NCGT aims at investigating the constitutional process of a coalition (an IEA) under a positive perspective (Chander and Tulkens, 2006). For positive perspective, it is meant the logical (rational) outcome of a given situation. In other words, the formation of the coalition must be in the self-interest of the constituting parties that act under the typical assumption of rationality. Consequently, the most important property that the coalition must hold is stability. This concept is operationally translated into the conditions that, once a coalition has been formed, no one of the non-signatories should find rewarding to enter the agreement and no one of the signatories has an incentive to leave (d'Aspremont et al., 1983). Mathematically, this is expressed as
where Πi ∈ S and ![]() indicate, respectively, the utility a country i enjoys being, and not being, part of coalition S. When the two inequalities are satisfied, the coalition S is stable. Two things worth to be mentioned. The first is that the focus on stability clearly amounts to consider primarily the number of coalition participants or, equivalently, the number of IEA's signatories. This, in fact, seems to be the primary objective of NCGT applied to this field (Zara et al., 2006b2006b). The second aspect relates to the fact that the stability concept used here is reminiscent of the stability set proposed in Von Neuman and Morgenstern (1947), a typical cooperative solution, although they are not coincident (Chander and Tulkens, 2006). In performing this analysis, however, a vast part of the non-cooperative literature, namely, the one adopting Reduced-Stage Game (RSG) models, considers only a particular type of free riding: external free- riding (Finus, 2008). With this expression, it is meant a country that stays outside the agreement but enjoys the positive externalities generated by it. The internal dimension of free riding, where a country joins the agreement but does not comply with it, instead, is rarely taken into consideration as recognized4 by McEvoy and Stranlund (2009) and Finus (2008). Disregarding this dimension implies to assume agreement enforceability. It then follows that this concept cannot be used any more as a discriminant between CGT and NCGT.
indicate, respectively, the utility a country i enjoys being, and not being, part of coalition S. When the two inequalities are satisfied, the coalition S is stable. Two things worth to be mentioned. The first is that the focus on stability clearly amounts to consider primarily the number of coalition participants or, equivalently, the number of IEA's signatories. This, in fact, seems to be the primary objective of NCGT applied to this field (Zara et al., 2006b2006b). The second aspect relates to the fact that the stability concept used here is reminiscent of the stability set proposed in Von Neuman and Morgenstern (1947), a typical cooperative solution, although they are not coincident (Chander and Tulkens, 2006). In performing this analysis, however, a vast part of the non-cooperative literature, namely, the one adopting Reduced-Stage Game (RSG) models, considers only a particular type of free riding: external free- riding (Finus, 2008). With this expression, it is meant a country that stays outside the agreement but enjoys the positive externalities generated by it. The internal dimension of free riding, where a country joins the agreement but does not comply with it, instead, is rarely taken into consideration as recognized4 by McEvoy and Stranlund (2009) and Finus (2008). Disregarding this dimension implies to assume agreement enforceability. It then follows that this concept cannot be used any more as a discriminant between CGT and NCGT.
Although the border line between the two approaches is more blurred than in other fields, nonetheless they remain deeply separated in a crucial point: results. NCGT generally predicts the formation of a small stable coalition (SSC) (Carraro and Siniscalco, 1993; Barrett, 1994; Diamantoudi and Sartzetakis, 2006), whereas the cooperative one asserts that the grand coalition (GSC) can be formed (Chander and Tulkens, 1995; Tulkens and Chander, 1997; Germain et al., 2010). Tulkens (1997) has dedicated a whole paper to explain in detail what causes this gap. The most important point is the fact that, in the cooperative approach, countries are given the Core solution (the comprehensive coalition) in the first step, and then are asked if they want to leave it, whereas the NCGT starts from the bottom, meaning the situation in which countries are singletons and have to agree to some form of cooperation (Tulkens, 1997). The other main difference, whose examination is postponed, is the fact that the cooperative approach assumes a strong reaction from the side of the coalition to the potential defection of one of its members. Such defection, in fact, causes a complete breaking up of the whole coalition. Apart from this last critical aspect, it is possible to note that the main difference is a matter of perspective, as the definition of Osborne and Rubinstein (1994) underlines.
At this point, however, one might wonder which is the utility of the cooperative approach if this disregards a crucial aspect such as the one of the IEA's formation process. The answer needs a premise. Full cooperation is globally optimal. This derives from well-known public goods theory (Samuelson, 1954) according to which a Pareto efficient solution in presence of a public good can be achieved only if all the interested parties are involved in the process of its allocation. From this, it follows that cooperation is desirable in itself. CGT, in fact, is said to adopt a normative perspective (Chander and Tulkens, 2006), rather than a positive one, meaning that it pursues cooperation as a goal, not a simple rational consequence. The aim becomes then to define the allocation that renders cooperation feasible and more acceptable. It can be said that it is an allocation, rather than a constitution, game. Its first goal, rendering cooperation feasible, is then subject to the constraint of satisfying individual rationality, whereas the second, related to acceptability, is bounded by the concepts of equity and fairness (Young, 1994). These can be given a general, normative meaning, or a narrower, practical one. In the first case, they have to be intended as a principle of justice, a moral attitude, whereas in the second they provide indications to shape concrete behaviours. This last interpretation presupposes a synthesis and, generally, a compromise, between the abstract principle and the material conditions of the case at hand (power considerations). The satisfaction of individual rationality constitutes this last element. The solution concepts adopted by CGT can be considered as different forms of compromise between these two requirements.
3. Solution Concepts
This section introduces and describes the most used solution concepts of CGT applied to the IEAs' field. It therefore considers only a subset of the many solution concepts adopted by CGT. Prevalence is given to single point rather than set solutions. The distinction between these two categories is self-contained in their names. Set solutions, in fact, define the whole space in which cooperation (GSC) can be sustained, whereas a single-point solution indicates a precise allocation able to perform the same task. The choice of focusing on the second category is due to this last consideration. A set solution, in fact, still leaves unsolved the problem of defining which equilibrium will emerge in the particular game at hand, providing just a space identification inside which multiple equilibria are possible (actually, every point in this space is an equilibrium). Another way to interpret the difference between the two categories recalls the distinction between feasibility and acceptability mentioned before. A set solution can be said to consider only the first element, providing an indication of all the possible allocations that are feasible, whereas a single-point solution is reached by adopting a specific equity concept, according to which there will be a unique allocation.
A further consideration needs to be done regarding the nature of the games under consideration. Cooperative games are usually divided in two categories: games with (TU) and without transferable utility (NTU) (Osborne and Rubinstein, 1994; Zara et al., 2006a2006a). The difference is easy to figure out. TU games assume that players' utility can be transferred among them (e.g. lump-sum payments in money or goods), whereas NTU games do not allow for this. Therefore, the TU assumption implies that the worth of a coalition, calculated as the sum of the utilities of its members, can be divided among them in any possible way (Zara et al., 2006a2006a). Conversely, in the NTU case, coalition members are constrained to enjoy the utility that they self-generate. Obviously, in this second class of games, it will be generally more difficult to obtain cooperation since transfers cannot be used as an instrument to induce it. Given that countries are the players in the considered game and that their utility is generally proxied by GDP, there seems to be no ground to refuse the TU assumption.
3.1 A Standard Coalitional Game and the Characteristic Function
Given a game Γ(N, v) with a number n of players (countries, in the present case, where n > 2), define as N the set of all players: N = 1, 2, ..., n. Furthermore, denote a coalition S as a strict subset of N : S⊂N. The set N is also a coalition, namely, the grand coalition, including all players. Finally, define as Σ the set that collects all the possible coalitions – among which the empty set Ø – which will necessarily have 2n elements. It has to be noted that the n players, called singletons, are also treated as single member coalitions. The following necessary step to characterize a coalitional game is to define a characteristic function v, intended as a real-valued function that assigns a value to each one of the coalitions included in the set Σ. The value v(S), since we are considering a TU game, can be interpreted as the total pay-off available for distribution among the members of coalition S (Osborne and Rubinstein, 1994). By assumption, v (Ø) = 0, meaning that the empty coalition has a zero value.
Definition. A TU coalitional game Γ(N, v) in characteristic function form consists of a finite set of players N and a function v that assigns to each non-empty subset S of N a real number v(S), representing the utility of S available for distribution among its members.
In the large set of games in characteristic function form, particular classes have been individuated according to the properties of the same characteristic function: convex (supermodular), and superadditive games. The first class is a subset of the second. In order for a game to be defined convex, the characteristic function must satisfy the following inequality:
Driessen (2013) shows the equivalence between convexity and supermodularity of v. A characteristic function is supermodular if:
From this last condition, it can be said that a cooperative convex game is one in which, starting from a given coalition, the marginal contribution a player brings to it increases monotonically by increasing the size of the same coalition. Superadditivity, instead, can be considered as a weaker version of convexity, requiring that the characteristic function satisfies the following property:5
It will be seen later on why these two properties are really important in a coalitional game. For the moment, it is sufficient to note that, when superadditivity holds, the characteristic value of every coalition S ≠ N cannot be higher than the value of the grand coalition. Obviously, this is true for convexity as well.
At this point, it is necessary to define the characteristic function itself
This is simply the sum of the utilities (indicated by Πi(.)) of all coalition's members, given the strategies (φi) adopted by all the players in the game. It is then possible to link a coalitional form of a game with its strategic form. From standard GT, it is known that an n-players game is composed by the 2n-tuple (Φ1, ..., Φn, Π1, ..., Πn) where Φi is the set of pure strategies of player i and Πi(φ1...φn) is the pay-off of player i if player 1 uses the strategy φ1 ∈ Φ1 and player 2 uses φ2 ∈ Φ2,..., and player n φn ∈ Φn (Ferguson, 2005). Therefore, from a standard game in strategic form, the passage to a coalitional form game entails to give a value to each possible coalition taking into consideration the strategies adopted by its members and the ones of the non-members. In the IEA game, the set of strategies Φi is the level of emissions or abatement that each country will undertake. Coalition members are supposed to coordinate their strategies in order to maximize their global welfare that, as just shown, coincides with the characteristic value of the same coalition (Zara et al., 2006b2006b). The strategies chosen by the players outside the coalition depend on the model assumptions. This topic will be discussed in the following section together with the first solution concept: the Core. Resuming what said in this section, an n-players game in coalitional form is a game defined by the pair (N, v) that focuses on the coalitions' outcome (characteristic value) rather than on the outcome achieved by single players.
3.2 The Core and Its Various Declinations: α, β and γ
In partial contradiction with what said before, the first solution concept that will be examined, the Core, is a set rather than a point solution. This deviation is due to the fact that the Core is a fundamental notion in CGT, it is useful to compute other point solutions and it is helpful in explaining the assumption regarding the non-members' behaviour in the IEA game. Assuming that v(N) is higher than every other v(S), it seems rational for the players of the game to form the grand coalition. The problem, in presence of transferable utility, becomes then to agree upon the amount that each player should receive (how to split the pie) (Ferguson, 2005). An imputation x = (x1, ..., xn) is a pay-off vector that defines the sum – the amount of utility – that each player should receive, if that imputation will be accepted. The Core (N,v) is defined as the set of all imputations that satisfies the following conditions:
- (1)

- (2)

The first condition is a simple consequence of rationality and states that the entire value of the grand coalition should be distributed among players (efficiency condition), whereas the second one is what really defines the Core. Basically, each player should get an amount at least equal to what she could get in any of the subcoalitions that she could form. In this way, no one has an incentive to leave the grand coalition. This second condition can be further divided into two parts. The first, quite obvious, says that every player must get more than what she could achieve playing alone: xi ⩾ v({i}) ∀i ∈ N. The second part, instead, includes also the other subcoalitions with two or more players and defines, through the condition ∑i ∈ Sxi ⩾ v(S), the stability of an imputation. In words, it can be said that the Core collects all the efficient imputations that satisfy stability (Ferguson, 2005). As anticipated, neither it considers any principle of equity or fairness nor it provides a clear indication on which imputation to prefer but still it discriminates between games that can support, on the ground of stability, the grand coalition and the ones that cannot. This last case happens when the Core is an empty set.
Recalling the definition of the characteristic value of a coalition S as v(S) = ∑i∈SΠi(φ1...φn), it is clear that its definition depends from the strategies adopted by its members as well as the ones undertaken by the players ‘outside’. Coalition members are supposed to act in order to maximize v(S) itself. It is then required to assume which is the behavioural pattern followed by the ‘outsiders’. Three such assumptions have emerged in the CGT literature. The first is the most pessimistic one, supposing that non-members will adopt the most detrimental strategy at their disposal in order to contrast S. Once this assumption is adopted, we will speak of α-characteristic function and α-Core. Therefore, v(S) will be defined by a maxmin principle, as to say, it is the maximum pay-off that a coalition can guarantee to itself knowing that non-members will act in order to minimize it:
The α-Core is simply the Core under this particular assumption. The β-characteristic function (and β-Core), instead, is obtained by adopting a minmax principle. In words, by assuming that the coalition can achieve the minimum among the maximum pay-offs that it is able to guarantee to itself after that the strategies of the players have been fixed (Zara et al., 2006a2006a):
The last Core concept, the γ-Core, has been developed by Tulkens and Chander (1997) specifically to deal with IEAs and other environmental games. The γ-characteristic function implies two main assumptions: first, that players remaining outside coalition S do not form any other coalition, so they act as singletons, and secondly that they do not take any particular action, neither to contrast nor to favour, the formed coalition. They behave neutrally, following self-interest in a rational way:

Outsiders (is ∈ N∖S), therefore, act in a competitive way both among each other and towards the formed coalition S, with the only aim of maximizing private utility.
3.2.1 Which Core is Appropriate for an IEA Game?
The α- and β-Cores, theorized by Aumann (1959), have been discussed in the early stage of development of the environmental CGT literature, but have been almost completely abandoned after the introduction of the γ-Core. Laffont (1977) has shown that, in a game characterized by an economy with detrimental externalities (such as environmental games), the α and β assumptions coincide. Maler (1989) has been the first to discuss the problem of these assumptions when applied to the environmental field. In its ‘Acid Rain’ game, he has hypothesized that there is no upper bound to the level of pollution that countries can produce. Since, as stated in the introduction, the strategy space of a country is given by the amount of pollution it will generate, this means that, under α and β assumptions, all the non-members will produce an infinite level of pollution. Even placing some kind of ‘technical’ upper bound to the level of pollution feasibly deliverable, this does not solve the conceptual problem of why non-members should actually adopt this strategy. Generating positive externalities, a coalition favours also outsiders, therefore it should be in their interest not to contrast its formation. Secondly, such a high level of pollution is detrimental, therefore irrational, also for themselves.
Tulkens and Chander (1997) have then envisaged a new type of Core concept, whose main assumption is the simple rationality of players. Instead of using their production economy, it is simpler to explain the idea behind it making use of the emissions' benefit and damage functions. Under this setting, the monetary utility [Πi] obtained by a country i is given by a benefit function [Bi( · )] having as argument own emissions [ei] – recall that emissions generate a benefit being a proxy for production and, consequently, consumption – minus a function [Di( · )] describing the environmental damage caused by pollution that, in presence of a global pollutant, will have as argument the sum of the emissions produced by all countries [∑iei]:

From basic optimality conditions, it is known that the pay-off of i is maximized when B′i = Di′. From what said till now, it becomes clear that the strategy space of i, under the sole assumption of rationality, becomes narrowly bounded till being a single value. It is possible to define the strategic choice of a country as a deterministic choice obtained by simply equating the first derivative of two known (by assumption) functions. Given that the damage function has the sum of all countries' emissions as its argument, this implicitly creates a strategic game that, in absence of any further assumption, takes the form of a Cournot game if the damage function is convex. The alternative would be a Stackelberg game in case a first move advantage is given to some players. In the dedicated literature, this assumption has been often used, guaranteeing the advantage to the coalition (Barrett, 1994; Diamantoudi and Sartzetakis, 2006; Sartzetakis and Strantza, 2013). However, it has been criticized as theoretically ungrounded by Finus (2008). It has to be noticed a strong convergence between the cooperative and the non-cooperative literature in representing the pay-off function of players. Generally, it is assumed a concave benefit and a convex damage function. The first complies with the non-satiety, but marginal declining satisfaction of consumption, that is standard in economic theory, whereas the second derives from environmental science according to which ecosystem resilience and absorption capacity suffer from saturation. The game so depicted has a single Nash equilibrium found as the solution of the maximization problem just described. Chander and Tulkens (1995) call it the disagreement point and it gives the characteristic value of the singletons' coalitions, v({i}), also called the reservation utility of players. Till here, CGT and NCGT do not show any difference.
Once players are allowed to form (or discuss the formation of) coalitions, they will act in the interest of the same coalition, if they are members, or in their private interest if non-members. Acting in the interest of the coalition translates in maximizing the sum of the pay-off functions of all its members:

The coalition will therefore act as a single entity and, in this role, will play the same game just described with all the other players, that, according to the γ assumption, will keep their rational, self-interested behaviour and will act as singletons. It must be noted that the γ assumption can be decomposed into two distinct assumptions, each related to one of the two (inter-connected) strategic spaces composing the IEA game. The first relates to the amount of pollution, the choice of the level of emissions, outsiders will undertake. The second regards their possibility to form one, or potentially more, competing coalitions other than the one currently existing. As said, this possibility is basically excluded. Whereas the first assumption is decisively justified, from a conceptual point of view, by rationality, the second, instead, appears more as a simplifying device.6 In fact, several papers, among which Eyckmans and Finus (2004), Buchner and Carraro (2005), Eyckmans et al. (2012) have dropped it, allowing for the coexistence of more than one coalition with several players. This would imply to switch from a characteristic to a partition function form game. This paper, however, will align with the vast majority of the literature on IEAs, both cooperative and non-cooperative, assuming that only a non-singleton coalition can be formed. A further point must be made. Recalling that a coalition always generate positive externalities, the γ assumption means that non-members, by standing as singletons, actually adopt the worst strategy at their disposal from the coalition point of view. As for the basic non-cooperative case, a unique Nash equilibrium will form (this has been called in Chander and Tulkens (1995) partial agreement Nash equilibrium – PANE). Another important property that follows from the structure of the game and from the γ assumption is that every change in the emissions level of one player will cause a partially offsetting reaction (best reply function) from the others (Finus, 2000). A way to avoid this is to use a quadratic benefit in combination with linear damage functions, for which there is no reply to a variation in the level of emissions of other players. However, this formulation misses to capture an important feature of the pollution problem: its increasing harmful effect.
Finally, it is possible to arrive at the distinctive feature between CGT and NCGT in their application to IEAs. Given that the γ assumption basically reproduces exactly the same behavioural pattern adopted by NCGT for non-members and considering that the way a coalition acts is also identical, the difference must be searched somewhere else. Tulkens and Chander (1997) have first tested two non-cooperative solution concepts: the strong Nash equilibrium and the coalition-proof Nash equilibrium. The first has been disregarded since it does not exist for this type of games, whereas the second, introduced by Bernheim et al. (1987), is actually the solution adopted by NCGT. Tulkens and Chander (1997) declared to be unsatisfied with this solution since it is suboptimal (not Pareto efficient) and since it implies that a deviation of a coalition (a set of members leaving a coalition) does not cause any reaction from the remaining members. As stated in the introduction, the main difference of the two approaches can be described as a matter of perspective. NCGT starts from the bottom, the disagreement point, and look at which coalition can be built, whereas CGT assumes the existence of the grand coalition and examines if there are incentives for leaving it. Anyway, this is not the end of the story. The reaction of the remaining members to a deviation of a coalition (as usual, in the CGT jargon, this means also a single player, a singleton) is also central in order to theoretically justify the feasibility of the cooperative approach. In fact, when they examine the incentive to leave the grand coalition, CGT adopters consider only the pay-offs that players can achieve forming sub-coalitions and the singletons' pay-offs are the ones obtained in the disagreement state. This means that the pay-offs achievable by being non-members (free riders, in the NCGT jargon) are simply disregarded. The justification of this strong limitation stays on the reaction of members to deviations that implies to break the coalition and to play the disagreement strategy (Tulkens and Chander, 1997). Much of the controversy between the two approaches has been focused on this assumption, with NCGT supporters claiming that this threat is not credible.
3.3 The Solution of Chander and Tulkens
The point solution proposed by Chander and Tulkens (1995) has two interesting and appealing properties. The first is that it lies in the Core, so that it preserves the individual rationality of cooperating. The second is that it uses the same elements of the countries' pay-off function (namely, the benefit and damage functions and their parameters) to define the imputation vector to be adopted. Each of its ith elements is composed by two parts: country i's pay-off obtained in the full cooperative case (S = N) plus a transfer: xi = Πi(e*i) + Ti, with e*i being the equilibrium level of emissions of country i participating to the grand coalition. The important part is constituted by the transfer Ti and the rule defining it:

where ![]() is the equilibrium level of emissions at the disagreement point. Since the sum of Ti over the is is equal to zero, it is easy to check that ∑ixi = ∑iΠ*i = v(N), so that the group rationality and efficiency condition is met. Explicitly writing the pay-off of a country when the grand coalition is implemented, Bi(e*i) − Di(∑ie*i), helps to easily understand what will be the final imputation received and the ratio behind this transfer scheme:
is the equilibrium level of emissions at the disagreement point. Since the sum of Ti over the is is equal to zero, it is easy to check that ∑ixi = ∑iΠ*i = v(N), so that the group rationality and efficiency condition is met. Explicitly writing the pay-off of a country when the grand coalition is implemented, Bi(e*i) − Di(∑ie*i), helps to easily understand what will be the final imputation received and the ratio behind this transfer scheme:

A country will then receive an amount equal to its benefit function valued at the disagreement point, so when its emissions and, consequently, the value of the same function, is maximum. To this, it is subtracted the value of the damage function with emissions as in the full cooperative case, so when it is the lowest. From what just said, it is clear that the term inside the square brackets is always negative. This term will then be subtracted proportionally to the magnitude of the parameter describing the importance of the environmental damage for a country compared to (divided by) the sum of the same parameter over all countries. In other words, the first, always positive, term is diminished in a way that is proportional to the vulnerability of a country to pollution. This is justified since pollutees need to pay polluters in order to induce them to cooperate by compensating them for their forgone benefits obtained by emitting. However, as explained in Chander and Tulkens (2006), this solution is actually favourable to pollutees. In fact, they will pay polluters just up to the point that will induce them to cooperate, but the actual surplus of cooperation is retained by the same pollutees.
3.4 The Shapley Value
The Shapley value (Shapley, 1953) is a point solution concept that has found some applications in the context of pollution problems, for example, in Botteon and Carraro (1997) and Petrosjan and Zaccour (2003). It can be considered part of a broad family of (both set and point) solution concepts that rely on the mechanism of objections and counter-objections well described in Osborne and Rubinstein (1994). This class of solutions, differently from the Core that poses only an ‘immediate’ feasibility constraint, considers the chain of events that the deviation of a coalition may trigger. Also in this case feasibility is the central aspect, but it is evaluated only on the ultimate outcome produced by a deviation (Osborne and Rubinstein, 1994). Parts of this category are: the Stable set, the Bargaining set, the Kernel, the Nucleolus and the Shapley value. The first, introduced by Von Neuman and Morgenstern (1947), will not be considered since it has never been applied to environmental problems. Furthermore, it is a superset of the Core (Osborne and Rubinstein, 1994) and not only, being a set solution, allows for multiple equilibria but, for a single game, there can be more Stable sets. The following three solutions are strongly interconnected being the first a superset of the second and this of the latter (Driessen, 2013). Only the Nucleolus, the sole point solution among the three, will be described specifically in the next section.
The mechanism of objections and counter-objections allows to define a stable state, obtained when they reciprocally nullify. At its base there is a mix of considerations about power relations and fairness. Different weights attributed to these elements give rise to the multiplicity of solution concepts mentioned. The Shapely value focuses primarily on the marginal contribution that a player brings to a coalition. The objections that a coalition member can claim to another player for a certain imputation are twofold. She can claim that, leaving the coalition, will cause a loss to that player greater, for this last, than accepting the alternative imputation she is proposing. Alternatively, she can object that there is the possibility for her and the other members to make a coalition without the accused player that will leave her better off and the remaining players at least as good as before. Basically, she can induce the others to exclude the contested player. A counter-objection is simply the same argumentation put forth by the accused player. An important consideration to be made is that the Shapley value considers at one time all the subgames present in a game. In other words, it requires that the objectioncounter-objection nullification holds for all the subgames. This last sentence expresses the balanced contributions property. In order for this property to hold, it is required to assign to each player a value ψ such that:
where (N∖{j}, vN∖{j}) and (N∖{i}, vN∖{i}) indicate the subgames of Γ(N, v) where players j and i are, respectively, excluded. The only value ψ that satisfies this condition is the Shapley value that, therefore, will be the imputation chosen (ψ = x) (Osborne and Rubinstein, 1994). The formula for calculating it is given by
where |S| indicates the cardinality of the coalition S. The term in the square brackets describes the contribution player i brings to the coalition S. The sum is used to consider the (marginal) contribution that a player provides to all the possible coalitions of a game (all the subgames). Finally, the expression preceding the square brackets is used to give a weight to each such contribution considering the probability a player has to actually ‘produce’ it. The denominator, in fact, is the number of all permutations of the n players, whereas the numerator expresses the number of these permutations in which the |S| members of S come first than player i ((|S| − 1)! ways), and then the remaining n − |S| players ((n − |S|)! ways). ψi is the average contribution brought by player i to the grand coalition if the players sequentially form this coalition in a random order (Ferguson, 2005). A possible extension of the Shapley value is to consider different probabilities in which coalitions can form. The random order just mentioned, in fact, implies to assume equal probabilities. In this case, we would speak of Weighted Shapley value. A literature review describing the various weighting schemes and computation devices adopted can be found in Kalai and Samet (1987).
Regarding the properties shown by the Shapley value, it has to be pointed that it is the only solution concept contemporaneously satisfying efficiency, symmetry, dummy axiom and additivity. According to Hoàng (2012), the satisfaction of all these properties is compensated by an important drawback since the Shapley value does not always fall into the Core. However, it does in convex games (Zara et al., 2006a2006). Applying this concept to IEA games leads to reward that countries having a high level of pollution. In fact, these are the ones whose inclusion in an agreement is most profitable from the global point of view. From another perspective, it could be said that the agreement would obtain scarce results without their presence. The solution of Chander and Tulkens takes into consideration this fact, but grants them only the sufficient benefits in order to make their participation rational. The surplus obtained from cooperation is given to polluted countries. With an imputation obtained through the Shapley value, instead, they have to give part of this surplus up to polluters.
3.5 The Nucleolus
The Nucleolus, introduced by Schmeidler (1969), is a point solution that, as anticipated, is contained both in the bargaining set and in the Kernel. Furthermore, when the Core is non-empty, it is also a subset of this last. In order to understand the mechanism of objections and counter-objections at its base, it is required to introduce the notion of the excess of S: e(S, x) = v(S) − x(S); where x(S) = ∑i ∈ Sxi (Osborne and Rubinstein, 1994). When e(S, x) is positive, it represents the amount that the coalition will loose if that imputation will be implemented. On the contrary, when negative, it constitutes the surplus that the coalition receives from that imputation. It is then possible to define an objection having as argument an imputation x and a coalition S with related excess e(S, x) to another imputation y if e(S, y) > e(S, x) (e.g. x(S) > y(S). A counter-objection is consistent if it does exist another coalition T for which e(T, x) > e(T, y) and e(T, x) ⩾ e(S, y). Compared to the Shapley value, it is possible to see that the Nucleolus uses coalitions as the main argument to make objections and counter-objections. Also in this case, the Nucleolus is defined as the equilibrium point where the two balance each other.
The other – actually the standard – way to define the Nucleolus is by saying that it individuates the imputations vector x for which the vector E(x) is lexicographically minimum (Osborne and Rubinstein, 1994). In order to understand this characterization, it is necessary to define the vector E(x) and the word ‘lexicographically’. Starting with an imputation vector x, it is possible to arrange the 2n − 2 coalitions' excesses in a non-increasing order. E(x) will then be the vector collecting this excesses: E(x) = el(S, x), l = 1, ..., 2n − 2. Now, consider an alternative imputation, y, and repeat the same operation creating E( y). It is then required to compare the first element (the one with the highest value, since they are ordered decreasingly) of the two vectors. The one having a lower value will be preferred. Once it is not possible to further minimize it, switch to the second element and continue till the last. The lower bound for minimizing the first element is actually given by the second one. In fact, when the first reaches this level, further minimizing it will cause it to move on the second place given that the E(x) vector must be ordered decreasingly. Therefore, as stated by Serrano (1999), the nucleolus maximizes recursively the pay-off of the worst-treated coalitions. The same author underlines that it can be interpreted as an application of the Rawlsian maximin principle (Rawls, 1971) applied to coalitions interpreted as independent subjects. The Nucleolus satisfies several properties that will be just mentioned. The first two are individual and group rationality. The third, being in the Core when this is not an empty set, is actually a proof in itself of the previous ones. The Nucleolus is unique (a point solution) and never empty. Finally, it satisfies consistency, covariance, anonymity and efficiency.
3.5.1 Computing the Nucleolus
The calculation of the Nucleolus requires a computational burdensome procedure even in presence of relatively simple ‘games’. In their presentation of an analytic procedure to compute it, Leng and Parlar (2010) provide a review of the various algorithms present in the literature to efficiently solve the linear programming (LP) system necessary to find it. Here, it will be simply presented the standard procedure without taking into consideration the problem of computational steps.
Recall the definition of the excess, e(S, x) = v(S) − ∑i ∈ Sxi, and remember that, excluding the empty and the grand coalition, there will be l = 2n − 2 excesses so that it is possible to write el(Sl, x). The Nucleolus is found by solving min xmax [e1(S1, x), ...el(Sl, x)]. A simple example, with three players, can help to further clarify the procedure. The coalitions' set will be Σ∖ Ø, N = (S1 = 1; S2 = 2; S3 = 3; S4 = 1, 2; S5 = 1, 3; S6 = 2, 3). The minimization problem will then be

This problem amounts to distribute the value v(N) among x1, x2 and x3 respecting the given conditions.
4. A Rawlsian Solution Concept Bounded by Individual Rationality
The aim of this section is to introduce an alternative solution concept that, although lying in the Core, fosters the redistribution of utility. In order to introduce it, two additional solution concepts will be shortly described.
4.1 The Strong ε-Core and the Least Core
The Strong ε-Core has been introduced by Shapley and Shubik (1966) as a way to find the Core even when this set is actually empty. They have shown that, for an appropriate value of ε ∈ ℜ there will always be an imputation lying in the Core. The Strong ε-Core can be defined as:
It can be seen that, when ε is positive and large enough, even a game with an empty Core will admit at least one element in this set. The value of ε can be interpreted as a penalty that members should pay in order to leave the grand coalition. Instead of thinking at ε as a value exogenously given, it is possible to interpret it as a variable to be minimized (Bilbao, 2000). This amounts to solve the following system of equations:

Its solution, that requires to find both the imputation vector and ε, being both variables, gives the Least Core. This is a point solution with redistributive properties similar to the Nucleolus. In order to understand why, let us hypothesize to have a game with non-empty Core. Furthermore, consider two coalitions with an equal number of members. One is ‘weak’, meaning that it has a low characteristic value, whereas the other is ‘strong’. In the minimization process, as said, both ε and the imputation vector will be defined. This means that, in order to have the lowest possible ε, the imputations of the members of the ‘weak’ coalition will be prioritized. Therefore, although the procedure to find (and the idea behind) the two solution concepts are quite different, the Least Core and the Nucleolus will give an imputation vector with similar characteristics.
4.2 The Minimum Feasible Core (MF Core)
The system of equations that is used to find the Least Core can be slightly changed to find another useful concept: the MF Core. This is not an interesting solution in itself, since it is not efficient, but can be used to define the pure surplus generated by cooperation once the individual rationality constraint has been satisfied. Consider the following system of equations:

Although very similar to the program defining the Least Core, two crucial modifications have been applied: the position of the variable and its maximization rather than minimization. Basically, this solution tells which is the minimum characteristic value that the grand coalition must have in order to sustain full cooperation or, in order for the Core to be non-empty. This value is simply found as v(N) − η. The value of η can therefore be interpreted as the pure surplus (if positive) of cooperation, whereas the associated imputation vector as the minimum amount that each player should receive in order not to leave the grand coalition. A negative η, such as a positive ε in the previous case, indicates that the game has an empty Core.
A final example can help to understand this mechanism. Let us think that each coalition S ≠ N is represented by an empty bottle. The bottles can have different dimensions and their volume is given by v(S). The owners of a bottle are the players member of that coalition. The grand coalition, instead, is a barrel having an amount of liquid equal to v(N). Now, under the assumption that one unit of liquid corresponds to a unit of volume, we need to give a certain amount of it to each player (defining an imputation) in order to fill up all the bottles. When a player is given a unit of liquid, this will contribute to fill in one unit of volume of all the bottles owned by her. Therefore, three conditions are possible. One is that there is not enough liquid to fill up all the bottles, one is that the liquid is exactly enough to do it and, finally, the last corresponds to have some spear liquid. Finding the MF Core tells us which would be the imputation in the middle case (how much liquid each player should receive), and, through the value of η, how much liquid we lack to reach this point (negative η) or how much there is in excess (positive η).
4.3 A ‘Revisited’ Nash Bargaining Solution and the Rawlsian Nucleolus
Remembering what said about the Nucleolus, this solution concept can be considered as a way to implement the Rawlsian maximin principle. This is actually true, but the potential flaw of this method in representing this principle is to consider coalitions as subjects. In reality, it does not really make sense to speak of the welfare of a coalition with two or more players. Welfare is an attribute of players alone. A redistributive principle should have them, and only them, as the main target. Before presenting a modified version of the Nucleolus that takes into consideration this aspect, it is opportune to further discuss the MF Core.
Remembering what said in the introduction, a solution concept is made of two parts. From one side, it has to satisfy power relations assuring individual (and group) rationality. From the other, it has to provide a fair and equitable division, therefore, it is required to possess such a criterion of fairness and equity imbued on it. The MF Core, however, allows to completely separate the two aspects given that it provides the minimum sufficient condition to satisfy the first requirement. Basically, after that the MF Core imputation vector has been established (since now on it will be identified as xη), a new game can be thought regarding the way of dividing the surplus η. A first obvious solution would be to divide it in equal parts so that the final imputation would be ![]() . This allocation sounds very appealing specially considering, as suggested, the splitting of the cooperative surplus as a cooperative bargaining problem whose starting condition is the imputation vector xη. This assumption would mean that the reservation utility of each country is: r({i}) = xηi. Therefore, it is easy to check that
. This allocation sounds very appealing specially considering, as suggested, the splitting of the cooperative surplus as a cooperative bargaining problem whose starting condition is the imputation vector xη. This assumption would mean that the reservation utility of each country is: r({i}) = xηi. Therefore, it is easy to check that ![]() is the allocation that maximizes the Nash Bargaining solution:
is the allocation that maximizes the Nash Bargaining solution: ![]() . Compared to the ‘classical’ Nash Bargaining solution, the difference stays in the alternative reservation utilized: the utility obtained in the disagreement point has been substituted by the MF Core imputation. Therefore, this solution is named ‘revisited’ Nash Bargaining solution. The fairness of this allocation, however, can be questioned. Splitting equally the surplus of cooperation, in fact, is surely equitable only if the ‘power game’ determining the MF Core imputation vector is taken for granted. In other words, the power asymmetries at its root are considered natural and are fully justified on a moral base. Redistribution, therefore, does not find any valid reason for being implemented.
. Compared to the ‘classical’ Nash Bargaining solution, the difference stays in the alternative reservation utilized: the utility obtained in the disagreement point has been substituted by the MF Core imputation. Therefore, this solution is named ‘revisited’ Nash Bargaining solution. The fairness of this allocation, however, can be questioned. Splitting equally the surplus of cooperation, in fact, is surely equitable only if the ‘power game’ determining the MF Core imputation vector is taken for granted. In other words, the power asymmetries at its root are considered natural and are fully justified on a moral base. Redistribution, therefore, does not find any valid reason for being implemented.
In the ‘global warming game’, however, this point of view can hardly be sustained. Indeed, it would imply to accept and to morally justify the fact that countries are affected differently from climate change on the simple base of their geographical position and that the most affected ones have to pay by themselves for this disadvantage. Furthermore, it also means to justify GDP inequalities and to wipe away the historical dimension of the pollution problem. Given the strong association between GDP level and the world share of cumulative emissions, measured in terms of CO2 ppm, this last element is the most difficult to accept (Shukla, 1999). A redistributive policy, in the IEA context, appears therefore an appropriate choice. However, unless introducing an altruistic attitude of countries that modifies their pay-off function, as done, for example, in Lange and Vogt (2003) and Grüning and Peters (2010), the postulate of self-interest imposes a strong lower bound on the amount that can be feasibly redistributed. This bound, as stated, is simply the MF Core imputation vector. The surplus, however, can be freely – meaning, without affecting the cooperative outcome – allocated in order to, at least partially, the starting asymmetries. The problem to be solved will then be:
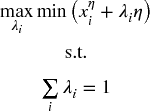
where min (xηi + λiη) = min (xη1 + λ1η, ..., xηn + λnη). Regarding the relation with the Nucleolus, the present solution can be considered as a modification of that concept in order to base its redistributive properties only on the singletons coalitions. Once solved for λi the given maximization problem, the final imputation vector will be: xRN = xηi + λiη, where the superscript ‘RN’ stays for Rawlsian Nucleolus, the chosen name for this solution concept.
5. A Numerical Comparison of Solution Concepts
The aim of this section is to provide a comparison of the countries' utility achieved in a standard IEA cooperative game applying the different solution concepts previously discussed: the Chander and Tulkens solution, the Shapley value, the Nucleolus, the Least Core, the revisited Nash Bargaining solution and the Rawlsian Nucleolus. The model used to perform this comparison is a standard economic–environmental model with a quadratic concave emissions benefit and a quadratic convex damage functions: ![]() ;
; ![]() , with E = ∑iei; i = 1, ..., n. As in Chander and Tulkens (1995), it will be adopted a γ-characteristic function, therefore, when a coalition form, the other members are supposed to pursue, as singletons, their self-interest. A country welfare function is given by
, with E = ∑iei; i = 1, ..., n. As in Chander and Tulkens (1995), it will be adopted a γ-characteristic function, therefore, when a coalition form, the other members are supposed to pursue, as singletons, their self-interest. A country welfare function is given by
The model, under the assumption of symmetric countries (identical parameters), can be easily solved analytically. For convenience, three cases are treated separately: the Nash equilibrium (all countries act as singletons), the partial Nash equilibrium (PANE) and the full cooperation case (grand coalition). Solving for emissions in the three cases gives



In the PANE case esi and ensi stay, respectively, for the emissions of a signatory and a non-signatory, |S| indicates the number of coalition members and |N| the total number of players.7 By plugging these values in the pay-off functions, it is possible to find the welfare of each country and, consequently, the characteristic value of the coalitions.
At the beginning of the previous chapter, two properties of characteristic functions have been mentioned: convexity and superadditivity. The importance of the first stems from the Bondareva–Shapley theorem, that establishes a sufficient and necessary condition for a game to have a non-empty Core: balancedness. A game is balanced when:
The collection of weights (ρS)S ∈ Σ is a vector of scalars in [0,1] with dimension 2n and it is balanced if, for every player i, the sum of it over all the coalitions that include i −2n − 1 coalitions – is equal to one: ∑S ∈ Σ, S∋iρS = 1 (Osborne and Rubinstein, 1994). Convex games are known to be always balanced, therefore they have a non-empty core (Dubey and Shapley, 1984). Superadditivity, instead, only guarantees that the characteristic value of the grand coalition is not lower than the characteristic value of any other subcoalition: v(N) ⩾ v(S), ∀ S⊂N. Recalling what said regarding the efficiency of an allocation referred to a public good, that is maximized when all the interested parties are included in its definition (Samuelson, 1954), it follows that a coalition should always get a benefit by expanding its membership. Superadditivity captures this, but it is not a sufficient condition to proof balancedness, therefore the Core might be empty.
Dubey and Shapley (1984) have proved balancedness for certain classes of not convex games, among which the production market game, the pure exchange market game and the transshipment game. Upon the introduction of certain mild restrictions – all the parameters (a, b and d) have positive values and no player generates a negative utility in any possible coalition – the present model, although not necessarily convex, always has a non-empty Core. The last claim has been proved by Helm (2001), whereas a simple numerical example, with identical players, is provided in Appendix A.1 in order to show that convexity is not a necessary property of the game. Superadditivity, instead, it is (proof is given in Appendix A.2).
A final remark is related to the restrictions on parameter values. Their strict positivity does not really need a justification since it is a necessary condition for the concavity of the benefit function and the convexity of the damage function to hold. Setting their values in order that no player in any coalition obtains a negative utility could appear more arbitrary. However, it seems quite natural that, even in the disagreement point, countries still enjoy a positive utility. Once this condition is assured – for the case of identical players it requires that ![]() – superadditivity implies that no player can be worst off in any coalition S (|S| ⩾ 2) than in the disagreement point. Furthermore, since a coalition generates positive externalities, also non-members cannot experience a reduction in their level of utility. Therefore, setting appropriate values for non-negative utilities in the disagreement point is sufficient to guarantee strictly positive utilities in all other cases. An analytic proof of this claim, for identical players, is given in Appendix A.3.
– superadditivity implies that no player can be worst off in any coalition S (|S| ⩾ 2) than in the disagreement point. Furthermore, since a coalition generates positive externalities, also non-members cannot experience a reduction in their level of utility. Therefore, setting appropriate values for non-negative utilities in the disagreement point is sufficient to guarantee strictly positive utilities in all other cases. An analytic proof of this claim, for identical players, is given in Appendix A.3.
5.1 Characteristic Values with Asymmetric Countries
The scenario with symmetric countries is not really interesting given the purpose of comparing the mentioned solution concepts. It can be checked, in fact, that, in this case, the imputation vector obtained and, therefore, countries' welfare, would be identical for all the solutions adopted. In order to give a touch of realism to the model while keeping its interpretation as simple as possible, only five countries will be considered and the parameters to vary are b and d. The first, that describes the magnitude of the marginal decrease of emissions benefits, is fundamental to determine the optimal level of the same emissions. For a given level of emissions, in fact, its magnitude is inversely correlated with the final utility achieved. It is therefore used to simulate the wealth, or the technological level, of a country. Three values will be used: High Wealth (HW) = 0.01, Medium Wealth (MW) = 0.02 and Low Wealth (LW) = 0.028. The parameter a, instead, will be kept equal for all countries and will be equal to 8. The other parameter to vary, d, represents the degree a country is affected by pollution. A higher value implies that a country is more vulnerable to the detrimental effects of climate change. Also in this case three levels will be adopted: high (HD = 0.0024), medium (MD = 0.00225) and low (LD = 0.002) vulnerability. Combining them, five types of countries are simulated. They are shown in decreasing order of ‘power endowment’:
- High Wealth – Low Damage HWLD: a = 8; b = 0.01; d = 0.002;
- High Wealth – High Damage HWHD: a = 8; b = 0.01; d = 0.0024;
- Medium Wealth – Medium Damage MWMD: a = 8; b = 0.02; d = 0.00225;
- Low Wealth – Low Damage LWLD: a = 8; b = 0.028; d = 0.002;
- Low Wealth – High Damage LWHD: a = 8; b = 0.028; d = 0.0024
5.2 A Comparison of Distributive Properties
In Table 1, it is possible to see the imputation vectors obtained with the different solution concepts. As said, each imputation corresponds to the final utility obtained by a country. The first two columns, in reality, show how pay-offs will be distributed in the Nash equilibrium (disagreement point) and in the grand coalition without any transfer scheme. The row displaying the summation of utilities testifies the benefit provided by cooperation. For a comparison between the characteristic value of the grand coalition and all the other partial coalitions, see Table B.1 in Appendix. It should also be noticed that the distribution obtained without transfer is actually the most egalitarian: the product of utilities is the highest compared with the one obtained from any other solution concept. However, this imputation does not satisfy the boundaries imposed by the MF Core, with the first two countries obtaining a lower value.
Table 1. Imputation Vectors from Different Solution Concepts.
| Countries | Nash Equilibrium | No Redistribution | Shapley Value |
| HWLD | 310.7 | 312.6 | 331.5 |
| HWHD | 292.3 | 302.7 | 314.2 |
| MWMD | 100.6 | 125.3 | 118.7 |
| LWLD | 54.9 | 79.8 | 70.8 |
| LWHD | 37.1 | 69.9 | 55.1 |
| Utility Sum. | 795.5 | 890.4 | 890.4 |
| Utility Prod. | 1.86E+010 | 6.62E+010 | 4.83E+010 |
| Countries | Least Core | CT solution | Nucleolus |
| HWLD | 329.6 | 327.8 | 329.6 |
| HWHD | 311.2 | 312.9 | 311.2 |
| MWMD | 119.5 | 119.9 | 119.6 |
| LWLD | 73.9 | 72.1 | 73.9 |
| LWHD | 56.1 | 57.7 | 56.1 |
| Utility Sum. | 890.4 | 890.4 | 890.4 |
| Utility Prod. | 5.08E+010 | 5.12E+010 | 5.08E+010 |
| Countries | MF Core | Rawlsian Nucleolus | ‘R’ Nash Barg. |
| HWLD | 326.8 | 326.8 | 334.3 |
| HWHD | 311.2 | 311.2 | 318.6 |
| MWMD | 110.1 | 110.1 | 117.5 |
| LWLD | 58.9 | 71.2 | 66.4 |
| LWHD | 46.2 | 71.2 | 53.6 |
| Utility Sum. | 853.2 | 890.4 | 890.4 |
| Utility Prod. | 3.05E+010 | 5.67E+010 | 4.46E+010 |
Comparing the various solution concepts, it can be first noticed that they are all efficient since the summation over all imputations is equal to the characteristic value of the grand coalition. The only one failing is the MF Core, but it has already been explained that this solution is not useful in itself. Furthermore, they all lie in the Core. The similarity between the Least Core and the Nucleolus is confirmed till the point that, with only one decimal number displayed, they appear identical. Regarding their (re)distributive properties, the Rawlsian Nucleolus is the one that advantages the most the ‘weakest’ countries and maximizes the product of utilities. The imputation having the opposite effect is not the one obtained with the Shapley value, as could have been expected, but the one realized through the revisited Nash Bargaining solution. Dividing the cooperative surplus in equal parts advantages strong players beyond the value of their marginal contribution over all coalitions.
An interesting comparison can be done between the CT solution and the Rawlsian Nucleolus. Chander and Tulkens (2006) affirm that their solution is the most favourable possible for pollutees. However, its redistributive properties are lower than the ones of the Rawlsian Nucleolus. One can suspect that the difference stays in the fact that this numerical example portrays differences both in the environmental damage parameter and in the emissions benefit one. This aspect, however, is not fundamental. Even allowing for symmetric benefit functions, the obtained imputation vector is different.8 This is due to the fact that in the CT solution pollutees are required to compensate polluters for their forgone emissions benefit. This is the lower bound, whereas the Rawlsian Nucleolus uses the MF Core as lower bound. The due compensation is reduced by the increase in the utility that also polluters enjoy thanks to a better environmental quality. However, what Chander and Tulkens claim is actually true. Furthermore, it is also appropriate their claim that this solution concept correspond to the polluters pay rule. However, another principle discussed during the Kyoto negotiation for dividing the burden of contrasting climate change refers to the concept of capacity, strictly related to the one of vulnerability (Heyward, 2007). The damage caused by pollution differs not only according to some physical properties such as the geographical position of a country, but also given its ability to take counteractive measures (resilience). This is likely to be positively correlated with the economic condition of a country. Unless the damage parameter already takes this into consideration, the Rawlsian Nucleolus appears to better address the vulnerability problem.
5.3 A Comparison of Incentives to, and Potential Losses from, Free Riding
This section will continue the comparison of solution concepts adopting a more non-cooperative perspective. In particular, they will be evaluated in light of their ability to prevent the damages from internal free riding. This last concept is different from the usual meaning that takes in the non-cooperative literature, where it is considered as the practice of non-participating to an environmental agreement benefiting from the positive externalities generated by a coalition. In this case, instead, the participation to the grand coalition is taken as granted. However, a country can decide to cheat and to re-optimize its emissions' level taking the optimal level (from the collective point of view) adopted by the other countries as given. In choosing how much to emit, an internal free rider will face the following maximization problem:
The subscript fr indicates the free rider and Π+fr is the pay-off obtained through the re-optimization. From the sum of all optimal emissions E*, it is subtracted the share produced by the same free rider, e*fr, that will now substitute it with the result obtained from the re-optimization problem, efr. Obviously, this level will be higher and will be found through the usual optimality condition: B′fr = Dfr′(E* − e*fr). In order to free ride, instead of simply leaving the grand coalition, a free rider must still obey to the transfer scheme adopted. Although it has not been provided any formula defining a transfer for the solutions other than the one suggested by Chander and Tulkens, this is easily found: Ti = xi − Πi*. This holds for all solution concepts and it is trivial to show that the final utility obtained by a country is equal to the imputation itself: Πi = Πi* + Ti = Πi* + (xi − Πi*) = xi. In presence of free riding, however, this pay-off is modified in the following way:

The re-optimization problem faced by a free rider and its solution are independent from the imputation adopted. Moreover, the utility gain obtained by free riding is also independent from the solution concept adopted. In order to see this, just recall the definition of the final free rider pay-off given above: Π+fr + (xfr − Π*fr). Its positive deviation from the utility that she would receive by respecting the rules, equal, as shown, to the same imputation, is given by: Π+fr + (xfr − Π*fr) − xfr = Π+fr − Πfr*. The imputations cancel out and what is left is a constant. The same holds for the loss suffered when it is another country to free ride. Table B.2 in Appendix displays all the countries' pay-offs for the different solution concepts in presence of free riding. Table 2, instead, shows the gains – on the main diagonal – and the losses – on all the other cells, obtained and suffered when the country displayed on the left column free ride. As said, this table is the same for every solution concept adopted. What changes, instead, is the ratio of the gains and losses over the utility achieved in complying with the coalition rules. In order to obtain this, it is simply necessary to divide each row of the previous table by the same imputation vector (through a cell by cell, not a matrix division). Table B.3 in Appendix displays all the coefficients so found. The values on the main diagonal can be interpreted as an index of the incentive a country has to free ride. In fact, if utility is measured in terms of GDP, this would translate in the percentage (after having been multiplied by 100) of GDP a country could obtain from a cheating behaviour. Obviously, the more favourable an imputation is to this country, the less significant the potential gain will be. Furthermore, this index, being built as a ratio of potential gains to a reference utility level, is not affected, in its representation of incentives, from the magnitude of the sole gains or from the starting conditions of a country. Proportionality should assure a balanced picture. On the other side, there are the remaining values of the described matrix. These represent the percentage loss a country would face in case another free ride. It is then a measure of risk in participating to a coalition with a given imputation vector. The more favourable is an imputation to this country, the lower will be the suffered damage (again, in terms of GDP percentage). It can be noticed that imputation vectors that favour wealthy nations reduce the risk that they will free ride. However, they also increase the damages suffered by other countries in case they will free ride. The opposite hold for imputations favouring weak countries. These last will be less tempted to free ride, but the avoided risk, at global level, will be less significant since the damage that they can inflict is lower. Finally, they will be less affected from deviations from wealthy notions that, however, will be more likely.
Table 2. Gains and Losses from Free Riding.
| Free Rider | HWLD | HWHD | MWMD | LWLD | LWHD |
| HWLD | 24.75 |
−13.85 |
−12.98 |
−11.54 |
−13.85 |
| HWHD | −10.95 |
22.50 |
−12.32 |
−10.95 |
−13.14 |
| MWMD | −5.52 |
−6.62 |
11.83 |
−5.52 |
−6.62 |
| LWLD | −4.05 |
−4.86 |
−4.55 |
8.98 |
−4.86 |
| LWHD | −3.86 |
−4.63 |
−4.34 |
−3.86 |
8.19 |
Note: Numbers in bold individuate the values relative to the player that is free riding.
The problem with such a matrix is that it does not give a clear and immediate touchstone for comparing solution concepts. What is required is a single index able to measure the overall risk caused by free riding when a given imputation is implemented. This single index can be built in the following way. In order to show the necessary steps, the index coefficients table related to the Shapley value (Table B.3) will be taken as an example. Let us write the transpose of it in matrix form:

The element in the main diagonal, the incentive indexes, are extracted in order to form a vector h, keeping the same vertical order:

Multiplying A, with the diagonal elements substituted by zeros, with h gives the vector q representing the overall risk faced by each country. In fact, each row of A displays the potential loss suffered by a country when each of the others free ride. The matrix multiplication with vector h weights the potential loss caused by a country deviation with the incentive that this country has to actually deviate. It can be contested that the vector h is used here as a measure of probability although it is actually far from being so. This critics is effectively reasonable. However, such a probability measure would be impossible to build, specially in this simple model setting. The magnitude of potential gains from free riding is therefore chosen as a second best, although with consciousness about its limitations. Once obtained the vector q, the final synthetic index is given by the summation of all its elements: q × i′ (where i' is a vector of ones having same length as q). Basically, the overall potential loss caused by free riding in a given coalition for a given imputation vector is given by the sum of the same potential losses faced by each country. Table 3 reports the built indexes for each solution concept.
Table 3. Overall Free Riding Potential Loss Index.
| Shapley value | −0.140809 |
| Chander and Tulkens solution | −0.136577 |
| Least Core | −0.137407 |
| Nucleolus | −0.137424 |
| Rawlsian Nucleolus | −0.127944 |
| ‘R’ Nash Barg. | −0.146289 |
From Table 3, it can be seen that, although the more redistributive solution concepts foster the incentive to free ride of wealthy nations, whose deviation is the most detrimental, this is more than compensated by the higher imputations attributed to the other countries. The final index of free riding potential losses is the lowest for the Rawlsian Nucleolus, followed by the Chander and Tulkens solution. This last is closely followed by the Nucleolus and the Least Core, again almost identical. Finally, the Shapley value and the revisited Nash Bargaining. This classification mirrors exactly the one representing the distributive properties of solution concepts. The fact that redistribution minimizes potential free riding losses can appear counter-intuitive. On this regard it has to be noticed that it is not the absolute value of the losses to be minimized (as seen, this is constant), but the proportion each country will loose compared to its starting pay-off. If it was the absolute value of the losses to be weighted by the pseudo measure of probability of free riding, the result would have been different. However, this index appears to be justified since it can be seen as a representation of the potential losses in terms of GDP percentages. The focus is on each country and on its relative wealth, rather than in the overall value of the loss. The potential contrast with the cooperative perspective, more focused on global wealth, is settled by the fact that, when examining the risk of free riding, each country evaluates it on the base of its own potential losses.
6. Conclusions
This paper has offered an overview of the most popular solution concepts derived from CGT that have found an application in the environmental field. In particular, the focus has been placed on a specific sub-topic, namely, the constitution of an IEA to control the emissions of pollutants. After having briefly revised some fundamental concepts of CGT and having characterized the specificities of the game theoretical framework underpinning an IEA, the differences between the cooperative and the NCGT approach have been examined concluding that the perspective from which they look at the problem is the main point of departure. The other important element differentiating them is the way in which coalition members reply to a deviation from a cooperative behaviour of one of them.
The solution concepts taken into consideration have been the Core, together with its refinements: the Strong ε-Core and the Least Core. Moreover, solution concepts based on the idea of objections and counter-objections have been discussed: the Shapley value and the Nucleolus. An important solution in the game theoretical field of environmental economics, namely, the Chander and Tulkens solution, has also been examined. Finally, two alternative concepts have been proposed: the Rawlsian Nucleolus and a revisited Nash Bargaining solution, both based on the idea of the MF Core. The Rawlsian Nucleolus has been named in this way for its redistributive properties that favour the most disadvantaged, whereas the latter solution split the cooperative gain in equal shares among the cooperating parts. The difference between the solution proposed originally by Nash is that the reservation utilities applied here are the ones obtained through the MF Core.
The last part of the paper has been dedicated to a numerical exercise based on a standard game of emissions optimization in order to compare the properties of the mentioned solution concepts in terms of welfare distribution and ability to minimize the potential damages of internal free riding. The conclusion of the first analysis has shown that the Rawlsian Nucleolus is actually the most beneficial solution for poor countries largely affected by the detrimental consequences of climate change. The redistribution obtained through this method goes beyond the one achieved by the CT solution since the surplus is assigned on the base of final utility – prioritizing countries with the lowest – and it has the MF Core imputation as lower bound rather than emissions benefit in the disagreement point. Whereas the latter concept can be identified with the principle of polluters pay, the first further includes the criterion of vulnerability. However, it has to be underlined that such concept, if applied in a real context, could over-represent the entitlements of poor countries simply given their low economic level. This would cause a detachment from the pure environmental field and could undermine the acceptability of this solution. The proposed revisited Nash Bargaining solution, instead, rewards wealthy nations, therefore high polluters, even more than the Shapley value.
The last part tries to introduce an element of non-cooperative game theory in the cooperative perspective underpinning the paper: free riding. The link between the two approaches has been a theme largely debated, specially in the environmental context. After more than 20 years a satisfactory solution has yet to come. This paper, therefore, does not pretend to achieve such goal. However, it offers an index, based on potential losses measured in terms of utility (GDP) proportions, that can be used as a preliminary instrument to evaluate the intrinsic free riding risk of losses present in a coalition for a given imputation vector. The ranking of the examined solution concepts under this regard mirrors exactly what emerged for the redistributive properties. The more a solution concept redistributes wealth, the lower will be the overall risk of losses due to free riding.
Notes
References
- Aumann, R.J. (1959) Acceptable points in general cooperative n-person games. Contributions to the Theory of Games 4: 287–324.
- Barrett, S. (1994) Self-enforcing international environmental agreements. Oxford Economic Papers 46: 878–894. Available at: http://www.jstor.org/stable/2663505 (Last accessed 15 April 2016).
- Bernheim, B.D., Peleg, B. and Whinston, M.D. (1987) Coalition-proof Nash equilibria I. Concepts. Journal of Economic Theory 42(1): 1–12.
- Bilbao, J.M. (2000) Cooperative Games on Combinatorial Structures. Dordrecht, The Netherlands: Kluwer Academic.
- Botteon, M. and Carraro, C. (1997) Environmental coalitions with heterogeneous countries: burden-sharing and carbon leakage. Fondazione Eni Enrico Mattei Working Paper (24.98). Available at: http://www.ekf.vsb.cz/export/sites/ekf/projekty/cs/weby/esf-0116/databaze-prispevku/1998/NDL1998-024_CC_coalition.pdf (Last accessed 15 April 2016).
- Buchner, B.K. and Carraro, C. (2005) Regional and sub-global climate blocs. A game-theoretic perspective on bottom-up climate regimes. Available at: http://papers.ssrn.com/sol3/papers.cfm?abstract_id=774144.
- Carraro, C. and Siniscalco, D. (1993) Strategies for the international protection of the environment. Journal of Public Economics 52(3): 309–328.
- Chander, P. and Tulkens, H. (1995) A core-theoretic solution for the design of cooperative agreements on transfrontier pollution. International Tax and Public Finance 2(2): 279–293.
- Chander, P. and Tulkens, H. (2006) Cooperation, stability and self-enforcement in international environmental agreements: a conceptual discussion. SCAPE Policy Research Working Paper Series 0609, National University of Singapore, Department of Economics, SCAPE. Available at: http://ideas.repec.org/p/sca/scaewp/0609.html (Last accessed 15 April 2016).
- Coase, R.H. (1960) The problem of social cost. Journal of Law and Economics 3: 1.
- d'Aspremont, C., Jacquemin, A., Gabszewicz, J.J. and Weymark, J.A. (1983) On the stability of collusive price leadership. Canadian Journal of Economics 14(1): 17–25.
- Diamantoudi, E. and Sartzetakis, E.S. (2002) International environmental agreements—the role of foresight. Technical Report. Available at: https://ideas.repec.org/p/aah/aarhec/2002-10.html (Last accessed 15 April 2016).
- Diamantoudi, E. and Sartzetakis, E.S. (2006) Stable international environmental agreements: an analytical approach. Journal of Public Economic Theory 8(2): 247–263.
- Driessen, T.S.H. (2013) Cooperative Games, Solutions and Applications, Vol. 3. Springer Science and Business Media, Berlin.
- Dubey, P. and Shapley, L.S. (1984) Totally balanced games arising from controlled programming problems. Mathematical Programming 29(3): 245–267.
- Eyckmans, J. and Finus, M. (2004) An almost ideal sharing scheme for coalition games with externalities. Available at: http://papers.ssrn.com/sol3/papers.cfm?abstract_id=643641 (Last accessed 15 April 2016).
- Eyckmans, J., Finus, M. and Mallozzi, L. (2012) A new class of welfare maximizing sharing rules for partition function games with externalities. Bath Economics Research Paper 6. Available at: http://opus.bath.ac.uk/32513/1/06_12.pdf (Last accessed 15 April 2016).
- Ferguson, T. (2005) Game theory: games in coalitional form. Available at: Game theory: Games in coalitional form.
- Finus, M. (2000). Game Theory and International Environmental Co-Operation: A Survey with an Application to the Kyoto-Protocol. Fondazione Eni Enrico Mattei. Available at: http://users.uom.gr/esartz/teaching/genvecon/IEAsurvey(Finus).pdf (Last accessed 15 April 2016).
- Finus, M. (2008) Game theoretic research on the design of international environmental agreements: insights, critical remarks, and future challenges. Available at: http://dspace.stir.ac.uk/bitstream/1893/1099/1/finus08,%20International%20Review.pdf (Last accessed 15 April 2016).
- Germain, M., Tulkens, H. and Magnus, A. (2010) Dynamic core-theoretic cooperation in a two-dimensional international environmental model. Mathematical Social Sciences 59(2): 208–226.
- Grüning, C. and Peters, W. (2010) Can justice and fairness enlarge international environmental agreements? Games 1(2): 137–158.
- Helm, C. (2001) On the existence of a cooperative solution for a coalitional game with externalities. International Journal of Game Theory 30(1): 141–146.
- Heyward, M. (2007) Equity and international climate change negotiations: a matter of perspective. Climate Policy 7(6): 518–534.
- Hoàng, N.-D. (2012) Part of the series Operations Research Proceedings. Algorithmic Cost Allocation Games: Theory and Applications (pp 599-604). Berlin: Springer Berlin Heidelberg.
- Kalai, E. and Samet, D. (1987) On weighted Shapley values. International Journal of Game Theory 16(3): 205–222.
- Köke, S. and Lange, A. (2013) Negotiating environmental agreements under ratification uncertainty. Beiträge zur Jahrestagung des Vereins für Socialpolitik 2013: Wettbewerbspolitik und Regulierung in einer globalen Wirtschaftsordnung Session: Climate Policy III, No. E04-V2. Available at: http://www.econstor.eu/handle/10419/79952 (Last accessed 15 April 2016).
- Laffont, J.-J. (1977) Effets Externes et Théorie économique, Vol. 13. Éditions du Centre national de la recherche scientifique.
- Lange, A. and Vogt, C. (2003) Cooperation in international environmental negotiations due to a preference for equity. Journal of Public Economics 87(9): 2049–2067.
- Leng, M. and Parlar, M. (2010) Analytic solution for the nucleolus of a three-player cooperative game. Naval Research Logistics 57(7): 667–672.
- Maler, K.-G. (1989) The acid rain game. Studies in Environmental Science 36: 231–252.
- McEvoy, D.M. and Stranlund, J.K. (2009) Self-enforcing international environmental agreements with costly monitoring for compliance. Environmental and Resource Economics 42(4): 491–508.
- Osborne, M.J. and Rubinstein, A. (1994) A Course in Game Theory. Cambridge: MIT Press.
- Perman, R., Ma, Y., McGilvray, J. and Common, M. (2003) Natural Resource and Environmental Economics, 3rd edn. Harlow: Pearson Education.
- Petrosjan, L. and Zaccour, G. (2003) Time-consistent Shapley value allocation of pollution cost reduction. Journal of Economic Dynamics and Control 27(3): 381–398.
- Rawls, J. (1971) A Theory of Justice. Cambridge, MA: Harvard University.
- Samuelson, P.A. (1954) The pure theory of public expenditure. Review of Economics and Statistics 36(4): 387–389.
- Sartzetakis, E.S. and Strantza, S. (2013) International environmental agreements: an emission choice model with abatement technology. Technical Report 5/2013, University of Macedonia. Available at: http://maecon.uom.gr/wp-content/uploads/2013/09/dp052013.pdf (Last accessed 15 April 2016).
- Schmeidler, D. (1969) The nucleolus of a characteristic function game. SIAM Journal on Applied Mathematics 17(6): 1163–1170. Available at: http://epubs.siam.org/doi/abs/10.1137/0117107 (Last accessed 15 April 2016).
- Serrano, R. (1999) Four lectures on the nucleolus and the kernel delivered at the Hebrew University of Jerusalem 10th summer school in economic theory. Department of Economics, Brown University, pp. 1–2. Available at: http://www.econ.brown.edu/faculty/Serrano/pdfs/nuclkern.pdf (Last accessed 15 April 2016).
- Shapley, L.S. (1953) A value for n-person games. In Classics in Game Theory. Princeton: Princeton University Press.
- Shapley, L.S. and Shubik, M. (1966) Quasi-cores in a monetary economy with nonconvex preferences. Econometrica: Journal of the Econometric Society 34(4): 805–827.
- Shukla, P.R. (1999) Justice, equity and efficiency in climate change: a developing country perspective. Fairness Concerns in Climate Change,Chapter 9, London: Earthscan Publications. Available at: http://www.decisioncraft.com/energy/papers/ecc/sidc/Equity/ecc.pdf (Last accessed 15 April 2016).
- Tulkens, H. (1997) Cooperation vs. free riding in international environmental affairs: two approaches. CORE Discussion Papers 1997052, Université catholique de Louvain, Center for Operations Research and Econometrics (CORE). Available at: http://ideas.repec.org/p/cor/louvco/1997052.html (Last accessed 15 April 2016).
- Tulkens, H. and Chander, P. (1997) The core of an economy with multilateral environmental externalities. International Journal of Game Theory 26(3): 379–401.
- Von Neuman, J. and Morgenstern, O. (1947) Theory of Games and Economic Behavior. Princeton: Princeton University Press.
- Young, H.P. (1994) Cost allocation. In Handbook of Game Theory with Economic Applications (chapter 2, pp. 1193–1235). Amsterdam: Elsevier. Available at: http://www.sciencedirect.com/science/article/pii/S15740-00505800669 (Last accessed 15 April 2016).
- Zara, S., Dinar, A. and Patrone, F. (2006a) Cooperative game theory and its application to natural, environmental, and water resource issues: 1. Basic theory. World Bank Policy Research Working Paper (November 2006), (4072). Available at: https://openknowledge.worldbank.org/handle/10986/8852 (Last accessed 15 April 2016).
- Zara, S., Dinar, A. and Patrone, F. (2006b) Cooperative game theory and its application to natural, environmental, and water resource issues: 2. Application to natural and environmental resources. World Bank Policy Research Working Paper (November 2006) (4073). Available at: http://dx.doi.org/10.1596/1813-9450-4073 (Last accessed 15 April 2016).
Appendix
A.1 Examples of convex and non-convex games
As a starting point, recall the supermodularity condition, remembering that proving supermodularity is equivalent to proof convexity:
Consider a standard environmental game where counties' pay-offs have the same form as described in Section 5. For simplicity, consider identical countries with the following parameters' values: a = 15, b = 0.02 and d = 0.00225. Since countries are identical, it is irrelevant which player i is chosen to check the supermodularity condition. Furthermore, the only relevant difference between coalitions is their cardinality. Therefore, by considering the cardinality of a coalition as a variable, named s, and considering the function f(s) = v(s) − v(s − 1), supermodularity, in this case, requires that ![]() . In Tables A.1 and A.2, it is possible to observe the normalized results of the mentioned environmental game for, respectively, 6 and 12 players (the parameters are kept constant in both cases). By comparing the last columns of the two tables, it is possible to observe that the 6 players game is actually convex since the values of the column monotonically increase in the number of coalition members (first column), whereas the game with 12 players is not. In fact, the last column of Table A.2 reaches the maximum value for the coalition with cardinality equal to eight.
. In Tables A.1 and A.2, it is possible to observe the normalized results of the mentioned environmental game for, respectively, 6 and 12 players (the parameters are kept constant in both cases). By comparing the last columns of the two tables, it is possible to observe that the 6 players game is actually convex since the values of the column monotonically increase in the number of coalition members (first column), whereas the game with 12 players is not. In fact, the last column of Table A.2 reaches the maximum value for the coalition with cardinality equal to eight.
Table A.1. Game with Six Players.
| Cardinality of S | Πi(S) | v(S) = |S|Πi(S) | v(S) − v(S − 1) |
| 1 | 0.0 | 0.0 | 0.0 |
| 2 | 9.5 | 19.1 | 19.1 |
| 3 | 29.9 | 89.6 | 70.6 |
| 4 | 56.0 | 223.8 | 134.2 |
| 5 | 83.2 | 416.0 | 192.2 |
| 6 | 108.4 | 650.2 | 234.2 |
Table A.2. Game with 12 Players.
| Cardinality of S | Πi(S) | v(S) = |S|Πi(S) | v(S) − v(S − 1) |
| 1 | 0.0 | 0.0 | 0.0 |
| 2 | 5.9 | 11.9 | 11.9 |
| 3 | 21.8 | 65.5 | 53.6 |
| 4 | 43.3 | 173.0 | 107.6 |
| 5 | 66.2 | 331.2 | 158.2 |
| 6 | 87.8 | 527.1 | 195.9 |
| 7 | 106.4 | 745.1 | 218.0 |
| 8 | 121.4 | 971.2 | 226.2 |
| 9 | 132.8 | 1195.1 | 223.9 |
| 10 | 140.9 | 1409.2 | 214.1 |
| 11 | 146.2 | 1607.7 | 198.5 |
| 12 | 148.7 | 1784.7 | 177.1 |
Table B.1. Characteristic Values for All Coalitions.
| Coalition | Coalition | ||||
| Characteristic Value | Members | Characteristic Value | Members | ||
| v({HWLD}) | 310.65 | 1 | v(16) | 724.61 | 1,2,3 |
| v({HWHD}) | 292.26 | 2 | v(17) | 479.93 | 1,3,4 |
| v({MWMD}) | 100.56 | 3 | v(18) | 416.26 | 1,4,5 |
| v({LWLD}) | 54.91 | 4 | v(19) | 462.86 | 2,3,4 |
| v({LWHD}) | 37.14 | 5 | v(20) | 399.00 | 2,4,5 |
| v(6) | 607.12 | 1,2 | v(21) | 202.67 | 3,4,5 |
| v(7) | 414.14 | 1,3 | v(22) | 661.11 | 1,2,5 |
| v(8) | 367.78 | 1,4 | v(23) | 464.37 | 1,3,5 |
| v(9) | 350.76 | 1,5 | v(24) | 675.94 | 1,2,4 |
| v(10) | 396.14 | 2,3 | v(25) | 447.37 | 2,3,5 |
| v(11) | 349.66 | 2,4 | v(26) | 807.00 | 1,2,3,4 |
| v(12) | 332.64 | 2,5 | v(27) | 794.27 | 1,2,3,5 |
| v(13) | 156.97 | 3,4 | v(28) | 743.10 | 1,2,4,5 |
| v(14) | 139.56 | 3,5 | v(29) | 542.03 | 1,3,4,5 |
| v(15) | 93.42 | 4,5 | v(30) | 526.37 | 2,3,4,5 |
| Grand Coalition v(N) | 890.40 | ||||
Table B.2. Pay-offs under Free Riding.
| Free Rider | Shapley Value | ||||
| HWLD | 356.28 | 300.38 | 105.73 | 59.29 | 41.24 |
| HWHD | 320.59 | 336.72 | 106.39 | 59.88 | 41.95 |
| MWMD | 326.02 | 307.60 | 130.54 | 65.31 | 48.47 |
| LWLD | 327.49 | 309.37 | 114.16 | 79.81 | 50.24 |
| LWHD | 327.68 | 309.60 | 114.37 | 66.97 | 63.28 |
| Chander and Tulkens solution | |||||
| HWLD | 352.57 | 299.02 | 106.89 | 60.54 | 43.89 |
| HWHD | 316.88 | 335.37 | 107.56 | 61.14 | 44.60 |
| MWMD | 322.31 | 306.24 | 131.70 | 66.56 | 51.12 |
| LWLD | 323.78 | 308.01 | 115.32 | 81.06 | 52.89 |
| LWHD | 323.97 | 308.24 | 115.54 | 68.23 | 65.93 |
| Least Core | |||||
| HWLD | 354.38 | 297.39 | 106.55 | 62.35 | 42.26 |
| HWHD | 318.68 | 333.73 | 107.22 | 62.94 | 42.97 |
| MWMD | 324.11 | 304.61 | 131.36 | 68.37 | 49.49 |
| LWLD | 325.58 | 306.38 | 114.98 | 82.87 | 51.25 |
| LWHD | 325.77 | 306.61 | 115.19 | 70.03 | 64.30 |
| Nucleolus | |||||
| HWLD | 354.35 | 297.37 | 106.63 | 62.33 | 42.24 |
| HWHD | 318.66 | 333.72 | 107.30 | 62.92 | 42.95 |
| MWMD | 324.09 | 304.60 | 131.44 | 68.35 | 49.47 |
| LWLD | 325.56 | 306.36 | 115.06 | 82.85 | 51.23 |
| LWHD | 325.75 | 306.59 | 115.27 | 70.01 | 64.28 |
| Rawlsian Nucleolus | |||||
| HWLD | 351.57 | 297.31 | 97.10 | 59.62 | 57.31 |
| HWHD | 315.87 | 333.66 | 97.77 | 60.21 | 58.02 |
| MWMD | 321.30 | 304.54 | 121.91 | 65.64 | 64.54 |
| LWLD | 322.77 | 306.31 | 105.53 | 80.14 | 66.31 |
| LWHD | 322.96 | 306.53 | 105.75 | 67.31 | 79.35 |
| ‘R’ Nash Barg. | |||||
| HWLD | 359.01 | 304.76 | 104.54 | 54.83 | 39.79 |
| HWHD | 323.32 | 341.10 | 105.21 | 55.42 | 40.50 |
| MWMD | 328.74 | 311.98 | 129.35 | 60.85 | 47.01 |
| LWLD | 330.22 | 313.75 | 112.97 | 75.35 | 48.78 |
| LWHD | 330.41 | 313.97 | 113.19 | 62.51 | 61.82 |
| HWLD | HWHD | MWMD | LWLD | LWHD | |
Note: Numbers in bold individuate the values relative to the player that is free riding.
Table B.3. Index Coefficients of Free Riding Incentives and Risk.
| Free Rider | Shapley Value | ||||
| HWLD | 0.07464 | −0.04407 | −0.10937 | −0.16293 | −0.25137 |
| HWHD | −0.03303 | 0.07160 | −0.10376 | −0.15458 | −0.23849 |
| MWMD | −0.01665 | −0.02108 | 0.09961 | −0.07794 | −0.12025 |
| LWLD | −0.01221 | −0.01546 | −0.03836 | 0.12676 | −0.08817 |
| LWHD | −0.01164 | −0.01474 | −0.03657 | −0.05448 | 0.14862 |
| Chander and Tulkens solution | |||||
| HWLD | 0.0755 | −0.0443 | −0.1083 | −0.1601 | −0.2398 |
| HWHD | −0.0334 | 0.0719 | −0.1028 | −0.1519 | −0.2275 |
| MWMD | −0.0168 | −0.0212 | 0.0986 | −0.0766 | −0.1147 |
| LWLD | −0.0123 | −0.0155 | −0.0380 | 0.1246 | −0.0841 |
| LWHD | −0.0118 | −0.0148 | −0.0362 | −0.0535 | 0.1418 |
| Least Core | |||||
| HWLD | 0.07507 | −0.04450 | −0.10862 | −0.15620 | −0.24681 |
| HWHD | −0.03322 | 0.07228 | −0.10305 | −0.14819 | −0.23416 |
| MWMD | −0.01675 | −0.02129 | 0.09893 | −0.07472 | −0.11807 |
| LWLD | −0.01228 | −0.01561 | −0.03810 | 0.12152 | −0.08657 |
| LWHD | −0.01171 | −0.01488 | −0.03632 | −0.05222 | 0.14592 |
| Nucleolus | |||||
| HWLD | 0.0751 | −0.0445 | −0.1085 | −0.1562 | −0.2469 |
| HWHD | −0.0332 | 0.0723 | −0.1030 | −0.1482 | −0.2342 |
| MWMD | −0.0167 | −0.0213 | 0.0989 | −0.0747 | −0.1181 |
| LWLD | −0.0123 | −0.0156 | −0.0381 | 0.1216 | −0.0866 |
| LWHD | −0.0117 | −0.0149 | −0.0363 | −0.0522 | 0.1460 |
| Rawlsian Nucleolus | |||||
| HWLD | 0.0757 | −0.0445 | −0.1179 | −0.1622 | −0.1946 |
| HWHD | −0.0335 | 0.0723 | −0.1119 | −0.1539 | −0.1846 |
| MWMD | −0.0169 | −0.0213 | 0.1074 | −0.0776 | −0.0931 |
| LWLD | −0.0124 | −0.0156 | −0.0414 | 0.1262 | −0.0683 |
| LWHD | −0.0118 | −0.0149 | −0.0394 | −0.0542 | 0.1151 |
| ‘R’ Nash Barg. | |||||
| HWLD | 0.0740 | −0.0435 | −0.1105 | −0.1739 | −0.2582 |
| HWHD | −0.0328 | 0.0706 | −0.1048 | −0.1650 | −0.2450 |
| MWMD | −0.0165 | −0.0208 | 0.1006 | −0.0832 | −0.1235 |
| LWLD | −0.0121 | −0.0152 | −0.0387 | 0.1353 | −0.0906 |
| LWHD | −0.0115 | −0.0145 | −0.0369 | −0.0581 | 0.1527 |
| HWLD | HWHD | MWMD | LWLD | LWHD | |
Note: Numbers in bold individuate the values relative to the player that is free riding.
A.2 Proof of superadditivity
Consider a standard environmental game as described in Section 5, having n players. For convenience, consider again the case where countries are identical. With ![]() identify the optimal level of emissions of player i at the disagreement point. Clearly, when forming a coalition, players cannot improve their utility by increasing the amount of emissions compared to the current one. This stems from the fact that, by maximizing the coalition utility, each member of the same coalition must take into consideration the damage caused to the others. For each player i ∈ S, therefore, optimal emissions shift from
identify the optimal level of emissions of player i at the disagreement point. Clearly, when forming a coalition, players cannot improve their utility by increasing the amount of emissions compared to the current one. This stems from the fact that, by maximizing the coalition utility, each member of the same coalition must take into consideration the damage caused to the others. For each player i ∈ S, therefore, optimal emissions shift from ![]() in the disagreement point to e*i(S): Bi′ = ∑i ∈ SD′i, when coalition S is formed. However, since being part of a coalition translates into maximizing the joint utility of its members, given by the sum of their private utilities, and given the fact that the level of emissions is a free variable, simply bounded to be non-negative, nothing prevent coalition members to adopt the same amount of emissions they had when they were not coalesced. Therefore, it cannot be that
in the disagreement point to e*i(S): Bi′ = ∑i ∈ SD′i, when coalition S is formed. However, since being part of a coalition translates into maximizing the joint utility of its members, given by the sum of their private utilities, and given the fact that the level of emissions is a free variable, simply bounded to be non-negative, nothing prevent coalition members to adopt the same amount of emissions they had when they were not coalesced. Therefore, it cannot be that ![]() , otherwise players in S would choose the level of emission
, otherwise players in S would choose the level of emission ![]() . This assures that v(S) is at least equal to the sum of v({i}) of all the members of S. By analogy, it is possible to extend the same reasoning to any union of disjoint coalitions. This proofs that v(S + T) ⩾ v(S) + v(T) ∀S∩T = Ø.
. This assures that v(S) is at least equal to the sum of v({i}) of all the members of S. By analogy, it is possible to extend the same reasoning to any union of disjoint coalitions. This proofs that v(S + T) ⩾ v(S) + v(T) ∀S∩T = Ø.
A.3 Proof of non-negativity of players pay-offs for all coalition sizes when all pay-offs in the disagreement point are positive
In Section 5 can be found the optimal level of emissions expressed in analytic form for the disagreement point, the PANE case and the grand coalition. By plugging in these expressions into the pay-off functions of a country, it is possible to derive the conditions assuring its non-negativity. By substituting, for ease of notation, the lower case s to |S| and n to |N| and starting with the disagreement point, we have
Solving it and eliminating the denominator (necessarily positive), it is possible to find that
For the grand coalition, instead, we have

This is always true for positive values of parameters since:
Finally, in the PANE case, for i ∈ S, we have

Simplifying and deleting the denominator leads to
By separating the components of the expression, it is possible to see that d2(n + s2 − s)2 − d2n2s2 > 0 since ![]() being s < n. It then remains
being s < n. It then remains
that is true for ![]() . Let us consider the case when S includes all players but one: s = n − 1. We then have
. Let us consider the case when S includes all players but one: s = n − 1. We then have
that is always true for positive a, b and d and n ⩾ 2. For s = 2, instead, we have
Compared to the parameters' restriction necessary to assure non-negativity in the disagreement point (![]() ), this is clearly milder and it actually becomes milder by increasing the size of S. For s = 3, for example:
), this is clearly milder and it actually becomes milder by increasing the size of S. For s = 3, for example: ![]() . This proofs that, for avoiding negative pay-offs, it is just necessary to settle appropriate parameters' values for the disagreement case.
. This proofs that, for avoiding negative pay-offs, it is just necessary to settle appropriate parameters' values for the disagreement case.
