2
Dilute Solution Properties of Emerging Hydrocolloids
Ali R. Yousefi1 and Seyed M.A. Razavi2
1 Department of Chemical Engineering, Faculty of Engineering, University of Bonab, Bonab, Iran
2 Food Hydrocolloids Research Center, Department of Food Science and Technology, Ferdowsi University of Mashhad, POBox: 91775-1163, Mashhad, Iran
2.1 Introduction
Hydrocolloids are broadly applied in food systems to enhance their quality by influencing their physical and organoleptic properties as thickening and gelling agents, stabilizers, and texture modifiers [1–4]. Nowadays, the demand for hydrocolloids from plants (e.g., plant cell walls, tree exudates, seeds, and seaweeds) is greater than those from animals (hyaluronan, chitin, gelatin, and chondroitin sulfate) because of greater benefits and a more consumer‐friendly image [5,6]. In addition to the common and commercial hydrocolloids like xanthan, locust bean, guar, dextran, pectin, and so on, nowadays novel hydrocolloids have been introduced by researchers which represent specific characteristics to some extent. Here, some of these novel hydrocolloids are briefly introduced. Hsian‐tsao (Mesona procumbens Hemsl) leaf gum is found to be used as a thickener, and it is reported that it strongly interacts with starch to form thermos‐reversible resilient gels [7]. Lepidium sativum seed gum (cress seed gum) is an annual herb from the Cruciferae family growing in Middle East countries, Europe, and the United States. This hydrocolloid is a culinary herb and has some health‐promoting properties [8]. Cress seeds have been used in traditional medicine for a long time to treat asthma, hypertension, hepatotoxicity, hyperglycemia, enuresis, and fractures [9]. Lallemantia royleana (Benth. in Walla.) is a folk medicinal plant of the Labiatae family, which grows naturally in Asia, Europe, and the Middle East, especially in various regions of Iran. Its common Persian name is Balangu–Shirazi [10]. The mucilage extracted from its seeds shows promise as an emerging source of hydrocolloids, according to recent reports [11]. Alyssum homolocarpum (Qodume Shirazi) is a member of the Cruciferae family, which has many traditional applications. Qodume is native to some Middle East countries including Egypt, Iraq, Iran, and Pakistan. Its mucilage has pharmaceutical applications and has recently been examined as a novel source of hydrocolloids [12]. Wild sage (Salvia macrosiphon) is one Iran's endemic plants, and its seed mucilage is a promising alternative to some commercial gums [13]. Basil (Ocimum basilicum) seed gum is one of the emerging hydrocolloids; it can be isolated from the seeds of basil herb and has recently found many applications in food formulations as a stabilizer, emulsifier, thickener, and gelling agent [14]. Canary seed is an annual cereal crop produced primarily in Canada and Argentina. The distinctive composition and characteristics of this crop make it a favorable cereal for food and industrial applications. Two varieties of hairless canary seed, including CDC Maria and C05041, are currently registered in Canada and have received GRAS (Generally Recognized as Safe) status [15].
Hydrocolloids indicate various functional attributes in the food system because of the physicochemical mechanism underlying their behaviors in a solution, such as their interactions with solvent molecules. Solubility, viscosity enhancing, binding, and many other characteristics are the most important thermodynamically controlled macromolecule–solvent interactions. As an easy and interesting approach, viscosity measurements in dilute domains can be applied to characterize the behavior of hydrocolloids [16]. In a dilute solution, the macromolecular chains are at an adequate distance from each other, and there are no notable interactions between the chains. Therefore, each isolated molecule occupies a discrete hydrodynamic volume within the solution and contributes individually to the bulk properties of the system almost independently [11]. An intense increase in viscosity upon dissolution in an aqueous solution is one of the hallmarks of macromolecules. When a few hydrocolloids dissolve in an aqueous solution, the intrinsic viscosity parameter can be extensively used for their analysis or characterization [17,18]. The limiting viscosity number or intrinsic viscosity is a molecular parameter that indicates the hydrodynamic volume occupied by a unit mass of macromolecule and depends primarily on the molecular size, conformation, and molecular weight as well as solvent quality [19,20]. On the basis of this definition, the intrinsic viscosity concept gives deep insight into the principal molecular attributes of macromolecules in solution. For a particular hydrocolloid, the attractive and repulsive interactions between chain segments can be altered by changing the solvent quality and/or electrostatic repulsion between chains elements via commonly used additives. Basically, it can be expected that any change in the intrinsic viscosity can cause changes in the molecular hydrodynamic volume, conformation, and macromolecular associations [11,13,20]. On the other hand, the mentioned interaction between solvent and cosolutes can reveal the functional properties of hydrocolloids.
The objective of this chapter is to address the dilute solution properties of the aforementioned emerging hydrocolloids and to investigate their structural and morphological characteristics to shed some light on their behavior in the concentrate and gel regimes.
2.2 Partial Specific Volume
The study of the partial specific volume (![]() ) of polymers in dilute solution has recently attracted some attention as a means of gaining information about polymer–solvent interactions. The partial specific volume can be determined with a pycnometer or densimeter. This parameter is very much affected by the molecular weight and concentration of the polymer as well as by the quality of the solvent. This value can be measured by calculating the slope of the (ρ‐ρ
0) versus concentration plot, where ρ and ρ
0 are the densities of the solution and solvent, respectively. Amini and Razavi [11] calculated the
) of polymers in dilute solution has recently attracted some attention as a means of gaining information about polymer–solvent interactions. The partial specific volume can be determined with a pycnometer or densimeter. This parameter is very much affected by the molecular weight and concentration of the polymer as well as by the quality of the solvent. This value can be measured by calculating the slope of the (ρ‐ρ
0) versus concentration plot, where ρ and ρ
0 are the densities of the solution and solvent, respectively. Amini and Razavi [11] calculated the ![]() value of Balangu seed gum solution in deionized water from density measurements at 20 °C (Table 2.1). This parameter was obtained to be 0.61 ml g−1, which was similar to the value of 0.63 ml g−1 reported for high‐methoxyl pectin [25] and Konjac mannan [27], and the value of 0.61 ml g−1 reported for guar and locust bean gums [17], but higher than the value reported for hyaluronan (0.56 ml g−1) by Cowman and Matsuoka [26]. Yousefi et al. [13] found a value of 0.48 ml g−1 for sage seed gum, which was similar to the value obtained for carboxymethyl chitins [22], but lower than for citrus pectin (0.57 ml g−1) [24], xanthan (0.60 ml g−1) [28], and Balangu seed gum (0.61 ml g−1) [11].
value of Balangu seed gum solution in deionized water from density measurements at 20 °C (Table 2.1). This parameter was obtained to be 0.61 ml g−1, which was similar to the value of 0.63 ml g−1 reported for high‐methoxyl pectin [25] and Konjac mannan [27], and the value of 0.61 ml g−1 reported for guar and locust bean gums [17], but higher than the value reported for hyaluronan (0.56 ml g−1) by Cowman and Matsuoka [26]. Yousefi et al. [13] found a value of 0.48 ml g−1 for sage seed gum, which was similar to the value obtained for carboxymethyl chitins [22], but lower than for citrus pectin (0.57 ml g−1) [24], xanthan (0.60 ml g−1) [28], and Balangu seed gum (0.61 ml g−1) [11].
Table 2.1 Partial specific volume (![]() ) for some hydrocolloids.
) for some hydrocolloids.
| Hydrocolloid | Partial specific volume (ml g−1) | Reference |
| Alyssum homolocarpum | 0.44 | [21] |
| Balangu seed gum | 0.61 | [11] |
| Basil seed gum | 0.62 | [14] |
| Carboxymethyl chitins | 0.47 | [22] |
| Chitosan | 0.58 | [23] |
| Citrus pectin | 0.57 | [24] |
| High‐methoxyl pectin | 0.63 | [25] |
| Hyaluronan | 0.56 | [26] |
| Guar and Locust bean gums | 0.61 | [17] |
| Konjac glucomannan | 0.63 | [27] |
| Sage seed gum | 0.48 | [13] |
| Xanthan | 0.60 | [28] |
The buoyancy of particles in food systems is an important factor that affects the sedimentation phenomenon. The buoyancy of a specific particle increases with the increase in the partial specific volume, and therefore the higher the partial specific volume for a polymer, the less the sedimentation [28]. Durchschlag [29] reported that the partial specific volume of native conjugated proteins in aqueous solution varies in the range 0.59–1.05 ml g−1. For another novel hydrocolloid, A. homolocarpum seed gum, a value of 0.44 ml g−1 was reported by Hesarinejad et al. [21], which is comparable to those determined for the sage seed gum (0.48 ml g−1) and carboxymethyl chitins (0.47 ml g−1). In another study, the partial specific volume of basil seed gum found to be about 0.62 ml g−1, which decreased upon increasing the temperature [14]. This value is very close to those reported for some hydrocolloids such as Balangu seed gum, high‐methoxyl pectin, guar, and locust bean gums, but higher than that for hyaluronan and chitosan.
2.3 Hydrogel Content
So far, researchers have defined hydrogels in many different ways. The most common of these is that a hydrogel is a water‐swollen, cross‐linked polymeric network produced by the simple reaction of one or more monomers. It is also defined as a polymeric material that represents the ability to swell and retain a significant fraction of water within its structure without dissolving in water [30]. Hydrogels have received much attention in the past 50 years, due to their exceptional promise in a wide range of applications [31,32]. The water absorption ability of hydrogels is associated with hydrophilic functional groups attached to the polymeric backbone, whereas their resistance to dissolution is due to cross‐links between network chains. Hydrogels can be designed with controllable responses such as shrinkage or expansion with changes in external environmental conditions [30]. They may exhibit dramatic volume transitions in response to a variety of physical and chemical stimuli, where the physical stimuli include temperature, electric or magnetic field, light, pressure, and sound, while the chemical stimuli include pH, solvent composition, ionic strength, and molecular species (Figure 2.1).

Figure 2.1 Stimuli response of swelling hydrogel.
Source: Adapted from Ahmed [30] with permission from Elsevier.
Biopolymer‐based hydrogels have attracted increasing attention due to their biocompatibility, biodegradability, and tissue‐mimicking consistency. It has been demonstrated that biopolymer‐based hydrogels are used in varied fields such as agriculture [33,34], hygiene, biomedical materials [35,36], pollutant adsorbents [37–39], biosensors [40], and so on. So far, various natural polymers or their salts such as sodium alginate, starch, protein, gelatin, hyaluronate, hemicelluloses, lignin, cellulose, chitin, and their derivatives have been applied to fabricate biopolymer‐based hydrogels [41]. The hydrogel content of hydrocolloids can be determined using the filtration method defined by Karazhiyan et al. [18]. In this method, a prepared solution of hydrocolloid is filtered under vacuum via filter paper. The filtrate is discarded, and the insoluble hydrogel is dried in an oven at 105 °C until a constant weight achieved. Then, the hydrogel content of hydrocolloid can be estimated as follows:
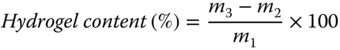
where m1, m2, and m3 are the sample weight, initial weight, and final weight of the filter paper, respectively. The hydrogel content of several novel hydrocolloids has been reported. The hydrogel content of Balangu seed gum solution in deionized water was determined to be about 46% insoluble [11]. The hydrogel content of basil seed gum was obtained as 73.6% [14], which is near to that of L. sativum seed gum (76%) [18]. It is found that the type and concentration of the cosolute can significantly affect the amount of hydrogel content. Accordingly, an increase in ionic strength or concentration of ions drastically increases the hydrogel content. In this case, divalent cations like Ca2+ are more effective in increasing the hydrogel content rather than monovalent cations like Na+. In this case, Amini and Razavi [11] stated that as a result of the increase in ionic strength, the hydrogel content of Balangu seed gum solutions at all levels of ion concentrations was observed to increase drastically, and the Ca2+ was more effective than the Na+. On the other hand, the hydrogel content of Balangu seed gum is enhanced by the presence of sugars (sucrose and lactose) compared to sugar‐free solution, and in most cases, it is not influenced significantly by increasing the sugar concentration.
The hydrogel, from the physical standpoint, probably indicates that the molecular species form large aggregates in solution so that their overall sizes exceed the pore size of the filter paper, making it impossible for them to pass through it. If this assumption is valid, it could be inferred that the hydrogel content would be used as a sensitive measure of molecular species aggregation in response to any alteration in solvent conditions [11].
2.4 Molecular Weight
The same polymer from different sources may have different molecular weights. All common synthetic polymers and most natural polymers (except proteins) have a distribution in molecular weights. Therefore, some molecules in a given sample of a hydrocolloid are larger than others. The two most important molecular weight averages are the number‐average molecular weight, M n , and the weight‐average molecular weight, M w , as follows:


where N i is the number of molecules of molecular weight M i .
For single‐peaked distributions, M
n
is usually near the peak. The weight‐average molecular weight is always larger than number‐average molecular weight. For simple distributions, M
w
may be 1.5–2.0 times M
n
. The ratio M
n
/M
w
, sometimes called the polydispersity index, provides a simple definition of the molecular weight distribution [42]. There are different methods for molecular weight determination of polymers. These techniques can be classified as osmometry techniques (membrane and vapor pressure osmometry) [43], end group analysis (this method is important particularly in the determination of the average molecular weight of step‐growth polymers) [44], light‐scattering technique (this technique thus relies on the measurement of light scattered at an angle to the incident ray as it passes through the target), sedimentation technique (this instrument measures sample concentration with respect to position from the center of a rapidly rotating cell) [43], and gel permeation chromatography (GPC) (the separation mechanism is essentially based on the differences in the size of polymer materials) [45]. The light scattering and GPC techniques are rather more common than the others as reported in the literature. In the case of the light‐scattering technique, the weight‐average molecular weight (M
w
) and the second virial coefficient (A
2) can be obtained from the scattered light intensities using a plot of the excess Rayleigh factor (![]() ) at the scattering angle (θ = 90°) as a function of polymer concentration (c) which is in the dilute solution regime. The following equations allow determination of M
w
[11]:
) at the scattering angle (θ = 90°) as a function of polymer concentration (c) which is in the dilute solution regime. The following equations allow determination of M
w
[11]:
where λ0 is the beam wavelength, K is a calibration constant, N
A
is Avogadro's number, n is the refractive index of solvent, and dn/dc is the specific refractive index increment. After constructing the Kc/
![]() versus curve at different concentrations, A
2 and M
w
can be calculated from the slope and the reciprocal of the intercept, respectively.
versus curve at different concentrations, A
2 and M
w
can be calculated from the slope and the reciprocal of the intercept, respectively.
According to the Zimm plot, the key equations at the limit of the zero angle (Eq. (2.4)) and zero concentration (Eq. (2.7)), respectively, relating the light‐scattering intensity to the weight‐average molecular weight M w and the z‐average radius of gyration R g are
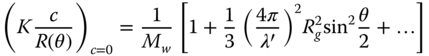
where λ′ is the wavelength of the light in solution (λ0/n 0), and R g is the gyration radius. To construct a Zimm plot, Eqs. (2.4) and (2.7) are added. To make a more aesthetic plot, the concentration term is usually multiplied by an arbitrary factor. Thus three quantities of interest can be determined from the same experiment: the weight‐average molecular weight, the z‐average radius of gyration, and the second virial coefficient. A useful practical equation for the determination of R g from a plot of K[c/R(θ)] versus sin2 θ/2 is [42]
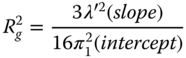
Equations (2.4) and (2.7) show the function K[c/R(θ)] in the limit of θ = 0 and c = 0, respectively. Three important pieces of information can be extracted from this experiment: the weight‐average molecular weight, z‐average radius of gyration, and the second virial coefficient [42]. A most powerful advance was the introduction of the Zimm plot, which enabled the radius of gyration, the molecular weight, and the second virial coefficient to be calculated from a single master figure, by plotting K[c/R(θ)] versus a function of both the angle and concentration (Figure 2.2).
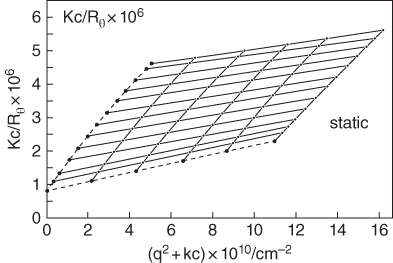
Figure 2.2 Zimm plots of a bacterial polysaccharide in 0.1 M NaCl obtained with the static light scattering technique. λ0 = 488 nm, dn/dc = 0.145 cm3 g−m. Static light‐scattering yields R g , M w , and A 2.
Source: Adapted from Dentini et al. [46] with permission from American Chemical Society.
Amini and Razavi [11] used the Debye plot (it is not capable of determining the z‐averaged square of the radius of gyration) rather than the Zimm plot one to determine the M w and A 2 of Balangu seed gum. Their results in the temperature range 30–50 °C showed that M w decreased significantly with increasing temperature from 30 to 50 °C. This reduction in M w may probably indicate that Balangu seed gum has been degraded or debranched at temperatures higher than 30 °C. Also, A 2 decreased with an increase in temperature, demonstrating that the solvent quality has been decreased. In contrast, for some hydrocolloids like pullulan, it is observed that A 2 does not change with temperature [47]. Similar results were found in the case of the influence of temperature on M w by Axelos and Branger [48] for pectin and by Morris et al. [25] for high‐methoxyl pectin. According to the literature, it has been reported that the M w of pullulan is not affected by temperature, and it seems that only the temperatures above 35 °C decrease this value slightly [47]. Mirabolhassani et al. [14] applied a dilute solution of basil seed gum with a concentration in the range of 0.0025–0.01 g ml−1 to measure M w using the light‐scattering method. Their results indicate that basil seed gum has a small molecular weight (3.66 × 105 Da). Using the same method, Razavi et al. [49] reported that the M w of sage seed gum was found to be 4.33 × 105 Da. These values of M w for the novel hydrocolloids were lower than those reported for guar gum (1.3 × 106 Da), locust bean gum (1.2 × 106 Da), mesquite gum (1.2 × 106 Da), and fenugreek gum (1.4 × 106 Da) [50–52].
2.5 Intrinsic Viscosity
The hydrodynamic volume occupied by a given polymer mass is defined as its intrinsic viscosity, [η], which is a parameter that can be measured by dilute solution viscosity measurement [53]. This parameter is a measure of the interaction of the molecular structure with the solution. In dilute solution domains, due to the separation of polymer chains, this parameter is closely in associated with the size and conformation of macromolecular chains in a specific solvent [19]. According to the literature, several theories in polymer physics relate the intrinsic viscosity to the molecular properties of polymers such as the molecular weight, overlap concentration, radius of gyration, and pore size of the concentrated polymers [54]. The intrinsic viscosity values are also important in determining the solubility parameters of polymers with a linear structure in different solvents [55]. Finally, the intrinsic viscosity values are important for probing the biological macromolecular structure and interaction with a solution [56]. The intrinsic viscosity is evaluated by measuring the viscosity of the polymer solution over a range of concentrations. The intrinsic viscosity is determined by either timing the flow of the solution through a capillary tube or by measuring the force required to rotate two concentric surfaces separated by the solution. The intrinsic viscosity [η] can be determined by measuring the viscosity of very‐low‐concentration solutions by calculating the following viscosities:
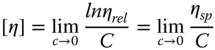
where η is the solution viscosity, η s is the solvent viscosity, η rel is the relative viscosity, and η sp is the specific viscosity.
Several equations have been developed to determine the intrinsic viscosity. According to the Huggins model [57] (Eq. (2.12)), the intrinsic viscosity [η] is obtained simply by extrapolating η sp /C data to zero concentration through a linear regression:
where k′ is the Huggins constant. Kraemer [58] reported that the intrinsic viscosity [η] could be obtained by extrapolating lnη rel /C values to zero concentration (Eq. (2.13)):

where k″ is the Kraemer constant. It is demonstrated that the methods that calculate the intrinsic viscosity on the basis of the slopes of plots had higher correlation coefficient and lower standard errors in comparison with methods that employ the intercepts of plots [59,60]. From this finding, the following three equations are shown to determine the intrinsic viscosity of the solutions on the basis of the slope of plots:
Tanglertpaibul and Rao model [61]:
Higiro et al. models [19]:
It should be noted that in addition to the capillary viscometer method, differential viscometer and light scattering and imaging techniques can be used to determine the intrinsic viscosity. The differential viscometer technique is based on a fluid analog of the Wheatstone bridge electrical circuit. The differential pressure across a bridge of four fluid capillaries is measured to evaluate the relative viscosity of the polymer solution [53]. The mean square displacement of the scattering (imaging) particles in the polymer solution is calculated using scattered intensity or particle position data. In the light scattering and imaging technique, the intrinsic viscosity of the solution is then evaluated using the Stokes–Einstein equation, which relates diffusivity and viscosity [62].
Amini and Razavi [11] observed good linear extrapolations for both Huggins and Kraemer plots (R2 > 0.97) in the case of determination of the intrinsic viscosity of Balangu seed gum in different solvent/cosolutes and temperatures. The intrinsic viscosity of Balangu seed gum at 20 °C in deionized water was observed to be 72.36 dl g−1 (Figure 2.3).

Figure 2.3 Typical dual Huggins–Kraemer plot of Balangu seed gum in deionized water at 20 °C.
Source: Adapted from Amini and Razavi [11] with permission from Elsevier.
Mirabolhassani et al. [14] reported that all the aforementioned models (Eqs. (2.12)–(2.16)) can be utilized for estimation of the intrinsic viscosity of basil seed gum at different temperatures and in the presence of different cosolutes; however, among them, the Higiro model showed the best fitting results (R 2 > 0.98). They found that the intrinsic viscosity of basil seed gum solution in deionized water was 11.38 dl g−1 at 25 °C. According to the results reported by Yousefi et al. [13], the obtained intrinsic viscosity from the models in which this value is calculated through the slopes of plots had a higher determination coefficient (R 2) and lower root mean square error ( RMSE ) than the intrinsic viscosity obtained from the intercepts of plots. Similar results were reported by McMillan [60], Razavi et al. [59], and Behrouzian et al. [8]. Their results demonstrate that the Higiro model (Eq. (2.15)) is the most suitable model for determination of the intrinsic viscosity of sage seed gum, which had the highest R 2 in the range 0.993–0.999 and the lowest RMSE in the range 0.002–0.041. Accordingly, the value of the intrinsic viscosity of sage seed gum in deionized water at 25 °C was found to be 10.11 dl g−1. Razavi et al. [59] reported that Tanglertpaibul and Rao's model is the best model to estimate the intrinsic viscosity of sage seed gum at 20, 30, and 40 °C, but in agreement with the results of Yousefi et al. [13], the Higiro model (Eq. (2.15)) is the best model to determine the intrinsic viscosity value of sage seed gum at different concentrations of NaCl and CaCl2 (0.5, 20, 50 mM). The results obtained by Irani et al. [63] showed that the Higiro model is the best among the models (Eqs. (2.12)–(2.16)) for intrinsic viscosity determination of both canary seed starch and wheat starch. They found that the intrinsic viscosity of canary seed varieties (C05041and CDC Maria) and wheat starches were 1.42, 1.46, and 1.70 dl g−1, respectively. Lai and Chiang [7] used the Huggins model to determine the intrinsic viscosity of hsian‐tsao leaf gum. The results obtained by Timilsena et al. [64] revealed that the intrinsic viscosity of chia seed gum can be precisely (R 2 > 0.950) determined by extrapolating the experimental data to infinite dilution using the Huggins and Kraemer models at 20 °C. The estimated intrinsic viscosity of chia seed gum in deionized water was in the range 16.34–16.63 dl g−1. These models (Huggins and Kraemer) also have been reported to be suitable (R 2 > 0.950) for determination of the intrinsic viscosity of A. homolocarpum seed gum [21]. Accordingly, the intrinsic value obtained for A. homolocarpum seed gum solution in deionized water at 25 °C was 18.34 dl g−1. For cress seed gum, it is found that the intrinsic viscosity attained by Tanglertpaibul and Rao model is the most accurate value (R 2 = 0.995) in comparison with the other models. The intrinsic viscosity obtained for this hydrocolloid in deionized water at 25 °C is about 3.92 dl g−1 [8].
Many studies on the dilute solution properties of hydrocolloids have underscored the fact that the intrinsic viscosity is highly sensitive to the solution temperature and concentration of the cosolutes. The intrinsic viscosity decreases with increasing temperature, possibly due to the increased kinetic energy and phase separation [13]. On the basis of thermodynamic principles, low temperatures are suitable for polymer–solvent interactions, which provide a negative enthalpy of polymer–solvent mixing. Phase separation takes place with increasing temperature because of the more unfavorable entropy contribution to the free entropy. Therefore, it can be concluded that the solvent quality decreases as the temperature increases [65]. By increasing the temperature from 25 to 65 °C, the intrinsic viscosity of sage seed gum decreased from 10.11 to 2.96 dl g−1. Similar results have been reported regarding the influence of temperature on the intrinsic viscosity of some conventional hydrocolloids such as pectin [25], dextran [66], L. sativum [18], chitosan [23], pullulan [47], gellan [67], hydroxypropyl cellulose [65], and hyaluronan [68]. In contrast, it has been shown that intrinsic viscosity of mesquite gum is not affected by ionic strength variation from 0.05 to 2.0 M [69]. Moreover, divalent cations (Mg2+ and Ca2+) had a more effective reduction in the intrinsic viscosity value of sage seed gum compared with the monovalent cations (Na+ and K+) when the salt concentration rose to 200 mM [13] (Figure 2.4).
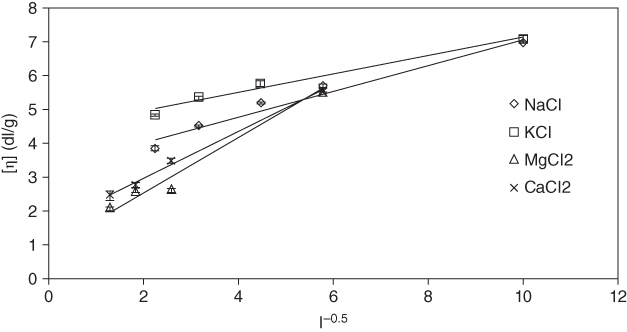
Figure 2.4 Intrinsic viscosity of sage seed gum as a function of the inverse square root of ionic strength at 25 °C.
Source: Adapted from Yousefi, Razavi, and Khodabakhsh Aghdam [13] with permission from Elsevier.
The intrinsic viscosity of Balangu seed gum decreased from 72.36 to 50.04 dl g−1 when the temperature was increased from 20 to 50 °C. In disagreement with these results, Stivala and Bahary [70] found that the intrinsic viscosity of levan was increased as the temperature increased from 25 to 57 °C. Also, it has been reported that temperature has little impact on alginate's intrinsic viscosity [71]. Lai and Chiang [7] found that the intrinsic viscosity of hsian‐tsao leaf gum is not affected by temperature, so that with increasing temperature from 20 to 40 °C, the intrinsic viscosity only decreased from 2.93 to 2.37 dl g−1. The hydrodynamic behavior (intrinsic viscosity) of hsian‐tsao leaf gum was found to be strongly affected by ion types (Na+, K+, Ca2+, and Mg2+) and ionic strength [7]. Although no research has been done on the influence of salts on the intrinsic viscosity of A. homolocarpum seed gum, it is observed that the apparent viscosity of this hydrocolloid decreases with addition of Na+, K+, Ca2+ (<0.01 M), and Mg2+ (<0.039 M), whereas it increases with the addition of Ca2+ and Mg2+ at concentrations higher than 0.01 and 0.039 M, respectively [12]. On the other hand, the intrinsic viscosity of A. homolocarpum seed gum decreases as the temperature increases up to 55 °C, but at 65 °C, the intrinsic viscosity increases, which can be attributed to the increased chain dimension of this polymer [21]. The variations in Balangu seed gum intrinsic viscosity with ionic strength revealed that it has a drastic effect compared to temperature, and the influence of the divalent cation (Ca2+) was more pronounced than that of the monovalent cation (Na+). The results obtained by Behrouzian et al. [8] demonstrated an initial abrupt decrease in the [η] value of cress seed gum as 25 mM NaCl was added, with a slight increase by 50 mM NaCl addition, followed by a very moderate decrease in [η] at higher NaCl concentrations. There was also a continual decrease in the intrinsic viscosity value up to 10 mM CaCl2, followed by almost no change in value with 15 mM CaCl2 addition. In addition to the salt influence, it is reported that the presence of sugars such as glucose, lactose, and sucrose at some concentrations decreases the intrinsic viscosity. This influence is found in some novel hydrocolloids like Balangu seed gum [11], cress seed gum [8], and two hairless canary seed starches (varieties C05041 and CDC Maria) [15]. Similar results have been reported for the influence of sucrose on the intrinsic viscosity of conventional hydrocolloids like guar and locust bean gums [72] and β‐glucan [73]. The reduction in intrinsic viscosity in the presence of sugars may be attributed to a decrease in the size of polymers in the course of a reduction in the level of macromolecules association. On the contrary, Richardson et al. [74] reported that the intrinsic viscosity of guar and locust bean gums increases at sucrose concentrations of 5%, 10%, and 20%, but it diminishes as the concentration of sucrose increases to 40%. This was in agreement with the results reported by Behrouzian et al. [8] for a novel hydrocolloid of cress seed gum, so that sucrose addition up to 10% and 20% w/w to cress seed gum solutions diminished the intrinsic viscosity, and further addition of this sugar up to 40% w/w resulted in intrinsic viscosity increase. This trend was similar to that observed with lactose addition in the range of 5%–15% w/w. Several factors are referred by the authors to explain this enhancement effect upon [η], such as a decrease in the dielectric constant of the solvent, the dehydration of sucrose or lactose, and the aggregation formation of macromolecules when sugar is added to aqueous hydrocolloid solution. It has been found that the intrinsic viscosity of pectin increases in the presence of sucrose [75]. On the other hand, Launay et al. [76] reported that the intrinsic viscosity of guar and locust bean gums is not influenced by increasing the sucrose concentration from 10% to 40%. Table 2.2 shows the intrinsic viscosity values of several hydrocolloids reported in the literature. It can be observed that the intrinsic viscosity of all novel hydrocolloids reported is lower than that of xanthan, but some of them like Balangu seed gum have a greater intrinsic viscosity in comparison with guar, locust bean, pectin, dextran, levan, and other conventional hydrocolloids. Among the novel hydrocolloids reported in this context, the intrinsic viscosity of Balangu seed gum is found to have the greatest value (72.36 dl g−1) followed by A. homolocarpum seed gum (18.34 dl g−1), chia gum (16.63 dl g−1), basil seed gum (11.38 dl g−1), sage seed gum (10.11 dl g−1), cress seed gum (3.92 dl g−1), hsian‐tsao leaf gum (2.93 dl g−1), and canary starches (1.42–1.46 dl g−1) in the temperature range 20–25 °C.
Table 2.2 Intrinsic viscosity of emerging hydrocolloids in comparison with some commercial ones.
| Hydrocolloid | Temperature (°C) | Solvent | [η] (ml g−1) | Reference |
| Xanthan | 25 | Deionized water | 11230 | [77] |
| 25 | Deionized water | 11034 | [78] | |
| 20 | Deionized water | 21421 | [19] | |
| Guar | 25 | Deionized water | 1580 | [52] |
| 25 | Deionized water | 1210 | [17] | |
| 25 | Deionized water | 1270 | [76] | |
| Locust bean | 25 | Deionized water | 1420 | [52] |
| 25 | Deionized water | 1103 | [79] | |
| 20 | Deionized water | 1249 | [19] | |
| Pectin | 25 | 50 mM citrate–phosphate buffer | 313.31 | [80] |
| 20 | 0.1 M phosphate buffer | 406 | [25] | |
| 25 | Deionized water | 2450 | [81] | |
| 35 | Deionized water | 215 and 294 | [82] | |
| Konjac mannan | 20 | 0.1 M phosphate buffer | 565–1300 | [27] |
| κ‐Carrageenan | 25 | Deionized water | 4120 | [83] |
| Dextran | 20 | Deionized water | 49.1 | [66] |
| Levan | 25 | Deionized water | 13.4 | [70] |
| 57 | Deionized water | 18.8 | [70] | |
| Tara gum | 25 | Deionized water | 1455 | [52] |
| Fenugreek | 25 | Deionized water | 1510 | [52] |
| Detarium senegalicum | 25 | Deionized water | 890 | [84] |
| Cassia nodosa | 25 | Deionized water | 1210 | [66] |
| B‐glucan | 20 | 2%–10% maltose | 281–466 | [73] |
| Hydroxypropylmethyl cellulose | 25 | Deionized water | 770 and 1300 | [85] |
2.5.1 Huggins Constant
The Huggins constant, denoted as k H , is a criterion of the polymer–polymer as well as polymer–solvent interaction in the dilute region and depends upon the molecular architecture and extent of coil expansion of the polymer [74,86]. The value of k H is usually in the range 0.3–1, although it is approximately 0.3–0.4 for resilient macromolecules with extended shapes in good solvents [56,87]. However, the values between 0.5 and 0.8 are for theta solvents and values higher than 1.0 indicate poor solvents and macromolecule aggregation.
The Huggins constant for some novel hydrocolloids has been reported in the literature. The value of k H obtained for A. homolocarpum seed gum in deionized water is found to be 0.301, which is close to the value of 0.3 in good solvents. In addition, with increasing temperature from 25–65 °C, k H obviously decreases from 0.30 to 0.13 [21], indicating smaller intermolecular (polymer–polymer) interaction for the heat‐treated A. homolocarpum seed gum or deteriorating solvent quality at elevated temperatures [88]. The value of k H calculated for Balangu seed gum at 20 °C in deionized water was 0.33. This value can also be considered as a value close to 0.3 in good solvents [11]. Moreover, by increasing the temperature, k H clearly decreased from its value in good solvent conditions, showing a worsening of the solvent quality and/or the occurrence of molecular associations at elevated temperatures [88], probably due to a decrease in space prohibition between adjacent molecules through debranching which makes association between them much easier than in their branched state. Irani et al. [63] reported values of k H for canary seed starches (CO5041 and CDC Maria) and wheat starch of 0.88–1.33 and 1.89, respectively, indicating the presence of aggregates in most samples. CDC Maria starch showed higher k H value compared to CO5041 starch, but there were no significant differences between the canary starches. The values of k H for canary starches were found to be lower than that for the wheat starch sample. These values of k H could suggest compact structures for the branched molecules of canary seed starches whose major fraction is amylopectin, and amylopectin would behave in 90% dimethyl sulfoxide (DMSO) solution like an aggregate of the linear polymer [89]. Similar results were reported for waxy maize starch (k H = 0.84–1.23) [90] and waxy corn starch (k H = 1.22) [89] in 90% DMSO solution. Timilsena et al. [64] obtained the k H values for chia seed gum solution with and without acid treatment. Their results indicated that k H was 0.74 for unhydrolyzed chia seed gum, 0.48 after hydrolyzing for 2 h, and 0.37 after hydrolyzing for 8 h. They stated that the lower values of k H in depolymerized chia seed gum implies that the viscosity of the polysaccharide is reduced appreciably due to hydrolysis. The decrease in k H indicates the improved aqueous solubility of depolymerized chia seed gum as compared to that of the unhydrolyzed counterpart. In addition, the higher value of k H for unhydrolyzed chia seed gum is indicative of additional polymer–polymer interactions in the aqueous solution due to the formation of intermolecular hydrogen bonds through the unsubstituted regions of the backbone [91]. It is found that the k H value increases to unity as the ionic strength of hydrocolloid solution increases. It may be concluded that the increase in k H suggests that the aggregation takes place when the ionic strength increases. Amini and Razavi [11] stated that the presence of lactose and sucrose causes k H to deviate from its value corresponding to good solvent conditions (0.3–0.4). This may be attributed to an increase in the interaction between macromolecules, making it less favorable for the macromolecules to interact with solvent.
2.6 Coil Overlap Parameter and Molecular Conformation
It is well known that the conformation of many macromolecules in a solution is the random coil, which is a polymer conformation where the monomer subunits are oriented randomly while still being bonded to adjacent units [92]. In a dilute solution, polymer coils move freely due to the sufficient space available between molecules, which have a trivial impact on each other [7]. During the transition from dilute solution to the semi‐dilute solution regime, an abrupt change takes place in concentration depending on the viscosity of the solution [88]. The corresponding polymer concentration is called the critical or coil overlap concentration (C*). To determine this parameter for hydrocolloids, a “master curve,” which is the plot of the logarithm of η SP versus the logarithm of C[η] is used. Empirically, it has been showed that for a wide range of “random coil” polysaccharides, logη sp varies approximately linearly with logC over the viscosity range 1 < η sp < 10, with a slope of about 1.4 (exponent b in Eq. (2.17)), indicating a dilute solution domain:
At higher values of η sp , however, the concentration dependence remarkably changes to a slope of about 3.3 (semi‐dilute and concentrated domains), because a point is reached where the individual coils start to entangle [13].
According to the results obtained by Yousefi et al. [13], the slope values calculated for sage seed gum at the temperature range 25–65 °C were in the range 1.04–1.13. This range of values indicates that the molecular conformation of sage seed gum is between the random coil and rod‐like [8]. When the Berry number (C[η]) exceeds one, the molecular entanglement begins in the concentrated regime [93]. The Berry number value for sage seed gum was found to be within the range 0.12–0.78 at all selected temperatures (25–65 °C) and salts concentrations (0–100 mM), indicating no coil overlap and that molecular entanglements occurred. The results of Mirabolhassani et al. [14] revealed that the Berry number of basil seed gum was less than one at selected temperatures and cosolute concentrations used, indicating absence of coil overlapping and the occurrence of molecular entanglements under different conditions. Furthermore, the slope of the power‐law model (Eq. (2.17)) or the b values of basil seed gum at temperatures of 35–65 °C ranged from 0.55 to 0.86, demonstrating that the molecular conformation of basil seed gum is probably rod‐like, but at 25 °C, it changed to a random coil conformation. Also, they reported that lactose and sucrose were able to change the basil seed gum conformation, and the booster random coil and the rod‐like structure were formed in the presence of lactose and sucrose, respectively. The Berry number obtained for hsian‐tsao leaf gum ranged from 3.10 to 4.32 and for decolorized hsian‐tsao leaf gum was 13.32–13.41, which showed significant deviation from the general behavior for most of the random coil polysaccharides [7]. Also, the slopes obtained for hsian‐tsao leaf gum ranging from 0.68 to 0.77 and from 1.77 to 1.89 in the dilute and entangled (semi‐diluted) domains, respectively. In the study of Behrouzian et al. [8], the slope value for cress seed gum was 1.08, indicating that the molecular conformation of this hydrocolloid is between random coil and rod‐like. Karazhiyan et al. [94] also suggested that the macromolecular component in L. sativum extract had a semi‐rigid chain conformation with intermediate flexibility between the random coil and rigid rod. The value of the Berry number was changed from 0.30 to 0.97 and from 0.39 to 1.16 when sucrose and lactose were used as cosolutes of cress seed gum. An increase in the concentration of sucrose and lactose was accompanied by an increase in the slope of the power‐law relation (Eq. (2.17)). Similar results were obtained in the presence of salts (NaCl and CaCl2). They concluded that sugars and salts are able to change the cress seed gum conformation, and the amplifier random coil structure is formed in the presence of these cosolutes. In the case of chia seed gum, it was reported that the transition from the dilute to the semi‐dilute regime occurs at a C[η] value close to 0.5, which is well below the critical reduced concentration C[η] = 0.77 predicted for flexible random coil polymers such as dextran and locust bean gum from the Fox–Flory theory [76]. A sharp change in gradient from 1.4 to 3.3 was reported earlier for the dilute and semi‐dilute regimes of disordered linear polysaccharides such as guar gum and carrageenan [88]. Heydari et al. [15] found that sugar has a clearer effect on the master cure slope of canary seed starches (CO5041 and CDC Maria) and wheat starch samples, and the influence of lactose was more remarkable than that of sucrose. They concluded that, due to increase sugar content of starch solutions and the enhancement of temperature, the master curve slope of each starch solution found to be enhanced compared with the value obtained for a sugar‐free solution. This trend proves that increasing the temperature and sugar concentration is bound to cause aggregation because of a decrease in solvent quality and/or decrease in available solvent for dissolving the biopolymer. The obtained b values (from Eq. (2.17)) for canary seed starches and wheat starch samples at temperatures of 25–55 °C and sugar concentrations were calculated as 1.15–1.31 and 1.2–1.32, respectively, confirming that molecular conformation of canary seed starches and wheat starch were the random coils. Higiro et al. [95] reported the b number in the dilute regime at 1.23 and 0.79, while studying locust bean gum and xanthan, respectively. They reported the molecular conformation of locust bean gum as a random coil and that of xanthan as a rod molecule.
2.7 Chain Flexibility Parameter
It is reported that under C* concentration of a polymer solution (Newtonian region), increasing temperature causes a decrease in viscosity which follows Arrhenius law [69]:
where η is the dynamic viscosity (Pa s), A is a constant number, E a is the activation energy of the flow process (kJ kg−1 mol−1), R is the universal gas constant (8.314 kJ kg−1mol−1 K−1), and T is the absolute temperature (K). It is reported that the chain flexibility of a polymer decreases with increasing temperature and can be calculated from the E a value [13]. By substitution of the intrinsic viscosity with the dynamic viscosity, the calculated slope for the natural logarithmic intrinsic viscosity versus the inverse of the absolute temperature (1/T) can be used to calculate the chain flexibility of a polymer due to its relation with E a .
The obtained value for chain flexibility parameter (E a /RT) and activation energy (E a ) of sage seed gum at temperatures of 25–65 °C were 3046.45 and 2.53 × 107 J kg−1 mol−1, respectively. The chain flexibility value of sage seed gum is much higher than those calculated by Chen and Tsaih [96] for chitosan (666–1334), by Milas and Rinaudo [97] for xanthan (900–1100), and by Amini and Razavi [11] for Balangu seed gum (1156.23). The achieved E a for sage seed gum was very close to the value of 2.5 × 107 J kg−1 mol−1 obtained for chitosan with 91% DD, while it is much higher than the value of 1.5 × 107 J kg−1 mol−1 determined for chitosan with 75% DD [98] and for Balangu seed gum (1 × 107 J kg−1 mol−1) [11], indicating that sage seed gum macromolecules entangled easier within themselves, which resulted in the higher E a value.
The chain flexibility parameter and E a values for Balangu seed gum were found to be 1156.53 and 1 × 107 J kg−1 mol−1, respectively [11]. It has been reported that the E a value of hydrocolloids usually ranges from 1 × 107 to 1 × 108 J kg−1 mol−1 [96]. It has been reported that the chain of larger‐molecular‐weight hydrocolloids is more flexible than that of smaller‐molecular‐weight ones [96]. Heydari et al. [15] found that the chain flexibility parameter and E a for CDC Maria canary starch at temperatures of 25–55 °C were estimated to be 190.2 and 4.32 × 106 J kg−1 mol−1, whereas the values of these parameters for C05041 canary starch were 185.81 and 4.22 × 106 J kg−1 mol−1 and, finally, for wheat starch sample were 214.57 and 4.87 × 106 J kg−1 mol−1, respectively. They concluded that wheat starch acquired an extended dimension in the solution in comparison with canary seed starches. The chain flexibility factor and activation energy E a for basil seed gum solution at temperatures in the range 25–65 °C were 665.35 (1 K−1) and 5.5 × 106 J kg−1 mol−1, respectively [14].
The chain flexibility parameter and activation energy E a for A. homolocarpum seed gum at selected temperatures (25–65 °C) were obtained as 618.54 and 0.51 × 107 J kg−1 mol−1, respectively. The value calculated for the chain flexibility parameter of A. homolocarpum seed gum was approximately the same as the value of 645 reported for cellulose diacetate [99] and higher than the value of 488 accounted for chitosan [23], and lower than those reported for xanthan (1100) [97], Balangu seed gum (1156.53) [11], chitosan (1334) [96], chitosan (2300) [100], and sage seed gum (3046.45) [13]. The literature shows that there is a good relationship between M W and chain flexibility, and that the greater the molecular weight of hydrocolloids, the greater the flexibility [7].
2.8 Stiffness Parameter
The stiffness parameter (S) is calculated using Eq. (2.19) from the intrinsic viscosity's slope at various ionic strengths against the inverse square root of the ionic strength (I−0.5) plot [7]:
where [η] ∞ is the intrinsic viscosity of infinite ionic strength. Although the constant S is a measure of polymer stiffness, it is strongly molecular weight dependent. For this reason, Smidsrød and Haug [101] have introduced the relative chain stiffness (B) as an independent stiffness parameter which is calculated accordingly:
where the ν parameter was found to be in the range 1.2–1.4, in which the average value of 1.3 is broadly used as a constant number. The value of [η]0.1 is the intrinsic viscosity at an ionic strength of 0.1 M [86].
The obtained [η] ∞ for Balangu seed gum solutions in the presence of Na+ and Ca2+ cations was reported to be 591.26 and 164.80 ml g−1, respectively [11]. Also, the S value found to be 0.346 ml M0.5 g−1 in the presence of Na+ and 0.507 ml M0.5 g−1 in the presence of Ca2+. The higher value of S in the case of divalent ions indicates that it caused contraction in Balangu seed gum chain to a greater extent in comparison with monovalent ions. The lower value of S for Balangu seed gum compared to the value obtained for tragacanthin (0.60) by Mohammadifar et al. [86] shows higher flexibility for Balangu seed gum. The value of S for decolorized hsian‐tsao leaf gum was found to be within the range 58.17–60.46 ml M0.5 g−1, which was higher than the values reported for hsian‐tsao leaf gum (10.68–11.45 ml M0.5 g−1). They concluded that this issue may be due to the much higher uronic acid content (53.88%) of decolorized hsian‐tsao compared to that of crude hsian‐tsao leaf gum (23.73%). The relative chain stiffness parameter (B) for decolorized hsian‐tsao leaf gum in the presence of monovalent salt addition (Na+ and K+) was obtained as 0.03. This value is similar to the value reported for crude decolorized hsian‐tsao leaf gum and L‐gulopyranosyluronic acid, while lower than that for carboxymethylcellulose, carrageenan, and chitosan [92].
In the case of sage seed gum, Na+ and K+ ions had greater [η] ∞ compared with Ca2+ and Mg2+ ions, and the greatest value was obtained in KCl solution (4.42 dl g−1). The stiffness parameter (S) obtained for sage seed gum in NaCl solution (0.381 dl M0.5 g−1) is close to that reported for Balangu seed gum (0.346 dl M0.5 g−1) [11] and lower than that reported for tragacanthin (0.6) [86]. In addition, this parameter in the presence of CaCl2 for sage seed gum (0.821) is greater than that for Balangu seed gum (0.507) [11]. In general, greater flexibility is observed for emerging hydrocolloids in monovalent salt solutions compared with divalent ones.
2.9 Coil Radius and Volume
The following model (Eq. (2.21)) stated by Antoniou et al. [66] proposed to calculate the hydrodynamic coil radius, R coil :

where M w and N A are the polymer weight‐average molecular weight and Avogadro's number (6.022 × 1023 mol−1), respectively. The coil volume, V coil , can be determined by the following equation, with the assumption that the shape of the coil is sphere like [13]:
For Balangu seed gum, R coil was significantly decreased by increasing the temperature from 20 to 50 °C [13]. Furthermore, the presence of ions and sugars also diminished R coil and V coil drastically. At the same temperature (20 °C), the order of their influence in comparison with its corresponding value in deionized water found to be Ca2+ > Na+ > lactose > sucrose. It was concluded that the ions are more effective than the sugars and/or temperature in decreasing the coil dimensions. Similar results were reported for dextran by Antoniou et al. [66]. According to the results obtained for basil seed gum by Mirabolhassani et al. [14], R coil was almost not influenced by a temperature increment, although at 65 °C, this value decreased and contraction of basil seed gum coil was observed. They reported that this may be related to the increase in the instability of the hydrogen bonds between basil seed gum and water molecules and the relative increase in stability of intramolecular interactions among the polymer segments of basil seed gum [96]. In the case of canary seed starches, Heydari et al. [15] found that the starch obtained from CDC Maria variety had higher R coil (4.00 nm) and V coil (267.5 nm3) values rather than that of C05041. Overall, the R coil and V coil values of canary seed starches are rather smaller than those of several hydrocolloids such as sage seed gum (6.23 nm and 1012.35 nm3), Balangu seed gum (9.95 nm and 4121.3 nm3), and wheat starch (4.467 nm and 378.37 nm3). Also, by adding sugars and increasing their concentrations, the coil radius and coil volume were decreased significantly. The results of Hesarinejad et al. [21] revealed that the R coil of A. homolocarpum seed gum was reduced slightly as the temperature increased from 25 to 55 °C; however, a small increase accompanied a subsequent increase in temperature to 65 °C. In addition, in the case of the effect of cosolutes, it was found that as sucrose and lactose concentrations increase, the R coil of A. homolocarpum seed gum decreases. The V coil of A. homolocarpum seed gum decreased with an increase in the sugar concentration as well. The variations were more pronounced in the presence of sucrose rather than of lactose [102]. It is reported that salt concentration and salt type (mono or divalent) have a pronounced influence on the R coil and V coil values of sage seed gum macromolecules [13]. In divalent solutions and higher concentrations regardless of the salt type, these values reduce to a greater extent. The obtained R coil value for sage seed gum in 50 mM NaCl solution (4.96 nm) was similar to that for Balangu seed gum (4.60 nm), while it was different at the same concentration in CaCl2 solution (4.37 nm for SSG and 3.77 nm for Balangu seed gum) [11]. Antoniou et al. [66] found that the R coil value for dextran T500 in single and binary good + bad solvents at 20 °C was between 13.7 and 19.7 nm, which was much higher than the obtained R coil value for sage seed gum.
2.10 Voluminosity and Shape Factor
The voluminosity or swollen specific volume parameter (V E ) is a measure of the volume of a solvated polymer molecule, which could be obtained from the intercept of the plot of the Y value (as shown in Eq. (2.23)) versus C (concentration) [59]:

V E exhibits the conformation of a polymer in different solvent conditions. Eq. (2.24) reveals the relationship between V E and intrinsic viscosity through another parameter, which is known as the shape factor (υv) or the viscosity increment:
The shape of the polymer's particles in a solution can be estimated through the shape factor. It is believed that the solvent association causes an anhydrous macromolecule to expand when suspended or dissolved in solution, so V E can be considered as a measure of the solvent associated with the macromolecule; or by definition, it is the volume of macromolecule in solution per its unit anhydrous mass. The viscosity increment (υ) is called the “universal shape function,” since unlike intrinsic viscosity, it can be directly related to the shape of a particle independent of its volume [56]. From experimental observations, the physical meaning of υ can be summarized as follows: (1) a value of 2.5 for υ indicates a spherical shape, (2) a higher value is associated with an ellipsoidal shape, and (3) different values of υ suggest an oblate or prolate shape for a polymer coil [66].
For Balangu seed gum, with increasing temperature and in the presence of ions, the values of V E and υ decreased significantly. The results of the shape function suggested that the effective molecular shape of Balangu seed gum approaches a sphere‐like configuration (υ = 2.5) as the temperature increases, showing a greater contraction at higher temperatures. Similar results have been reported by Antoniou et al. [66] regarding the effect of temperature on the shape function of dextran. Also, in the case of sugars, an increase in the concentration of sucrose caused the V E and υ of Balangu seed gum to decrease; however, lactose did not follow a definite trend. Yousefi et al. [13] have stated that the shape factor of sage seed gum macromolecule at temperatures of 25–65 °C is an oblate or prolate, whereas, in the presence of slats, the shape is found to be roughly ellipsoidal. They observed that with increasing temperature from 25 to 65 °C, the shape factor value increased from 2.19 to 2.46, indicating the lesser flexibility of sage seed gum macromolecules at higher temperatures. In a poor solvent, the monomers of individual polymers effectively attract each other to minimize their contacts with the solvent molecules, so a roughly spherical or ellipsoidal shape forms which has less flexibility [103]. For basil seed gum, the values of V E and υ were not affected by temperature; however, a remarkable reduction was seen for the υ parameter at 65 °C. The results obtained for the υ parameter indicated that the effective molecular shape of basil seed gum approaches a sphere‐like configuration (υ = 2.5) as the temperature increases, showing more contraction at higher temperatures. By increasing the sucrose or lactose concentration, the V E value of basil seed gum increased, whereas the υ value decreased [14]. In the case of canary seed starch, it is reported that by raising the temperature, the V E value declined, indicating that the coil dimension of canary seed starches and/or the solvent power decreased. Canary seed starches had a υ value of around 2.5 at 25 °C, indicating that the shape factor of the samples at this temperature was spherical. With increasing temperature, the υ value increased, showing that the higher the temperatures, the less the flexibility of canary starches. In addition, at 25 °C, the shape factor value of canary seed starches decreased slightly in the presence of sugars, although it did not obey a regular trend in some cases [15]. Hesarinejad et al. [21] have stated that V E and υ of A. homolocarpum seed gum diminish slightly up to 55 °C. The results obtained for the shape function indicate that as the temperature increases, the influential molecular shape of A. homolocarpum seed gum approaches a sphere‐like configuration, depicting more contraction at higher temperatures. In another study, Hesarinejad et al. [102] reported that the V E value of A. homolocarpum seed gum decreased with increasing sucrose and lactose concentration, which demonstrates the negative influence of these sugars on the volume of A. homolocarpum seed gum molecules. The value of υ in deionized water was less than 2.5, showing the spherical shape of the molecule, while this value was more than 2.5 in sucrose and lactose solutions, indicating that the molecules tended to be ellipsoidal in shape.
2.11 Hydration Parameter
The associated solvent with the macromolecule in solution can be regarded as that which is either chemically attached through hydrogen bonds or physically absorbed by the macromolecule, and its extent could be represented by the hydration parameter (δ). This parameter is considered as the level to which aqueous solvent can be added to a dry macromolecule beyond which there is no change in a macromolecular property other than dilution of the sample. It is possible to assign a value for δ (dimensionless) from viscosity measurements by the following relation [56]:
where ρ
s
is the density (g dl−1), V
E
is the swollen specific volume or voluminosity (dl g−1), and ![]() is the partial specific volume (dl g−1).
is the partial specific volume (dl g−1).
It was observed by Hesarinejad et al. [21] that the value of δ for A. homolocarpum seed gum decreases by increasing the temperature up to 55 °C. The results obtained regarding the A. homolocarpum seed gum hydration parameter at 65 °C suggest the plausible reduction in the associated solvent through hydrogen bonds and/or physical entrainment, leading to an enhancement in the intermolecular interactions (i.e., aggregation) between unsolvated chains. Similar results have been obtained for basil seed gum at 65 °C [14]. The value of δ for Balangu seed gum was found to decrease with rising temperature and ionic strength, although the reduction in the δ parameter affected by ionic strength was more pronounced [14]. They reported that the δ parameter was not influenced by sucrose concentration, while this value decreased slightly with an increase in lactose concentration. In the case of canary seed starches, the δ value of CDC Maria variety was more influenced by lactose concentration rather than by sucrose concentration, while its value for the C05041 variety diminished further upon increasing the concentration of sucrose [15]. Sugars might compact the molecules by penetrating into the amorphous regions of the starch molecules and create bridges, thereby decreasing the hydration volume and as a result, reducing viscosity [104].
2.12 Conclusion and Future Trends
In this chapter, the dilute solution properties of some emerging natural hydrocolloids under various conditions were investigated. Among the emerging natural hydrocolloids, the partial specific volumes of Balangu seed gum (0.61 ml g−1) and basil seed gum (0.62 ml g−1) were found to be greater than those of others which were almost close to the values reported for xanthan (0.60 ml g−1) and pectin (0.57 ml g−1). The hydrogel content of basil seed gum (73.6%) in deionized water was near to that of L. sativum seed gum (76%), and these values were greater than that of Balangu seed gum (46%). The hydrogel content of novel hydrocolloids was strongly affected by the type and concentration of cosolutes in the solutions. In the case of the molecular weight (M w ), it was found that Balangu seed gum had higher M w (3.65 × 106 g mol−1) than other emerging hydrocolloids and also many other common hydrocolloids as well. The intrinsic viscosity of emerging hydrocolloids can be classified into (1) hydrocolloids with high intrinsic viscosity value (Balangu seed gum), (2) hydrocolloids with medium value of intrinsic viscosity (A. homolocarpum seed gum, chia seed gum, basil seed gum, sage seed gum), and (3) hydrocolloids with low intrinsic viscosity value (canary seed starch, hsian‐tsao leaf gum, cress seed gum), as compared with the intrinsic viscosity values reported for common hydrocolloids. The reported results clearly demonstrated that the intrinsic viscosity of emerging hydrocolloids is remarkably influenced by the type and concentration of cosolutes such as salts and sugars in solutions and by temperature as well. The Berry number value reported for novel hydrocolloids in the dilute solution regime indicated that their molecular conformations were between the random coil and rod‐like. The obtained values of the chain flexibility parameter (E a /RT) and activation energy (E a ) for sage seed gum were 3046.45 and 2.53 × 107 J kg−1 mol−1, respectively, which were greater than those of other emerging hydrocolloids. The stiffness parameter (S) values of Balangu seed gum and sage seed gum were found to be almost similar, especially in the presence of monovalent cations, but were much lower than that of hsian‐tsao leaf gum. The coil radius and volume of the emerging hydrocolloids were diminished in the presence of salts and sugars, and Balangu seed gum was found to have a greater R coil (9.95 nm) and V coil (4121.3 nm3) than others. The voluminosity and shape factor of novel hydrocolloids were influenced by temperature and the type of cosolutes in solutions. For Balangu seed gum and A. homolocarpum seed gum, the value of the hydration parameter (δ) was found to decrease with increasing temperature and ionic strength, but the reduction in the δ parameter affected by ionic strength was more pronounced. The various properties and characteristics of emerging natural hydrocolloids reported in this chapter show that they have good potential to be used as thickening, stabilizing, and gelling agents as compared with commercial ones.
Although hydrocolloids have historically been used in food systems to control rheological properties and texture, consumers are being made increasingly aware of their nutritional benefits. Most of the emerging natural hydrocolloids investigated in this chapter have nutritional and pharmaceutical benefits. In addition, owing to safety, availability, low processing costs, and good functionality, it seems that emerging natural hydrocolloids have excellent potential for application as additives for various food, cosmetics, and pharmaceutical systems. Moreover, due to the distinctive characteristics, it is hoped that in many cases emerging natural hydrocolloids will be easily used as a substitute for common hydrocolloids.
References
- 1 Yousefi, A.R., Eivazlou, R., and Razavi, S.M.A. (2016). Steady shear flow behavior of sage seed gum affected by various salts and sugars: time‐independent properties. International Journal of Biological Macromolecules 91: 1018–1024.
- 2 Yousefi, A.R. and Razavi, S.M.A. (2016). Steady shear flow behavior and thixotropy of wheat starch gel: impact of chemical modification, concentration and saliva addition. Journal of Food Process Engineering 39: 31–43.
- 3 Yousefi, A.R. and Razavi, S.M.A. (2015). Dynamic rheological properties of wheat starch gels as affected by chemical modification and concentration. Starch‐Stärke 67: 567–576.
- 4 Yousefi, A.R., Razavi, S.M.A., and Norouzy, A. (2015). In vitro gastrointestinal digestibility of native, hydroxypropylated and cross‐linked wheat starches. Food & Function 6: 3126–3134.
- 5 Yousefi, A.R. and Razavi, S.M.A. (2017). Modeling of glucose release from native and modified wheat starch gels during in vitro gastrointestinal digestion using artificial intelligence methods. International Journal of Biological Macromolecules 97: 752–760.
- 6 Moosavi‐Nasab, M. and Yousefi, A.R. (2011). Biotechnological production of cellulose by Gluconacetobacter xylinus from agricultural waste. Iranian Journal of Biotechnology 9: 94–101.
- 7 Lai, L.‐S. and Chiang, H.‐F. (2002). Rheology of decolorized hsian‐tsao leaf gum in the dilute domain. Food Hydrocolloids 16: 427–440.
- 8 Behrouzian, F., Razavi, S.M., and Karazhiyan, H. (2014). Intrinsic viscosity of cress (Lepidium sativum) seed gum: effect of salts and sugars. Food Hydrocolloids 35: 100–105.
- 9 Ghante, M.H., Badole, S.L., and Bodhankar, S.L. (2011). Nuts and Seeds in Health and Disease Prevention, 521–525. Elsevier.
- 10 Naghibi, F., Mosaddegh, M., Mohammadi Motamed, M., and Ghorbani, A. (2010). Labiatae family in folk medicine in Iran: from ethnobotany to pharmacology. Iranian Journal of Pharmaceutical Research 63–79.
- 11 Amini, A.M. and Razavi, S.M. (2012). Dilute solution properties of Balangu (Lallemantia royleana) seed gum: effect of temperature, salt, and sugar. International Journal of Biological Macromolecules 51: 235–243.
- 12 Koocheki, A., Mortazavi, S.A., Shahidi, F. et al. (2009). Rheological properties of mucilage extracted from Alyssum homolocarpum seed as a new source of thickening agent. Journal of Food Engineering 91: 490–496.
- 13 Yousefi, A.R., Razavi, S.M.A., and Khodabakhsh Aghdam, S. (2014). Influence of temperature, mono‐and divalent cations on dilute solution properties of sage seed gum. International Journal of Biological Macromolecules 67: 246–253.
- 14 Mirabolhassani, S.E., Rafe, A., and Razavi, S.M.A. (2016). The influence of temperature, sucrose and lactose on dilute solution properties of basil (Ocimum basilicum) seed gum. International Journal of Biological Macromolecules 93: 623–629.
- 15 Heydari, A., Razavi, S.M.A., and Irani, M. Effect of temperature and selected sugars on dilute solution properties of two hairless canary seed starches compared with wheat starch. International Journal of Biological Macromolecules 2017.
- 16 Doublier, J. and Cuvelier, G. (2006). Gums and hydrocolloids: functional aspects. In: Carbohydrates in Food, 2e (ed. A.‐C. Eliasson), 233. New York: Marcel Dekker.
- 17 Gaisford, S.E., Harding, S.E., Mitchell, J.R., and Bradley, T.D. (1986). A comparison between the hot and cold water soluble fractions of two locust bean gum samples. Carbohydrate Polymers 6: 423–442.
- 18 Karazhiyan, H., Razavi, S., Phillips, G.O. et al. (2011). Physicochemical aspects of hydrocolloid extract from the seeds of Lepidium sativum. International Journal of Food Science and Technology 46: 1066–1072.
- 19 Higiro, J., Herald, T., Alavi, S., and Bean, S. (2007). Rheological study of xanthan and locust bean gum interaction in dilute solution: effect of salt. Food Research International 40: 435–447.
- 20 Stephen, A.M. (1995). Food Polysaccharides and Their Applications. CRC Press.
- 21 Hesarinejad, M.A., Razavi, S.M., and Koocheki, A. (2015). Alyssum homolocarpum seed gum: dilute solution and some physicochemical properties. International Journal of Biological Macromolecules 81: 418–426.
- 22 Pavlov, G.M., Korneeva, E.V., Harding, S.E., and Vichoreva, G.A. (1998). Dilute solution properties of carboxymethylchitins in high ionic‐strength solvent. Polymer 39: 6951–6961.
- 23 Pogodina, N., Pavlov, G., Bushin, S. et al. (1986). Conformational characteristics of chitosan molecules as demonstrated by diffusion‐sedimentation analysis and viscometry. Polymer Science U.S.S.R. 28: 251–259.
- 24 Harding, S.E., Berth, G., Ball, A. et al. (1991). The molecular weight distribution and conformation of citrus pectins in solution studied by hydrodynamics. Carbohydrate Polymers 16: 1–15.
- 25 Morris, G.A., Foster, T.J., and Harding, S.E. (2002). A hydrodynamic study of the depolymerisation of a high methoxy pectin at elevated temperatures. Carbohydrate Polymers 48: 361–367.
- 26 Cowman, M.K. and Matsuoka, S. (2005). Experimental approaches to hyaluronan structure. Carbohydrate Research 340: 791–809.
- 27 Kök, M.S., Abdelhameed, A.S., Ang, S. et al. (2009). A novel global hydrodynamic analysis of the molecular flexibility of the dietary fibre polysaccharide konjac glucomannan. Food Hydrocolloids 23: 1910–1917.
- 28 Dhami, R., Harding, S.E., Jones, T. et al. (1995). Physico‐chemical studies on a commercial food‐grade xanthan – I. Characterisation by sedimentation velocity, sedimentation equilibrium and viscometry. Carbohydrate Polymers 27: 93–99.
- 29 Durchschlag, H. (1989). Determination of the partial specific volume of conjugated proteins. Colloid and Polymer Science 267: 1139–1150.
- 30 Ahmed, E.M. (2015). Hydrogel: preparation, characterization, and applications: a review. Journal of Advanced Research 6: 105–121.
- 31 Lee, P.I. (1991). Absorbent polymer technology: Lisa Brannon‐Peppas and Ronald S. Harland (Eds.), Elsevier Science Publishers B.V., Amsterdam, 1990, 278 pp. $107.75 or Dfl.210.00. Journal of Controlled Release 17: 297–298.
- 32 Li, Y., Huang, G., Zhang, X. et al. (2013). Magnetic hydrogels and their potential biomedical applications. Advanced Functional Materials 23: 660–672.
- 33 Arbona, V., Iglesias, D.J., Jacas, J. et al. (2005). Hydrogel substrate amendment alleviates drought effects on young citrus plants. Plant and Soil 270: 73–82.
- 34 Zohuriaan‐Mehr, M., Pourjavadi, A., Salimi, H., and Kurdtabar, M. (2009). Protein‐and homo poly(amino acid)‐based hydrogels with super‐swelling properties. Polymers for Advanced Technologies 20: 655–671.
- 35 Jayakumar, R., Prabaharan, M., Kumar, P.S. et al. (2011). Biomaterials based on chitin and chitosan in wound dressing applications. Biotechnology Advances 29: 322–337.
- 36 Lee, K.Y. and Mooney, D.J. (2001). Hydrogels for tissue engineering. Chemical Reviews 101: 1869–1880.
- 37 Li, G., Du, Y., Tao, Y. et al. (2010). Iron (II) cross‐linked chitin‐based gel beads: preparation, magnetic property and adsorption of methyl orange. Carbohydrate Polymers 82: 706–713.
- 38 Paulino, A.T., Guilherme, M.R., Reis, A.V. et al. (2006). Removal of methylene blue dye from an aqueous media using superabsorbent hydrogel supported on modified polysaccharide. Journal of Colloid and Interface Science 301: 55–62.
- 39 Dwivedi, C., Gupta, A., Chaudhary, A., and Nandi, C.K. (2014). Gold nanoparticle chitosan composite hydrogel beads show efficient removal of methyl parathion from waste water. RSC Advances 4: 39830–39838.
- 40 Adhikari, B. and Majumdar, S. (2004). Polymers in sensor applications. Progress in Polymer Science 29: 699–766.
- 41 Shen, X., Shamshina, J.L., Berton, P. et al. (2016). Hydrogels based on cellulose and chitin: fabrication, properties, and applications. Green Chemistry 18: 53–75.
- 42 Sperling, L.H. (2005). Introduction to Physical Polymer Science. Wiley.
- 43 Oberlerchner, J.T., Rosenau, T., and Potthast, A. (2015). Overview of methods for the direct molar mass determination of cellulose. Molecules 20: 10313–10341.
- 44 Schindler, A. and Harper, D. (1979). Polylactide. II. Viscosity–molecular weight relationships and unperturbed chain dimensions. Journal of Polymer Science Part A: Polymer Chemistry 17: 2593–2599.
- 45 Moore, J. (1996). Gel permeation chromatography. I. A new method for molecular weight distribution of high polymers. Journal of Polymer Science Part A: Polymer Chemistry 34: 1833–1841.
- 46 Dentini, M., Coviello, T., Burchard, W., and Crescenzi, V. (1988). Solution properties of exocellular microbial polysaccharides. 3. Light scattering from gellan and from the exocellular polysaccharide of Rhizobium trifolii (strain TA‐1) in the ordered state. Macromolecules 21: 3312–3320.
- 47 Buliga, G.S. and Brant, D.A. (1987). Temperature and molecular weight dependence of the unperturbed dimensions of aqueous pullulan. International Journal of Biological Macromolecules 9: 71–76.
- 48 Axelos, M. and Branger, M. (1993). The effect of the degree of esterification on the thermal stability and chain conformation of pectins. Food Hydrocolloids 7: 91–102.
- 49 Razavi, S.M.A., Cui, S.W., Guo, Q., and Ding, H. (2014). Some physicochemical properties of sage (Salvia macrosiphon) seed gum. Food Hydrocolloids 35: 453–462.
- 50 López‐Franco, Y., Cervantes‐Montaño, C., Martínez‐Robinson, K. et al. (2013). Physicochemical characterization and functional properties of galactomannans from mesquite seeds (Prosopis spp.). Food Hydrocolloids 30: 656–660.
- 51 Brummer, Y., Cui, W., and Wang, Q. (2003). Extraction, purification and physicochemical characterization of fenugreek gum. Food Hydrocolloids 17: 229–236.
- 52 Wu, Y., Cui, W., Eskin, N., and Goff, H. (2009). An investigation of four commercial galactomannans on their emulsion and rheological properties. Food Research International 42: 1141–1146.
- 53 Lee, J. and Tripathi, A. (2005). Intrinsic viscosity of polymers and biopolymers measured by microchip. Analytical Chemistry 77: 7137–7147.
- 54 Yamakawa, H. (1971). Modern Theory of Polymer Solutions. Harper & Row.
- 55 Bustamante, P., Navarro‐Lupión, J., and Escalera, B. (2005). A new method to determine the partial solubility parameters of polymers from intrinsic viscosity. European Journal of Pharmaceutical Sciences 24: 229–237.
- 56 Harding, S.E. (1997). The intrinsic viscosity of biological macromolecules. Progress in measurement, interpretation and application to structure in dilute solution. Progress in Biophysics and Molecular Biology 68: 207–262.
- 57 Huggins, M.L. (1942). The viscosity of dilute solutions of long‐chain molecules. IV. Dependence on concentration. Journal of the American Chemical Society 64: 2716–2718.
- 58 Kraemer, E.O. (1938). Molecular weights of celluloses and cellulose derivates. Industrial & Engineering Chemistry 30: 1200–1203.
- 59 Razavi, S.M., Moghaddam, T.M., Emadzadeh, B., and Salehi, F. (2012). Dilute solution properties of wild sage (Salvia macrosiphon) seed gum. Food Hydrocolloids 29: 205–210.
- 60 McMillan, D.E. (1974). A comparison of five methods for obtaining the intrinsic viscosity of bovine serum albumin. Biopolymers 13: 1367–1376.
- 61 Tanglertpaibul, T. and Rao, M. (1987). Intrinsic viscosity of tomato serum as affected by methods of determination and methods of processing concentrates. Journal of Food Science 52: 1642–1645.
- 62 Wang, W.‐C., Reinhall, P.G., and Yee, S. (1999). Fluid viscosity measurement using forward light scattering. Measurement Science and Technology 10: 316.
- 63 Irani, M., Razavi, S.M., Abdel‐Aal, E.‐S.M. et al. (2016). Dilute solution properties of canary seed (Phalaris canariensis) starch in comparison to wheat starch. International Journal of Biological Macromolecules 87: 123–129.
- 64 Timilsena, Y.P., Adhikari, R., Kasapis, S., and Adhikari, B. (2015). Rheological and microstructural properties of the chia seed polysaccharide. International Journal of Biological Macromolecules 81: 991–999.
- 65 Kasaai, M.R. (2008). Calculation of viscometric constants, hydrodynamic volume, polymer–solvent interaction parameter, and expansion factor for three polysaccharides with different chain conformations. Carbohydrate Research 343: 2266–2277.
- 66 Antoniou, E., Themistou, E., Sarkar, B. et al. (2010). Structure and dynamics of dextran in binary mixtures of a good and a bad solvent. Colloid and Polymer Science 288: 1301–1312.
- 67 Ogawa, E., Takahashi, R., Yajima, H., and Nishinari, K. (2006). Effects of molar mass on the coil to helix transition of sodium‐type gellan gums in aqueous solutions. Food Hydrocolloids 20: 378–385.
- 68 Cleland, R.L. (1979). Effect of temperature on the limiting viscosity number of hyaluronic acid and chondroitin 4‐sulfate. Biopolymers 18: 1821–1828.
- 69 Goycoolea, F., Morris, E., Richardson, R., and Bell, A. (1995). Solution rheology of mesquite gum in comparison with gum arabic. Carbohydrate Polymers 27: 37–45.
- 70 Stivala, S.S. and Bahary, W.S. (1978). Some dilute‐solution parameters of the levan of Streptococcus salivarius in various solvents. Carbohydrate Research 67: 17–21.
- 71 Haug, A. and Smidsrod, O. (1962). Determination of intrinsic viscosity of alginates. Acta Chemica Scandinavica 16: 1569–1578.
- 72 Elfak, A.M., Pass, G., and Morley, R.G. (1977). The viscosity of dilute solutions of guar gum and locust bean gum with and without added sugars. Journal of the Science of Food and Agriculture 28: 895–899.
- 73 Grimm, A., Krüger, E., and Burchard, W. (1995). Solution properties of β‐d‐(1,3)(1,4)‐glucan isolated from beer. Carbohydrate Polymers 27: 205–214.
- 74 Richardson, P.H., Willmer, J., and Foster, T.J. (1998). Dilute solution properties of guar and locust bean gum in sucrose solutions. Food Hydrocolloids 12: 339–348.
- 75 Chen, T.‐S. and Joslyn, M. (1967). The effect of sugars on viscosity of pectin solutions: I. Comparison of corn syrup with sucrose solutions. Journal of Colloid and Interface Science 23: 399–406.
- 76 Launay, B., Cuvelier, G., and Martinez‐Reyes, S. (1997). Viscosity of locust bean, guar and xanthan gum solutions in the Newtonian domain: a critical examination of the log (η sp) o ‐log c[η] o master curves. Carbohydrate Polymers 34: 385–395.
- 77 Achayuthakan, P., Suphantharika, M., and Rao, M. (2006). Yield stress components of waxy corn starch–xanthan mixtures: effect of xanthan concentration and different starches. Carbohydrate Polymers 65: 469–478.
- 78 Viturawong, Y., Achayuthakan, P., and Suphantharika, M. (2008). Gelatinization and rheological properties of rice starch/xanthan mixtures: effects of molecular weight of xanthan and different salts. Food Chemistry 111: 106–114.
- 79 Sittikijyothin, W., Torres, D., and Gonçalves, M. (2005). Modelling the rheological behaviour of galactomannan aqueous solutions. Carbohydrate Polymers 59: 339–350.
- 80 Hwang, J. and Kokini, J.L. (1992). Contribution of the side branches to rheological properties of pectins. Carbohydrate Polymers 19: 41–50.
- 81 Sato, A.C., Oliveira, P.R., and Cunha, R.L. (2008). Rheology of mixed pectin solutions. Food Biophysics 3: 100.
- 82 Yoo, S.‐H., Fishman, M.L., Hotchkiss, A.T., and Lee, H.G. (2006). Viscometric behavior of high‐methoxy and low‐methoxy pectin solutions. Food Hydrocolloids 20: 62–67.
- 83 Nickerson, M., Paulson, A., and Hallett, F. (2004). Dilute solution properties of κ‐carrageenan polysaccharides: effect of potassium and calcium ions on chain conformation. Carbohydrate Polymers 58: 25–33.
- 84 Wang, Q., Ellis, P.R., Ross‐Murphy, S.B., and Burchard, W. (1997). Solution characteristics of the xyloglucan extracted from Detarium senegalense Gmelin. Carbohydrate Polymers 33: 115–124.
- 85 Jumel, K., Harding, S.E., Mitchell, J.R. et al. (1996). Molar mass and viscometric characterisation of hydroxypropylmethyl cellulose. Carbohydrate Polymers 29: 105–109.
- 86 Mohammadifar, M.A., Mousavi, S.M., Kiumarsi, A., and Williams, P.A. (2006). Solution properties of tragacanthin (water‐soluble part of gum tragacanth exudate from Astragalus gossypinus). International Journal of Biological Macromolecules 38: 31–39.
- 87 Pamies, R., Cifre, J.G.H., Martínez, M.d.C.L., and de la Torre, J.G. (2008). Determination of intrinsic viscosities of macromolecules and nanoparticles. Comparison of single‐point and dilution procedures. Colloid and Polymer Science 286: 1223–1231.
- 88 Morris, E., Cutler, A., Ross‐Murphy, S. et al. (1981). Concentration and shear rate dependence of viscosity in random coil polysaccharide solutions. Carbohydrate Polymers 1: 5–21.
- 89 Wang, F., Sun, Z., and Wang, Y.‐J. (2001). Study of xanthan gum/waxy corn starch interaction in solution by viscometry. Food Hydrocolloids 15: 575–581.
- 90 Millard, M.M., Dintzis, F.R., Willett, J., and Klavons, J.A. (1997). Light‐scattering molecular weights and intrinsic viscosities of processed waxy maize starches in 90% dimethyl sulfoxide and H2O. Cereal Chemistry 74: 687–691.
- 91 Cheng, Y., Brown, K.M., and Prud'homme, R.K. (2002). Preparation and characterization of molecular weight fractions of guar galactomannans using acid and enzymatic hydrolysis. International Journal of Biological Macromolecules 31: 29–35.
- 92 Lapasin, R. and Pricl, S. (1995). Rheology of Industrial Polysaccharides: Theory and Applications, 250–494. Springer.
- 93 Hager, B. and Berry, G. (1982). Moderately concentrated solutions of polystyrene. I. Viscosity as a function of concentration, temperature, and molecular weight. Journal of Polymer Science Part B: Polymer Physics 20: 911–928.
- 94 Karazhiyan, H., Razavi, S.M., Phillips, G.O. et al. (2009). Rheological properties of Lepidium sativum seed extract as a function of concentration, temperature and time. Food Hydrocolloids 23: 2062–2068.
- 95 Higiro, J., Herald, T., and Alavi, S. (2006). Rheological study of xanthan and locust bean gum interaction in dilute solution. Food Research International 39: 165–175.
- 96 Chen, R.H. and Tsaih, M.L. (1998). Effect of temperature on the intrinsic viscosity and conformation of chitosans in dilute HCl solution. International Journal of Biological Macromolecules 23: 135–141.
- 97 Milas, M. and Rinaudo, M. (1986). Properties of xanthan gum in aqueous solutions: role of the conformational transition. Carbohydrate Research 158: 191–204.
- 98 Wang, W. and Xu, D. (1994). Viscosity and flow properties of concentrated solutions of chitosan with different degrees of deacetylation. International Journal of Biological Macromolecules 16: 149–152.
- 99 Kamide, K. and Saito, M. (1987). Biopolymers, 1–56. Springer.
- 100 Skjåk‐Bræk, G., Anthonsen, T., and Sandford, P. (1989). Chitin and Chitosan. London: Elsevier.
- 101 Smidsrød, O. and Haug, A. (1971). Estimation of the relative stiffness of the molecular chain in polyelectrolytes from measurements of viscosity at different ionic strengths. Biopolymers 10: 1213–1227.
- 102 Hesarinejad, M.A., Razavi, S.M.A., Koocheki, A., and Mohammadifar, M.A. (2017). A study on the effects of sucrose and lactose on the rheological properties of Alyssum homolocarpum seed gum in dilute solutions. Iranian Food Science and Technology Research Journal 1396.
- 103 Montesi, A., Pasquali, M., and MacKintosh, F. (2004). Collapse of a semiflexible polymer in poor solvent. Physical Review E 69: 021916.
- 104 Spies, R. (1982). Effect of sugars on starch gelatinization. Cereal Chemistry 59: 128–131.
