CHAPTER III
SYMMETRIC SPACES OF RANK ONE
1. Flat tori
Let (X, g) be a flat Riemannian manifold of dimension n. We first suppose that X is the circle S1 of length ![]() endowed with the Riemannian metric
endowed with the Riemannian metric ![]() where t is the canonical coordinate of S1 defined modulo L. It is easily seen that this space X is infinitesimally rigid
and that a 1-form on X satisfies the zero-energy condition if and only if it is exact.
where t is the canonical coordinate of S1 defined modulo L. It is easily seen that this space X is infinitesimally rigid
and that a 1-form on X satisfies the zero-energy condition if and only if it is exact.
In this section, we henceforth suppose that n 2. We recall that ![]() that the operator D1 is equal to Dg, and that the sequence (1.50) is exact. Let h be a section of
that the operator D1 is equal to Dg, and that the sequence (1.50) is exact. Let h be a section of ![]() over an open subset of X. According to formulas (1.20) and (1.21), we see that
over an open subset of X. According to formulas (1.20) and (1.21), we see that ![]() is equal to the section
is equal to the section ![]() of B and that
of B and that

In the remainder of this section, we suppose that (X, g) is a flat torus of dimensio![]() n 2. We may consider X as the quotient of the space
n 2. We may consider X as the quotient of the space ![]() endowed with the Euclidean metric g0. In fact, there is a latti
endowed with the Euclidean metric g0. In fact, there is a latti![]() ce generated by a basis
ce generated by a basis ![]() such that X is equal to the quotient
such that X is equal to the quotient![]() We shall identify a tensor on X with the
We shall identify a tensor on X with the![]() -invariant tensor on
-invariant tensor on ![]() which it determines. Clearly, a tensor on
which it determines. Clearly, a tensor on ![]() which is invariant under the group of all translations of
which is invariant under the group of all translations of ![]() induces a tensor on X. Let
induces a tensor on X. Let ![]() be the standard coordinate system of
be the standard coordinate system of ![]() In particular, for
In particular, for ![]() the vector field
the vector field ![]() and the 1-form dxj on
and the 1-form dxj on ![]() are invariant under the group of all translations of
are invariant under the group of all translations of ![]() and therefore induce a parallel vector field and a parallel 1-form on X, which we shall denote by
and therefore induce a parallel vector field and a parallel 1-form on X, which we shall denote by ![]() j and
j and ![]() j , respectively. Clearly,
j , respectively. Clearly,

where the coefficients![]() and
and ![]() are real numbers. Hence the space of all parallel sections of
are real numbers. Hence the space of all parallel sections of ![]() (resp. of
(resp. of ![]() over X is isomorphic to the space of all differential forms of degree k (resp. of all symmetric p-forms) on
over X is isomorphic to the space of all differential forms of degree k (resp. of all symmetric p-forms) on ![]() with constant coefficients. In particular, the metric g is equal to the symmetric 2-form
with constant coefficients. In particular, the metric g is equal to the symmetric 2-form ![]() Since the cohomology group
Since the cohomology group ![]() is isomorphic to
is isomorphic to ![]() , where x is an arbitrary point of X, we know that the space of harmonic forms of degree k on X is equal to the space of all
parallel sections of
, where x is an arbitrary point of X, we know that the space of harmonic forms of degree k on X is equal to the space of all
parallel sections of ![]() over X.
over X.
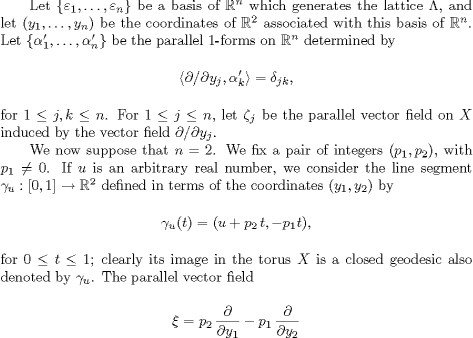
PROPOSITION 3.1. Let X be a flat torus of dimensi![]() on 2. Let
on 2. Let ![]() be a vector field on X. Then
be a vector field on X. Then ![]() is a Killing vector field if and only if
is a Killing vector field if and only if ![]()
PROOF: We consider the 1-for![]() on X. First, we suppose that X is a flat torus of dimension 2 and we consider the volume form =
on X. First, we suppose that X is a flat torus of dimension 2 and we consider the volume form = ![]() of X. According to formula (1.4), the 1-form satisfies the relations
of X. According to formula (1.4), the 1-form satisfies the relations ![]() and
and ![]() Since a harmonic 1-form on X is parallel, we may write
Since a harmonic 1-form on X is parallel, we may write

Y From the first of the preceding equations, we infer that the function f on ![]()
2 can be written in the form f ![]() where fj is a real-valued function on
where fj is a real-valued function on![]() depending only on xj . Then the second equality tells us that the function f1 - f2 on
depending only on xj . Then the second equality tells us that the function f1 - f2 on ![]() is harmonic and so is constant. It follows that the two functions f1 and f2 are also constant, and so the 1-form
is harmonic and so is constant. It follows that the two functions f1 and f2 are also constant, and so the 1-form![]() and the vector fiel
and the vector fiel![]() d are parallel. Now, we assume that the dimension of X is
d are parallel. Now, we assume that the dimension of X is![]() 2. Let x0 be a point of X and let
2. Let x0 be a point of X and let ![]() 1 and
1 and ![]() be parallel vector fields on X; then there is a totally geodesic flat 2-torus Y of X containing x0 such that the vectors
be parallel vector fields on X; then there is a totally geodesic flat 2-torus Y of X containing x0 such that the vectors![]() an
an![]() 2(x0) are tangent to Y . We consider the vector fiel
2(x0) are tangent to Y . We consider the vector fiel![]() on Y , whose value at x
on Y , whose value at x![]() is equal to the orthogonal projection of
is equal to the orthogonal projection of ![]() onto the subspace
onto the subspace ![]() of Tx. If
of Tx. If ![]() is the natural imbedding and
is the natural imbedding and ![]() is the Riemannian metric on Y induced by g, according to Lemma 1.1 we have
is the Riemannian metric on Y induced by g, according to Lemma 1.1 we have ![]() and we know that is a Killing vector field on Y . Therefore the 1-form
and we know that is a Killing vector field on Y . Therefore the 1-form ![]() on Y is parallel. Since i is a totally geodesic imbedding, it follows that
on Y is parallel. Since i is a totally geodesic imbedding, it follows that
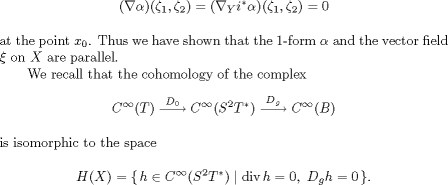
PROPOSITION 3.2. Let X be a flat torus of dimensi![]() on 2. Let h be a symmetric 2-form on X. Then the following assertions are equivalent:
on 2. Let h be a symmetric 2-form on X. Then the following assertions are equivalent:
(i) We have ![]() h = 0.
h = 0.
(ii) We have ![]() h = 0.
h = 0.
(iii) The section h belongs to H(X).
PROOF: Let ![]() , be parallel vector fields on X. If f is the real-valued function on X equal to
, be parallel vector fields on X. If f is the real-valued function on X equal to ![]() according to formula (1.52), we obtain the relation
according to formula (1.52), we obtain the relation
![]()
Therefore if ![]() h vanishes, the function f is constant; since the parallel vector field
h vanishes, the function f is constant; since the parallel vector field![]() s and are arbitrary, we see that
s and are arbitrary, we see that ![]() vanishes. If h is a parallel section of
vanishes. If h is a parallel section of ![]() according to (3.1) we see that Dgh = 0, and so h belongs to H(X). Finally, suppose that (iii) holds. Then according to (3.3),
we see that Tr h
according to (3.1) we see that Dgh = 0, and so h belongs to H(X). Finally, suppose that (iii) holds. Then according to (3.3),
we see that Tr h ![]() therefore Tr h is constant. Formula (3.2) now tells us that
therefore Tr h is constant. Formula (3.2) now tells us that ![]()
From Proposition 3.2, it follows that the space H(X) is equal to the space
![]()
of all parallel sections of ![]() According to remarks made in 3, Chapter I, we know that the cohomology group
According to remarks made in 3, Chapter I, we know that the cohomology group ![]() is isomorphic to this space, and therefore also to the vector space
is isomorphic to this space, and therefore also to the vector space ![]() where x is an arbitrary point of X; thus the dimension of this cohomology group is equal to n(n + 1)/2. Other proofs of these
results are given in [2] and [15] (see Proposition 17.1 of [15]). From Proposition 3.2 and the decomposition (1.11), it follows
that an element h of
where x is an arbitrary point of X; thus the dimension of this cohomology group is equal to n(n + 1)/2. Other proofs of these
results are given in [2] and [15] (see Proposition 17.1 of [15]). From Proposition 3.2 and the decomposition (1.11), it follows
that an element h of ![]() satisfying Dgh = 0 can be written in the form
satisfying Dgh = 0 can be written in the form
![]()
where ![]() is a vector field on X and h0 is a parallel section of
is a vector field on X and h0 is a parallel section of ![]() over X.
over X.
LEMMA 3.3. Let u be a parallel symmetric p-form on a flat torus X of dimensio![]() n 2. If the form u satisfies the zero-energy condition, then it vanishes.
n 2. If the form u satisfies the zero-energy condition, then it vanishes.
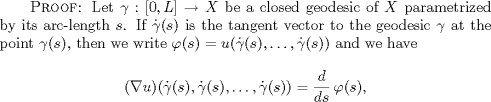
for all 0 s![]() L. Our hypothesis tells us that the functio
L. Our hypothesis tells us that the functio![]() n on [0,L] is constant. If u over the interval [0,L] vanishes, and hence the expression
n on [0,L] is constant. If u over the interval [0,L] vanishes, and hence the expression ![]() vanishes for all
vanishes for all ![]() L. If x is a point of X, we know that the set Cx of vectors of Tx - {0}, for which
L. If x is a point of X, we know that the set Cx of vectors of Tx - {0}, for which ![]() is a closed geodesic of X, is a dense subset of Tx. From these last two observations, we obtain the desired result.
is a closed geodesic of X, is a dense subset of Tx. From these last two observations, we obtain the desired result.
LEMMA 3.4. Let h be a symmetric 2-form and u be a 1-form on a flat torus X of dimension ![]() 2 which satisfy the zero-energy condition.
2 which satisfy the zero-energy condition.
(i) If the symmetric 2-form h satisfies the relation Dgh = 0, then it is a Lie derivative of the metric.
(ii) If the 1-form u satisfies the relation du = 0, then it is exact.
PROOF: We first suppose that the relation Dgh = 0 holds. As we saw above, we have the decomposition (3.4), where is![]() a vector field on X and h0 is a parallel symmetric 2-form on X. According to Lemma 2.6, the form h0 also satisfies the zero-energy
condition. From Lemma 3.3 with p = 2, we infer that h0 vanishes, and so the equality
a vector field on X and h0 is a parallel symmetric 2-form on X. According to Lemma 2.6, the form h0 also satisfies the zero-energy
condition. From Lemma 3.3 with p = 2, we infer that h0 vanishes, and so the equality ![]() holds. If the 1-form is closed, then we may write
holds. If the 1-form is closed, then we may write
![]()
where f is a real-valued function on X and![]() is a harmonic 1-form on X. Clearly, also satisfies the zero-energy condition. We saw above that is parallel; hence by Lemma
3.3 with p = 1, we see that
is a harmonic 1-form on X. Clearly, also satisfies the zero-energy condition. We saw above that is parallel; hence by Lemma
3.3 with p = 1, we see that![]() is equal to df.
is equal to df.
on ![]() is tangent to the line segment u
is tangent to the line segment u![]() . If is a symmetric p-form on X and is the
. If is a symmetric p-form on X and is the![]() -invariant symmetric p-form determined by
-invariant symmetric p-form determined by ![]() , then the integral of
, then the integral of![]() over the closed geodesic
over the closed geodesic ![]() of X is given by
of X is given by
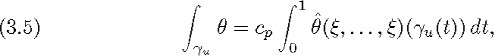
where the constant ![]() depends only on the integers p, p1 and p2 and the basis {1, 2} of R
depends only on the integers p, p1 and p2 and the basis {1, 2} of R
2.
![]() The following proposition is due to Michel [46].
The following proposition is due to Michel [46].
PROPOSITION 3.5. The X-ray transform for functions on a flat torus of dimension n 2 is injective.
PROOF: We first suppose that n = 2. Let f be a complex-valued function on X satisfying the zero-energy condition; we also
denote by f the -invariant function on ![]() which it determines. We consider the Fourier series
which it determines. We consider the Fourier series
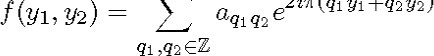
of the function f on ![]() where the
where the![]() are its Fourier coefficients. We now fix a pair of integers (p1, p2); we suppose that
are its Fourier coefficients. We now fix a pair of integers (p1, p2); we suppose that ![]() and we consider the closed geodesic
and we consider the closed geodesic ![]() of X, which we associated above with the integers p1 and p2 and with the real number
of X, which we associated above with the integers p1 and p2 and with the real number ![]() . We then consider the function on
. We then consider the function on ![]() whose value at
whose value at ![]() is equal to the integral of
is equal to the integral of ![]() over the closed geodesic
over the closed geodesic![]() , where c0 is the constant appearing in the equality (3.5), with p = 0; according to our hypothesis, the function
, where c0 is the constant appearing in the equality (3.5), with p = 0; according to our hypothesis, the function![]() vanishes identically. Therefore the sum
vanishes identically. Therefore the sum
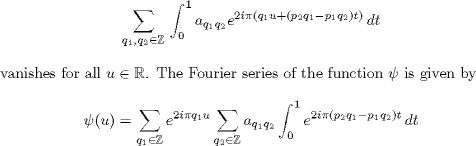
for ![]() Since the Fourier coefficient of
Since the Fourier coefficient of![]() corresponding to the integer p1 vanishes, we see that
corresponding to the integer p1 vanishes, we see that ![]() = 0. A similar argument shows that the coefficient
= 0. A similar argument shows that the coefficient ![]() vanishes when p1 = 0 and
vanishes when p1 = 0 and ![]() Thus the function f is constant and therefore vanishes. Since an arbitrary point of a flat torus of dimension
Thus the function f is constant and therefore vanishes. Since an arbitrary point of a flat torus of dimension ![]() 2 is contained in a totally geodesic flat torus of dimension 2, we obtain the desired result in all cases.
2 is contained in a totally geodesic flat torus of dimension 2, we obtain the desired result in all cases.
PROPOSITION 3.6. Suppose that X is a flat torus of dimension 2. Let h be a symmetric 2-form and![]() be a 1-form on X; suppose that these two forms satisfy the zero-energy condition. Then we have the relations Dgh = 0 and
be a 1-form on X; suppose that these two forms satisfy the zero-energy condition. Then we have the relations Dgh = 0 and
![]() = 0.
= 0.
PROOF: We consider the -invariant symmetric forms
![]()
on ![]() determined by h and
determined by h and ![]() , where a, b, c, a1, a2 are
, where a, b, c, a1, a2 are ![]() -invariant functions on
-invariant functions on ![]() We now fix a pair of integers (p1, p2); we suppose that
We now fix a pair of integers (p1, p2); we suppose that ![]() and we consider the closed geodesic
and we consider the closed geodesic ![]() of X which we associated above with the integers p1 and p2 and with the real number u. We consider the functions
of X which we associated above with the integers p1 and p2 and with the real number u. We consider the functions ![]() and
and ![]() on
on ![]() whose values at
whose values at ![]() are equal to the integrals of
are equal to the integrals of ![]() / and h/c2 over the closed geodesi
/ and h/c2 over the closed geodesi![]() respectively; here cp is the constant appearing in the equality (3.5). According to our hypotheses, the functions
respectively; here cp is the constant appearing in the equality (3.5). According to our hypotheses, the functions ![]() and
and ![]() vanish identically. The Fourier series of the function
vanish identically. The Fourier series of the function ![]() is given by
is given by

respectively. Since ![]() is a frame for the tangent bundle of
is a frame for the tangent bundle of ![]() we see that Dgh = 0 and
we see that Dgh = 0 and ![]()
THEOREM 3.7. A flat torus of dimension 2 is infinitesimally rigid.
THEOREM 3.8. A differential form of degree 1 on a flat torus of dimension ![]() 2 satisfies the zero-energy condition if and only if it is exact.
2 satisfies the zero-energy condition if and only if it is exact.
We now simultaneously prove Theorems 3.7 and 3.8. Let h be a symmetric 2-form and ![]() be a 1-form on the flat torus X, both of which satisfy the zero-energy condition. Let x be an arbitrary point of X and
be a 1-form on the flat torus X, both of which satisfy the zero-energy condition. Let x be an arbitrary point of X and ![]() be an orthonormal set of vectors of Tx. If F is the subspace
be an orthonormal set of vectors of Tx. If F is the subspace ![]() of Tx, then Y = ExpxF is a closed totally geodesic submanifold of X isometric to a flat 2-torus. Let
of Tx, then Y = ExpxF is a closed totally geodesic submanifold of X isometric to a flat 2-torus. Let ![]() be the natural imbedding; the forms
be the natural imbedding; the forms ![]() and
and ![]() Y satisfy the zero-energy condition. If gY is the metric on Y induced by g, Proposition 3.6 tells us that DgY
Y satisfy the zero-energy condition. If gY is the metric on Y induced by g, Proposition 3.6 tells us that DgY ![]() and
and ![]() According to Proposition 1.14,(i), the restriction
According to Proposition 1.14,(i), the restriction ![]() of the section Dgh of B to Y vanishes. Hence we have
of the section Dgh of B to Y vanishes. Hence we have
![]()
Thus these equalities holds for all ![]() and we see that Dgh = 0 and
and we see that Dgh = 0 and ![]() . According to Lemma 3.4, the symmetric form h on X is Lie derivative of the metric and the 1-form
. According to Lemma 3.4, the symmetric form h on X is Lie derivative of the metric and the 1-form![]() is exact.
is exact.
THEOREMS 3.7 and 3.8 are due to Michel [46]; our proofs of these theorems are essentially the same as those given by Estezet [12]. The next theorem, which generalizes both of these theorems, was proved by Michel [46] when the integer p is equal to 0, 1 or an odd integer and by Estezet [12] in all the other cases.

PROOF: We consider the vector field![]() on Y , whose value at
on Y , whose value at ![]() is equal to the orthogonal projection o
is equal to the orthogonal projection o![]() f (x) onto the subspace
f (x) onto the subspace ![]() of Tx.
of Tx.
![]()
According to Lemma 1.1, we have ![]() and we know that YYis a Killing vector field on Y . Therefore by Proposition 3.1, we see that
and we know that YYis a Killing vector field on Y . Therefore by Proposition 3.1, we see that ![]() and so the 1-form
and so the 1-form ![]() on Y is harmonic. Thus we have
on Y is harmonic. Thus we have ![]() If
If ![]() satisfies the zero-energy condition, then so does the 1-form
satisfies the zero-energy condition, then so does the 1-form ![]() on Y ; by Theorem 3.8, the 1-form
on Y ; by Theorem 3.8, the 1-form ![]() on the flat torus Y is exact and therefore vanishes.
on the flat torus Y is exact and therefore vanishes.
![]() 2. The projective spaces
2. The projective spaces
THEOREM 3.11. A symmetric space of rank one, which is not isometric to a sphere, is infinitesimally rigid.
THEOREM 3.12. Let X be a symmetric space of rank one, which is not isometric to a sphere. A differential form of degree 1 on X satisfies the zero-energy condition if and only if it is exact.
Most of the results described in the remainder of this section are to be found in Chapter 3 of [5]. Let n be an intege![]() r 1. Let
r 1. Let ![]() be one of the fields
be one of the fields ![]() We set
We set ![]() We endow
We endow ![]() with its right vector space structure over
with its right vector space structure over ![]() with the Hermitian scalar product
with the Hermitian scalar product


We endow the sphere ![]() with the Riemannian metric
with the Riemannian metric ![]() induced by the scalar product (3.7), and the projective space
induced by the scalar product (3.7), and the projective space ![]() with the Rie-mannian metric g determined by
with the Rie-mannian metric g determined by

(i) We have u, v= 0.
(ii) The vector ![]() is orthogonal to the subspace
is orthogonal to the subspace ![]() with respect to the metric g.
with respect to the metric g.
(iii) The subspaces ![]() and
and ![]() are orthogonal with respect to the metric g.
are orthogonal with respect to the metric g.
If ![]() are non-zero vectors of
are non-zero vectors of ![]() such that the subspaces
such that the subspaces ![]() are mutually orthogonal, then we have the decomposition
are mutually orthogonal, then we have the decomposition

When ![]() in the remainder of this section we suppose that n 2. If In denotes the unit matrix of order n, the element
in the remainder of this section we suppose that n 2. If In denotes the unit matrix of order n, the element
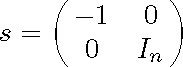
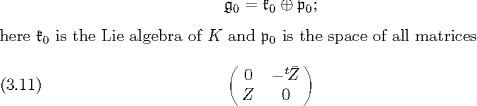
of ![]() where
where ![]() is viewed as a column vector and t
is viewed as a column vector and t![]() is its conjugate transpose. We identify p0 with the vector space
is its conjugate transpose. We identify p0 with the vector space ![]() and, in particular, the element (3.11) of p0 with the vector Z of
and, in particular, the element (3.11) of p0 with the vector Z of ![]() The adjoint action of K on
The adjoint action of K on ![]() p0 is expressed by
p0 is expressed by
![]()
where ![]() is the element (3.10) of K and
is the element (3.10) of K and ![]() When
When ![]() we know that
we know that ![]() is also the Lie algebra of
is also the Lie algebra of ![]()
The following result is proved in![]() D of [5, Chapter 3].
D of [5, Chapter 3].

The following result is a direct consequence of Proposition 3.14.

In ![]() G of [5, Chapter 3], the structure of symmetric space of rank one is defined on the Cayley plane X. An analogue of Proposition
3.14 holds for the Cayley plane. In fact, the inclusion of the quaternions
G of [5, Chapter 3], the structure of symmetric space of rank one is defined on the Cayley plane X. An analogue of Proposition
3.14 holds for the Cayley plane. In fact, the inclusion of the quaternions ![]() H into the Cayley algebra gives rise to closed totally geodesic submanifolds of X isometric to
H into the Cayley algebra gives rise to closed totally geodesic submanifolds of X isometric to ![]() HP2.
HP2.
3. The real projective space
Let ![]() be the complexification of the Lie algebra of G = SO(n+1) and let be the dual of the group G. Let
be the complexification of the Lie algebra of G = SO(n+1) and let be the dual of the group G. Let ![]() and
and ![]() be the elements of which are the equivalence classes of the trivial G-module
be the elements of which are the equivalence classes of the trivial G-module ![]() and of the irreducible G-module
and of the irreducible G-module ![]() respectively.
respectively.
We view the sphere X = Sn = ![]() as the irreducible symmetric space SO(n + 1)/SO(n). The set of eigenvalues of the Laplacia
as the irreducible symmetric space SO(n + 1)/SO(n). The set of eigenvalues of the Laplacia![]() n acting on
n acting on ![]() consists of all the integers
consists of all the integers ![]() where k is an intege
where k is an intege![]() r 0. The eigenspace
r 0. The eigenspace ![]() of
of![]() associated with the eigenvalue
associated with the eigenvalue ![]() consists of all the complex-valued functions on X which are restrictions to Sn of harmonic polynomials of degree k on
consists of all the complex-valued functions on X which are restrictions to Sn of harmonic polynomials of degree k on ![]() According to observations made in
According to observations made in ![]() 7, Chapter II and by Proposition 2.1, we know that Hk is an irreducible SO(n + 1)-submodule of
7, Chapter II and by Proposition 2.1, we know that Hk is an irreducible SO(n + 1)-submodule of ![]() and that the direct sum
and that the direct sum 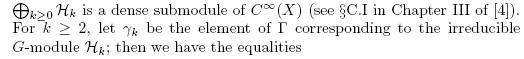
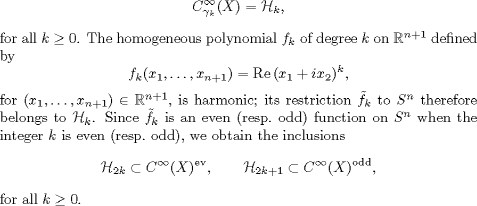
Let Y be the real projective space ![]() endowed with the metric
endowed with the metric ![]() which we view as an irreducible symmetric space. According to 7, Chapter II and the isomorphism (2.19), we see that the set
of eigenvalues of the Laplacian
which we view as an irreducible symmetric space. According to 7, Chapter II and the isomorphism (2.19), we see that the set
of eigenvalues of the Laplacian ![]() acting on
acting on ![]() consists of all the integers 2k, with k
consists of all the integers 2k, with k ![]() 0; moreover if k is an even integer, the G-module
0; moreover if k is an even integer, the G-module ![]() is equal to the space of functions on Y obtained from Hk by passage to the quotient. Therefore
is equal to the space of functions on Y obtained from Hk by passage to the quotient. Therefore ![]() is a dense subspace of
is a dense subspace of ![]() and the first non-zero eigenvalue of the Laplacian
and the first non-zero eigenvalue of the Laplacian ![]() acting on
acting on ![]() is equal to 2(n + 1).
is equal to 2(n + 1).
We consider the closed geodesi![]() c of Sn, which is the great circle defined by
c of Sn, which is the great circle defined by
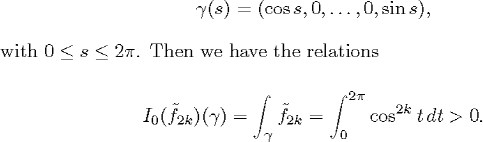
The following result is a consequence of the above observations and Proposition 2.29, with ![]()
PROPOSITION 3.17. An even function on the sphere Sn, with n![]() 2, whose X-ray transform vanishes, vanishes identically.
2, whose X-ray transform vanishes, vanishes identically.
In![]() Chapter II, we noted that the preceding proposition is equivalent to assertion (i) of Theorem 2.23 and also that it implies
that the X-ray transform for functions is injective on the real projective space
Chapter II, we noted that the preceding proposition is equivalent to assertion (i) of Theorem 2.23 and also that it implies
that the X-ray transform for functions is injective on the real projective space ![]() with
with ![]()
Theorem 3.18. The X-ray transform for functions on a symmetric space of compact type of rank one, which is not isometric to a sphere, is injective.
PROOF: According to Proposition 3.14 and the discussion which follows this proposition, each point of such a projective space
X is contained in a closed totally geodesic submanifold of X isometric to the projective plane ![]() The desired result is then a consequence of Theorem 2.23,(ii).
The desired result is then a consequence of Theorem 2.23,(ii).
THEOREM 3.18, together with Proposition 2.26, implies that the X-ray transform for functions on a symmetric space X of compact type is injective if and only if X is not isometric to a sphere. Theorem 3.18 is also a consequence of Theorem 2.24.
We shall now establish the infinitesimal rigidity of the real projective space ![]() a result due to Michel [45]. We first consider the case of the real projective plane.
a result due to Michel [45]. We first consider the case of the real projective plane.
PROPOSITION 3.19. The real projective plane ![]() is infinitesimally rigid.
is infinitesimally rigid.
PROOF: Let h be a symmetric 2-form on X satisfying the zero-energy condition. We know that the relation (1.64) holds on the
sphere ![]() which is a covering space of X; therefore we may write
which is a covering space of X; therefore we may write
![]()
where ![]() is a vector field and f is a realvalued function on X. I
is a vector field and f is a realvalued function on X. I![]() is closed geodesic of X
is closed geodesic of X
Since the Lie derivative ![]() satisfies the zero-energy condition, we see that the function f also satisfies the zero-energy condition. From Theorem 2.23,(ii),
with n = 2, we deduce that the function f vanishes and so we have h
satisfies the zero-energy condition, we see that the function f also satisfies the zero-energy condition. From Theorem 2.23,(ii),
with n = 2, we deduce that the function f vanishes and so we have h ![]()
THEOREM 3.20. The real projective space X ![]() with n
with n![]() 2, is infinitesimally rigid.
2, is infinitesimally rigid.
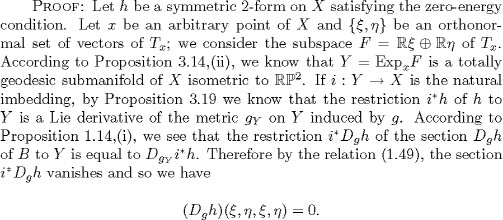
Thus this last equality holds for all ![]() and we see that Dgh = 0. According to Theorem 1.18, the sequence (1.51) is exact, and so h is a Lie derivative of the metric.
and we see that Dgh = 0. According to Theorem 1.18, the sequence (1.51) is exact, and so h is a Lie derivative of the metric.
The proof of Proposition 3.19 given above is due to Bourguignon and our proof of Theorem 3.20 is inspired by the one given
in Chapter 5 of [5]. We now present a variant of the version given in [30, 2![]() ] of Michel’s original proof of Proposition 3.19 (see [45]).
] of Michel’s original proof of Proposition 3.19 (see [45]).
We suppose that (X, g) is the real projective plane ![]() Let
Let ![]() be an arbitrary closed geodesic parametrized by its arc-length. We set
be an arbitrary closed geodesic parametrized by its arc-length. We set![]() and let
and let ![]() be the tangent vector to the geodesic at
be the tangent vector to the geodesic at ![]() , for 0
, for 0 ![]() t . We choose a unit vector
t . We choose a unit vector ![]() orthogonal to e1(0) and consider the family of tangent vectors
orthogonal to e1(0) and consider the family of tangent vectors ![]() with
with ![]() obtained by parallel transport of the vector e2 along
obtained by parallel transport of the vector e2 along ![]() . Clearly, if u is an element of
. Clearly, if u is an element of ![]() , we have
, we have

for ![]() the first equality holds because div h = 0, while the second one is obtained using the expression for the curvature of (X,
g). The lemma is now a consequence of (3.12).
the first equality holds because div h = 0, while the second one is obtained using the expression for the curvature of (X,
g). The lemma is now a consequence of (3.12).
LEMMA 3.23. Let X be the real projective plane ![]() If h
If h![]() satisfies div h = 0, then
satisfies div h = 0, then
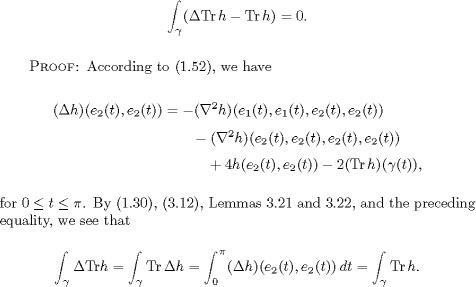
LEMMA 3.24. Let X be the real projective plane ![]() If h
If h![]() satisfies div h = 0, then Tr h vanishes.
satisfies div h = 0, then Tr h vanishes.
PROOF: According to Theorem 2.23,(ii), with n = 2, and Lemma 3.23, we see that ![]() As the first non-zero eigenvalue of the Laplacian acting on
As the first non-zero eigenvalue of the Laplacian acting on ![]() is equal to 6, we see that Tr h = 0.
is equal to 6, we see that Tr h = 0.
LEMMA 3.25. Let X be the real projective plane ![]() An element h of
An element h of ![]() satisfying div h = 0 vanishes.
satisfying div h = 0 vanishes.
PROOF: Let h be an element of ![]() satisfying div h = 0. According to Lemma 3.24 and the equality (1.53), the symmetric 2-form h belongs to H(X). Then Proposition 1.20 tells us that h vanishes.
satisfying div h = 0. According to Lemma 3.24 and the equality (1.53), the symmetric 2-form h belongs to H(X). Then Proposition 1.20 tells us that h vanishes.
Now Proposition 3.19 is a direct consequence of Proposition 2.13 and Lemma 3.25.
Our approach to the rigidity questions, which led us to the criteria of Theorem 2.49 and the methods introduced in [22] for the study of the complex quadrics, were partially inspired by the proof of the infinitesimal rigidity of the real projective plane which we have just completed. The correspondence between the arguments given here in the case of the real projective plane and those used in the case of the complex quadric is pointed out in [30].
The following result is due to Michel [47].
THEOREM 3.26. A differential form of degree 1 on the real projective space ![]() , with n
, with n![]() 2, satisfies the zero-energy condition if and only if it is exact.
2, satisfies the zero-energy condition if and only if it is exact.
In the case of the real projective plane ![]() according to Proposition 2.20, we see that Theorem 3.26 is a consequence of Proposition 3.29,(ii), which is proved below;
on the other hand, the proof of this theorem given in [47] for
according to Proposition 2.20, we see that Theorem 3.26 is a consequence of Proposition 3.29,(ii), which is proved below;
on the other hand, the proof of this theorem given in [47] for ![]() is elementary and requires only Stokes’s theorem for functions in the plane. In fact, the result given by Theorem 3.26 for
the real projective plane implies the result in the general case. Let X be the real projective space
is elementary and requires only Stokes’s theorem for functions in the plane. In fact, the result given by Theorem 3.26 for
the real projective plane implies the result in the general case. Let X be the real projective space ![]() , with n 2; by Proposition 3.14, we easily see that an element of
, with n 2; by Proposition 3.14, we easily see that an element of ![]() which vanishes when restricted to the totally geodesic surfaces of X isometric to the real projective plane, must be equal
to 0. Then the desired result for X is a consequence of Proposition 2.51,(ii).
which vanishes when restricted to the totally geodesic surfaces of X isometric to the real projective plane, must be equal
to 0. Then the desired result for X is a consequence of Proposition 2.51,(ii).
The following result due to Bailey and Eastwood [1] generalizes both Theorems 3.20 and 3.26.
In the case p = 2, the assertion of Theorem 3.27 was first established by Estezet (see [12] and [29]).
![]() 4. The complex projective space
4. The complex projective space
In this section, we suppose that X is the complex projective space ![]() with n 1, endowed with the metric g of
with n 1, endowed with the metric g of![]() 2. We have seen that g is the Fubini-Study metric of constant holomorphic curvature 4. We denote by J the complex structure
of X. As in
2. We have seen that g is the Fubini-Study metric of constant holomorphic curvature 4. We denote by J the complex structure
of X. As in ![]() 2, we identify X with the Hermi-tian symmetric space G/K, where G is the group SU(n + 1) and K is its subgroup S(U(n) × U(1)).
2, we identify X with the Hermi-tian symmetric space G/K, where G is the group SU(n + 1) and K is its subgroup S(U(n) × U(1)).
× The c![]() urvature tensor Rof X is given by
urvature tensor Rof X is given by
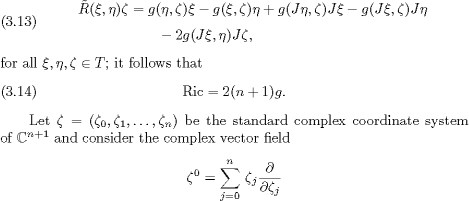


![]()
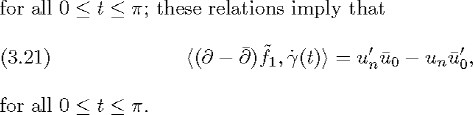
Let ![]() be the complexification of the Lie algebra of K. The group of all diagonal matrices of G is a maximal torus of G and of K.
The complexification
be the complexification of the Lie algebra of K. The group of all diagonal matrices of G is a maximal torus of G and of K.
The complexification ![]() t of the Lie algebra
t of the Lie algebra ![]() of this torus is a Cartan subalgebra of the semi-simple Lie algebra g and also of the reductive Lie algebra
of this torus is a Cartan subalgebra of the semi-simple Lie algebra g and also of the reductive Lie algebra ![]() For 0
For 0 ![]() the linear form
the linear form ![]() sending the diagonal matrix with a0, a1, . . . , an
sending the diagonal matrix with a0, a1, . . . , an ![]() as its diagonal entries into aj , is purely imaginary on
as its diagonal entries into aj , is purely imaginary on ![]() Then
Then

Then we see that highest weight of the irreducible G-module Hk is equal to ![]() and that
and that ![]() is a highest weight vector of this module. Clearly, we have
is a highest weight vector of this module. Clearly, we have
![]()
The fibers of the homogeneous vector bundles ![]()
![]() and T0,1 at the point
and T0,1 at the point![]() of X considered in
of X considered in ![]() 2 are irreducible K-modules of highest weights equal to
2 are irreducible K-modules of highest weights equal to ![]() and
and ![]() respectively. According to the branching law for G and K (see Proposition 3.1 of [14]), from the results of [14,
respectively. According to the branching law for G and K (see Proposition 3.1 of [14]), from the results of [14,![]() 4] we obtain the following:
4] we obtain the following:
PROPOSITION 3.28. Let X be the complex projective space ![]() with n
with n![]() 1.
1.

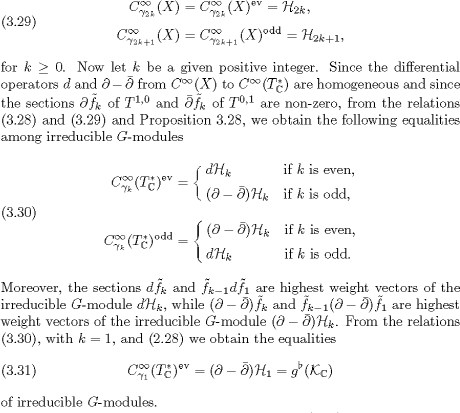
For the remainder of this section, we suppose that n = 1 and that ![]() We consider the sphere S2 =
We consider the sphere S2 = ![]() the mapping
the mapping
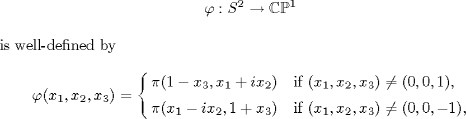
where (x1, x2, x3![]() ) R3, with x21 + x22 + x23
) R3, with x21 + x22 + x23 ![]() = 1. The two expressions for correspond to the stereographic projections whose poles are the points (0, 0, 1) and (0, 0,-1),
respectively, and so we know that is
= 1. The two expressions for correspond to the stereographic projections whose poles are the points (0, 0, 1) and (0, 0,-1),
respectively, and so we know that is ![]() a diffeomor-phism. We also consider the involuti
a diffeomor-phism. We also consider the involuti![]() on of
on of ![]() which sends the point
which sends the point ![]() of CP1, where u is a non-zero vector of
of CP1, where u is a non-zero vector of ![]() into the point
into the point![]() where v is a non-zero vector of
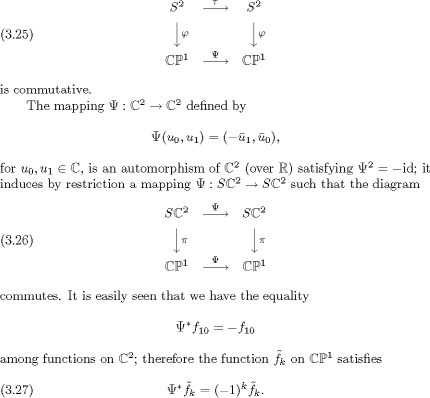
where v is a non-zero vector of ![]() orthogonal to u. I
orthogonal to u. I![]() is the anti-podal involution of the sphere S2, it is easily verified that the diagram
is the anti-podal involution of the sphere S2, it is easily verified that the diagram
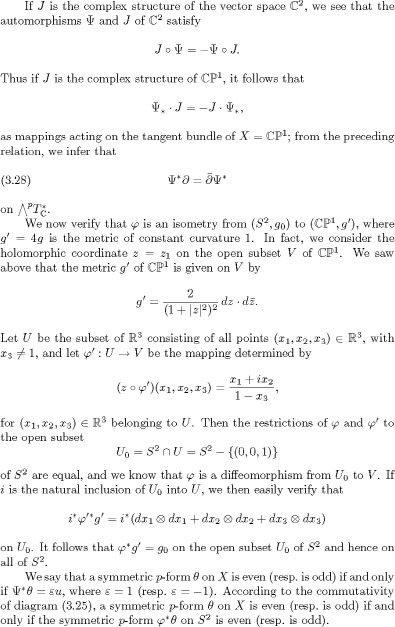
Since Hk is an irreducible G-module which contains the function f
![]() by (3.27) we see that
by (3.27) we see that
According to the commutativity of diagram (3.25), the first assertion of the next proposition is equivalent to the result concerning the sphere S2 given by Proposition 3.17; moreover according to Proposition 2.20, the second assertion of the next proposition implies the result concerning the real projective plane stated in Theorem 3.26.
PROPOSITION 3.29. Let X be the complex projective space ![]() .
.
(i) An even function on X, whose X-ray transform vanishes, vanishes identically.
(ii) An even differential form of degree 1 on X satisfies the zero-energy condition if and only if it is exact.
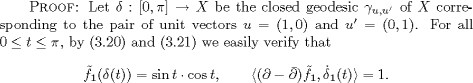

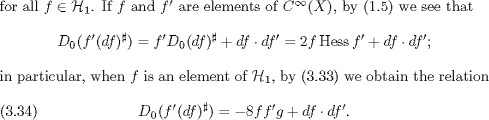
5. The rigidity of the complex projective space

We now introduce various families of closed connected totally geodesic submanifolds of X. Let x be a point of X, and let ![]() be the family of all closed connected totally geodesic surfaces of X passing through x of the form ExpxF, where F is the
subspace of the tangent space Tx generated by an orthonormal set of vectors
be the family of all closed connected totally geodesic surfaces of X passing through x of the form ExpxF, where F is the
subspace of the tangent space Tx generated by an orthonormal set of vectors ![]() of Tx satisfying
of Tx satisfying ![]() Let F2,x be the family of all closed connected totally geodesic surfaces of X passing through x of the form ExpxF, where
F is the subspace
Let F2,x be the family of all closed connected totally geodesic surfaces of X passing through x of the form ExpxF, where
F is the subspace ![]() of the tangent space Tx determined by a unitary vectror
of the tangent space Tx determined by a unitary vectror ![]() of Tx. We consider the G-invariant families
of Tx. We consider the G-invariant families
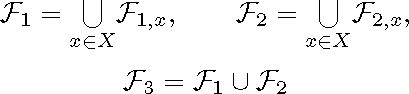
of closed connected totally geodesic surfaces of X.
According to Proposition 3.14, a surface belonging to the family F1 is isometric to the real projective plane with its metric of constant curvature 1, while a surface belonging to the family F2 is isometric to the complex projective line with its metric of constant curvature 4.
For j = 1, 2, 3, we consider the sub-bundle ![]() of B consisting of those elements of B which vanish when restricted to the submanifolds of Fj . An element u of B belongs
to N1 if and only if the relation
of B consisting of those elements of B which vanish when restricted to the submanifolds of Fj . An element u of B belongs
to N1 if and only if the relation


LEMMA 3.34. Let h be an element of ![]() belonging to
belonging to ![]() Then we have D1h = 0.
Then we have D1h = 0.
PROOF: By Lemma 3.33, we know that h also belongs to ![]() Hence by Proposition 2.45, with F = F3, we see that
Hence by Proposition 2.45, with F = F3, we see that ![]() By Proposition 3.31, we therefore know that D1h = 0.
By Proposition 3.31, we therefore know that D1h = 0.
The equivalence of assertions (i) and (iii) of the following theorem is originally due to Michel [45]. We now provide an alternate proof of Michel’s result following [13, 8].
THEOREM 3.35. Let h be a symmetric 2-form on ![]() with n
with n![]() 2. The following assertions are equivalent:
2. The following assertions are equivalent:
(i) The symmetric 2-form h belongs to ![]()
(ii) We have D1h = 0.
(iii) The symmetric 2-form h is a Lie derivative of the metric g.

LEMMA 3.37. Let X be the complex projective space ![]() with n
with n![]() 2. The following assertions are equivalent:
2. The following assertions are equivalent:
(i) The space X is infinitesimally rigid.
(ii) (3.37)
(ii) The complex (3.37) is exact.
LEMMA 3.38. A real-valued function f on X satisfies ![]() if and only if it vanishes identically.
if and only if it vanishes identically.
PROOF: Let f be a real-valued function f on X satisfying ![]() According to Lemma 3.36, the symmetric 2-form fg satisfies the zero-energy condition, and so the X-ray transform
According to Lemma 3.36, the symmetric 2-form fg satisfies the zero-energy condition, and so the X-ray transform ![]() of f vanishes. From Theorem 3.18, we obtain the vanishing of f.
of f vanishes. From Theorem 3.18, we obtain the vanishing of f.

Since 2n is not an eigenvalue of ![]() , it follows that f vanishes identically. In fact, according to Lichnerowicz’s theorem (see [43, p. 135] or Theorem D.I.1
in Chapter III of [4]) and (3.14), we see that the first non-zero eigenvalue of
, it follows that f vanishes identically. In fact, according to Lichnerowicz’s theorem (see [43, p. 135] or Theorem D.I.1
in Chapter III of [4]) and (3.14), we see that the first non-zero eigenvalue of![]() is > 2(n + 1); we have also seen that the first non-zero eigenvalue of is equal to 4(n + 1).
is > 2(n + 1); we have also seen that the first non-zero eigenvalue of is equal to 4(n + 1).
The following theorem gives us the infinitesimal rigidity of the complex projective spaces of dimensio![]() n 2.
n 2.
THEOREM 3.39. The complex projective space ![]() with n
with n![]() 2, is infinitesimally rigid.
2, is infinitesimally rigid.
The infinitesimal rigidity of the complex projective spaces of dimension 2 was first proved by Tsukamoto [53]; in fact, he
first proved directly the infinitesimal rigidity of ![]() and then used the above-mentioned result of Michel given by Theorem 3.35 to derive the rigidity of the complex projective
spaces of dimension > 2. Other proofs of Theorem 3.39 may be found in [14] and [18].
and then used the above-mentioned result of Michel given by Theorem 3.35 to derive the rigidity of the complex projective
spaces of dimension > 2. Other proofs of Theorem 3.39 may be found in [14] and [18].
We can also obtain the infinitesimal rigidity of the complex projective spaces of dimension![]() 2 from the rigidity of the complex projective plane by means of Theorem 2.47. In fact, we apply this theorem to the family
F equal to F3 and to the family
2 from the rigidity of the complex projective plane by means of Theorem 2.47. In fact, we apply this theorem to the family
F equal to F3 and to the family ![]() consisting of all closed totally geodesic submanifolds of X isometric to
consisting of all closed totally geodesic submanifolds of X isometric to ![]() according to Propositions 3.14, 3.31 and 3.32, we know that the hypotheses of Theorem 2.47 hold.
according to Propositions 3.14, 3.31 and 3.32, we know that the hypotheses of Theorem 2.47 hold.
We remark that the equivalence of assertions (i) and (iii) of Theorem 3.35 may be obtained from Theorem 3.39 and Lemma 3.33 without requiring Propositions 3.31 and 3.32.
We now present an outline of the proof of Theorem 3.39 given in [14]. Since the differential operator ![]() is homogeneous and the differential operator D0 is elliptic, according to Lemma 3.37 and Proposition 2.3 we see that the
space X is infinitesimally rigid if and only if the complex
is homogeneous and the differential operator D0 is elliptic, according to Lemma 3.37 and Proposition 2.3 we see that the
space X is infinitesimally rigid if and only if the complex
![]()
is exact for![]() all .
all .
We choose a Cartan subalgebra of ![]() and fix a system of positive roots of
and fix a system of positive roots of ![]() . If
. If![]() is an arbitrary element of , we determine the multiplicities of the G-modules
is an arbitrary element of , we determine the multiplicities of the G-modules ![]() and
and ![]() and then describe an explicit basis for the weight subspace
and then describe an explicit basis for the weight subspace ![]() Wof
Wof ![]() corresponding to its highest weight in terms of elements of the eigenspaces Hk; either the multiplicity of C(S2TC) is equal
to 4 and the multiplicity of
corresponding to its highest weight in terms of elements of the eigenspaces Hk; either the multiplicity of C(S2TC) is equal
to 4 and the multiplicity of ![]() is equal to 2, or these two multiplicities ar
is equal to 2, or these two multiplicities ar![]() e 2. Since X is an irreducible Hermitian symmetric space, according to (2.28) the multiplicity of the G-module
e 2. Since X is an irreducible Hermitian symmetric space, according to (2.28) the multiplicity of the G-module ![]() is equal to 2. The multiplicity of
is equal to 2. The multiplicity of ![]() is also equal to 2; in fact, we show that
is also equal to 2; in fact, we show that

Before proceeding to the description of another proof of Theorem 3.39 given in [18], we shall prove the following result which first appeared in [17].
THEOREM 3.40. A differential form of degree 1 on ![]() with n
with n![]() 2, satisfies the zero-energy condition if and only if it is exact.
2, satisfies the zero-energy condition if and only if it is exact.
PROOF: Let F be the sub-bundle of ![]() consisting of those elements of
consisting of those elements of ![]() which vanish when restricted to the submanifolds of F1. An element of
which vanish when restricted to the submanifolds of F1. An element of ![]() belongs to F if and only if the relation
belongs to F if and only if the relation
![]()
holds for all vectors ![]() satisfying
satisfying ![]() An elementary algebraic computation shows that F is the line bundle generated by the
An elementary algebraic computation shows that F is the line bundle generated by the ![]() form
form![]() of X. Let
of X. Let![]() be a differential form of degree 1 on X. Since any closed geodesic of X is contained in a submanifold belonging to the family
F1, we see that the 1-form
be a differential form of degree 1 on X. Since any closed geodesic of X is contained in a submanifold belonging to the family
F1, we see that the 1-form ![]() satisfies the zero-energy condition if and only if the restrictions
satisfies the zero-energy condition if and only if the restrictions ![]() of to the submanifolds belonging to the family F1 satisfy the zero-energy condition. According to Theorem 3.26, the latter
property of holds if and only if
of to the submanifolds belonging to the family F1 satisfy the zero-energy condition. According to Theorem 3.26, the latter
property of holds if and only if ![]() is a section of F. We now suppose that the 1-form satisfies the zero-energy condition; our previous observations imply that
satisfies the relation
is a section of F. We now suppose that the 1-form satisfies the zero-energy condition; our previous observations imply that
satisfies the relation
![]()
where f is a real-valued function on X. From this equality, we infer that
![]()
and so f is constant. Since the![]() is harmonic, the function f vanishes and so the form
is harmonic, the function f vanishes and so the form![]() is closed; hence is exact.
is closed; hence is exact.
The simplicity of the preceding proof, which is based on a remark of Demailly (see [18]), rests on the correct interpretation
of the bundle F. This observation led us to a new proof of the infinitesimal rigidity of ![]() , which can be found in [18] and which requires a minimal amount of harmonic analysis. For symmetric 2-forms, the analogue
of the bundle F of the preceding proof is the bundle N1, and its interpretation is given by Proposition 3.30.
, which can be found in [18] and which requires a minimal amount of harmonic analysis. For symmetric 2-forms, the analogue
of the bundle F of the preceding proof is the bundle N1, and its interpretation is given by Proposition 3.30.
We now describe the proof of Theorem 3.39 given in [18]. We consider the first-order differential operator ![]() introduced in
introduced in ![]() 3, Chapter I. Clearly, if u is a section of B satisfying
3, Chapter I. Clearly, if u is a section of B satisfying ![]() = 0, from the definition of
= 0, from the definition of ![]() we infer that
we infer that ![]() D1u = 0. The following two results are proved in [18].
D1u = 0. The following two results are proved in [18].
LEMMA 3.41. Let ![]() be a section of the bundle
be a section of the bundle ![]() over an open subset of X satisfying
over an open subset of X satisfying
![]()
Then the 2-form ![]() is closed.
is closed.
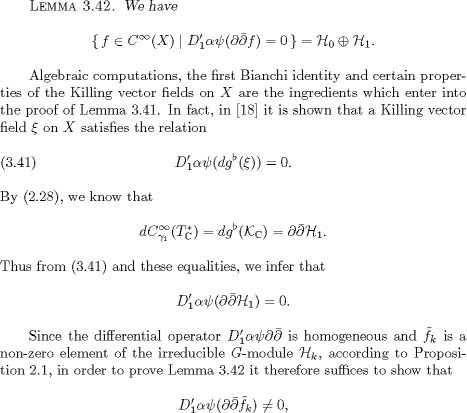
for all integers k > 1. This inequality is verified in the appendix of [18].
Let h be a symmetric 2-form on X satisfying the zero-energy condition.
B From Proposition 3.19, it follows that h belongs to the space ![]() By Proposition 2.44 or Lemma 1.15, we see that D1h is a section of N1/. Thus by Proposition 3.30, there exists a section
By Proposition 2.44 or Lemma 1.15, we see that D1h is a section of N1/. Thus by Proposition 3.30, there exists a section![]() of
of ![]() over X such that
over X such that
![]()
Then Lemma 1.17 tells us that the equality (3.40) holds; hence according to Lemma 3.41, the 2-form ![]() is closed. Therefore there exists a constant
is closed. Therefore there exists a constant ![]() and a real-valued function f on X such that
and a real-valued function f on X such that
![]()
Since![]() is parallel, we know that
is parallel, we know that ![]() Hence the equality (3.40) implies that
Hence the equality (3.40) implies that ![]() By Lemma 3.42, the function f belongs to
By Lemma 3.42, the function f belongs to ![]() . Therefore without loss of generality, we may assume that the function f belongs to H1.
. Therefore without loss of generality, we may assume that the function f belongs to H1.
We consider the subspaces

Thus by Proposition 3.30, we see that Dg(f1g) is a section of N1. Then Lemma 3.38 tells us that the function f1 vanishes identically.
Therefore ![]() D1halso vanishes, and so D1h = 0. By Proposition 3.32 and Theorem 1.18, the complex (1.24) is exact, and so h is a Lie derivative
of the metric.
D1halso vanishes, and so D1h = 0. By Proposition 3.32 and Theorem 1.18, the complex (1.24) is exact, and so h is a Lie derivative
of the metric.
![]() 6. The other projective spaces
6. The other projective spaces
Let X be a projective space equal either to the quaternionic projective space ![]() with n 2, or to the Cayley plane. Let F1 be the family of all closed connected totally geodesic surfaces of X which are isometric
either to the real projective plane with its metric of constant curvature 1 or to the sphere S2 with its metric of constant
curvature 4. Let F2 be the family of all closed connected totally geodesic submanifolds of X isometric to the projective plane
with n 2, or to the Cayley plane. Let F1 be the family of all closed connected totally geodesic surfaces of X which are isometric
either to the real projective plane with its metric of constant curvature 1 or to the sphere S2 with its metric of constant
curvature 4. Let F2 be the family of all closed connected totally geodesic submanifolds of X isometric to the projective plane
![]() We verify that every surface belonging to the family F1 is contained in a submanifold belonging to the family F2 (see Proposition
3.14, [45,
We verify that every surface belonging to the family F1 is contained in a submanifold belonging to the family F2 (see Proposition
3.14, [45,![]() 3.2] and [17, 3]).
3.2] and [17, 3]).
We consider the sub-bundle N = NF1 of B consisting of those elements of B which vanish when restricted to the submanifolds of F1. The following proposition can be proved by means of computations similar to the ones used in [14] to prove Proposition 3.31 and the relation (3.36) for the complex projective spaces.
PROPOSITION 3.43. Let X be equal to the quaternionic projective space ![]() with n
with n![]() 2, or to the Cayley plane. Then we have
2, or to the Cayley plane. Then we have
![]()
We remark that the equalities of the preceding proposition imply that the relation (1.48) holds for the space X. Thus we may
apply Theorem 2.47 to the families F1 and F2 in order to obtain the following result from the infinitesimal rigidity of ![]()
Theorem 3.44. The quaternionic projective space ![]() with n
with n![]() 2, and the Cayley plane are infinitesimally rigid.
2, and the Cayley plane are infinitesimally rigid.
Theorem 3.45. Let X be equal to the quaternionic projective space ![]() with n
with n![]() 2, or to the Cayley plane. A differential form of degree 1 on X satisfies the zero-energy condition if and only if it is
exact.
2, or to the Cayley plane. A differential form of degree 1 on X satisfies the zero-energy condition if and only if it is
exact.
PROOF: According to Propositions 3.14 and 3.15 (see Corollary 3.26 of [5]) when X is equal to a quaternionic projective space,
or by observations made in [17,![]() 3] when X is the Cayley plane, we easily see that
3] when X is the Cayley plane, we easily see that
![]()
By Theorem 3.40, we know that the hypotheses of Theorem 2.51 are satisfied. The latter theorem now gives us the desired result.
Let ![]() be the family of all closed connected totally geodesic submani-folds of X isometric to the projective line
be the family of all closed connected totally geodesic submani-folds of X isometric to the projective line ![]() (which is a sphere of dimension 4), or to the projective line over the Cayley algebra (which is a sphere of dimension 8),
as the case may be. By means of the methods which we used in 5 to prove Theorem 3.35 for the complex projective spaces, we
may also derive the following theorem, which is weaker than Theorem 3.44.
(which is a sphere of dimension 4), or to the projective line over the Cayley algebra (which is a sphere of dimension 8),
as the case may be. By means of the methods which we used in 5 to prove Theorem 3.35 for the complex projective spaces, we
may also derive the following theorem, which is weaker than Theorem 3.44.
THEOREM 3.46. Let X be equal to the quaternionic projective space ![]() with n
with n![]() 2, or to the Cayley plane. If h is a symmetric 2-form on X, the following assertions are equivalent:
2, or to the Cayley plane. If h is a symmetric 2-form on X, the following assertions are equivalent:
(i) The symmetric 2-form h belongs to (![]()
(ii) We have D1h = 0.
(iii) The symmetric 2-form h is a Lie derivative of the metric g.
The equivalence of assertions (i) and (iii) of Theorem 3.46 were first proved by Michel (see [45]). In [53], Tsukamoto deduced
the infinitesimal rigidity of X from this result of Michel, the infinitesimal rigidity of ![]() and the exactness of sequence (1.51) for the sphere of dimension 2; he requires the equality (1.57) of Proposition 1.14,(i)
and uses an argument similar to the one appearing in the proof of Theorem 3.20.
and the exactness of sequence (1.51) for the sphere of dimension 2; he requires the equality (1.57) of Proposition 1.14,(i)
and uses an argument similar to the one appearing in the proof of Theorem 3.20.
