CHAPTER II
RADON TRANSFORMS ON SYMMETRIC SPACES
![]() 1. Outline
1. Outline
In this chapter, we introduce the Radon transforms for functions and symmetric forms on a symmetric space (X, g) of compact
type, namely the X-ray transform and the maximal flat transform. In ![]() we present results concerning harmonic analysis on homogeneous spaces and use them to study these Radon transforms in
we present results concerning harmonic analysis on homogeneous spaces and use them to study these Radon transforms in ![]() and to describe properties of certain spaces of symmetric forms in
and to describe properties of certain spaces of symmetric forms in ![]() The notions of rigidity in the sense of Guillemin and of infinitesimal rigidity of the space X are introduced in
The notions of rigidity in the sense of Guillemin and of infinitesimal rigidity of the space X are introduced in ![]() in this section, we also state the fundamental result of Guillemin [35] concerning isospectral deformations of the metric
g of X (Theorem 2.14). In 4, we present Grinberg’s theorem concerning the injectivity of the maximal flat Radon transform
for functions on X; when the space (X, g) is irreducible, from this result we infer that, if the space X is rigid in the sense
of Guillemin, it is necessarily equal to its adjoint space. In
in this section, we also state the fundamental result of Guillemin [35] concerning isospectral deformations of the metric
g of X (Theorem 2.14). In 4, we present Grinberg’s theorem concerning the injectivity of the maximal flat Radon transform
for functions on X; when the space (X, g) is irreducible, from this result we infer that, if the space X is rigid in the sense
of Guillemin, it is necessarily equal to its adjoint space. In ![]() criteria for the rigidity of the space X are given in terms of harmonic analysis. Some lemmas concerning irreducible G-modules,
where G is a compact semi-simple Lie group, proved in
criteria for the rigidity of the space X are given in terms of harmonic analysis. Some lemmas concerning irreducible G-modules,
where G is a compact semi-simple Lie group, proved in ![]() are used in our study of symmetric forms on an irreducible symmetric space presented in
are used in our study of symmetric forms on an irreducible symmetric space presented in ![]() . Results concerning the space of infinitesimal Einstein deformations of an irreducible symmetric space can be found in
. Results concerning the space of infinitesimal Einstein deformations of an irreducible symmetric space can be found in ![]() Our criteria for the infinitesimal rigidity or the rigidity in the sense of Guillemin of an irreducible symmetric space are
given in
Our criteria for the infinitesimal rigidity or the rigidity in the sense of Guillemin of an irreducible symmetric space are
given in ![]()
![]() Homogeneous vector bundles and harmonic analysis
Homogeneous vector bundles and harmonic analysis
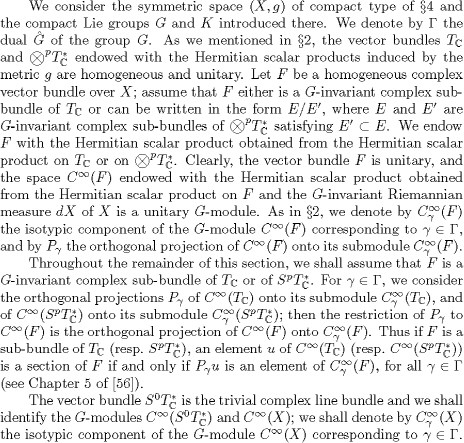
Let (X, g) be a Riemannian manifold which may be written as a homogeneous space G/K, where G is a compact Lie group and K is a closed subgroup of G. We assume that the group G acts by isometries on the Riemannian
manifold X. If F is a homogeneous vector bundle over X, then the space ![]() (F) is a G-module.
(F) is a G-module.
Let F be a complex homogeneous vector bundle over X endowed with a Hermitian scalar product. We endow the space ![]() of sections of F over X with the Hermitian scalar product obtained from the scalar product on F and the Riemannian measure
dX of X. If the vector bundle F is unitary in the sense of [56,
of sections of F over X with the Hermitian scalar product obtained from the scalar product on F and the Riemannian measure
dX of X. If the vector bundle F is unitary in the sense of [56,![]() 2.4], then the space
2.4], then the space ![]() (F) is a unitary G-module. Let x0 be the point of X corresponding to the coset of the identity element of G. The action of
G on the fiber F0 of F at the point x0 of X induces a representation
(F) is a unitary G-module. Let x0 be the point of X corresponding to the coset of the identity element of G. The action of
G on the fiber F0 of F at the point x0 of X induces a representation![]() of K on F0. Then F is isomorphic to the homogeneous vector bundle
of K on F0. Then F is isomorphic to the homogeneous vector bundle ![]() and we shall identify these two homogeneous vector
and we shall identify these two homogeneous vector


Let ![]() and
and ![]() be the Lie algebras of G and K, respectively. In this section, we henceforth suppose that G/K is a reductive homogeneous
space; this means that there is an Ad(K)-invariant complement of
be the Lie algebras of G and K, respectively. In this section, we henceforth suppose that G/K is a reductive homogeneous
space; this means that there is an Ad(K)-invariant complement of ![]() in
in![]() This assumption always holds when the compact group G is connected and semi-simple and (G,K) is a Riemannian symmetric pair
of compact type. Let F1, F2, F3 be complex homogeneous vector bundles over X endowed with Hermitian scalar products. Assume
that these vector bundles are unitary.
This assumption always holds when the compact group G is connected and semi-simple and (G,K) is a Riemannian symmetric pair
of compact type. Let F1, F2, F3 be complex homogeneous vector bundles over X endowed with Hermitian scalar products. Assume
that these vector bundles are unitary.
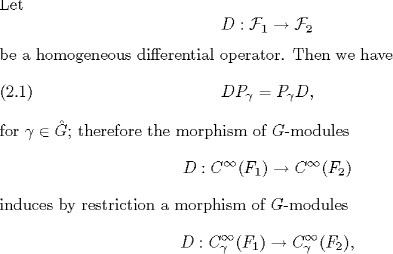
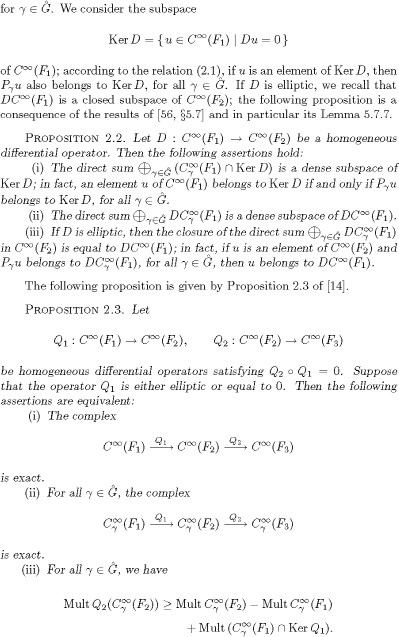
PROOF: First, suppose that assertion (ii) holds. Then according to Proposition 2.2,(i) the subspace Ker![]() Q2 is equal to the closure of
Q2 is equal to the closure of
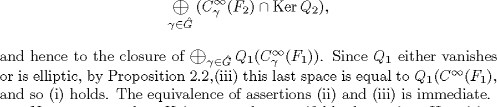
Now suppose that X is a complex manifold, that g is a Hermitian metric and that the group G acts by holomorphic isometries
on X. Then the vector bundles ![]() and
and ![]() are homogeneous sub-bundles of TC, while the vector bundles
are homogeneous sub-bundles of TC, while the vector bundles ![]() and
and![]() are homogeneous sub-bundles of
are homogeneous sub-bundles of ![]() The isomorphisms of vector bundles (1.70) are G-equivariant. Therefore for all
The isomorphisms of vector bundles (1.70) are G-equivariant. Therefore for all ![]() G, the isomorphism of vector bundles
G, the isomorphism of vector bundles ![]() : induces isomor-phisms of G-modules
: induces isomor-phisms of G-modules
![]()
![]() 3. The Guillemin and zero-energy conditions
3. The Guillemin and zero-energy conditions
Let (X, g) be a Riemannian manifold. For p 0, we consider the symmetrized covariant derivative

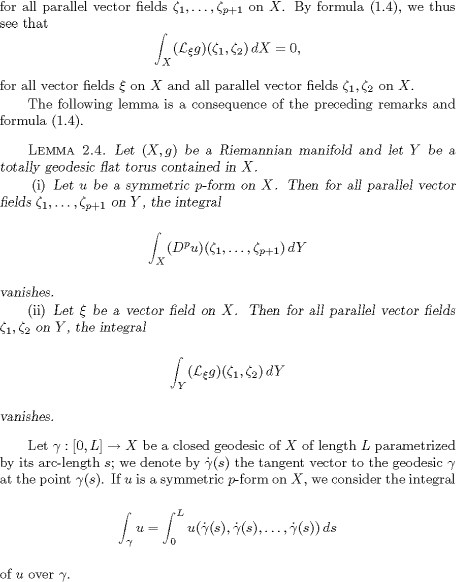
Definition 2.5. We say that a symmetric p-form u on X satisfies the zero-energy condition if, for every closed geodesi![]() of X, the integral of u over
of X, the integral of u over![]() vanishes.
vanishes.
Let Y be a totally geodesic submanifold of X; clearly, if ![]() is a symmetric p-form on X satisfying the zero-energy condition, then the restriction of
is a symmetric p-form on X satisfying the zero-energy condition, then the restriction of ![]() u to Y also satisfies the zero-energy condition. From Lemma 2.4, we obtain the following result:
u to Y also satisfies the zero-energy condition. From Lemma 2.4, we obtain the following result:
LEMMA 2.6. If u is a symmetric p-form on X, then the symmetric (p + 1)-form Dpu satisfies the zero-energy condition. A symmetric 2-form on X, which is equal to a Lie derivative of the metric g, satisfies the zero-energy condition.
DEFINITION 2.7. We say that the Riemannian metric g on X is a CL-metric if all its geodesics are periodic and have the same length L.
If g is a CL-metric, we say that X is a CL-manifold; then the geodesic flow on the unit tangent bundle of (X, g) is periodic with least period L.
The following proposition is due to Michel (Proposition 2.2.4 of [45]; see also Proposition 5.86 of [5]).
PROPOSITION 2.8. Let![]() be a one-parameter family of CL-metrics on X, for
be a one-parameter family of CL-metrics on X, for ![]() with g0 = g. Then the infinitesimal deformation h =
with g0 = g. Then the infinitesimal deformation h = ![]() of {gt} satisfies the zero-energy condition.
of {gt} satisfies the zero-energy condition.

From the previous equalities, we infer that
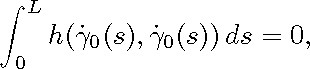
and so h satisfies the zero-energy condition.
DEFINITION 2.9. We say that a symmetric p-form u on a compact locally symmetric space X satisfies the Guillemin condition if, for every maximal
flat totally geodesic torus Z contained in X and for all unitary parallel vector fields ![]() on Z, the integral
on Z, the integral

vanishes.
From Lemma 2.4, we obtain:
LEMMA 2.10. Let X be a compact locally symmetric space. If u is a symmetric p-form on X, then the symmetric (p+1)-form Dpu satisfies the Guillemin condition. If ![]() is a vector field on X, the symmetric 2-form
is a vector field on X, the symmetric 2-form ![]() on X satisfies the Guillemin condition.
on X satisfies the Guillemin condition.
Thus every exact one-form on a compact locally symmetric space satisfies the Guillemin and zero-energy conditions.
LEMMA 2.11. Let X be a flat torus. A symmetric p-form on X satisfying the zero-energy condition also satisfies the Guillemin condition.
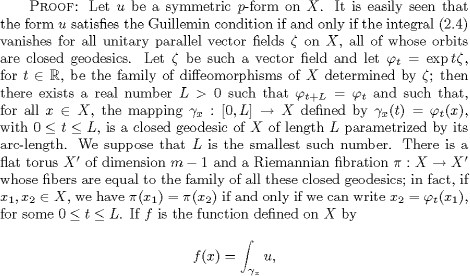
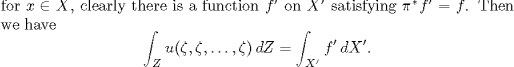
If u satisfies the zero-energy condition, then the functions f and ![]() vanish, and so, by the preceding formula,
vanish, and so, by the preceding formula, ![]() satisfies the Guillemin condition.
satisfies the Guillemin condition.
If (X, g) is a compact locally symmetric space, according to Lemma 2.11 a symmetric p-form on X satisfying the zero-energy condition also satisfies the Guillemin condition.
DEFINITION 2.12. We say that a compact locally symmetric space X is rigid in the sense of Guillemin (resp. infinitesimally rigid) if the only symmetric 2-forms on X satisfying the Guillemin (resp. the zero-energy) condition are the Lie derivatives of the metric g.
If X is a compact locally symmetric space X and p 0 is an integer, we consider the space ![]() of all sections of
of all sections of ![]() satisfying the zero-energy condition. According to Lemma 2.6, we have the inclusion
satisfying the zero-energy condition. According to Lemma 2.6, we have the inclusion
![]()
By formula (1.4), we see that the infinitesimal rigidity of the compact locally symmetric space X is equivalent to the equality
![]() On the other hand, the equality
On the other hand, the equality ![]() means that every differential form of degree 1 on X satisfying the zero-energy condition is exact.
means that every differential form of degree 1 on X satisfying the zero-energy condition is exact.
PROPOSITION 2.13. Let X be a compact locally symmetric space. Then the following assertions are equivalent:
(i) Every symmetric 2-form h on X, which satisfies the Guillemin (resp. the zero-energy) condition and the relation div h = 0, vanishes.
(ii) The space X is rigid in the sense of Guillemin (resp. is infinitesimally rigid).
PROOF: First assume that assertion (i) holds. Let ![]() h be a symmetric 2-form on X satisfying the Guillemin (resp. the zero-energy) condition. According to the decomposition (1.11),
we may write
h be a symmetric 2-form on X satisfying the Guillemin (resp. the zero-energy) condition. According to the decomposition (1.11),
we may write
![]()
where ![]() is an element of
is an element of ![]() satisfying div h0 = 0 and
satisfying div h0 = 0 and ![]() Clearly, by Lemma 2.10 (resp. Lemma 2.6), the symmetric 2-form h0 also satisfies the Guillemin (resp. the zero-energy) condition;
our assumption implies that
Clearly, by Lemma 2.10 (resp. Lemma 2.6), the symmetric 2-form h0 also satisfies the Guillemin (resp. the zero-energy) condition;
our assumption implies that![]() vanishes, and so h is a Lie derivative of the metric. Therefore (ii) is true. According to the decomposition (1.11), we see
that assertion (i) is a direct consequence of (ii).
vanishes, and so h is a Lie derivative of the metric. Therefore (ii) is true. According to the decomposition (1.11), we see
that assertion (i) is a direct consequence of (ii).
We now assume that (X, g) is a symmetric space of compact type. If the space X is rigid in the sense of Guillemin, it is also infinitesimally rigid. If X is a space of rank one, the closed geodesics of X are the maximal flat totally geodesic tori of X, and so the notions of Guillemin rigidity and infinitesimal rigidity for X are equivalent.
Consider a family of Riemannian metrics {gt} on X, for ![]() , with
, with
tg0 = g. We say that {gt} is an isospectral defo![]() rmation of g if the spectrum Spec(X, gt) of the metric
rmation of g if the spectrum Spec(X, gt) of the metric ![]() gt is equal to Spec(X, g), for all
gt is equal to Spec(X, g), for all ![]() < . We say that the space (X, g) is infinitesimally spectrally rigid (i.e., spectrally rigid to first-order) if, for every
such isospectral deformation {gt} of g, there is a one-parameter family of diffeomorphisms
< . We say that the space (X, g) is infinitesimally spectrally rigid (i.e., spectrally rigid to first-order) if, for every
such isospectral deformation {gt} of g, there is a one-parameter family of diffeomorphisms ![]() t of X such that gt
t of X such that gt ![]() to first-order in t at t = 0, or equivalently if the infinitesimal deformation d
to first-order in t at t = 0, or equivalently if the infinitesimal deformation d ![]() is a Lie derivative of the metric g.
is a Lie derivative of the metric g.
In [35], Guillemin proved the following result:
THEOREM 2.14. A symmetric 2-form on a symmetric space (X, g) of compact type, which is equal to the infinitesimal deformation of an isospectral deformation of g, satisfies the Guillemin condition.
This theorem leads us to Guillemin’s criterion for the infinitesimal spectral rigidity of a symmetric space of compact type which may be expressed as follows:
Theorem 2.15. If a symmetric space of compact type is rigid in the sense of Guillemin, it is infinitesimally spectrally rigid.
![]() 4. Radon transforms
4. Radon transforms
Let (X, g) be a symmetric space of compact type. Then there is a Riemannian symmetric pair (G,K) of compact type, where G
is a compact, connected semi-simple Lie group and K is a closed subgroup of G such that the space X is isometric to the homogeneous
space G/K endowed with a G-invariant metric. We identify X with G/K, and let x0 be the point of X corresponding to the coset
of the identity element of G in G/K. Since the maximal flat totally geodesic tori of X are conjugate under the action of G
on X, the space![]() of all such tori is a homogeneous space of G. We also consider the set
of all such tori is a homogeneous space of G. We also consider the set![]() of all closed geodesics of X; when the rank of X is equal to one, the
of all closed geodesics of X; when the rank of X is equal to one, the![]() n is equal to .
n is equal to .
A Radon transform for functions on X assigns to a function on X its integrals over a class of totally geodesic submanifolds of X of a fixed dimension. Here we shall consider two such Radon transforms, the maximal flat Radon transform and the X-ray transform.
The maximal flat Radon transform for functions on X assigns to a real-valued function f on X the function ![]() f on
f on ![]() , whose value at a torus Z is the integral
, whose value at a torus Z is the integral
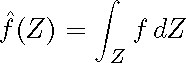
of f over Z. Clearly this transform is injective if every function on X satisfying the Guillemin condition vanishes. The X-ray
transform for functions on X assigns to a real-valued function f on X the function ![]() on
on ![]() , whose value at a closed geode
, whose value at a closed geode![]() sic is the integral
sic is the integral
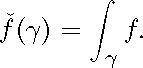
Clearly this transform is injective if every function on X satisfying the zero-energy condition vanishes. If the rank of X is equal to one, the maximal flat Radon transform for functions on X coincides with the X-ray transform for functions on X.
Let f be a real-valued function on X. If Z is a torus belonging to and if is a unitary parallel vector field on Z, then we see that
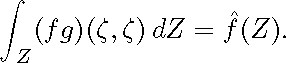
On the other hand, if ![]() is a closed geodesic of X, we have
is a closed geodesic of X, we have

Thus the maximal flat Radon (resp. the X-ray) transform of f vanishes if and only if the symmetric 2-form fg satisfies the Guillemin (resp. the zero-energy) condition.
If X is an irreducible symmetric space of compact type, we recall that g is an Einstein metric and that Ric ![]() = g, where is a positive real number; moreover, the space E(X) of infinitesimal Einstein deformations of the metric g is a
G-submodule of
= g, where is a positive real number; moreover, the space E(X) of infinitesimal Einstein deformations of the metric g is a
G-submodule of ![]()
PROPOSITIOn 2.16. Let X be an irreducible symmetric space of compact type, which is not isometric to a sphere. If X is rigid in the sense of Guillemin (resp. is infinitesimally rigid), then the maximal flat Radon (resp. the X-ray) transform for functions on X is injective.
PROOF: Assume that X is rigid in the sense of Guillemin (resp. is infinitesimally rigid). Let f be real-valued function on
the Einstein manifold X; suppose that the function ![]() (resp. the function
(resp. the function ![]() vanishes. Then the symmetric 2-form fg on X satisfies the Guillemin (resp. the zero-energy) condition. Therefore we may write
vanishes. Then the symmetric 2-form fg on X satisfies the Guillemin (resp. the zero-energy) condition. Therefore we may write
![]() where is a vector field on X. According to Proposition 1.6, the function f vanishes, and so the corresponding Radon transform
for functions is injective.
where is a vector field on X. According to Proposition 1.6, the function f vanishes, and so the corresponding Radon transform
for functions is injective.
Let ![]() be a finite group of isometries of X of order q. If F is a vector bundle equal either to a sub-bundle of
be a finite group of isometries of X of order q. If F is a vector bundle equal either to a sub-bundle of ![]() TC or to a sub-bundle of
TC or to a sub-bundle of ![]() invariant under the group
invariant under the group![]() , we denote by
, we denote by ![]() )the space consisting of all
)the space consisting of all![]() -invariant sections of F; if the vector bundle F is also invariant under the group G and if the isometries
-invariant sections of F; if the vector bundle F is also invariant under the group G and if the isometries ![]() of commute with the action of G, then
of commute with the action of G, then ![]() is a G-submodule of
is a G-submodule of ![]() If F is the trivial complex line bundle, we consider the G-submodule
If F is the trivial complex line bundle, we consider the G-submodule ![]() of -invariant functions on X.
of -invariant functions on X.
We suppose that the group acts without fixed points. Then the quotient ![]() is a manifold and the natural projection
is a manifold and the natural projection![]() : X Y is a covering projection. Thus the metric g induces a Riemannian metric
: X Y is a covering projection. Thus the metric g induces a Riemannian metric ![]() on Y such
on Y such ![]() at gY = g. Clearly the space Y is locally symmetric.
at gY = g. Clearly the space Y is locally symmetric.
A symmetric p-form ![]() on X is invariant under the group if and only if there is a symmetric p-form
on X is invariant under the group if and only if there is a symmetric p-form ![]() on Y such that
on Y such that ![]() The projection induces an isomorphism
The projection induces an isomorphism

If X is an irreducible symmetric space, then X and Y are Einstein manifolds; according to the definition of the spaces E(X)
and E(Y ) of infinitesimal Einstein deformations, we see that the projection![]() and the isomorphism (2.5) induce an isomorphism
and the isomorphism (2.5) induce an isomorphism

Throughout the remainder of this section, we also suppose that the isometries of commute with the action of G on X; then Y
is a homogeneous space of G. Assume furthermore that there is a subgroup ![]() of G containing K and a G-equivariant diffeomorphism
of G containing K and a G-equivariant diffeomorphism ![]() which have the following properties:
which have the following properties:
(i) (G,![]() ) is a Riemannian symmetric pair;
) is a Riemannian symmetric pair;
(ii) when we identify X with G/K, the project![]() ion is equal to the natural projection
ion is equal to the natural projection ![]() .
.
Under these conditions, the space (Y, gY ) is isometric to the symmetric space ![]() of compact type endowed with a G-invariant metric.
of compact type endowed with a G-invariant metric.

holds for all symmetric p-forms ![]() on Y .
on Y .
From the above observations, we deduce
From the above observations, we deduce the following:
LEMMA 2.17. Suppose that the quotient ![]() is a symmetric space. Then a symmetric p-form u on Y satisfies the Guillemin (resp. the zero-energy) condition if and only
if the symmetric p-form
is a symmetric space. Then a symmetric p-form u on Y satisfies the Guillemin (resp. the zero-energy) condition if and only
if the symmetric p-form ![]() u on X, which is invariant under the group , satisfies the Guillemin (resp. the zero-energy) condition.
u on X, which is invariant under the group , satisfies the Guillemin (resp. the zero-energy) condition.
By Lemma 2.17, we see that the maximal flat Radon (resp. the X-ray) transform for functions on Y is injective if and only
if the restriction of the the maximal flat Radon (resp. the X-ray) transform for functions on X to the space ![]() is injective. From Lemma 2.17 and the equality (2.6), we deduce the following three results:
is injective. From Lemma 2.17 and the equality (2.6), we deduce the following three results:
PROPOSITION 2.18. Suppose that the quotient ![]() is a symmetric space. Then the following assertions are equivalent:
is a symmetric space. Then the following assertions are equivalent:
(i) Every symmetric 2-form on the space X, which is invariant under the group and satisfies the Guillemin condition, is a Lie derivative of the metric.
(ii) The space Y is rigid in the sense of Guillemin.
PROPOSITION 2.19. Suppose that the quotient ![]() is a symmetric space. Then the following assertions are equivalent:
is a symmetric space. Then the following assertions are equivalent:
(i) Every symmetric 2-form on the space X, which is invariant under the group and satisfies the zero-energy condition, is a Lie derivative of the metric.
(ii) The space Y is infinitesimally rigid.
PROPOSITION 2.20. Suppose that the quotient ![]() is a symmetric space. Then the following assertions are equivalent:
is a symmetric space. Then the following assertions are equivalent:
(i) Every differential form of degree 1 on the space X, which is invariant under the group and satisfies the Guillemin (resp. the zero-energy) condition, is exact.
(ii) Every differential form of degree 1 on the space Y , which satisfies the Guillemin (resp. the zero-energy) condition, is exact.
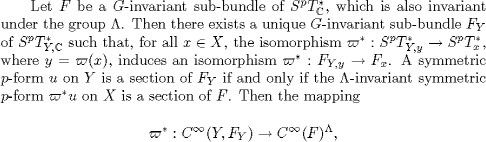
induced by (2.5), is an isomorphism of G-modules.
The following proposition is a consequence of Lemma 2.17.
PROPOSITION 2.21. Suppose that the quotient ![]() is a symmetric space. Let F be a sub-bundle of
is a symmetric space. Let F be a sub-bundle of ![]() SpTinvariant under the groups G and
SpTinvariant under the groups G and ![]() , and let FY be the G-invariant sub-bundle of
, and let FY be the G-invariant sub-bundle of ![]() induced by F. Then the following assertions are equivalent:
induced by F. Then the following assertions are equivalent:
(i) Any section of the vector bundle F over the space X, which is invariant under the group and satisfies the Guillemin (resp. the zero-energy) condition, vanishes.
(ii) Any section of the vector bundle FY over the space Y , which satisfies the Guillemin (resp. the zero-energy) condition, vanishes.
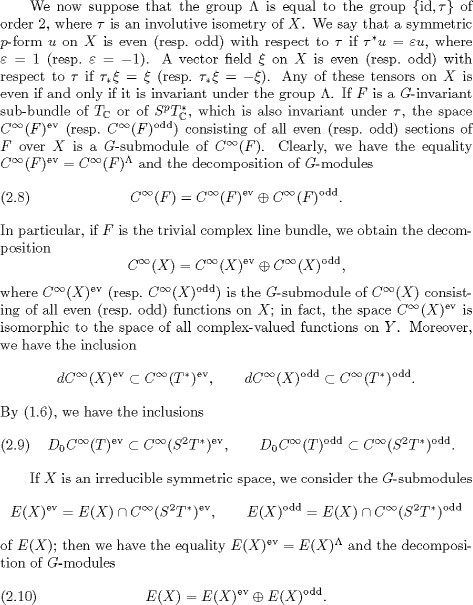
Let Z be a maximal flat totally geodesic torus of X. Since pr![]() eserves Z, if f is an odd function on X, we see that the integral of f over Z vanishes. Therefore the odd functions on X satisfy
the Guillemin condition, and so belong to the kernel of the maximal flat Radon transform for functions.
eserves Z, if f is an odd function on X, we see that the integral of f over Z vanishes. Therefore the odd functions on X satisfy
the Guillemin condition, and so belong to the kernel of the maximal flat Radon transform for functions.
PROPOSITION 2.22. We suppose that the group ![]() is equal to the group {id,
is equal to the group {id, ![]() } of order 2, where
} of order 2, where![]() is an involutive isometry of X, and that the quotient
is an involutive isometry of X, and that the quotient ![]() is a symmetric space. Then an odd symmetric p-form on X satisfies the Guillemin condition, and the maximal flat Radon transform
for functions on X is not injective. Moreover, the space X is not rigid in the sense of Guillemin.
is a symmetric space. Then an odd symmetric p-form on X satisfies the Guillemin condition, and the maximal flat Radon transform
for functions on X is not injective. Moreover, the space X is not rigid in the sense of Guillemin.
PROOF: Let ![]() u be an odd symmetric p-form on X and let Z be a maximal flat totally geodesic torus of X. I
u be an odd symmetric p-form on X and let Z be a maximal flat totally geodesic torus of X. I![]() f is a parallel vector field on Z, then the function (
f is a parallel vector field on Z, then the function (![]() on Z is odd, that is,
on Z is odd, that is,
![]()
for all ![]() hence its integral over Z vanishes. We now construct an odd symmetric 2-form
hence its integral over Z vanishes. We now construct an odd symmetric 2-form ![]() on X which is not a Lie derivative of the metric. Let
on X which is not a Lie derivative of the metric. Let ![]() be a point of X and U be a open neighborhood of x for which
be a point of X and U be a open neighborhood of x for which ![]() . By Lemma 1.13 and remarks made in
. By Lemma 1.13 and remarks made in ![]() , Chapter I, we know that the infinitesimal orbit of the curvature
, Chapter I, we know that the infinitesimal orbit of the curvature ![]() Bis a vector bundle and that the quotient bundle
Bis a vector bundle and that the quotient bundle ![]() B/Bis non-zero. According to
B/Bis non-zero. According to![]() 1, Chapter I, the morphism (D1) : S2TS2
1, Chapter I, the morphism (D1) : S2TS2![]() TB/Bis surjective; hence we may choose a symmetric 2-form h on X whose support is contained in U and which satisfies (D1h)(x
TB/Bis surjective; hence we may choose a symmetric 2-form h on X whose support is contained in U and which satisfies (D1h)(x![]() ) = 0. We know that h is not a Lie derivative of the metric on any neighborhood of x. The symmetric 2-form h
) = 0. We know that h is not a Lie derivative of the metric on any neighborhood of x. The symmetric 2-form h![]() = h - h on X is odd and its restriction to U is equal to h. Hence the form
= h - h on X is odd and its restriction to U is equal to h. Hence the form ![]() hsatisfies the Guillemin condition, and so the space X is not rigid in the sense of Guillemin.
hsatisfies the Guillemin condition, and so the space X is not rigid in the sense of Guillemin.
In ![]() Chapter III, we shall prove that the X-ray transform for functions on the sphere Sn, with
Chapter III, we shall prove that the X-ray transform for functions on the sphere Sn, with ![]() is injective on the space of all even functions (see Proposition 3.17). Clearly, this result is equivalent to assertion (i)
of the following theorem. By Lemma 2.17, we know that assertions (i) and (ii) of this theorem are equivalent. We point out
that assertion (i) of this theorem in the case of the 2-sphere S2 is a classic result due to Funk.
is injective on the space of all even functions (see Proposition 3.17). Clearly, this result is equivalent to assertion (i)
of the following theorem. By Lemma 2.17, we know that assertions (i) and (ii) of this theorem are equivalent. We point out
that assertion (i) of this theorem in the case of the 2-sphere S2 is a classic result due to Funk.
THEOREM 2.23. (i) The kernel of the X-ray transform for functions on the sphere (Sn, g0), with n![]() 2, is equal to the space of all odd functions on Sn.
2, is equal to the space of all odd functions on Sn.
(ii) The X-ray transform for functions on the real projective space ![]() with n
with n![]() 2, is injective.
2, is injective.
The adjoint space of the symmetric space X is the symmetric space which admits X as a Riemannian covering and is itself not
a Riemannian covering of another symmetric space. For example, the adjoint space of the n-sphere Sn, with n 2, is the real
projective space ![]()
In [34], Grinberg generalized Theorem 2.23 and proved the following:
THEOREM 2.24. The maximal flat Radon transform for functions on a symmetric space X of compact type is injective if and only if X is equal to its adjoint space.
By Proposition 2.22, the sphere ![]() is not infinitesimally rigid. Hence from Proposition 2.16 and Theorem 2.24, we obtain the following necessary condition for
Guillemin rigidity:
is not infinitesimally rigid. Hence from Proposition 2.16 and Theorem 2.24, we obtain the following necessary condition for
Guillemin rigidity:
THEOREM 2.25. Let X be an irreducible symmetric space of compact type. If X is rigid in the sense of Guillemin, then X is equal to its adjoint space.
In Chapter III, we shall show that the X-ray transform for functions on a flat torus of dimension > 1 is injective; this result is due to Michel [46] (see Proposition 3.5). If the symmetric space X is of rank q, each point of X is contained in a totally geodesic flat torus of dimension q of X (see Theorem 6.2 in Chapter V of [36]). Thus from the injectivity of the X-ray transform for functions on a flat torus, we deduce the following:
PROPOSITION 2.26. The X-ray transform for functions on a symmetric space X of compact type of rank > 1 is injective.
We now extend the definitions of the maximal flat Radon transform and the X-ray transform to symmetric p-forms. Let L be the
vector bundle over![]() whose fiber at a point Z is the space of all parallel vector fields on the flat torus Z. This vector bundle is a homogeneous
G-bundle over and its rank is equal to the rank of the symmetric space X. We consider the p-th symmetric product
whose fiber at a point Z is the space of all parallel vector fields on the flat torus Z. This vector bundle is a homogeneous
G-bundle over and its rank is equal to the rank of the symmetric space X. We consider the p-th symmetric product ![]() of the dual
of the dual ![]() Lof L. The space
Lof L. The space ![]() of all symmetric p-forms on X and the space (
of all symmetric p-forms on X and the space (![]() of all sections of
of all sections of ![]() ove
ove ![]() are G-modules. The maximal flat Radon transform for symmetric p-forms on X is the morphism of G-modules
are G-modules. The maximal flat Radon transform for symmetric p-forms on X is the morphism of G-modules
![]()
which assigns to a symmetric p-form u on X the section ![]() of
of ![]() who se value at the point
who se value at the point ![]() Z is determined by
Z is determined by
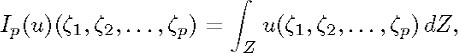
where 1, 2![]() , . . . , p are elements of LZ. The kernel Np of this mapping Ip is the G-submodule of
, . . . , p are elements of LZ. The kernel Np of this mapping Ip is the G-submodule of ![]() equal to the space consisting of all symmetric p-forms on X which satisfy the Guillemin condition. The com-plexification
equal to the space consisting of all symmetric p-forms on X which satisfy the Guillemin condition. The com-plexification
![]() of the space
of the space ![]() shall be viewed as the G-submodule of
shall be viewed as the G-submodule of ![]() equal to the kernel of the morphism of G-modules
equal to the kernel of the morphism of G-modules
![]()
induced by the mapping Ip. The mapping I0 coincides with the maximal flat Radon transform for functions defined above, while the mapping I2 was introduced in [23].
The X-ray transform for symmetric p-forms on X is the linear mapping ![]() sending an element (SpT) into the real-valued function on p
sending an element (SpT) into the real-valued function on p![]() u Cu whose value at the closed geodesic is the integral
u Cu whose value at the closed geodesic is the integral
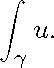
The kernel of this mapping ![]() is equal to the space
is equal to the space ![]() of all symmetric p-forms on X satisfying the zero-energy condition. Then according to Lemma 2.11, we have
of all symmetric p-forms on X satisfying the zero-energy condition. Then according to Lemma 2.11, we have
![]()

fo![]() r G, is a morphism of G-modules. The complexification
r G, is a morphism of G-modules. The complexification ![]() of the space
of the space ![]() shall be viewed as the G-submodule
shall be viewed as the G-submodule
of ![]() consisting of all complex symmetric p-forms on X which satisfy the zero-energy condition.
consisting of all complex symmetric p-forms on X which satisfy the zero-energy condition.
When the rank of X is equal to one, the vector bundle L is a line bundle; in this case, the X-ray transform for symmetric p-forms, which may be viewed as a morphism of G-modules
![]()
determines the maximal flat Radon transform for symmetric p-forms.
![]() 5. Radon transforms and harmonic analysis
5. Radon transforms and harmonic analysis
Let 0 be the subset of consisting of those element![]() s of for which the G-module
s of for which the G-module ![]() is non-zero. It is well-known that
is non-zero. It is well-known that![]() , for 0, the G-module
, for 0, the G-module ![]() is irreducible (see Theorem 4.3 in Chapter V of [37]).
is irreducible (see Theorem 4.3 in Chapter V of [37]).


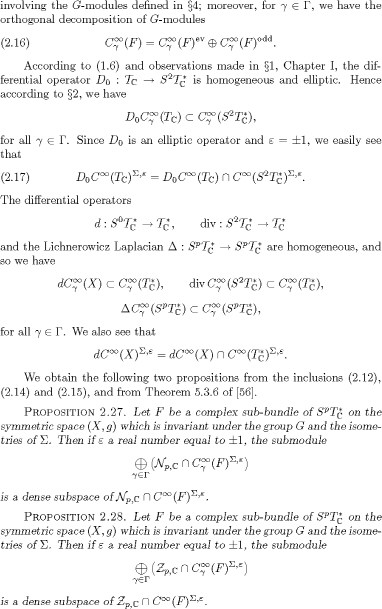
The following proposition is a direct consequence of Propositions 2.27 and 2.28.
PROPOSITION 2.29. Let (X, g) be a symmetric space of compact type. Let ![]() be a finite set of isometries of X which commute with the action of G on X and let
be a finite set of isometries of X which commute with the action of G on X and let![]() be a real number equal to
be a real number equal to ![]() .
.
±(i) The restriction of the maximal flat Radon transform for functions on X to the space ![]() ,is injective if and only if the equality
,is injective if and only if the equality

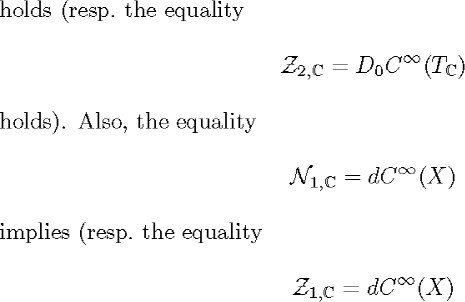
implies) that any differential form of degree 1 on X satisfying the Guillemin (resp. the zero-energy) condition is exact.
PROPOSITION 2.30. Let (X, g) be a symmetric space of compact type. Let ![]() be a finite set of isometries of X which commute with the action of G on X and let
be a finite set of isometries of X which commute with the action of G on X and let![]() be a real number equal to
be a real number equal to ![]() .
.
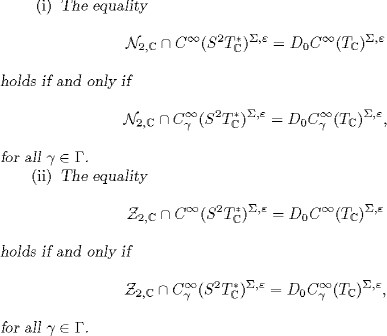
PROOF: Since D0 is an elliptic homogeneous differential operator, the assertions of the proposition follow from the first inclusion of (2.15), the equality (2.17) and from Propositions 2.2,(iii), 2.27 and 2.28.
PROPOSITION 2.30 gives us the following criteria for the Guillemin rigidity and the infinitesimal rigidity of X, which are analogous to the criteria for the injectivity of the Radon transforms for functions on X obtained from Proposition 2.29:
PROPOSITION 2.31. Let (X, g) be a symmetric space of compact type.
(i) The space X is rigid in the sense of Guillemin if and only if
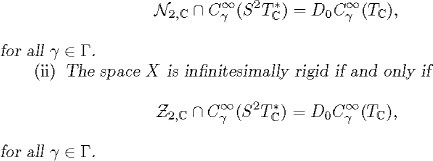
PROPOSITION 2.32. Let (X, g) be a symmetric space of compact type. Let ![]() be a finite set of isometries of X which commute with the action of G on X and let
be a finite set of isometries of X which commute with the action of G on X and let![]() be a real number equal to
be a real number equal to ![]() .
.
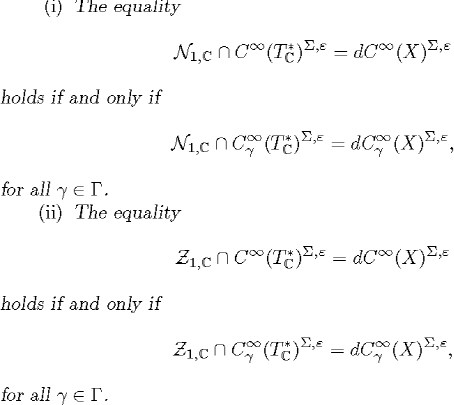
PROOF: Since the exterior differential operator d acting on ![]() is an elliptic homogeneous differential operator, the assertions of the proposition follow from (2.15) and Propositions 2.2,(iii),
2.27 and 2.28.
is an elliptic homogeneous differential operator, the assertions of the proposition follow from (2.15) and Propositions 2.2,(iii),
2.27 and 2.28.
PROPOSITION 2.32 gives us the following criteria, which are analogous to the criteria for the Guillemin rigidity and the infinitesimal rigidity of X given by Proposition 2.31:
PROPOSITION 2.33. Let (X, g) be a symmetric space of compact type. The following assertions are equivalent:
(i) A differential form of degree 1 on the space X satisfies the Guil-lemin (resp. the zero-energy) condition if and only if it is exact.

Suppose that ![]() is equal to a finite group of isometries of X which commute with the action of G and that
is equal to a finite group of isometries of X which commute with the action of G and that![]() is equal to +1. Assume that acts without fixed points and that the quotient space
is equal to +1. Assume that acts without fixed points and that the quotient space ![]() is a symmetric space; furthermore, assume that there is a subgroup
is a symmetric space; furthermore, assume that there is a subgroup ![]() of G containing K and a G-equivariant diffeomorphism
of G containing K and a G-equivariant diffeomorphism ![]() satisfying properties (i) and (ii) o
satisfying properties (i) and (ii) o![]() 4. If the vector bundle F is a sub-bundle of
4. If the vector bundle F is a sub-bundle of ![]() which is invariant under , we consider the sub-bundle FY of
which is invariant under , we consider the sub-bundle FY of ![]() determined by F; then
determined by F; then ![]() for , the G-submodule
for , the G-submodule

of G-modules.
We choose a Cartan subalgebra of the complexification g of the Lie algebra of G and fix a system of positive roots of![]() g. Let p be an integer equal to 1 or 2 and consider the corresponding homogeneous differential operator
g. Let p be an integer equal to 1 or 2 and consider the corresponding homogeneous differential operator ![]() We consider the following properties which the space X and the group might possess:
We consider the following properties which the space X and the group might possess:
(Ap) L![]() et be an arbitrary element of , and let u be an arbitrary highest weight vector of the G-module
et be an arbitrary element of , and let u be an arbitrary highest weight vector of the G-module ![]() if the section u satisfies the Guillemin condition, then u belongs to
if the section u satisfies the Guillemin condition, then u belongs to ![]() ).
).
(Bp) ![]() Let be an arbitrary element of , and let u be an arbitrary highest weight vector of the G-module
Let be an arbitrary element of , and let u be an arbitrary highest weight vector of the G-module ![]() if the section u satisfies the zero-energy condition, then u belongs to
if the section u satisfies the zero-energy condition, then u belongs to ![]() ).
).
According to the relation (1.4) and Propositions 2.30 and 2.32, we see that in order to prove that the equality
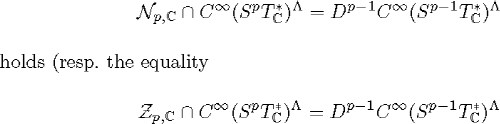
holds), it suffices to verify that X and possess property Ap (resp. property Bp).
Thus according to Proposition 2.18 (resp. Proposition 2.19) and the relation (1.4), we know that, if the space X and the group possess property A2 (resp. property B2), the space Y is rigid in the sense of Guillemin (resp. is infinitesimally rigid). On the other hand, according to Proposition 2.20, if the space X and the group possess property A1 (resp. property B1), then every differential form of degree 1 on the space Y , which satisfies the Guillemin (resp. the zero-energy) condition, is exact.
These methods for proving the rigidity of a symmetric space of compact type were first introduced in [14] in the case of the
complex projective space (see![]() 5, Chapter III). The analogous method for proving the injectivity of Radon transforms for functions described above was first
used by Funk to prove Theorem 2.23 for the 2-sphere S2 and the real projective plane
5, Chapter III). The analogous method for proving the injectivity of Radon transforms for functions described above was first
used by Funk to prove Theorem 2.23 for the 2-sphere S2 and the real projective plane ![]() (see also Proposition 3.17); it was also applied by Grinberg in [31] to other projective spaces. The methods described above
will be applied to the real Grassmannian
(see also Proposition 3.17); it was also applied by Grinberg in [31] to other projective spaces. The methods described above
will be applied to the real Grassmannian ![]() of 2-planes in
of 2-planes in ![]() and to the complex quadric
and to the complex quadric ![]() Qn of dimension n. In fact, they shall be used in Chapter VI to show that the real Grassmannian
Qn of dimension n. In fact, they shall be used in Chapter VI to show that the real Grassmannian ![]() is rigid in the sense of Guillemin and that the complex quadric
is rigid in the sense of Guillemin and that the complex quadric ![]() is infinitesimally rigid, and i
is infinitesimally rigid, and i![]() n 4, Chapter X to show that the real Grassmannian
n 4, Chapter X to show that the real Grassmannian ![]() is infinitesimally rigid. Also the criterion for the exactness of a differential form of degree 1, which we have just described,
shall be used in the case of the real projective plane
is infinitesimally rigid. Also the criterion for the exactness of a differential form of degree 1, which we have just described,
shall be used in the case of the real projective plane ![]() in Chapter III, in the case of the complex quadric
in Chapter III, in the case of the complex quadric ![]() and of the real Grassmannian
and of the real Grassmannian ![]() with n 3, in
with n 3, in![]() 11, Chapter VI, and in the case of the real Grassmannian
11, Chapter VI, and in the case of the real Grassmannian ![]() in
in![]() 4, Chapter X.
4, Chapter X.
![]() 6. Lie algebras
6. Lie algebras
Let g be a complex semi-simple Lie algebra. The Casimir element of ![]() operates by a scalar (
operates by a scalar (![]() on an irreducible finite-dimensional g-module
on an irreducible finite-dimensional g-module ![]() If g is simple, the Casimir element of
If g is simple, the Casimir element of ![]() acts on the irreducible g-module
acts on the irreducible g-module ![]() corresponding to the adjoint representation of g by the identity mapping, and so
corresponding to the adjoint representation of g by the identity mapping, and so ![]() (see Theorem 3.11.2 of [55]).
(see Theorem 3.11.2 of [55]).
LEMMA 2.34. Let ![]() be a complex semi-simple Lie algebra. Let V1 and V2 be irreducible finite-dimensional
be a complex semi-simple Lie algebra. Let V1 and V2 be irreducible finite-dimensional ![]() modules. Then the
modules. Then the ![]() modules V1 and V2 are isomorphic if and only if c(V1) = c(V2).
modules V1 and V2 are isomorphic if and only if c(V1) = c(V2).
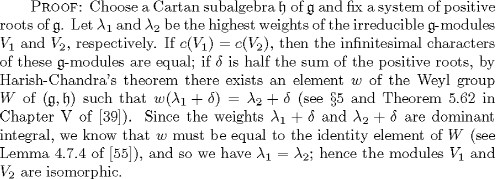
Let G be a compact connected, semi-simple Lie group, whose Lie algebra we denote by ![]() A complex G-module V can be viewed as a
A complex G-module V can be viewed as a ![]() g0-module and so the Casimir element of
g0-module and so the Casimir element of![]() operates on V ; if V is an irreducible G-module, the Casimir element of
operates on V ; if V is an irreducible G-module, the Casimir element of ![]() acts by the scalar c(V ) on V .
acts by the scalar c(V ) on V .
From Lemma 2.34, we obtain the following result:
LEMMA 2.35. Let G be a compact connected, semi-simple Lie group. Let V1 and V2 be irreducible complex G-modules. Then the G-modules V1 and V2 are isomorphic if and only if c(V1) = c(V2).
7. Irreducible symmetric spaces
We consider the symmetric space (X, g) of compact type of![]() 4. We write X as the homogeneous space G/K, where G is a compact, connected semi-simple Lie group and K is a closed subgroup
of G. We suppose that g is a G-invariant metric and that (G,K) is a Riemannian symmetric pair of compact type. We continue
to denote by
4. We write X as the homogeneous space G/K, where G is a compact, connected semi-simple Lie group and K is a closed subgroup
of G. We suppose that g is a G-invariant metric and that (G,K) is a Riemannian symmetric pair of compact type. We continue
to denote by![]() the dual
the dual ![]() of the group G. Let
of the group G. Let ![]() x0 be the point of X corresponding to the coset of the identity element of G. If
x0 be the point of X corresponding to the coset of the identity element of G. If ![]() and
and![]() are the Lie algebras of G and K, respectively, we consider the Cartan decomposition
are the Lie algebras of G and K, respectively, we consider the Cartan decomposition ![]() corresponding to the Riemannian symmetric pair (G,K), where
corresponding to the Riemannian symmetric pair (G,K), where ![]() is a subspace of
is a subspace of ![]() We identify
We identify ![]() with the tangent space to X at the point x0. If B is the Killing form of the Lie algebra
with the tangent space to X at the point x0. If B is the Killing form of the Lie algebra ![]() of G, then the restriction of -B to
of G, then the restriction of -B to ![]() induces a G-invariant Riemannian metric
induces a G-invariant Riemannian metric ![]() on X. The complexifications
on X. The complexifications ![]() of
of ![]() and
and ![]() of
of ![]() are K-modules. We denote by Skp the k-th symmetric product of
are K-modules. We denote by Skp the k-th symmetric product of ![]() and by
and by ![]() the K-submodule of S2p consisting of those elements of S2p of trace zero with respect to the Killing form of
the K-submodule of S2p consisting of those elements of S2p of trace zero with respect to the Killing form of![]() g. The isotropy group K acts on
g. The isotropy group K acts on ![]() in fact, the K-modules
in fact, the K-modules ![]() and S2 x0 0TC,are isomorphic to the x0
and S2 x0 0TC,are isomorphic to the x0
K-modules ![]() and
and ![]() respectively. If X is an irreducible Hermitian symmetric space, then we have
respectively. If X is an irreducible Hermitian symmetric space, then we have
![]()
If X is an irreducible symmetric space which is not Hermitian, then we have
![]()
The G-module
![]()
of all Killing vector fields on X is isomorphic to ![]() We identify its com-plexification
We identify its com-plexification ![]() with the G-module
with the G-module
![]()
of complex vector fields on X, which is isomorphic to ![]() We know that a Killing vector fiel
We know that a Killing vector fiel![]() on X satisfies the relation
on X satisfies the relation ![]() thus the subspaces g(KC) and dC(X) of C(TC) are orthogonal.
thus the subspaces g(KC) and dC(X) of C(TC) are orthogonal.
![]() K) The
K) The ![]() Lichnerowicz Laplacian
Lichnerowicz Laplacian ![]() acting on
acting on ![]() is self-adjoint and its eigenvalues are non-negative real numbers. Since the Laplacian
is self-adjoint and its eigenvalues are non-negative real numbers. Since the Laplacian ![]() acting on
acting on ![]() SpTCis elliptic, the eigenspace
SpTCis elliptic, the eigenspace
![]()
of the Laplacian ![]() corresponding to the eigenvalue
corresponding to the eigenvalue ![]() is finite-dimensional. The Casimir element of
is finite-dimensional. The Casimir element of ![]() acts by a scalar
acts by a scalar ![]() an irreducible G-module which is a representative
an irreducible G-module which is a representative ![]() of . According to [41, 5], the action of the Lichnerowicz Laplacian
of . According to [41, 5], the action of the Lichnerowicz Laplacian
![]()
corresponding to the metric ![]() on X on the G-module
on X on the G-module ![]() coincides with the action of the Casimir element of g0 on this G-module. Thus, fo
coincides with the action of the Casimir element of g0 on this G-module. Thus, fo![]() we see that
we see that ![]() is an eigenspace of the Lichnerowicz Laplacian g0 with eigenvalue c. Since the operato
is an eigenspace of the Lichnerowicz Laplacian g0 with eigenvalue c. Since the operato ![]() acting on
acting on ![]() is elliptic
is elliptic![]() and real-analytic, the elements of
and real-analytic, the elements of ![]() C) are real-analytic sections of
C) are real-analytic sections of ![]() If F is a complex sub-bundle of
If F is a complex sub-bundle of ![]() invariant under the group G, then the Laplaci
invariant under the group G, then the Laplaci![]() an preserves the space
an preserves the space ![]()
We suppose throughout the remainder of this section that X is an irreducible symmetric space. According to Lemma 1.21, the
metric g is a positive multiple of g0 and is an Einstein metric. In fact, by formula (1.65), we have Ric =![]() , where is a positive real number, and
, where is a positive real number, and ![]() = moreover, the Lichnerowicz Laplacian corresponding to the metric g is equal
= moreover, the Lichnerowicz Laplacian corresponding to the metric g is equal ![]() . Let F be a complex sub-bundle of
. Let F be a complex sub-bundle of ![]() invariant under the group
invariant under the group ![]() from Lemma 2.35 and the above remarks concerning the Laplacia
from Lemma 2.35 and the above remarks concerning the Laplacia![]() we infer that the G-submodule
we infer that the G-submodule ![]() is equal to the eigenspace of
is equal to the eigenspace of![]() acting on
acting on ![]() associated with the eigenval
associated with the eigenval![]() Moreover, by Proposition 2.1 and the preceding remark, for
Moreover, by Proposition 2.1 and the preceding remark, for ![]() we see that, if the eigenspace
we see that, if the eigenspace

(Xfor al![]() If is an element of , according to (2.12) (resp. to (2.14)), we know that
If is an element of , according to (2.12) (resp. to (2.14)), we know that ![]() belongs to
belongs to ![]() (resp. to
(resp. to ![]() therefore so does
therefore so does ![]() . Since
. Since ![]() (resp.
(resp. ![]() is a closed subspace of
is a closed subspace of ![]() we see that
we see that ![]() also belongs to this subspace.
also belongs to this subspace.
PROPOSITION 2.37. Let (X, g) be an irreducible symmetric space of compact type. Let E be a G-invariant sub-bundle of ![]() and let h be a symmetric 2-form on X. Assume that there is a real number ì such that
and let h be a symmetric 2-form on X. Assume that there is a real number ì such that
![]()
(i) If ì is not an eigenvalue of the Laplacia![]() n acting on
n acting on ![]() then h is a section of E.
then h is a section of E.
(ii) Assume that ì is an eigenvalue of the Laplacia![]() n acting on
n acting on ![]() and suppose that h satisfies div h = 0 and Tr h = 0. Then we can write h = h1 +h2, where h1 is a section of
and suppose that h satisfies div h = 0 and Tr h = 0. Then we can write h = h1 +h2, where h1 is a section of ![]() and
and ![]() is a section of E satisfying
is a section of E satisfying
![]()
moreover, if h satisfies the Guillemin (resp. the zero-energy) condition, then we may require that h1 and h2 also satisfy the Guillemin (resp. the zero-energy) condition.

The desired result is a direct consequence of formulas (1.39) and (1.10).
We now further assume that X is of type I, i.e. is not equal to a simple Lie group (see [36, p. 439]). We may suppose that
the Lie group G is simple; then the complexification ![]() g of the Lie algebra
g of the Lie algebra ![]() g0 is simple. Let
g0 is simple. Let ![]() 1 be the element of
1 be the element of![]() which is the equivalence class of the irreducible G-module g
which is the equivalence class of the irreducible G-module g![]() . We know that c1
. We know that c1 ![]() = 1, and hence we have 1
= 1, and hence we have 1 ![]() = 2. This observation and the above remarks concerning the Lichnerowicz Laplacians, together with the Frobenius reciprocity
theorem, give us the following result:
= 2. This observation and the above remarks concerning the Lichnerowicz Laplacians, together with the Frobenius reciprocity
theorem, give us the following result:

Since E(X)C is G-submodule of ![]() we know that E(X)C is equal to the direct sum of k copies of the irreducible G-module
we know that E(X)C is equal to the direct sum of k copies of the irreducible G-module ![]() , where
, where ![]() is the integer MultE(X)C; it follows that the G-module E(X) is isomorphic to the direct sum of k copies of g0. Moreover,
we infer that the vanishing of the space dimC HomK
is the integer MultE(X)C; it follows that the G-module E(X) is isomorphic to the direct sum of k copies of g0. Moreover,
we infer that the vanishing of the space dimC HomK![]() implies that the space E(X) vanishes.
implies that the space E(X) vanishes.
Since the G-module KC is isomorphic to g, we see that
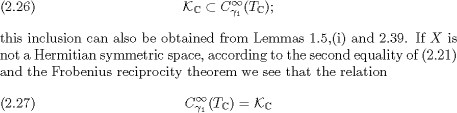
holds. If X is a Hermitian symmetric space, by (2.20) we see that ![]() is an irreducible G-module; since the decomposition of
is an irreducible G-module; since the decomposition of ![]() given by (1.69) is G-invariant, by (2.20) we obtain the orthogonal decompositions
given by (1.69) is G-invariant, by (2.20) we obtain the orthogonal decompositions
![]()
whose components ![]() and
and ![]() are irreducible G-modules isomorphic to
are irreducible G-modules isomorphic to ![]() .
.
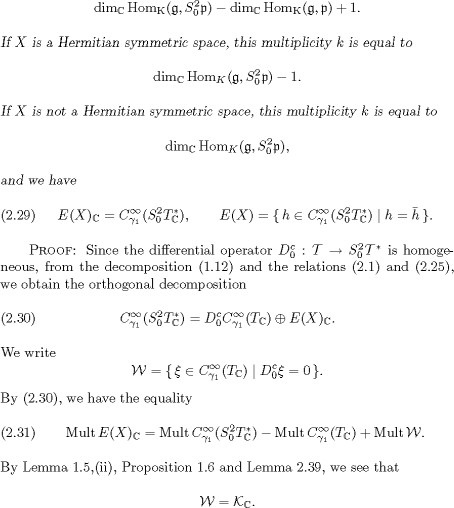
PROPOSITION 2.40. Let (X, g) be an irreducible symmetric space of compact type, which is not equal to a simple Lie group or
to the sphere S2. Then the space E(X) of infinitesimal Einstein deformations of X is a Gmodule isomorphic to the direct sum
of k copies of the irreducible G-module ![]() and its multiplicity k is equal to
and its multiplicity k is equal to
Hence the equality (2.31) becomes
![]()
Since Mult![]() is equal to one, the preceding equality together with the Frobenius reciprocity theorem gives us the first assertion of the
proposition. The other assertions of the proposition then follow from the second equalities of (2.20) and (2.21).
is equal to one, the preceding equality together with the Frobenius reciprocity theorem gives us the first assertion of the
proposition. The other assertions of the proposition then follow from the second equalities of (2.20) and (2.21).
In [42], Koiso also showed that the assertions of the previous proposition also hold when the irreducible space X is a simple Lie group. The following lemma is stated without proof by Koiso (see Lemma 5.5 of [42]); for the irreducible symmetric spaces
![]()
with p, q 2, we shall verify the results of this lemma in Chapter IV (see Lemma 4.1), Chapter V (see Lemma 5.15) and ![]() 3, Chapter VIII.
3, Chapter VIII.
LEMMA 2.41. Let (X, g) be a simply-connected irreducible symmetric space of compact type which is not equal to a simple Lie
group. If X is Hermitian, then the space Hom ![]() , is one-dimensional and if X is not Hermitian the space Hom
, is one-dimensional and if X is not Hermitian the space Hom![]() , vanishes, unless X is one of the spaces appearing in the following table which gives the dimension of the space Hom
, vanishes, unless X is one of the spaces appearing in the following table which gives the dimension of the space Hom![]() ,
,
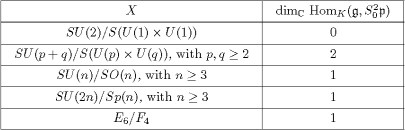
The first two spaces X of this table are Hermitian, while the last three are not Hermitian.
Since the space ![]() is isometric to the sphere S
is isometric to the sphere S
2, by Lemma 2.41 and the equalities (2.25) we know that the space E(X) vanishes when X is the sphere S2; we also proved this result directly in 3, Chapter I. Therefore from Proposition 2.40 and Lemma 2.41, we obtain the results of Theorem 1.22 when the space X of this theorem is not equal to a simple Lie group; moreover, when X is equal to one of the last four spaces of the table of Lemma 2.41, we see that the G-module E(X) is isomorphic to g0.
Thus according to Proposition 2.40 and Lemma 2.41, if X is an irreducible symmetric space of compact type, which is not equal
to a simple Lie group, the space E(X) either vanishes or is isomorphic to the G-module ![]() .
.
From the relations (2.17), (2.18) and (2.26), we obtain the following result:
PROPOSITION 2.42. Let (X, g) be an irreducible symmetric space of compact type, which is not equal to a simple Lie group,
and let ![]() be the element of
be the element of![]() which is the equivalence class of the irreducible G-module
which is the equivalence class of the irreducible G-module ![]() . Let be a finite set of isometries of X which commute with the action of G on X and let
. Let be a finite set of isometries of X which commute with the action of G on X and let![]() be a real number equal to ±1.
be a real number equal to ±1.
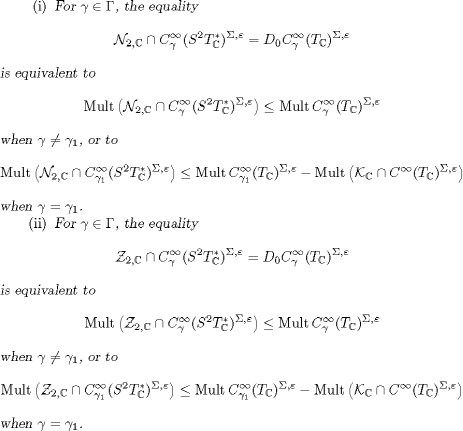
Let d be the integer which is equal to 1 when X is a Hermitian symmetric space and equal to 0 otherwise. According to (2.27)
and (2.28), we know that the multiplicity of the G-module ![]() is equal to d+1. Since its G-submodule
is equal to d+1. Since its G-submodule ![]() is isomorphic to
is isomorphic to ![]() from Propositions 2.31 and 2.42 we deduce the following criteria for Guillemin rigidity and infinitesimal rigidity:
from Propositions 2.31 and 2.42 we deduce the following criteria for Guillemin rigidity and infinitesimal rigidity:
PROPOSITION 2.43. Let (X, g) be an irreducible symmetric space of compact type, which is not equal to a simple Lie group,
and let ![]() be the element of
be the element of ![]() which is the equivalence class of the irreducible G-module
which is the equivalence class of the irreducible G-module ![]() .
.
(i) If the inequality
![]()
holds for a![]() ll , with
ll , with ![]() = 1, and if the inequality
= 1, and if the inequality

We choose a Cartan subalgebra of g and fix a system of positive roots of![]() If W is a G-submodule of
If W is a G-submodule of ![]() wit
wit![]() the dimension of its weight subspace, corresponding to the highest weight of
the dimension of its weight subspace, corresponding to the highest weight of ![]() is equal to the multiplicity of W. Thus according to Proposition 2.43,(i), to prove the Guillemin rigidity of X, it suffices
to successively carry out the following steps:
is equal to the multiplicity of W. Thus according to Proposition 2.43,(i), to prove the Guillemin rigidity of X, it suffices
to successively carry out the following steps:

According to Proposition 2.43,(ii), to prove the infinitesimal rigidity of X, it suffices to carry out the steps (i) and (ii) given above and then the following step:
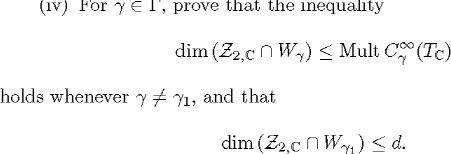
These methods for proving the rigidity of an irreducible symmetric space of compact type implement the criteria described
at the end of ![]() 5. They were first used in [14] to show that the complex projective space is infinitesimally rigid (se
5. They were first used in [14] to show that the complex projective space is infinitesimally rigid (se![]() e 5, Chapter III).
e 5, Chapter III).
8. Criteria for the rigidity of an irreducible symmetric space
We consider the symmetric space (X, g) of compact type of![]() 4 and 7 and continue to view X as the homogeneous space G/K. We recall that a closed connected totally geodesic submanifold
Y of X is a symmetric space; moreover, if x is a point of Y and the tangent space to Y at x is equal to the subspace V of
Tx, then the submanifold Y is equal to the subset ExpxV of X (see
4 and 7 and continue to view X as the homogeneous space G/K. We recall that a closed connected totally geodesic submanifold
Y of X is a symmetric space; moreover, if x is a point of Y and the tangent space to Y at x is equal to the subspace V of
Tx, then the submanifold Y is equal to the subset ExpxV of X (see![]() 7 in Chapter IV of [36]).
7 in Chapter IV of [36]).
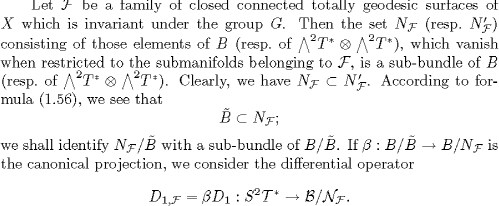
Let ![]() be a family of closed connected totally geodesic submanifolds of X. We denote by
be a family of closed connected totally geodesic submanifolds of X. We denote by ![]() the subspace of
the subspace of ![]() consisting of all symmetric 2-forms h which satisfy the following condition: for all subman-ifolds
consisting of all symmetric 2-forms h which satisfy the following condition: for all subman-ifolds ![]() the restriction of h to Z is a Lie derivative of the metric of Z induced by g. By Lemma 1.1, we know that
the restriction of h to Z is a Lie derivative of the metric of Z induced by g. By Lemma 1.1, we know that ![]() is a subspace of
is a subspace of ![]() We consider the following properties which the family
We consider the following properties which the family ![]() Fmight possess:
Fmight possess:
(I) If a section of ![]() Sover X satisfies the Guillemin condition, then its restriction to an arbitrary submanifold of X belonging to the family
Sover X satisfies the Guillemin condition, then its restriction to an arbitrary submanifold of X belonging to the family ![]() satisfies the Guillemin condition.
satisfies the Guillemin condition.
(II) Every submanifold of X belonging to Fis rigid in the sense of Guillemin.
(III) Every submanifold of X belonging to ![]() is infinitesimally rigid.
is infinitesimally rigid.
If the family ![]() possesses properties (I) and (II), then we see that
possesses properties (I) and (II), then we see that
![]()
On the other hand, the restriction of an element of ![]() to an arbitrary sub-manifold of X belonging to the family
to an arbitrary sub-manifold of X belonging to the family ![]() satisfies the zero-energy condition; hence if the family
satisfies the zero-energy condition; hence if the family ![]() possesses property (III), we have the inclusion
possesses property (III), we have the inclusion
![]()
Z2 From Lemma 1.16, we obtain:
PROPOSITION 2.44. Let (X, g) be a symmetric space of compact type. Let F be a family of closed connected totally geodesic
surfaces of X which is invariant under the group G, and let ![]() be a family of closed connected totally geodesic submanifolds of X. Assume that each surface of X belonging to F is contained
in a submanifold of X belonging to
be a family of closed connected totally geodesic submanifolds of X. Assume that each surface of X belonging to F is contained
in a submanifold of X belonging to ![]() F. A symmetric 2-form h on X belonging to
F. A symmetric 2-form h on X belonging to ![]() satisfies the relation
satisfies the relation ![]()
PROPOSITION 2.45. Let (X, g) be a symmetric space of compact type. Let F be a G-invariant family of closed connected totally
geodesic surfaces of X with positive constant curvature. Let h be an element of ![]() Then the following assertions are equivalent:
Then the following assertions are equivalent:
(i) The symmetric 2-form h belongs to ![]()
(iii) The symmetric 2-form h satisfies ![]()
PROOF: By Lemma 1.15, we know that assertion (i) implies (ii). Now suppose that assertion (ii) holds. Let Y be a totally geodesic
submani-fold of X belonging to the family F and let ![]() be the natural imbedding. Then we have
be the natural imbedding. Then we have ![]() 0. If
0. If ![]() is the Riemannian metric on Y induced by g, by Proposition 1.14,(i) the restriction
is the Riemannian metric on Y induced by g, by Proposition 1.14,(i) the restriction ![]() of h to the manifold Y satisfies
of h to the manifold Y satisfies ![]() Theorem 1.18 gives us the exactness of the sequence (1.51) corresponding to the Riemannian manifold (Y, gY ) with positive
constant curvature; therefore the form
Theorem 1.18 gives us the exactness of the sequence (1.51) corresponding to the Riemannian manifold (Y, gY ) with positive
constant curvature; therefore the form ![]() on Y is a Lie derivative of the metric
on Y is a Lie derivative of the metric ![]() . Thus we know that h belongs to
. Thus we know that h belongs to ![]() and so assertion (ii) implies (i). Since
and so assertion (ii) implies (i). Since ![]() the equivalence of assertions (ii) and (iii) is a consequence of Proposition 1.14,(ii).
the equivalence of assertions (ii) and (iii) is a consequence of Proposition 1.14,(ii).
PROPOSITION 2.46. Let (X, g) be a symmetric space of compact type. Let Fbe a family of closed connected totally geodesic submanifolds of X.
![]() (i) Suppose that each closed geodesic of X is contained in a subman-ifold of X belonging to the family
(i) Suppose that each closed geodesic of X is contained in a subman-ifold of X belonging to the family ![]() Then we have the inclusion
Then we have the inclusion
![]()
(ii) Suppose that the sequence (1.24), corresponding to an arbitrary submanifold of X belonging to the family ![]() is exact. Let h be an element of C(S2T) satisfying the relation D1h = 0. Then h belongs to
is exact. Let h be an element of C(S2T) satisfying the relation D1h = 0. Then h belongs to ![]()
![]() (iii) Suppose that the hypothesis of (i) and of (ii) hold, and that the space X is infinitesimally rigid. Then the sequence
(1.24) is exact.
(iii) Suppose that the hypothesis of (i) and of (ii) hold, and that the space X is infinitesimally rigid. Then the sequence
(1.24) is exact.
PROOF: Let h be an element of ![]() C(S2T). First, suppose that h belongs to
C(S2T). First, suppose that h belongs to ![]() and that the hypothesis of (i) holds. Let be an arbitrary closed geodesic of X; then there is a submanifold Y of X belonging
to the family
and that the hypothesis of (i) holds. Let be an arbitrary closed geodesic of X; then there is a submanifold Y of X belonging
to the family ![]() containing
containing![]() . Let
. Let ![]() be the natural inclusion. Since the symmetric 2-form
be the natural inclusion. Since the symmetric 2-form ![]() on Y is a Lie derivative of the metric of Y , the integral of h over
on Y is a Lie derivative of the metric of Y , the integral of h over![]() vanishes; thus the symmetric 2-form h satisfies the zero-energy condition and assertion (i) holds. Next, let Y be an arbitrary
submanifold of X belonging to the family
vanishes; thus the symmetric 2-form h satisfies the zero-energy condition and assertion (i) holds. Next, let Y be an arbitrary
submanifold of X belonging to the family ![]() and let
and let ![]() be the natural inclusion. If D1,Y is the differential operator on the symmetric space Y defined in
be the natural inclusion. If D1,Y is the differential operator on the symmetric space Y defined in![]() 1, Chapter I, according to formula (1.58) of Proposition 1.14 the relation D1h = 0 implies that
1, Chapter I, according to formula (1.58) of Proposition 1.14 the relation D1h = 0 implies that ![]() and if the sequence (1.24) for Y is exact, it follows that
and if the sequence (1.24) for Y is exact, it follows that ![]() is a Lie derivative of the metric of Y . Thus assertion (ii) is true. Finally, assertion (iii) is a direct consequence of
(i) and (ii).
is a Lie derivative of the metric of Y . Thus assertion (ii) is true. Finally, assertion (iii) is a direct consequence of
(i) and (ii).
THEOREM 2.47. Let (X, g) be a symmetric space of compact type. Let F be a family of closed connected totally geodesic surfaces
of X which is invariant under the group G, and let ![]() Fbe a family of closed connected totally geodesic submanifolds of X. Assume that each surface of X belonging to F is contained
in a submanifold of X belonging to
Fbe a family of closed connected totally geodesic submanifolds of X. Assume that each surface of X belonging to F is contained
in a submanifold of X belonging to ![]() Suppose that the relation (1.48) and the equality
Suppose that the relation (1.48) and the equality
![]()
hold.
(i) A symmetric 2-form h on X belonging to ![]() is a Lie derivative of the metric g.
is a Lie derivative of the metric g.
(ii) If the family ![]() possesses properties (I) and (II), then the symmetric space X is rigid in the sense of Guillemin.
possesses properties (I) and (II), then the symmetric space X is rigid in the sense of Guillemin.
(iii) If the family ![]() possesses property (III), then the symmetric space X is infinitesimally rigid.
possesses property (III), then the symmetric space X is infinitesimally rigid.
PROOF: First, let h be a symmetric 2-form h on X belonging to ![]() By Proposition 2.44, we see that
By Proposition 2.44, we see that ![]() According to the equality (2.32), we therefore know that D1h = 0. By the relation (1.48) and Theorem 1.18, the sequence (1.24)
is exact, and so we see that h is a Lie derivative of the metric g. Thus we have proved assertion (i). Now assume that the
family
According to the equality (2.32), we therefore know that D1h = 0. By the relation (1.48) and Theorem 1.18, the sequence (1.24)
is exact, and so we see that h is a Lie derivative of the metric g. Thus we have proved assertion (i). Now assume that the
family ![]() satisfies the hypothesis of (i) (resp. of (ii)). Then we know that the space N2 (resp. the space
satisfies the hypothesis of (i) (resp. of (ii)). Then we know that the space N2 (resp. the space ![]() is contained in
is contained in ![]() Assertion (ii) (resp. (iii)) is a consequence of (i).
Assertion (ii) (resp. (iii)) is a consequence of (i).
We now assume that (X, g) is an irreducible symmetric space of compact type; then we have Ric ![]() where is a positive real number.
where is a positive real number.
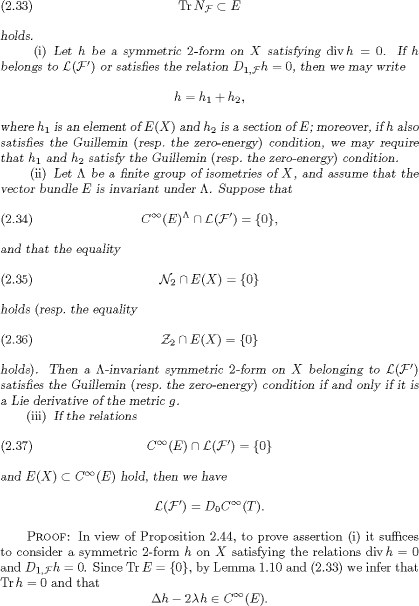
THEOREM 2.48. Let (X, g) be an irreducible symmetric space of compact type. Let F be a family of closed connected totally
geodesic surfaces of X which is invariant under the group G, and let ![]() be a family of closed connected totally geodesic submanifolds of X. Let E be a G-invariant sub-bundle 0T. Assume that each
surface of X belonging to F is contained of
be a family of closed connected totally geodesic submanifolds of X. Let E be a G-invariant sub-bundle 0T. Assume that each
surface of X belonging to F is contained of ![]() in a submanifold of X belonging to
in a submanifold of X belonging to ![]() and suppose that the relation
and suppose that the relation
Assertion (i) is now a consequence of Proposition 2.37,(ii), with ![]() Next, let k be a symmetric 2-form on X belonging to
Next, let k be a symmetric 2-form on X belonging to ![]() According to the decomposition (1.11), we may write k as
According to the decomposition (1.11), we may write k as
![]()
where h is an element of ![]() satisfying div h = 0, which is uniquely determined by k, and where
satisfying div h = 0, which is uniquely determined by k, and where ![]() is a vector field on X. If k is invariant under a finite grou
is a vector field on X. If k is invariant under a finite grou![]() p of isometries of X, clearly h is also
p of isometries of X, clearly h is also ![]() -invariant. Since
-invariant. Since ![]() is an element of
is an element of ![]() the 2-form h also belongs to
the 2-form h also belongs to ![]() According to (i), we may write h = h1 + h2, where h1 is an element of E(X) and h2 is a section of E. If k satisfies the Guillemin
(resp. the zero-energy) condition, according to Lemma 2.10 (resp. Lemma 2.6) so does h, and we may suppose that h1 also satisfies
the Guillemin (resp. the zero-energy) condition. First, if
According to (i), we may write h = h1 + h2, where h1 is an element of E(X) and h2 is a section of E. If k satisfies the Guillemin
(resp. the zero-energy) condition, according to Lemma 2.10 (resp. Lemma 2.6) so does h, and we may suppose that h1 also satisfies
the Guillemin (resp. the zero-energy) condition. First, if ![]() then h1 and h are also sections of E; if moreover the equality (2.37) holds, then h vanishes and so k is equal to
then h1 and h are also sections of E; if moreover the equality (2.37) holds, then h vanishes and so k is equal to ![]() Next, under the hypotheses of (ii), if k is -invariant and satisfies the Guillemin (resp. the zero-energy) condition and
if the equality (2.35) (resp. the equality (2.36)) holds, then h is a -invariant section of E; according to (2.34), we infer
that h vanishes, and so k is equal to
Next, under the hypotheses of (ii), if k is -invariant and satisfies the Guillemin (resp. the zero-energy) condition and
if the equality (2.35) (resp. the equality (2.36)) holds, then h is a -invariant section of E; according to (2.34), we infer
that h vanishes, and so k is equal to ![]() We have thus verified both assertions (ii) and (iii).
We have thus verified both assertions (ii) and (iii).
Since the differential operator ![]() corresponding to the family F of Theorem 2.48 is homogeneous, according to the proof of Proposition 2.37 the sections h1
and h2 given by Theorem 2.48,(i) satisfy the relations
corresponding to the family F of Theorem 2.48 is homogeneous, according to the proof of Proposition 2.37 the sections h1
and h2 given by Theorem 2.48,(i) satisfy the relations
![]()
The following theorem gives criteria for the Guillemin rigidity or the infinitesimal rigidity of an irreducible symmetric space of compact type.
Theorem 2.49. Let (X, g) be an irreducible symmetric space of compact type. Let F be a family of closed connected totally
geodesic surfaces of X which is invariant under the group G, and let ![]() be a family of closed connected totally geodesic submanifolds of X. Let E be a G-invariant sub-bundle of
be a family of closed connected totally geodesic submanifolds of X. Let E be a G-invariant sub-bundle of ![]() Assume that each surface of X belonging to F is contained in a submanifold of X belonging to
Assume that each surface of X belonging to F is contained in a submanifold of X belonging to ![]() and suppose that the relations (2.33) and (2.37) hold.
and suppose that the relations (2.33) and (2.37) hold.
(i) If the family ![]() possesses properties (I) and (II) and if the equality (2.35) holds, then the symmetric space X is rigid in the sense of Guillemin.
possesses properties (I) and (II) and if the equality (2.35) holds, then the symmetric space X is rigid in the sense of Guillemin.
(ii) If the family ![]() possesses property (III) and if the equality (2.36) holds, then the symmetric space X is infinitesimally rigid.
possesses property (III) and if the equality (2.36) holds, then the symmetric space X is infinitesimally rigid.
PROOF: Under the hypotheses of (i) (resp. of (ii)), a symmetric 2-form h on X satisfying the Guillemin (resp. the zero-energy)
condition belongs to ![]() by Theorem 2.48,(ii), with = {id}, we see that h is a Lie derivative of the metric g.
by Theorem 2.48,(ii), with = {id}, we see that h is a Lie derivative of the metric g.
According to Proposition 2.13, we know that the equality (2.35) (resp. the equality (2.36)) is a necessary condition for the Guillemin rigidity (resp. the infinitesimal rigidity) of X.
If we take E = {0} in Theorem 2.49, we obtain the following corollary of Theorem 2.49:
THEOREM 2.50. Let (X, g) be an irreducible symmetric space of compact type. Let F be a family of closed connected totally
geodesic surfaces of X which is invariant under the group G, and let ![]() be a family of closed connected totally geodesic submanifolds of X. Assume that each surface of X belonging to F is contained
in a submanifold of X belonging to
be a family of closed connected totally geodesic submanifolds of X. Assume that each surface of X belonging to F is contained
in a submanifold of X belonging to ![]() F. Suppose that the equality
F. Suppose that the equality
![]()
holds. Then assertions (i) and (ii) of Theorem 2.49 hold.
Thus according to Theorem 2.50, when X is an irreducible space, in Theorem 2.47 in order to obtain assertion (ii) (resp. assertion (iii)) of the latter theorem we may replace the hypothesis that the relation (1.48) holds by the hypothesis that the equality (2.35) (resp. the equality (2.36)) holds.
We again assume that X is an arbitrary symmetric space of compact type. We consider the following properties which the family
![]() might possess:
might possess:
(IV) If a one-form over X satisfies the Guillemin condition, then its restriction to an arbitrary submanifold of X belonging
to the family ![]() satisfies the Guillemin condition.
satisfies the Guillemin condition.
(V) If Y is an arbitrary submanifold of X belonging to the family ![]() every form of degree one on Y satisfying the Guillemin is exact.
every form of degree one on Y satisfying the Guillemin is exact.
(VI) If Y is an arbitrary submanifold of X belonging to the family ![]() every form of degree one on Y satisfying the zero-energy is exact.
every form of degree one on Y satisfying the zero-energy is exact.
We consider the subset ![]() of 2Tconsisting of those elements of 2
of 2Tconsisting of those elements of 2![]() which vanish when restricted
which vanish when restricted 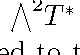 to the submanifolds belonging to the family
to the submanifolds belonging to the family ![]() if the family
if the family ![]() is invariant under the group G, then
is invariant under the group G, then ![]() is a sub-bundle of 2
is a sub-bundle of 2![]()
THEOREM 2.51. Let (X, g) be a symmetric space of compact type. Let F and ![]() be two families of closed connected totally geodesic submanifolds of X. Assume that each submanifold of X belonging to F
is contained in a submanifold of X belonging to
be two families of closed connected totally geodesic submanifolds of X. Assume that each submanifold of X belonging to F
is contained in a submanifold of X belonging to ![]() , and suppose that
, and suppose that
![]()
(i) If the family ![]() possesses properties (IV) and (V), then a differential form of degree one on X satisfies the Guillemin condition if and only
if it is exact.
possesses properties (IV) and (V), then a differential form of degree one on X satisfies the Guillemin condition if and only
if it is exact.
(ii) If the family ![]() possesses property (VI), then a differential form of degree one on X satisfies the zero-energy condition if and only if it
is exact.
possesses property (VI), then a differential form of degree one on X satisfies the zero-energy condition if and only if it
is exact.
PROOF: Suppose that the family ![]() possesses properties (IV) and (V) (resp. possesses property (VI)). L
possesses properties (IV) and (V) (resp. possesses property (VI)). L![]() be a 1-form on X satisfying the Guillemin (resp. the zero-energy) condition. Consider a submanifold Y of X belonging to the
family
be a 1-form on X satisfying the Guillemin (resp. the zero-energy) condition. Consider a submanifold Y of X belonging to the
family ![]() According to our hypotheses, the restriction of
According to our hypotheses, the restriction of![]() to Y satisfies the Guillemin (resp. the zero-energy) condition; it follows that the 1-form
to Y satisfies the Guillemin (resp. the zero-energy) condition; it follows that the 1-form ![]() on Y is closed. Hence the restriction of the 2-form
on Y is closed. Hence the restriction of the 2-form ![]() to Y vanishes, and so the restriction of
to Y vanishes, and so the restriction of ![]() to an arbitrary submanifold of X belonging to the family F vanishes. From the relation (2.38), we infer that
to an arbitrary submanifold of X belonging to the family F vanishes. From the relation (2.38), we infer that![]() is closed. Since the cohomology group
is closed. Since the cohomology group ![]() vanishes, the form is exact.
vanishes, the form is exact.
