4 Applications of Trigonometry

In This Chapter

4.1 Solving Right Triangles

4.2 Applications of Right Triangles

4.3 Law of Sines

4.4 Law of Cosines

4.5 Simple Harmonic Motion

     CHAPTER 4 Review Exercises

The wine rack shown is constructed from right triangles.

A Bit of History Trigonometry was developed from early efforts to advance the study of astronomy by predicting the paths and positions of the heavenly bodies and to improve accuracy in navigation and the reckoning of time and calendars.

Much of the mathematics developed in the eighteenth century arose in an attempt to describe certain physical phenomena. For example, what is the shape of a sail under the pressure of the wind? What is the shape of a vibrating elastic string (for example, a violin string or guitar string) fixed at both ends? Answers to such questions frequently involve the trigonometric functions.

Ernst Florens Chladni (1756-1827), a German physicist, applied mathematician, and musician developed an interesting technique for studying the vibration of elastic plates. After sprinkling fine sand on drumlike surfaces, he drew a violin bow along the edges to start them vibrating. The French Academy of Sciences offered a prize for the best mathematical description of this phenomenon. In 1816 Marie-Sophie Germain (1776-1831), a largely self-taught French mathematician, won the Paris Academy's prize with her paper on elasticity.

In this chapter we put to use the trigonometric functions developed in the last two chapters to solve various kinds of mathematical and applied problems.

4.1 Solving Right Triangles

Introduction The expression “to solve a triangle” means that we wish to find the length of each side and the measure of each angle in the triangle. We can solve any right triangle if we know either two sides or one acute angle and one side. As the examples in this section will show, sketching and labeling the triangle is an essential part of the solution process. It will be our general practice to label a right triangle as shown in FIGURE 4.1.1. The three vertices will be denoted by A, B, and C with C at the vertex of the right angle. We denote the angles at A and B by the Greek letters α and β (alpha and beta) and the lengths of the sides opposite these angles by a and b, respectively. The length of the side opposite the right angle at the vertex C is denoted by c.

Applications of right triangle trigonometry in fields such as surveying and navigation involve solving right triangles. We will consider these applications in the next section.

FIGURE 4.1.1 Standard Labeling for a right triangle

EXAMPLE 1 Solving a Right Triangle

Solve the right triangle having a hypotenuse of length 4 √3 and one 60° angle.

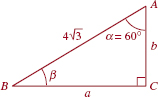
FIGURE 4.1.2 Right triangle in Example 1

Solution First we make a sketch of the triangle and label it as shown in FIGURE 4.1.2. We wish to find a, b, and β. Since α and β are complementary angles, α + β = 90° yields

β = 90° – α = 90° – 60° = 30°.

We are given the length of the hypotenuse, namely hyp = 4 √3. To find a, the length of the side opposite the angle α = 60°, we select the sine function. From sin α = opp/hyp, we obtain

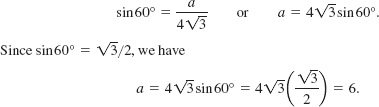
Finally, for the length b of the side adjacent to the 60° angle, we select the cosine function. From cos α = adj/hyp, we obtain

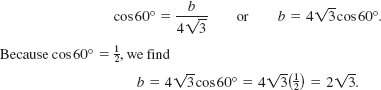
In Example 1 once we determined a, we could have found b by using either the Pythagorean theorem or the tangent function. In general, there are usually several ways to solve a triangle.

Some calculators have a key labeled stor or sto.

Use of a Calculator If angles other than 30°, 45°, or 60° are involved in a problem, we obtain approximations of the desired trigonometric function values from a calculator. For the remainder of this chapter, whenever an approximation is used, we will round the final results to the nearest hundredth unless the problem specifies otherwise. To take full advantage of the calculator's accuracy, store the computed values of the trigonometric functions in the calculator for subsequent calculations. If, instead, a rounded version of a displayed value is written down and then later keyed back into the calculator, the accuracy of the final result may be diminished.

In many applications we are able to determine the value of one of the trigonometric functions, say sin θ, and we wish to find θ. We can do so by using the inverse trigonometric functions and a calculator. Recall, the inverse trigonometric functions are denoted by sin^-1 or arcsin, cos^-1 or arccos, tan^-1 or arctan, and so on. Scientific calculators have keys labeled (Read the manual for your calculator if you need more explanation.)

On HP scientific calculators the notation asin, acos, and atan is used.

EXAMPLE 2 Find θ

Use a calculator to find an acute angle θ measured in (a) degrees and (b) radians for which cos θ = 0.5.

Solution (a) We must first set the calculator in degree mode. We enter 0.5 and then press the appropriate key. The resulting display is 60. Thus, . (b) With the calculator set in radian mode, we enter 0.5 and then press the same key; the display reads 1.0471976. Thus for cos θ = 0.5, . Note that 1.0471976 is a decimal approximation of π/3.

EXAMPLE 3 Solving a Right Triangle

Solve the right triangle with legs of length 4 and 5.

Solution After sketching and labeling the triangle as shown in FIGURE 4.1.3, we see that we need to find c, α, and β. From the Pythagorean theorem, the hypotenuse c is given by

To find β, we will use tan β = opp/adj. (By choosing to work with the given quantities, we avoid error due to previous approximations.) Thus we have

The inverse tangent key of a calculator set in degree mode yields . Since α and β are complementary angles, we use α = 90° – β to obtain .

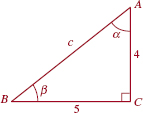
FIGURE 4.1.3 Right triangle in Example 3

4.1 Exercises Answers to selected odd-numbered problems begin on page ANS-12.

In Problems 1-12, find the indicated unknowns. Each problem refers to the triangle shown in FIGURE 4.1.4.

1 a = 4, β = 27°; b, c

2 c = 10, β = 49°; a, b

3 b = 8, β = 34.33°; a, c

4 c = 25, α = 50°; a, b

5 b = 1.5, c = 3; α, β, a

6 a = 5, b = 2; α, β, c

7 a = 4, b = 10; α, β, c

8 b = 4, α = 58°; a, c

9 a = 9, c = 12; α, β, b

10 b = 3, c = 6; α, β, a

11 b = 20, α = 23°; a, c

12 a = 11, α = 33.5°; b, c

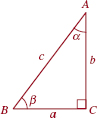
FIGURE 4.1.4 Triangle for Problems 1-12

For Discussion

(a) A regular n-gon is an n-sided polygon inscribed in a circle; the polygon is formed by n equally spaced points on the circle. Suppose the polygon shown in FIGURE 4.1.5 represents a regular n-gon inscribed in a circle of radius r. Use right triangle trigonometry to show that the area A(n) of the n-gon is given by

(b) Compute A₁₀₀, A₅₀₀, and A₁₀₀₀.

(c) Discuss: Is A(n) approaching a number as n → ∞?

FIGURE 4.1.5 Inscribed n-gon for Problem 13

4.2 Applications of Right Triangles

Introduction Right triangle trigonometry can be used to solve many practical problems, particularly those involving lengths, heights, and distances.

FIGURE 4.2.1 Tree in Example 1

EXAMPLE 1 Finding the Height of a Tree

A kite is caught in the top branches of a tree. If the 90-ft kite string makes an angle of 22° with the ground, estimate the height of the tree by finding the distance from the kite to the ground.

Solution Let h denote the height of the kite. From FIGURE 4.2.1 we see that

A calculator set in degree mode gives h ≈ 33.71 ft.

EXAMPLE 2 Length of a Saw Cut

A carpenter cuts the end of a 4-in.-wide board on a 25° bevel from the vertical, starting at a point in. from the end of the board. Find the lengths of the diagonal cut and the remaining side. See FIGURE 4.2.2.

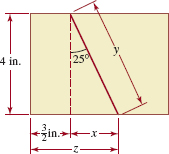
FIGURE 4.2.2 Saw cut in Example 2

Solution Let x, y, and z be the (unknown) dimensions, as labeled in FIGURE 4.2.2. It follows from the definition of the tangent function that

To find y we observe that

Since

Angles of Elevation and Depression The angle between an observer's line of sight to an object and the horizontal is given a special name. As FIGURE 4.2.3 illustrates, if the line of sight is to an object above the horizontal, the angle is called an angle of elevation, whereas if the line of sight is to an object below the horizontal, the angle is called an angle of depression.

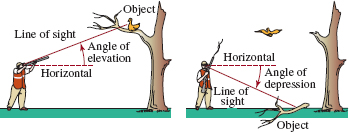
FIGURE 4.2.3 Angles of elevation and depression

EXAMPLE 3 Using Angles of Elevation

A surveyor uses an instrument called a theodolite to measure the angle of elevation between ground level and the top of a mountain. At one point the angle of elevation is measured to be 41°. A half kilometer farther from the base of the mountain, the angle of elevation is measured to be 37°. How high is the mountain?

Solution Let h represent the height of the mountain. FIGURE 4.2.4 shows that there are two right triangles sharing the common side h, so we obtain two equations in two unknowns z and h:

We can solve each of these for h, obtaining, respectively,

Equating the last two results gives an equation from which we can determine the distance z:

Solving for z gives us

Using h = z tan 41° we find the height h of the mountain to be

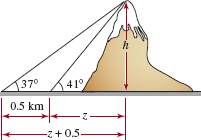
FIGURE 4.2.4 Mountain in Example 3

EXAMPLE 4 Glide Path

Most airplanes approach San Francisco International Airport (SFO) on a straight 3° glide path starting at a point 5.5 miles from the field. A few years ago, the FAA experimented with a computerized two-segment approach where a plane approaches the field on a 6° glide path starting at a point 5.5 mi out and then switches to a 3° glide path 1.5 mi from the point of touchdown. The point of this experimental approach was to reduce the noise of the planes over the outlying residential areas. Compare the height of a plane P' using the standard 3° approach with the height of a plane P using the experimental approach when both planes are 5.5 mi from the airport.

Solution For purposes of illustration, the angles and distances shown in FIGURE 4.2.5 are exaggerated.

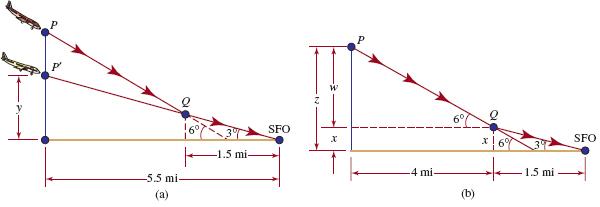
FIGURE 4.2.5 Glide paths in Example 4

First, suppose y is the height of plane P' on the standard approach when it is 5.5 mi out from the airport. As we see in FIGURE 4.2.5(a),

Because distances from the airport are measured in miles, we convert y to feet

Now, suppose z is the height of plane P on the experimental approach when it is 5.5 mi out from the airport. As shown in FIGURE 4.2.5(b), z = x + w, so we use two right triangles to obtain

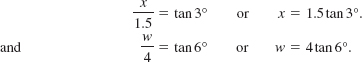
Hence the approximate height of plane P at a point 5.5 mi out from the airport is

In other words, plane P is approximately 1113 ft higher than plane P'.

Words Into Functions Section 1.10 was devoted to setting up or constructing functions that were described or expressed in words. As emphasized in that section, this is a task that you will surely face in a course in calculus. Our final example illustrates a recommended procedure of sketching a figure and labeling quantities of interest with appropriate variables.

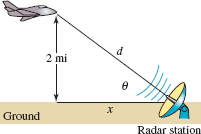
FIGURE 4.2.6 Plane in Example 5

EXAMPLE 5 Functions That Involve Trigonometry

A plane flying horizontally at an altitude of 2 miles approaches a radar station as shown in FIGURE 4.2.6.

(a) Express the distance d between the plane and the radar station as a function of the angle of elevation θ.

(b) Express the angle of elevation θ of the plane as a function of the horizontal separation x between the plane and the radar station.

Solution As shown in FIGURE 4.2.6, θ is an acute angle in a right triangle.

(a) We can relate the distance d and the angle θ by sin θ = 2/d. Solving for d gives

where 0 < θ ≤ 90°

(b) The horizontal separation x and θ are related by tan θ = 2/x. We make use of the inverse tangent function to solve for θ:

where 0 < x < ∞

4.2 Exercises Answers to selected odd-numbered problems begin on page ANS-12.

1. A building casts a shadow 20 m long. If the angle from the tip of the shadow to a point on top of the building is 69°, how high is the building?

2. Two trees are on opposite sides of a river, as shown in FIGURE 4.2.7. A baseline of 100 ft is measured from tree T₁, and from that position the angle β to T₂ is measured to be 29.7°. If the baseline is perpendicular to the line segment between T₁ and T₂, find the distance between the two trees.

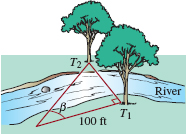
FIGURE 4.2.7 Trees and river in Problem 2

3. A 50-ft tower is located on the edge of a river. The angle of elevation between the opposite bank and the top of the tower is 37°. How wide is the river?

4. A surveyor uses a geodometer to measure the straight-line distance from a point on the ground to a point on top of a mountain. Use the information given in FIGURE 4.2.8 to find the height of the mountain.

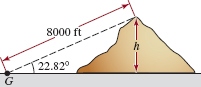
FIGURE 4.2.8 Mountain in Problem 4

5. An observer on the roof of building A measures a 27° angle of depression between the horizontal and the base of building B. The angle of elevation from the same point to the roof of the second building is 41.42°. What is the height of building B if the height of building A is 150 ft? Assume buildings A and B are on the same horizontal plane.

6. Find the height h of a mountain using the information given in FIGURE 4.2.9.

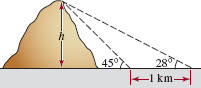
FIGURE 4.2.9 Mountain in Problem 6

7. The top of a 20-ft ladder is leaning against the edge of the roof of a house. If the angle of inclination of the ladder from the horizontal is 51°, what is the approximate height of the house and how far is the bottom of the ladder from the base of the house?

8. An airplane flying horizontally at an altitude of 25,000 ft approaches a radar station located on a 2000-ft-high hill. At one instant in time, the angle between the radar dish pointed at the plane and the horizontal is 57°. What is the straight-line distance in miles between the airplane and the radar station at that particular instant?

9. A 5-mi straight segment of a road climbs a 4000-ft hill. Determine the angle that the road makes with the horizontal.

10. A box has dimensions as shown in FIGURE 4.2.10. Find the length of the diagonal between the corners P and Q. What is the angle θ formed between the diagonal and the bottom edge of the box?

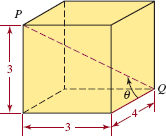
FIGURE 4.2.10 Box in Problem 10

11. Observers in two towns A and B on either side of a 12,000-ft mountain measure the angles of elevation between the ground and the top of the mountain. See FIGURE 4.2.11. Assuming that the towns and the mountaintop lie in the same vertical plane, find the horizontal distance between them.

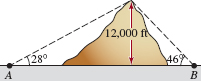
FIGURE 4.2.11 Mountain in Problem 11

12. A drawbridge measures 7.5 m from shore to shore, and when completely open it makes an angle of 43° with the horizontal. See FIGURE 4.2.12(a). When the bridge is closed, the angle of depression from the shore to a point on the surface of the water below the opposite end is 27°. See FIGURE 4.2.12(b). When the bridge is fully open, what is the distance d between the highest point of the bridge and the water below?

FIGURE 4.2.12 Drawbridge in Problem 12

13. A flagpole is located at the edge of a sheer 50-ft cliff at the bank of a river of width 40 ft. See FIGURE 4.2.13. An observer on the opposite side of the river measures an angle of 9° between her line of sight to the top of the flagpole and her line of sight to the top of the cliff. Find the height of the flagpole.

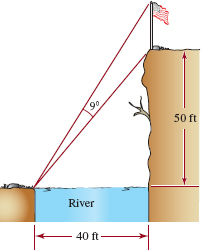
FIGURE 4.2.13 Flagpole in Problem 13

14. From an observation site 1000 ft from the base of Mt. Rushmore the angle of elevation to the top of the sculpted head of George Washington is measured to be 80.05°, whereas the angle of elevation to the bottom of his head is 79.946°. Determine the height of George Washington's head.

15. The length of a Boeing 747 airplane is 231 ft. What is the plane's altitude if it subtends an angle of 2° when it is directly above an observer on the ground? See FIGURE 4.2.14.

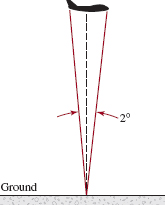
FIGURE 4.2.14 Airplane in Problem 15

16. The height of a gnomon (pin) of a sundial is 4 in. If it casts a 6-in. shadow, what is the angle of elevation of the Sun?

Bust of George Washington on Mt. Rushmore

17. Weather radar is capable of measuring both the angle of elevation to the top of a thunderstorm and its range (the horizontal distance to the storm). If the range of a storm is 90 km and the angle of elevation is 4°, can a passenger plane that is able to climb to 10 km fly over the storm?

Sundial

18. Cloud ceiling is the lowest altitude at which solid cloud is present. The cloud ceiling at airports must be sufficiently high for safe takeoffs and landings. At night the cloud ceiling can be determined by illuminating the base of the clouds with a searchlight pointed vertically upward. If an observer is 1 km from the searchlight and the angle of elevation to the base of the illuminated cloud is 8°, find the cloud ceiling. See FIGURE 4.2.15. (During the day cloud ceilings are generally estimated by sight. However, if an accurate reading is required, a balloon is inflated so that it will rise at a known constant rate. Then it is released and timed until it disappears into the cloud. The cloud ceiling is determined by multiplying the rate by the time of the ascent; trigonometry is not required for this calculation.)

FIGURE 4.2.15 Searchlight in Problem 18

19. Assuming that the Earth is a sphere, show that C_θ = C_e cosθ, where C_θ is the circumference of the parallel of latitude at the latitude angle θ and C_e is the Earth's circumference at the equator. See FIGURE 4.2.16. [Hint: R cosθ = r.]

FIGURE 4.2.16 Earth in Problem 19

20. Use Problem 19 and the fact that the radius R of the Earth is 6400 km to find:

(a) the circumference of the Arctic Circle, which lies at 66°33' N (66.55° N) latitude, and

(b) the distance “around the world” at the 58°40' N (58.67° N) latitude.

21. The distance between the Earth and the Moon varies as the Moon revolves around the Earth. At a particular time the geocentric parallax angle shown in FIGURE 4.2.17 is measured to be 1°. Calculate to the nearest hundred miles the distance between the center of the Earth and the center of the Moon at this instant. Assume that the radius of the Earth is 3963 miles.

FIGURE 4.2.17 Angle in Problem 21

Mt. Etna

22. The final length of a volcanic lava flow seems to decrease as the elevation of the lava vent from which it originates increases. An empirical study of Mt. Etna gives the final lava flow length L in terms of elevation h by the formula

L = 23 – 0.0053h,

where L is measured in kilometers and h is measured in meters. Suppose that a Sicilian village at elevation 750 m is on a 10° slope directly below a lava vent at 2500 m. See FIGURE 4.2.18. According to the formula, how close will the lava flow get to the village?

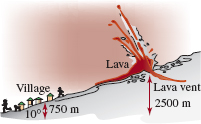
FIGURE 4.2.18 Lava flow in Problem 22

23. As shown in FIGURE 4.2.19, two tracking stations S₁ and S₂ sight a weather balloon between them at elevation angles α and β, respectively. Express the height h of the balloon in terms of α and β, and the distance c between the tracking stations.

FIGURE 4.2.19 Weather balloon in Problem 23

24. An entry in a soapbox derby rolls down a hill. Using the information given in FIGURE 4.2.20, find the total distance d₁ + d₂ that the soapbox travels.

FIGURE 4.2.20 Soapbox in Problem 24

25. Find the height and area of the isosceles trapezoid shown in FIGURE 4.2.21.

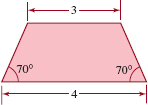
FIGURE 4.2.21 Trapezoid in Problem 25

26. Consider the blue rectangle circumscribed around the red rectangle in FIGURE 4.2.22. With the aid of calculus it can be shown that the area of the blue rectangle is greatest when θ = π/4. Find this area in terms of a and b.

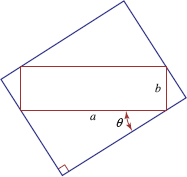
FIGURE 4.2.22 Rectangles in Problem 26

In Problems 27-30, proceed as in Example 5 and translate the words into an appropriate function.

27. A tracking telescope, located 1.25 km from the point of a rocket launch, follows a vertically ascending rocket. Express the height h of the rocket as a function of the angle of elevation θ

28. A searchlight one-half mile offshore illuminates a point P on the shore. Express the distance d from the searchlight to the point of illumination P as a function of the angle θ shown in FIGURE 4.2.23.

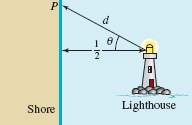
FIGURE 4.2.23 Searchlight in Problem 28

29. A statue is placed on a pedestal as shown in FIGURE 4.2.24. Express the viewing angle θ as a function of the distance x from the pedestal.

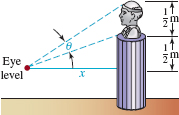
FIGURE 4.2.24 Viewing angle in Problem 29

30. A woman on an island wishes to reach a point R on a straight shore on the mainland from a point P on the island. The point P is 9 mi from the shore and 15 mi from point R. See FIGURE 4.2.25. If the woman rows a boat at a rate of 3 mi/h to a point Q on the mainland, then walks the rest of the way at a rate of 5 mi/h, express the total time it takes the woman to reach point R as a function of the indicated angle θ. [Hint: Distance = rate X time.]

FIGURE 4.2.25 Woman rowing to shore in Problem 30

4.3 Law of Sines

Introduction In Section 4.1 we saw how to solve right triangles. In this and the next section we consider two techniques for solving general triangles.

FIGURE 4.3.1 General triangle

Law of Sines Consider the triangle ABC, shown in FIGURE 4.3.1, with angles α, β, and γ, and corresponding opposite sides BC, AC, and AB. If we know the length of one side and two other parts of the triangle, we can then find the remaining three parts. One way of doing this is by the Law of Sines.

PROOF: Although the Law of Sines is valid for any triangle, we will prove it only for acute triangles—that is, a triangle in which all three angles α, β, and γ, are less than 90°. As shown in FIGURE 4.3.2, let h be the length of the altitude from vertex A to side BC. Since the altitude is perpendicular to the base BC it determines two right triangles. Consequently, we can write

Thus (2) gives

Equating the two expressions in (3) gives c sin β = b sin γ so that

If we use the altitude from the vertex C to the side AB, it follows in the same manner that

Combining (4) and (5) yields the result in (1).

FIGURE 4.3.2 Acute triangle

EXAMPLE 1 Determining the Parts of a Triangle

Find the remaining parts of the triangle shown in FIGURE 4.3.3.

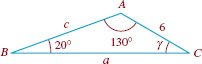
FIGURE 4.3.3 Triangle in Example 1

Solution Let β = 20°, α = 130°, and b = 6. Because the sum of the angles in a triangle is 180° we have γ + 20° + 130 = 180° and so γ = 180° – 20° – 130° = 30°. From (1) we then see that

We use the first equality in (6) to solve for a:

The second equality in (6) gives c:

EXAMPLE 2 Height of a Building

A building is situated on the side of a hill that slopes downward at an angle of 15°. The Sun is uphill from the building at an angle of elevation of 42°. Find the building's height if it casts a shadow 36 ft long.

Solution Denote the height of the building on the downward slope by h and construct a right triangle QPS as shown in FIGURE 4.3.4. Now α + 15° = 42° so that α = 27°. Since QPS is a right triangle, γ + 42° = 90° gives γ = 90° – 42° = 48°. From the Law of Sines (1),

FIGURE 4.3.4 Triangle QPS in Example 2

In Examples 1 and 2, where we were given two angles and a side opposite one of these angles, each triangle had a unique solution. However, this may not always be true for triangles where we know two sides and an angle opposite one of these sides. The next example illustrates the latter situation.

EXAMPLE 3 Two Triangles

Find the remaining parts of the triangle with β = 50°, b = 5, and c = 6.

Solution From the Law of Sines, we have

From a calculator set in degree mode, we obtain γ ≈ 66.82°. At this point it is essential to recall that the sine function is also positive for second quadrant angles. In other words, there is another angle satisfying 0° ≤ γ ≤ 180° for which sin γ ≈ 0.9193. Using 66.82° as a reference angle we find the second quadrant angle to be 180° – 66.82° = 113.18°. Therefore, the two possibilities for γ are and . Thus, as shown in FIGURE 4.3.5, there are two possible triangles ABC₁and ABC₂ satisfying the given three conditions.

FIGURE 4.3.5 Triangles in Example 3

To complete the solution of triangle ABC₁ (FIGURE 4.3.5(a)), we first find α₁ = 180° – γ₁ – β or . To find the side opposite this angle we use

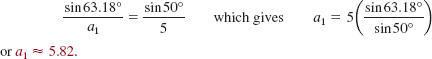
To complete the solution of triangle ABC₂ (FIGURE 4.3.5(b)), we find α₂ = 180° – γ₂ – β or . Then from

Ambiguous Case When solving triangles, the situation where two sides and an angle opposite one of these sides are given is called the ambiguous case. We have just seen in Example 3 that the given information may determine two different triangles. In the ambiguous case other complications can arise. For instance, suppose that the length of sides AB and AC (that is, c and b, respectively) and the angle β in triangle ABC are specified. As shown in FIGURE 4.3.6, we draw the angle β and mark off side AB with length c to locate the vertices A and B. The third vertex C is located on the base by drawing an arc of a circle of radius b (the length of AC) with center A. As shown in FIGURE 4.3.7, there are four possible outcomes of this construction:

FIGURE 4.3.6 Horizontal base, the angle β, and side AB

• The arc does not intersect the base and no triangle is formed.

• The arc intersects the base in two distinct points C₁ and C₂ and two triangles are formed (as in Example 3).

FIGURE 4.3.7 Solution possibilities for the ambiguous case in the Law of Sines

• The arc intersects the base in one point and one triangle is formed.

• The arc is tangent to the base and a single right triangle is formed.

EXAMPLE 4 Determining the Parts of a Triangle

Find the remaining parts of the triangle with β = 40°, b = 5, and c = 9.

Solution From the Law of Sines (1), we have

Since the sine of any angle must be between —1 and 1, sin γ ≈ 1.1570 is impossible. This means the triangle has no solution; the side with length b is not long enough to reach the base. This is the case illustrated in FIGURE 4.3.7(a).

4.3 Exercises

Answers to selected odd-numbered problems begin on page ANS-12.

In Problems 1-16, refer to FIGURE 4.3.1.

In Problems 1—16, use the Law of Sines to solve the triangle.

1. α = 80°, β = 20°, b = 7

2. α = 60°, β = 15°, c = 30

3. β = 37°, γ = 51°, a = 5

4. α = 30°, γ = 75°, a = 6

5. β = 72°, b = 12, c = 6

6. α = 120°, a = 9, c = 4

7. γ = 62°, b = 7, c = 4

8. β = 110°, γ = 25°, a = 14

9. γ = 15°, a = 8, c = 5

10. α = 55°, a = 20, c = 18

11. γ = 150°, b = 7, c = 5

12. α = 35°, a = 9, b = 12

13. β = 30°, a = 10, b = 7

14. α = 140°, γ = 20°, c = 12

15. α = 20°, a = 8, c = 27

16. α = 75°, γ = 45°, b = 8

Miscellaneous Applications

17. Length of a Pool A 10-ft rope that is available to measure the length between two points A and B at opposite ends of a kidney-shaped swimming pool is not long enough. A third point C is found such that the distance from A to C is 10 ft. It is determined that angle ACB is 115° and angle ABC is 35°. Find the distance from A to B. See FIGURE 4.3.8.

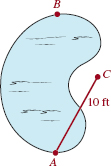
FIGURE 4.3.8 Pool in Problem 17

18. Width of a River Two points A and B lie on opposite sides of a river. Another point C is located on the same side of the river as B at a distance of 230 ft from B. If angle ABC is 105° and angle ACB is 20°, find the distance across the river from A to B.

19. Length of a Telephone Pole A telephone pole makes an angle of 82° with the level ground. As shown in FIGURE 4.3.9, the angle of elevation of the Sun is 76°. Find the length of the telephone pole if its shadow is 3.5 m. (Assume that the tilt of the pole is away from the Sun and in the same plane as the pole and the Sun.)

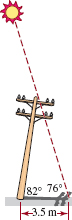
FIGURE 4.3.9 Telephone pole in Problem 19

20. Not on the Level A man 5 ft 9 in. tall stands on a sidewalk that slopes down at a constant angle. A vertical street lamp directly behind him causes his shadow to be 25 ft long. The angle of depression from the top of the man to the tip of his shadow is 31°. Find the angle a, as shown in FIGURE 4.3.10, that the sidewalk makes with the horizontal.

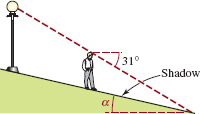
FIGURE 4.3.10 Sloping sidewalk in Problem 20

21. How High? If the man in Problem 20 is 20 ft down the sidewalk from the lamppost, find the height of the light above the sidewalk.

22. Plane with an Altitude Angles of elevation to an airplane are measured from the top and the base of a building that is 20 m tall. The angle from the top of the building is 38°, and the angle from the base of the building is 40°. Find the altitude of the airplane.

23. Angle of Drive The distance from the tee to the green on a particular golf hole is 370 yd. A golfer hits his drive and paces its distance off at 210 yd. From the point where the ball lies, he measures an angle of 160° between the tee and the green. Find the angle of his drive off the tee measured from the dashed line from the tee to the green shown in FIGURE 4.3.11.

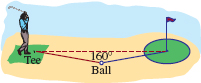
FIGURE 4.3.11 Angle of drive in Problem 23

24. In Problem 23, what is the distance from the ball to the green?

4.4 Law of Cosines

Introduction Triangles for which we know either three sides or two sides and the included angle (that is, the angle formed by the given sides) cannot be solved directly using the Law of Sines. The method we consider next can be used to solve triangles in these two cases.

Pythagorean Theorem In a right triangle, such as the one shown in FIGURE 4.4.1, the length c of the hypotenuse is related to the lengths a and b of the other two sides by the Pythagorean theorem

This last equation is a special case of a general formula that relates the lengths of the sides of any triangle.

FIGURE 4.4.1 Right triangle

Law of Cosines A generalization of (1) is called the Law of Cosines. Like the Law of Sines, (1) of Section 4.3, the Law of Cosines is valid for any triangle. But for convenience, we will prove the last two equations in (2) of Theorem 4.4.1 using again the acute triangle given in FIGURE 4.3.2.

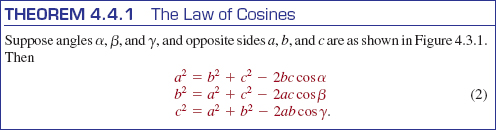
PROOF: Let P denote the point where the altitude from the vertex A intersects side BC. Then, since both BPA and CPA in FIGURE 4.4.2 are right triangles we have from (1),

and

Now the length of BC is a = c cos β + b cos γ so that

Moreover, from (4),

Substituting (5) and (6) into (3) and simplifying yields the third equation in (2):

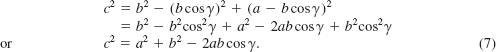
Note that equation (7) reduces to the Pythagorean theorem (1) when γ = 90°.

Similarly, if we use b cos γ = a — c cos β and h² = c² — (c cos β)² to eliminate b cos γ and h² in (4), we obtain the second equation in (2).

FIGURE 4.4.2 Acute triangle

EXAMPLE 1 Determining the Parts of a Triangle

Find the remaining parts of the triangle shown in FIGURE 4.4.3.

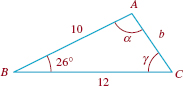
FIGURE 4.4.3 Triangle in Example 1

Solution First, if we call the unknown side b and identify a = 12, c = 10, and β = 26°, then from the second equation in (2) we can write

Therefore, b² ≈ 28.2894 and so .

Next, we use the Law of Cosines to determine the remaining angles in the triangle in FIGURE 4.4.3. If γ is the angle at the vertex C, then the third equation in (2) gives

With the aid of a calculator and the inverse cosine we find . Note that since the cosine of an angle between 90° and 180° is negative, there is no need to consider two possibilities as we did in Example 3 in Section 4.3. Finally, the angle at the vertex A is

In Example 1, observe that after b is found, we know two sides and an angle opposite one of these sides. Hence we could have used the Law of Sines to find the angle γ.

In the next example we consider the case in which the lengths of the three sides of a triangle are given.

EXAMPLE 2 Determining the Angles in a Triangle

Find the angles α, β, and γ in the triangle shown in FIGURE 4.4.4.

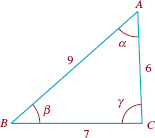
FIGURE 4.4.4 Triangle in Example 2

Solution We use Law of Cosines to find the angle opposite the longest side:

A calculator then gives . Although we could use the Law of Cosines, we choose to find β by the Law of Sines:

Since γ is the angle opposite the longest side it is the largest angle in the triangle, so β must be an acute angle. Thus, sin β ≈ 0.6659 yields . Finally, from

Bearing In navigation directions are given using bearings. A bearing designates the acute angle that a line makes with the north-south line. For example, FIGURE 4.4.5(a) illustrates a bearing of S40°W, meaning 40 degrees west of south. The bearings in Figures 4.4.5(b) and 4.4.5(c) are N65°E and S80°E, respectively.

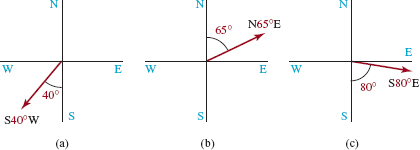
FIGURE 4.4.5 Three examples of bearings

EXAMPLE 3 Bearings of Two Ships

Two ships leave a port at 7:00 am, one traveling at 12 knots (nautical miles per hour) and the other at 10 knots. If the faster ship maintains a bearing of N47°W and the other ship maintains a bearing of S20°W, what is their separation (to the nearest nautical mile) at 11:00 am that day?

Solution Since the elapsed time is 4 hours, the faster ship has traveled 4 · 12 = 48 nautical miles from port and the slower ship 4 · 10 = 40 nautical miles. Using these distances and the given bearings, we can sketch the triangle (valid at 11:00 am) shown in FIGURE 4.4.6. In the triangle, c denotes the distance separating the ships and γ is the angle opposite that side. Since 47° + γ + 20° = 180° we find γ = 113°. Finally, the Law of Cosines

gives Thus the distance between the ships (to the nearest nautical mile) is 74 nautical miles.

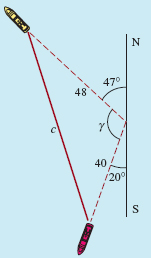
FIGURE 4.4.6 Ships in Example 3

4.4 Exercises Answers to selected odd-numbered problems begin on page ANS-12.

In Problems 1–16, refer to FIGURE 4.3.1 on page 188.

In Problems 1-16, use the Law of Cosines to solve the triangle.

1. γ = 65°, a = 5, b = 8

2. β = 48°, a = 7, c = 6

3. a = 8, b = 10, c = 7

4. γ = 31.5°, a = 4, b = 8

5. γ = 97.33°, a = 3, b = 6

6. a = 7, b = 9, c = 4

7. a = 11, b = 9.5, c = 8.2

8. α = 162°, b = 11, c = 8

9. a = 5, b = 7, c = 10

10. a = 6, b = 5, c = 7

11. a = 3, b = 4, c = 5

12. a = 5, b = 12, c = 13

13. a = 6, b = 8, c = 12

14. β = 130°, a = 4, c = 7

15. α = 22°, b = 3, c = 9

16. β = 100°, a = 22.3, b = 16.1

Miscellaneous Applications

17. How Far? A ship sails due west from a harbor for 22 nautical miles. It then sails S62°W for another 15 nautical miles. How far is the ship from the harbor?

18. How Far Apart? Two hikers leave their camp simultaneously, taking bearings of N42°W and S20°E, respectively. If they each average a rate of 5 km/h, how far apart are they after 1 h?

19. Bearings On a hiker's map point A is 2.5 in. due west of point B and point C is 3.5 in. from B and 4.2 in. from A, respectively. See FIGURE 4.4.7. Find (a) the bearing of A from C, (b) the bearing of B from C.

FIGURE 4.4.7 Triangle in Problem 19

20. How Long Will It Take? Two ships leave port simultaneously, one traveling at 15 knots and the other at 12 knots. They maintain bearings of S42°W and S10°E, respectively. After 3 hr the first ship runs aground and the second ship immediately goes to its aid.

(a) How long will it take the second ship to reach the first ship if it travels at 14 knots?

(b) What bearing should it take?

21. A Robotic Arm A two-dimensional robot arm “knows” where it is by keeping track of a “shoulder” angle a and an “elbow” angle β. As shown in FIGURE 4.4.8, this arm has a fixed point of rotation at the origin. The shoulder angle is measured counterclockwise from the x-axis, and the elbow angle is measured counterclockwise from the upper to the lower arm. Suppose that the upper and lower arms are both of length 2 and that the elbow angle β is prevented from “hyper-extending” beyond 180°. Find the angles α and β that will position the robot's hand at the point (1, 2).

FIGURE 4.4.8 Robotic arm in Problem 21

22. Which Way? Two lookout towers are situated on mountain tops A and B, 4 mi from each other. A helicopter firefighting team is located in a valley at point C, 3 mi from A and 2 mi from B. Using the line between A and B as a reference, a lookout spots a fire at an angle of 40° from tower A and 82° from tower B. See FIGURE 4.4.9. At what angle, measured from CB, should the helicopter fly in order to head directly for the fire?

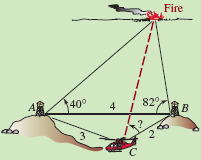
FIGURE 4.4.9 Fire in Problem 22

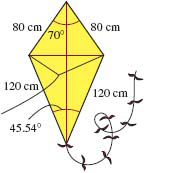
23. Making a Kite For the kite shown in FIGURE 4.4.10, use the Law of Cosines to find the lengths of the two dowels required for the diagonal supports.

FIGURE 4.4.10 Kite in Problem 23

24. Distance Across a Canyon From the floor of a canyon it takes 62 ft of rope to reach the top of one canyon wall and 86 ft to reach the top of the opposite wall. See FIGURE 4.4.11. If the two ropes make an angle of 123°, what is the distance d from the top of one canyon wall to the other?

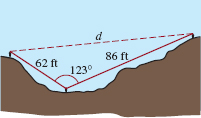
FIGURE 4.4.11 Canyon in Problem 24

For Discussion

25. Use the Law of Cosines to derive Heron's formula,^*

for the area of a triangle with sides a, b, c where .

26. Use Heron's formula in Problem 25 to find the area of a triangular garden plot if the lengths of the three sides are 25, 32, and 41 m, respectively.

27. Corner Lot Find the area of the irregular corner lot shown in FIGURE 4.4.12. [Hint: Divide the lot into two triangular lots as shown and then find the area of each triangle. Use Heron's formula in Problem 25 for the area of the acute triangle.]

28. Use Heron's formula in Problem 25 to find the area of a triangle with vertices located at (3, 2), (–3, –6), and (0, 6) in a rectangular coordinate system.

29. Blue Man The effort in climbing a flight of stairs depends largely on the flexing angle of the leading knee. A simplified blue stick-figure model of a person walking up a staircase indicates that the maximum flexing of the knee occurs when the back leg is straight and the hips are directly over the heel of the front foot. See FIGURE 4.4.13. Show that

FIGURE 4.4.12 Corner lot in Problem 27

where θ is the knee joint angle, 2a is the length of the leg, R is the rise of a single stair step, and T is the width of a step. [Hint: Let h be the vertical distance from hip to heel of the leading leg, as shown in the figure. Set up two equations involving h: one by applying the Pythagorean theorem to the right triangle whose hypotenuse consists of the back leg of length 2a and the other by using the Law of Cosines on the angle θ. Then eliminate h and solve for cos θ.]

FIGURE 4.4.13 Blue man in Problem 29

4.5 Simple Harmonic Motion

Introduction Many physical objects vibrate or oscillate in a regular manner, repeatedly moving back and forth over a definite time interval. Some examples are clock pendulums, a mass on a spring, sound waves, strings on a guitar when plucked, the human heart, tides, and alternating current. In this section we will focus on mathematical models of the undamped oscillatory motion of a mass on a spring.

Before proceeding with the main discussion we need to discuss the graph of the sum of constant multiples of cos Bx and sin Bx, that is, y = c₁ cos Bx + c₂sin Bx, where c₁ and c₂ are constants.

Addition of Two Sinusoidal Functions In Section 3.2 we examined the graphs of horizontally shifted sine and cosine graphs. It turns out that any linear combination of a sine function and a cosine function of the form

where c₁ and c₂ are constants, can be expressed either as a shifted sine function y = Asin(Bx + ∅), B > 0, or as a shifted cosine function y = Acos(Bx + ∅). Note that in (1) the sine and cosine functions have the same period 2π/B.

EXAMPLE 1 Addition of a Sine and a Cosine

Graph the function .

Solution Using a graphing utility we have shown in FIGURE 4.5.1 four cycles of the graphs of y = cos 2x (in red) and (in green). It is apparent in FIGURE 4.5.2 that the period of the sum of these two functions is π, the common period of cos 2x and sin 2x. Also apparent is that the blue graph is a horizontally shifted sine (or cosine) function. Although FIGURE 4.5.2 suggests that the amplitude of the function , the exact phase shift of the graph is certainly is not apparent.

FIGURE 4.5.1 Superimposed graphs of in Example 1

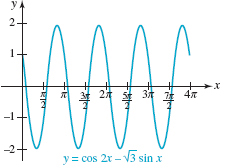
FIGURE 4.5.2 Graph of the sum in Example 1

The sine form y = Asin(Bx + ∅)is slightly easier to use than the cosine form y = Acos(Bx + ∅)

Reduction to a Sine Function We examine only the reduction of (1) to the form

where A and ∅ are defined by

and

PROOF: To prove (2), we use the sum formula (7) of Section 3.4:

 

EXAMPLE 2 Example 1 Revisited

Express as a single sine function.

Solution With the identifications c₁ = 1, c₂ = – √3, and B = 2, we have from (3) and (4),

Although tan ∅ = –1/√3 we cannot blindly assume that . The angle we take for ∅ must be consistent with the algebraic signs of sin ∅ and cos ∅. Because sin ∅ > 0 and cos ∅ < 0 the terminal side of the angle ∅ lies in the second quadrant. But since the range of the inverse tangent function is the interval (–π/2, π/2), tan^-1(–1/√3) = -π/6 is a fourth-quadrant angle. The correct angle is found by using the reference angle π/6 for tan^-1(–1/√3) to find the second-quadrant angle

Therefore can be rewritten as

Hence the graph of is the graph of y = 2 sin 2x, which has amplitude 2, period 2π/2 = π, and is shifted 5 π/12 units to the left.

Simple Harmonic Motion Consider the motion of a mass on a spring as shown in FIGURE 4.5.3. In the absence of frictional or damping forces, a mathematical model for the displacement (or directed distance) of the mass measured from a position called the equilibrium position is given by the function

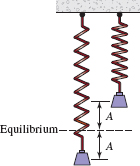
FIGURE 4.5.3 An undamped spring/mass system exhibits simple harmonic motion

Oscillatory motion modeled by the function (5) is said to be simple harmonic motion. More precisely, we have the following definition.

where A, ω > 0, and ∅ are constants, is said to exhibit simple harmonic motion.

Special cases of the trigonometric functions in (6) are y(t) = A sin ωt, y(t) = A cos ωt, and y(t) = c₁ cos ωt, + c₂sin ωt,.

Terminology The function (5) is said to be the equation of motion of the mass. Also, in (5), where k is the spring constant (an indicator of the stiffness of the spring), m is the mass attached to the spring (measured in slugs or kilograms), y₀ is the initial displacement of the mass (measured above or below the equilibrium position), v₀ is the initial velocity of the mass, t is time measured in seconds, and the period  p of motion is p = 2π/ω seconds. The number f = 1/p = 1/(2π/ω) = ω/2π is called the frequency of motion. The frequency indicates the number of cycles completed by the graph per unit time. For example, if the period of (5) is, say, p = 2 seconds, then we know that one cycle of the function is complete in 2 seconds. The frequency f = 1/p = means one-half of a cycle is complete in 1 second.

In the study of simple harmonic motion it is convenient to recast the equation of motion (5) as a single expression involving only the sine function:

The reduction of (5) to the sine function (7) can be done in exactly the same manner as illustrated in Example 2. In this situation we make the following identifications between (2) and (5):

EXAMPLE 3 Equation of Motion

(a) Find the equation of simple harmonic motion (5) for a spring mass system if slug, .

(b) Find the period and frequency of motion.

Solution(a) We begin with the simple harmonic motion equation (5). Since , therefore (5) becomes

(b) The period of motion is second; the frequency is cycles per second.

EXAMPLE 4 Example 3 Continued

Express the equation of motion (8) as a single sine function (7).

Solution With we find the amplitude of motion is

Then from

we can see from algebraic signs sin ∅ < 0 and cos ∅ > 0 that the terminal side of the angle ∅ lies in the fourth quadrant. Hence the correct value of ∅ is tan^-1(–4) = –1.3258. The equation of motion is then . As shown in FIGURE 4.5.4, the amplitude of motion is . Since we are assuming that that is no resistance to the motion, once the spring/mass system is set in motion the model indicates it stays in motion bouncing back and forth between its maximum displacement feet above the equilibrium position and a minimum of feet below the equilibrium position.

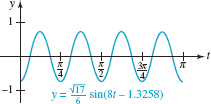
FIGURE 4.5.4 Graph of the equation of motion in Example 4

Only in the two cases, c₁ > 0, c₂ > 0 or c₁ < 0, c₂ > 0, can we use tan ∅ in (4) to write ∅ = tan^-1(c₁/c₂). (Why?) Correspondingly, ∅ is a first or a fourth-quadrant angle.

4.5 Exercises Answers to selected odd-numbered problems begin on page ANS-12.

In Problems 1-6, proceed as in Example 2 and reduce the given trigonometric expression to the form y = A sin(Bx + ∅). Sketch the graph and give the amplitude, the period, and the phase shift.

1.

2.

3.

4.

5.

6. y = sin x + cos x

In Problems 7-10, proceed as in Examples 3 and 4 and use the given information to express the equation of simple harmonic motion (5) for a spring/mass system in the trigonometric form (7). Give the amplitude, period, and frequency of motion.

7.

8.

9.

10.

11. The equation of simple harmonic motion of a spring/mass system is . Determine the initial displacement y₀and initial velocity v₀ of the mass. [Hint: Use (5).]

12. Use the equation of simple harmonic motion of the spring/mass system given in Problem 11 to find the times for which the mass passes through the equilibrium position y = 0.

Miscellaneous Applications

13. Electrical Circuits Under certain conditions the current I(t) in an electrical circuit at time t is given by . Express I(t) as a single sine function of the form given in (7). [Hint: Review the sum formula in (7) of Theorem 3.4.3.]

CHAPTER 4 Review Exercises Answers to selected odd-numbered problems begin on page ANS-12.

A. True/False_____________________________

In Problems 1-4, answer true or false.

1. In a right triangle, the side opposite the 90° angle is the longest side.____

2. If the angle of elevation to the top of a pole from the end of its 10 ft shadow is 30°, then the pole is 5 ft high.____

3. There may exist two triangles that satisfy two given sides and an angle opposite one of these sides.____

4. The Pythagorean theorem is a special case of the Law of Cosines.____

B. Fill in the Blanks______________________

In Problems 1-6, fill in the blanks.

1. In a right triangle if the hypotenuse has length 10 in. and one leg has length 5 in., then the other leg has length___________.

2. If α and β are the acute angles in a right triangle, then α + β =___________.

3. To solve a triangle in which you know two angles and a side of opposite one of these angles, you would use the Law of___________first.

4. The ambiguous case refers to solving a triangle when___________are given.

5. To solve a triangle in which you know two sides and the included angle, you would use the Law of___________first.

6. When the function is written as a single shifted sine function, then the amplitude of its graph is___________.

C. Exercises______________________________

In Problems 1-4, solve the triangle satisfying the given conditions.

1. α = 30°, β = 70°, b = 10

2. γ = 145°, a = 25, c = 20

3. α = 51°, b = 20, c = 10

4. a = 4, b = 6, c = 3

5. A surveyor 100 m from the base of an overhanging cliff measures a 28° angle of elevation from that point to the top of the cliff. See FIGURE 4.R.1. If the cliff makes an angle of 65° with the horizontal ground, determine its height h

FIGURE 4.R.1 Cliff in Problem 5

6. A rocket is launched from ground level at an angle of elevation of 43°. If the rocket hits a drone target plane flying at 20,000 ft, find the horizontal distance between the rocket launch site and the point directly beneath the plane. What is the straight-line distance between the rocket launch site and the target plane?

7. A competition water skier leaves a ramp at point R and lands at S. See FIGURE 4.R.2. A judge at point J measures an as 47°. If the distance from the ramp to the judge is 110 ft, find the length of the jump. Assume that is 90°.

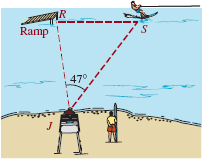
FIGURE 4.R.2 Water skier in Problem 7

8. The angle between two sides of a parallelogram is 40°. If the lengths of the sides are 5 and 10 cm, find the lengths of the two diagonals.

9. A weather satellite orbiting the equator of the Earth at a height of H = 36,000 km spots a thunderstorm to the north at P at an angle of θ = 6.5° from its vertical. See FIGURE 4.R.3.

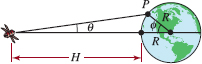
FIGURE 4.R.3 Satellite in Problem 9

(a) Given that the Earth's radius is approximately R = 6370 km, find the latitude ∅ of the thunderstorm.

(b) Show that angles θ and ∅ are related by

10. It can be shown that a basketball of diameter d approaching the basket from an angle θ to the horizontal will pass through a hoop of diameter D if D sin θ > d, where 0° ≤ θ ≤ 90°. See FIGURE 4.R.4. If the basketball has diameter 24.6 cm and the hoop has diameter 45 cm, what range of approach angles θ will result in a basket?

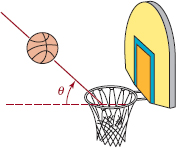
FIGURE 4.R.4 Basketball in Problem 10

11. Each of the 24 NAVSTAR Global Positioning System (GPS) satellites orbits the Earth at an altitude of h = 20,200 km. Using this network of satellites, an inexpensive hand-held GPS receiver can determine its position on the surface of the Earth to within 10 m. Find the greatest distance s (in km) on the surface of the Earth that can be observed from a single GPS satellite. See FIGURE 4.R.5. Take the radius of the Earth to be 6370 km. [Hint: Find the central angle θ subtended by s.]

FIGURE 4.R.5 GPS satellite in Problem 11

12. An airplane flying horizontally at a speed of 400 miles per hour is climbing at an angle of 6° from the horizontal. When it passes directly over a car traveling 60 miles per hour, it is 2 miles above the car. Assuming that the airplane and the car remain in the same vertical plane, find the angle of elevation from the car to the airplane after 30 minutes.

13. A house measures 45 ft from front to back. The roof measures 32 ft from the front of the house to the peak and 18 ft from the peak to the back of the house. See FIGURE 4.R.6. Find the angles of elevation of the front and back parts of the roof.

FIGURE 4.R.6 House in Problem 13

14. The angle between two sides of a parallelogram is 40°. If the lengths of sides are 5 and 10 cm, find the lengths of the two diagonals.

In Problems 15-22, translate the words into an appropriate function.

15. A 20-ft-long water trough has ends in the form of isosceles triangles with sides that are 4 ft long. See Figure 1.10.19 in Exercises 1.10. As shown in FIGURE 4.R.7, let θ denote the angle between the vertical and one of the sides of a triangular end. Express the volume of the trough as a function of 2θ.

FIGURE 4.R.7 End of water trough in Problem 15

16. A person driving a car approaches a freeway sign as shown in FIGURE 4.R.8. Let θ be her viewing angle of the sign and let x represent her horizontal distance (measured in feet) to that sign. Express θ as a function of x.

FIGURE 4.R.8 Freeway sign in Problem 16

17. As shown in FIGURE 4.R.9, a plank is supported by a sawhorse so that one end rests on the ground and the other end rests against a building. Express the length of the plank as a function of the indicated angle θ.

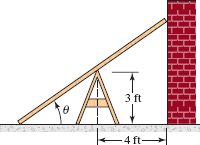
FIGURE 4.R.9 Plank in Problem 17

18. A farmer wishes to enclose a pasture in the form of a right triangle using 2000 ft of fencing on hand. See FIGURE 4.R.10. Show that the area of the pasture as a function of the indicated angle θ is

FIGURE 4.R.10 Pasture in Problem 18

19. Express the volume of the box shown in FIGURE 4.R.11 as a function of the indicated angle θ.

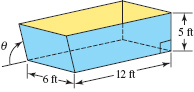
FIGURE 4.R.11 Box in Problem 19

20. A corner of an 8.5 in. X 11 in. piece of paper is folded over to the other edge of the paper as shown in FIGURE 4.R.12. Express the length L of the crease as a function of the angle θ shown in the figure.

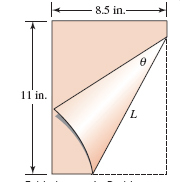
FIGURE 4.R.12 Folded paper in Problem 20

21. A gutter is to be made from a sheet of metal 30 cm wide by turning up the edges of width 10 cm along each side so that the sides make equal angles ∅ with the vertical. See FIGURE 4.R.13. Express the cross-sectional area of the gutter as a function of the angle ∅.

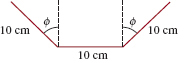
FIGURE 4.R.13 Gutter in Problem 21

22. A metal pipe is to be carried horizontally around a right-angled corner from a hallway 8 feet wide into a hallway that is 6 feet wide. See FIGURE 4.R.14. Express the length L of the pipe as a function of the angle θ shown in the figure.

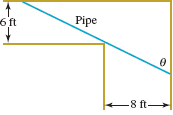
FIGURE 4.R.14 Pipe in Problem 22

23. Lighthouse of Alexandria In an article from the online encyclopedia Wikipedia, the height of the Lighthouse of Alexandria, one of the Seven Wonders of the Ancient World, is estimated to have been between 393 ft and 450 ft. The article goes on to say that there are ancient claims that the light could be seen on the ocean up to 29 miles away. Use the right triangle in FIGURE 4.R.15 along with the two given heights h to determine the accuracy of the 29 mile claim. Assume that the radius of the Earth is r = 3963 mi. and s is distance in miles measured on the ocean. [Hint: Use 1 ft = 1/5280 mi and (7) of Section 2.1.]

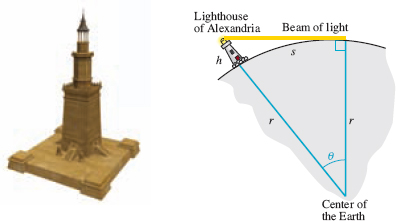
FIGURE 4.R.15 Lighthouse in Problem 23

---

^*The drawbridge shown in FIGURE 4.2.12, where the span is continuously balanced by a counterweight, is called a bascule bridge.

^*This formula is named after the Greek mathematician Heron but should actually be credited to Archimedes.