Appendix A Indicial Notation

A particular class of tensor, a vector, requires only a single subscript to describe each of its components. Often the components of a tensor require more than a single subscript for definition. For example, second-order or second-rank tensors, such as those of stress or inertia, require double subscripting: τ_ij, I_ij. Quantities such as temperature and mass are scalars, classified as tensors of zero rank.

Tensor or indicial notation, here briefly explored, offers the advantage of succinct representation of lengthy equations through the minimization of symbols. In addition, physical laws expressed in tensor form are independent of the choice of coordinate system, and therefore similarities in seemingly different physical systems are often made more apparent. That is, indicial notation generally provides insight and understanding not readily apparent to the relative newcomer to the field. It results in a saving of space and serves as an aid in nonnumerical computation. The displacement components u, v, and w, for instance, are written u₁, u₂, u₃ (or u_x, u_y, u_z), and collectively as u_i, with the understanding that the subscript i can be 1, 2, and 3 (or x, y, z). Similarly, the coordinates themselves are represented by x₁, x₂, x₃, or simply x_i(i = 1, 2, 3), and x_x, x_y, x_z, or x_i(i = x, y, z).

Two simple conventions enable us to write most equations developed in this text in indicial notation. These conventions, relative to range and summation, are as follows:

Range convention: When a lowercase alphabetic subscript is unrepeated, it takes on all values indicated.

Summation convention: When a lowercase alphabetic subscript is repeated in a term, then summation over the range of that subscript is indicated, making unnecessary the use of the summation symbol.

For example, on the basis of these conventions, the equations of equilibrium (1.11) may now be written

(A.1a)

where x_x = x, x_y = y, and x_z = z. The repeated subscript is j, indicating summation. The unrepeated subscript is i. Here i is termed the free index, and j, the dummy index.

If in the foregoing expression the symbol ∂/∂x is replaced by a comma, we have

(A.1b)

where the subscript after the comma denotes the coordinate with respect to which differentiation is performed. If no body forces exist, Eq. (A.1b) reduces to τ_ij,j = 0, indicating that the sum of the three stress derivatives is zero.

Similarly, the strain–displacement relations are expressed more concisely by using commas. Thus, Eq. (2.4) may be stated as follows:

(A.2)

The equations of transformation of the components of a stress tensor, in indicial notation, are represented by

(A.3a)

Alternatively,

(A.3b)

The repeated subscripts i and j imply the double summation in Eq. (A.3a), which, upon expansion, yields

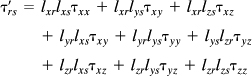
By assigning r, s = x, y, z and noting that τ_rs = τ_sr, the foregoing leads to the six expressions of Eq. (1.24).

The transformation relating coordinates x, y, z to x′, y′, z′ is applicable to the components of the strain in a manner analogous to that of the stress:

(A.4a)

Conversely,

(A.4b)

These equations represent the law of transformation for a strain tensor of rank 2. The introduction of the summation convention is attributed to A. Einstein (1879–1955). This notation, in conjunction with the tensor concept, has far reaching consequences not restricted to its notational convenience [Refs. A.1 and A.2].