MANNED INTERPLANETARY TRAVEL
9.1 IT’S NOT AN OCEAN VOYAGE OR A PLANE RIDE
In Robert Heinlein’s novel Podkayne of Mars, the eponymous heroine takes a voyage from her home on Mars colony along the first leg of a projected three-legged voyage to Venus, Earth, and back home again [118]. The ship she travels on, the Tricorn, is essentially an ocean liner moved into space; it has fancy dining halls, a ballroom, and first-class, second-class, and steerage compartments. The ship is described in detail. As is customary in a Heinlein novel, the science is pretty good: the one place where it departs most from reality is the “torch” drive, which is essentially direct matter-energy conversion, allowing the ship to travel under its own acceleration for a good part of the voyage. Even so, the trip to Venus alone takes several months because of the huge distances involved.
Other novels are filled with similar voyages. Many of them depict long voyages in cramped quarters, and in some the plots are driven by the ennui that results. In Arthur C. Clarke’s 2001: A Space Odyssey, three of the five crewmen aboard the Discovery are placed in suspended animation because the limited life support aboard the ship couldn’t handle more than two crewmen for the multiyear voyage (to Saturn’s moons in the novel, Jupiter’s in the movie). So: trips to low Earth orbit take a few hours; a trip to the Moon takes a few days; and trips to even the nearer planets take months to years, even in science fiction novels, at least in scientifically accurate ones. Why is that?
Well, this is a combination of two related factors, distance and gravity. At its closest approach, Venus, the nearest planet to Earth, is about 40 million km from the Earth, or 110 times farther away than the Moon. Mars is even farther away: at the closest approach, it is 78 million km from Earth. The Apollo astronauts traveled to the Moon in three days; at the same average speed, it would have taken them almost a year to reach Venus.
The other tricky part, gravity, is due to the fact that all of the planets in the Solar System are in orbit around the Sun; they must follow the laws of gravity as they orbit it, as must any spacecraft launched from Earth to another planet. While the spacecraft is traveling from Earth to Mars, it is essentially a tiny planet in orbit around the Sun. This means it can’t simply be pointed at Mars and launched off: Mars is moving in its orbit. Even if the craft could somehow travel on a straight line, when it got there Mars would be somewhere else. And the rocket doesn’t move on a straight line: as I said, it’s essentially a little planet too.
9.2 KEPLER’S THREE LAWS
We need to understand how planets orbit the Sun. Johannes Kepler postulated three laws governing orbital dynamics. He presented them as empirical laws, but Isaac Newton showed later that each of the laws was a consequence of his (Newton’s) law of universal gravitation. The derivation, which is complicated, may be found in any textbook on orbital dynamic, such as Fundamentals of Astrodynamics [31].
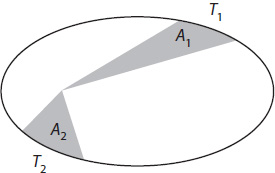
Kepler’s first law. The shape of the orbit is an ellipse, with the Sun at one focus. This is surprising to some people, who imagine that the orbits of planets around the Sun are perfect circles. In fact, the “perfection” of the circular shape was so powerful a notion that for several thousand years most astronomers felt the orbits had to be circles, or at least circles traveling on circles. An ellipse is the shape you get when you place two thumbtacks into a board, tie a string between them so that the string is slack, and, taking a pencil, pull the string taught and trace out the resultant figure on the board (fig. 9.1). It looks like a squashed circle. The foci of the ellipse are the points where the thumbtacks are. Interestingly, there is no easy way to get this result. Richard Feynman presented a lecture in which he showed how to derive the result without using calculus, but his geometrical derivation, while “elementary,” is much more difficult than standard ones using differential equations [101]. Another interesting thing is that while the Sun is at one focus, there’s nothing particular at the other one.
Three useful definitions: half the length of the ellipse along the long way is called the semimajor axis (designated a), half the short way is the semiminor axis (b), and the eccentricity, e, is defined as

The larger the eccentricity, the more squashed the ellipse is. As you might guess, an ellipse with e = 0 is a circle.
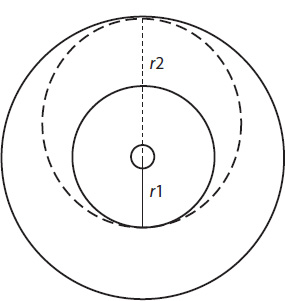
Kepler’s second law. If we draw a line segment between the planet and the Sun, and let the segment sweep out a sector, the rate at which the area of the sector increases is constant. Another way to put this is, equal areas are swept by the line segment in equal times. This is illustrated in figure 9.2. The law of areas tells us that the planet moves faster when closer to the Sun, as the arc swept out by the line segment in a given amount of time has to be bigger than when the planet is farther away, to sweep out the same area.
Kepler’s third law. We’ve seen Kepler’s third law before in chapters 6 and 8. I am now going to present this law in the form that astronomers use, because it is much simpler than how physicists present it. Essentially, this involves using the right units to make the problem simpler. I did this once before in chapter 8, where I simplified the formula for the distance of a circular orbit in terms of the period of the orbit and the mass of the planet it is circling. In that case I used a period measured in units of days and a mass measured in units of the mass of the Earth. Because planets circle the Sun, I am now going to use units of period of years because planets take years to circle the Sun. I’m going to measure masses in units where the Sun’s mass (1.99 × 1030 kg) is 1, because the stars have masses around the same as the Sun’s. I’m also not presenting it as Kepler understood it but as it is derived from Newton’s law of universal gravitation.
Let’s start by considering a planet in an elliptical orbit around the Sun. It has some average distance from the Sun, a, which can be shown to be equal to the semimajor axis of the ellipse. We are going to measure the distance, a, in astronomical units (AU): 1 AU is the average distance from the Earth to the Sun (1.5 × 108 km, or 93 million miles). It takes a certain amount of time to go around the Sun; call that its period, P. We’ll measure the period in years; thus, the average distance and period for Earth are a = 1 AU and P = 1 year. For Mars, they are a = 1.52 AU and P = 1.88 years.
The final thing we need is the mass of the star, the object these satellites, the planets, and our spacecraft are in orbit around. Call this M: we’ll measure this in units in which the mass of our own Sun is equal to 1. I do all this because using these units makes the formula easier. Converting to metric units from this point is then easy, and can be done in one step. Using these, Kepler’s third law states that for all satellites orbiting a primary with mass M,

If we have two objects whose masses are roughly equal in orbit around each other, we have to modify the formula slightly (we’ll come to this later on).
For planets (or any other objects) circling the Sun under the influence of gravity,
![]()
because M = 1 in these units. This is almost all we need to know to plot simple trajectories for space voyages to other worlds.
9.3 THE HOHMANN TRANSFER ORBIT
Let’s imagine a trip from Earth to Mars. A realistic space voyage is not much like what is shown on TV or in the movies: most movies or TV shows picture the spacecraft with their engines turned on all the time, like a plane or boat. This is not necessary and in fact wastes fuel and energy: airplanes have their engines on all the time because of air resistance. If the engine were turned off, the plane would crash in a short time.
The same isn’t true in space. In a vacuum the resistance is so low that the biggest problem with the Space Shuttle was getting it out of orbit when it had to return to Earth! Otherwise it would have continued circling the planet for years or centuries. This is even more true in interplanetary space, where the vacuum is even greater: a spacecraft placed in orbit around the Sun could conceivably circle it for billions of years, just as the planets do.1
To get a spacecraft from Earth to Mars, we want to put it into an orbit around the Sun that intersects the orbits of Earth and Mars. The simplest example of such is a Hohmann transfer, or cotangent, orbit. It was first thought up by Walter Hohmann in his 1925 work, The Attainability of Heavenly Bodies; the 1961 NASA translation is available free on the web [124]. I’ll approximate the orbits of Mars and Earth as perfect circles with radii 1.52 and 1.00 AU, respectively. I’m also going to assume that the orbits lie in the same plane. Neither of these assumptions is correct, but both are close. The eccentricity of each orbit is .0167 and 0.0935, and the orbit of Mars is tilted by only about 1.85 degrees from Earth’s. (All astronomical data are taken from appendix 4 of the textbook 21st Century Astronomy [130]). To get the spacecraft from Earth to Mars, we place it in an orbit that is tangent to Earth’s orbit at its closest approach to the Sun (its perihelion) and tangent to Mars’s orbit at its farthest distance from the Sun (the aphelion). Figure 9.3 shows the geometry of the Hohmann orbit.
We’ll get into how to do this in the next section. For now, what you need to realize is that we now know almost anything we could want to know about the orbit. For example, the trip time is half of the orbital period, which we can figure out from Kepler’s third law: the semimajor axis of the ellipse is the average of the two radii, or 1.26 AU. The period is therefore P = 1.263/2 = 1.4 years, meaning the total trip time to Mars is 0.7 years, or 255 days.
The spacecraft doesn’t use its engines for most of the trip. For most of the trip it acts as a satellite of the Sun, moving under the influence of gravity. In principle, there are only two times during the outward voyage where it burns fuel: when it leaves Earth’s orbit and when it enters the orbit of Mars. The reason is that the spacecraft needs to be moving faster than Earth does around the Sun to get into the orbit, and when it reaches Mars it is moving more slowly than Mars does in its orbit. At each of these points, the spacecraft needs to burn fuel to change its velocity. This leads us to the concept of “delta v” (Δv): the change in velocity needed to make an orbital correction.
To calculate this we need to get the speed of the two spacecraft at perihelion and aphelion. This can be calculated by using two ideas, the conservation of energy and the conservation of angular momentum. I will spare you the details of the calculation. At perihelion, the spacecraft’s velocity is

and at aphelion its velocity is

Here, Ms is the mass of the Sun, rp is the distance from the Sun at perihelion, and ra is the distance of the Sun at aphelion. One problem: we have to express everything in metric units to get this to work out correctly. (Note, however, that this will work for any planet in any orbit circling any star.) Putting the numbers in, vp = 32,670 m/s and va = 21,495 m/s. The velocity at perihelion is greater than the velocity of Earth in its orbit (29,7500 m/s) and the velocity at aphelion is less than the velocity of Mars in its orbit (24,100 m/s). These are the two times when we need to burn rocket fuel: Δvp represents the change in velocity at perihelion to get the rocket into the Hohmann orbit and Δva represents the change in the velocity at aphelion to get the rocket into the orbit of Mars. Δvp = 2,925 m/s and Δva = 2,633 m/s.
This isn’t so bad at first glance; the Δv values are smaller than the speeds needed to get from the ground into near Earth orbit (roughly 7,000 m/s). In fact, the two values are about equal to the exhaust speed of a typical chemical propellant; the rocket equation from chapter 6 tells us that we need a mass ratio of about 2.8 for a spacecraft to reach this speed. I’m going to round this up to 3 to make calculations easier. Therefore, for a 10,000 kg payload spacecraft, we need a total mass of about 30,000 kg. That is, if we consider only one orbital maneuver.
The tricky part is that we have to perform this maneuver four times for a manned spacecraft, resulting in two Δv’s going out and two coming back. The payload ratios are multiplicative. If the ratio is 3 for one maneuver, it becomes 32 = 9 for two, 33 = 27 for three, and so on. If we don’t assume that the crew can find fuel on Mars (more on this below), the net payload ratio is therefore 34 = 81. This can be done. A 10,000 kg payload requires a total spacecraft mass of 810,000 kg. But it gets trickier when we consider the launch schedule.
One other issue is that I have assumed that the spacecraft started from an orbit that was the same as Earth’s orbit around the Sun; however, to get into that orbit, it essentially must free itself of Earth’s gravitational attraction, which in itself is a fairly high Δv maneuver, about 11 km/s. I’m ignoring this for the time being, but it and any Δv’s needed to move the craft into orbit around Mars must be added into the entire Δv budget.2
9.5 GETTING BACK
It’s pretty obvious that the planets must be aligned in a precise way to send this spacecraft out. To get the orbits to work out, we need to start at a time when Mars will be in the correct place at the end of the trip. That is, at the end of the trip, Mars must be opposite, with respect to the Sun, where Earth was at the trip’s start.
The math behind this is a little finicky, but I calculate that such proper alignments happen once every 26 months. You don’t have to use a cotangent orbit, of course, but it makes sense to try to use the one that requires minimum energy. However, once at Mars you have to wait around for a while for the planets to line up again to go back. This means that the crew not only has to survive for 255 days going out but must endure more than a year waiting around for the orbits to be right again, and spend another 255 days coming back. In other words, they must while away nearly three years either in space or on the surface of Mars. You see right away why it is much simpler to send out unmanned probes. We don’t need to worry about keeping them alive or getting them back. Probes like the Mars Rovers are much smaller, much lighter, and much more fuel efficient than a manned probe, and so are much, much cheaper. A manned Mars mission would cost more than $10 billion, possibly more than $100 billion, because of the need for the crew to return to Earth alive. Of course, there have been recent suggestions that we send out people who would simply stay on Mars for the rest of their lives, mooting a return trip. At the present level of technology, this is unfeasible. It is simply a slow form of suicide for the “colonists” involved.
9.6 GRAVITATIONAL SLINGSHOTS AND CHAOTIC ORBITS
I said in the last section that the Hohmann transfer orbit is the minimum-cost orbit, but that isn’t exactly true. In reality, it is the minimum-cost orbit only if we ignore the gravitational fields of the planets the spacecraft is traveling between and consider only how the gravitational field of the Sun influences the spacecraft. This simplification is what is known in physics as a two-body problem; the only bodies being considered are the Sun and the spaceship, so the spaceship travels on a nice elliptical orbit, as Kepler’s laws demand. However, Kepler’s laws work only if we can ignore the gravitational influence of the planets along the trajectory of the spacecraft.
In chapter 7 I discussed one solution to the three-body problem, the Lagrange points between Earth and Moon. These represent periodic orbits of the system. There’s another way one can make use of a third planetary body, however: to change the orbit of a spacecraft by a close maneuver to the planet. This is known as a gravitational slingshot because one can use the gravitational field of the planet to change not only the direction of the spacecraft but also its speed. It can be used to save fuel costs for long interplanetary voyages. The most famous uses of such slingshot orbits were for the Voyager probes, which were launched in the late 1970s; the probes looked like pinballs as they seemed to careen off the outer planets. The purpose for using the gravitational slingshot was to visit as many of the outer planets as possible using the two probes. One side effect of the mission was that the probes were left with a speed greater than the escape velocity of the Solar System; they are true interstellar voyagers, if slow ones.
Here’s the basic idea. As the spacecraft goes around the Sun, we arrange it so that its orbit passes close by (within a few million miles) of one of the planets or planetoids around the sun.3 It uses the gravitational field of the planet to change the direction it is heading in and the speed it is traveling at. The maneuver takes place over a short time, short enough that we can consider only the motion of the planet and the spacecraft as it goes around it. We’ll ignore the gravitational attraction of the Sun so that we can approximate the motion of the planet and spacecraft as being on straight lines. In science fiction, an interesting example of this occurs in Larry Niven’s novel Protector, in which the Brennan-monster uses the gravitational field of a neutron star to make a right-angle turn in space; it is interesting because the direction change is made at relativistic speeds, which is a complication I will not go into here.
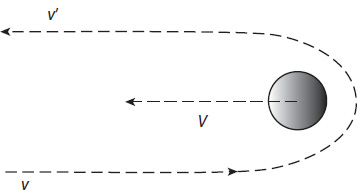
I’ll do only the simplest case, and leave a more complicated example for the problems. It’s remarkable how little information we need to calculate the final speed of the spacecraft. In the simple example, we will assume that initially the spacecraft is moving in the opposite direction as the planet, makes a 180-degree turn around it, and ends up moving in the other direction. If the planet is moving with speed V and the spacecraft is initially moving with speed v, after it goes around the planet the spacecraft will be moving with a new speed, v′ = v +2V. This comes from the conservation of momentum and energy and is independent of the details of the maneuver. All we need to know is the speeds and the initial and final directions of the spacecraft with respect to the motion of the planet.
The neat thing about this is that if the planet is moving in a circular orbit, its speed is 70% of the escape speed of the Solar System at that distance from the Sun. Therefore, the new speed of the spacecraft will be greater than the speed needed to completely escape from the system. In principle, we can use maneuvers like this to get a spacecraft anywhere in the Solar System. The issue, of course, is that the planet has to be in the right place at the right time to be able to do this; the Voyager spacecraft were launched during a window of time in which one could do several of these maneuvers on a single voyage, but such alignments of the planets are rare.
The third body of choice on a mission from Earth to Mars is the Moon. It has several nice features: it is pretty big, meaning it has a high gravitational field; it is close, at least compared to Mars, so we don’t have to spend a lot of fuel to get there; and it has no atmosphere, so the spacecraft can dive close to its surface on the voyage out. This is the basis of the popular Mission to Mars ride at EPCOT in Disneyworld.
Another means of utilizing a third body is by means of a powered slingshot to increase the speed of the spacecraft. In this case a spacecraft makes a flyby of a planet, carrying out a Δv maneuver at the point of closest approach (the periapse) of the orbit. This effectively multiplies the effectiveness of the maneuver. Let us say that the spacecraft is moving at speed v and makes a Δv change in its speed very far from a planet (in the same direction that the spacecraft is already moving). The new speed is v′ = v + Δv. However, if it is traveling at speed v far away from a planet, at periapse it will be moving at speed
![]()
where ![]() is the escape velocity from a planet with mass Mp (at distance r from its center). If it makes the same Δv maneuver at periapse, the speed of the spacecraft when it gets far away from the planet will be
is the escape velocity from a planet with mass Mp (at distance r from its center). If it makes the same Δv maneuver at periapse, the speed of the spacecraft when it gets far away from the planet will be

which is greater than v + Δv.4 The planet can be used to boost the Δv maneuver.
In the late 1980s Edward Belbruno pointed out that, at least in the case of travel from the Earth to the Moon, there were classes of orbits (which he called “chaotic orbits”) that included the effects of the Moon and Sun on the shape of the orbits. These orbits could be used to get a spaceship from the Earth to the Moon at a fraction of the energy cost of the Hohmann orbit. The price is a much longer transit time, about a month instead of three days. Such chaotic orbits were used to successfully transfer the Japanese Hiten probe from an orbit around the Earth to the Moon in 1990 [35, pp. 2ff.].
Unfortunately, none of these options to reduce the fuel and energy costs reduces the most critical parameter for manned spaceflight, the time it takes. If we want to send people to the planets on relatively low-energy orbits, we are constrained by the laws of orbital dynamics to trips that take years, and we therefore must deal with the vexing problem of keeping people alive in space for years at a time. As we saw in chapter 4, this comes with a very high sticker price, such that to date, none of the space missions to Mars or farther out has been manned.
Again the question arises, are the benefits worth the costs? Science fiction writers of the 1950s and 1960s blithely wrote about the exploration of Mars as if it were the exploration of some uncharted region on Earth, like the Conquistadors in Central and South America. Let’s consider the ground conditions on Mars to see whether this is reasonable.
The average temperature on Mars is about the same as that of Antarctica. The atmosphere is almost too thin to be considered poisonous; at “sea level” on Mars the pressure is about the same as at the top of Mount Everest, and the atmosphere is an unbreathable mix mostly composed of carbon dioxide. There is very little surface water, even in the form of ice, even at the poles (which are mostly frozen carbon dioxide.) There is strong evidence that water once flowed on the surface of Mars billions of years ago, but it’s gone now. It is barely possible that life exists on Mars at the bacterial level. We have sent several unmanned probes there to see, and so far no evidence of life has been found.
No one can stay on Mars without protection, meaning warmth and atmosphere. If astronauts were to stay there for many months, they would have two choices: either stay in a craft in orbit around Mars and explore the surface using robots, or descend to the surface and construct a habitable base. We already explore Mars using robots; the only advantage to controlling the robots from Mars orbit is that the delay time is considerably shorter (a second or so, compared to 4.3 minutes when Mars is at its closest to Earth). I’m not sure that this is enough of a gain over exploration of Mars using a robot probe to justify the factor of at least 100 in costs for such a mission.
If they descended to the surface, the question becomes what they could do on the surface that a robot probe couldn’t. This is very relevant considering the added costs of creating a base they could live in. In previous chapters we’ve considered the costs of bringing an Earth-like environment into space; any base would most likely cost billions of dollars, and there would be no a priori way to test whether it would work for the long time which our hardy explorers would need to stay in it.
The whole question of manned space exploration is a vexing one. Most science fiction writers of the 1940s through the 1980s and later foresaw the existence of colonies on Mars and on the moons of the giant planets. Robert Heinlein’s novel Farmer in the Sky, for example, posits that mankind has terraformed Jupiter’s moon Ganymede and used it for farming purposes to feed overpopulated Earth. On a more recent note, TV shows such as the Star Trek franchise, Babylon 5, and the old and new Battlestar Galactica series implicitly assume that mankind will spread out beyond planet Earth. This assumption, which is deeply ingrained in science fiction culture, comes from a time before the “rise of the robots”; no one thought that computing power, the basis of today’s unmanned robotic explorers, would increase as rapidly as it has, or be as cheap as it is. It isn’t clear there is anything that can be done by a human in space that can’t be done more cheaply and easily by a machine. At least one writer has called the notion of manned space travel “old-fashioned”. From a scientific standpoint, I think this is correct. Manned space travel is too costly and has too few benefits to justify its existence.
Most readers might now expect a closing paragraph in which I extoll the nonscientific benefits of manned space exploration: the thrill of the exploration of the unknown; the idea that mankind needs new frontiers if it is not to stagnate; the worry that if mankind is stuck on one planet, a disaster could destroy us. These are appealing ideas. But manned space exploration clearly will not happen unless we find better ways of getting off-planet and creating homelike places elsewhere. I’d like to construct an analogy: we are in the same situation with regard to manned spaceflight today as Charles Babbage was with respect to computing in the 1860s. He invented the basic ideas for the modern computer and tried to implement them using the mechanical technology of his day. The technology was marginally not good enough to allow his analytical engine to be built. We seem to be in the same situation today: chemical rockets with exhaust speeds of a few thousand meters per second are marginally good enough to launch unmanned probes traveling slowly through the Solar System but are completely inadequate for manned missions. In the next chapter we examine solutions that have been proposed for getting out there more quickly.
1. To quote the song “Shoehorn with Teeth” by the band They Might Be Giants: “Tour the world / In a Heavy Metal band / They run out of gas / The plane can never land.” Untrue for planes, true for spacecraft.
2. For a full calculation, including escape velocities from Mars and Earth, see the paper “Journey to Mars” by Arthur Stinner and John Begoray [227]. One issue with this paper is that the authors do not discuss the fuel costs correctly. They assume fuel costs proportional to kinetic energy of the spacecraft instead of using the rocket equation.
3. Technically speaking, this is a collision problem, even though the spacecraft doesn’t actually collide with the planet (at least, so we hope). The original idea for this may be due to the mathematician Stanislaw Ulam in his Los Alamos report LAMS-2219 [75, pp. 25–26] [240, chap. 9].
4. This seems paradoxical and a violation of the conservation of energy. What is happening is that the spacecraft is losing gravitational potential energy by getting closer to the planet. By burning the fuel closer to the planet you are gaining not only the kinetic energy from the spent fuel but also the potential energy it has “lost” because the spent fuel will remain close to the planet.