SPACE COLONIES
7.1 HABITATS IN SPACE
In the 1930s the science fiction writer George O. Smith penned a series of stories that were subsequently collected into a book titled The Complete Venus Equilateral [222]. The stories take place on a large space station placed in the orbit of Venus. That is, it circles the Sun in the same orbit Venus follows, but located 60 degrees ahead of Venus in orbit; it is not circling Venus. The space station serves as a radio relay, shuttling messages between Earth, Venus, and Mars, and is crewed by a few hundred engineers and support staff. The stories featured the adventures of Donald Channing, the chief engineer, and his attempts to keep the station running despite interference from mad scientists, evil bureaucrats, and space pirates. They are pure space opera and a lot of fun; additionally, the Venus Equilateral station has all the features of later proposals for real structures built in space. It has a large crew, its own ecology, and artificial “gravity,” provided by rotating the structure; it is also placed in one of the Lagrange points of Venus’s orbit around the Sun.
Communications satellites have taken away the need for a manned station, even if we establish colonies on other planets. However, there is still serious discussion of large manned space stations. The rationale for them can be summarized in a few points:
• They can be used as platforms in orbit for assembling deep space probes, which can be launched from there at a fraction of the cost as from Earth.
• They can be used to generate power for Earth from solar power (undiluted by Earth’s atmosphere and weather, and available 24 hours a day, 365 days a year).
• They can be used for industries that benefit from a zero-G environment, such as the growth of large industrial crystals.
• They can be used for industries that require extremely high-vacuum environments.
• They could potentially be used to move heavily polluting industries off Earth and into space.
• They could be used for space tourism, as large orbital hotels.
• They represent the next step in humankind’s move from Earth to inhabit the cosmos.
As in previous chapters, I’ll take a hard-nosed look at these reasons. First, however, we need to look into the science behind the station.
7.2 O’NEILL COLONIES
I will first describe a community of what I like to call “moderate” size; it is larger than the first model habitat, but far below the dimensions that might be built. “Island Three” is efficient enough in the use of materials that it might be built in the early years of the next century…. Within the limits of present technology, “Island Three” could have a diameter of four miles, a length of twenty miles, and a total land area of five hundred square miles, supporting a population of several million people.
—GERARD K. O’NEILL, THE HIGH FRONTIER
In 1977 Gerard K. O’Neill, a physicist at Princeton University, suggested that with then current technology it was possible to build permanent habitats in space with all of the comforts of life on Earth [189]. He proposed building large space stations capable of permanent habitation for thousands or even millions of people. These structures were typically spherical or cylindrical in shape and spun on their axes to provide artificial gravity for the people living inside them.
The idea for such a structure predates him. O’Neill cites a number of predecessors, including Konstantin Tsiolkovsky and J. D. Bernal, who had similar ideas before him. The science fiction writer Arthur C. Clarke also considered Bernal an intellectual precursor, saying that Bernal’s book The World, the Flesh and the Devil was one of the most important he had ever read. However, it was O’Neill’s book that brought these ideas to the wider community, particularly as it was published shortly after the first Moon landings, when the wider human exploration of space seemed to be just around the corner.
O’Neill’s motivations for building these space colonies are noble: on reading his book, one gets the sense of a liberal-minded man concerned for the future of the human race. He envisioned a time when the bulk of Earth’s population would live off-planet in these habitats, when heavy industry would be moved into space to prevent it polluting the Earth, when the asteroid field and the Moon were mined for raw materials. In an article in the journal Physics Today he estimated that by 2008, more than 20 million people could be living off-Earth, and that by 2060 more people could be living off-Earth than on it [188]. He calculated the sticker cost of such stations to be about $30 billion for a colony housing 10,000 people (about $100 billion in 2013 money), and that such stations could pay for themselves in 20 years’ time. His work led to several incredibly detailed NASA studies of large space colonies involving multiple designs. The first of them is the impressive Space Settlements: A Design Study, a 185-page investigation of topics as diverse as launch systems, radiation shielding, and how to plan a space-based community to avoid the psychological stresses of living in a completely artificial environment [10]. The full text of this report is available on the web, along with other studies up through 1992, the same year in which Gerard O’Neill died.
The great realization was that a man falling off a roof would not feel his own weight.
—ALBERT EINSTEIN
One of the many features of life in a space station, in both science fiction and real life, is that there are essentially two realistic options: either the station is essentially gravity-free or the station is spun to provide a simulation of gravity. The third option presented in science fiction stories is some form of artificial “gravity,” a la Star Trek, but as far as anyone knows, this is impossible.
The first option, as in real life aboard the International Space Station (ISS), is that the people in the station float around in a seemingly gravity-free way. However, why they do this is subtle, as a calculation of the acceleration of gravity on the space station shows. For a spherical planet, the acceleration of gravity at any distance r away from the center of the planet is given by the formula
![]()
The ISS’s orbit is about 400 km (4×105 m) above the ground. Therefore, the distance from the center of the Earth is the radius of the Earth (6,400 km) plus the height of the orbit (400 km), for a grand total of r = 6, 800 km = 6.8 × 106 m. The weight of an object is equal to the acceleration of gravity multiplied by its mass (W = mg). Because the acceleration of gravity decreases with distance from the center of the planet, we expect that someone’s weight decreases as well. This is why a lot of people think that people are “weightless” on the space station: they must be far enough away from the center of the Earth that their weight drops off to nearly nothing. This is untrue. Let’s take the example of an adult male with a mass of 80 kg. On the surface of the Earth, the acceleration of gravity is 9.8 m/s2. Therefore, his weight is 80 kg × 9.8 m/s2 = 784 N. (The Newton, N, is the unit of force in the metric system; it has units of kgm/s2). If we repeat the calculation for the space station, the acceleration of gravity on board the station, using the numbers given above, is 8.7 m/s2, meaning that the 80 kg man’s weight on the station is W′ = 8.7 m/s2 × 80 kg = 694 N, or about 88% of his weight on Earth. The terms “zero-G” or “microgravity” environment to describe the station are misnomers; the correct idea is that the astronauts aboard the Space Station are in free fall.
Free fall is a subtle concept. There are two ideas we need to discuss here:
• The idea that a falling person would not feel his or her own weight, and
• Why an object that is falling need not hit the ground.
7.3.1 Why a Falling Person Doesn’t Feel His or Her Own Weight:
I … who have made the test, assure you that a cannonball that weighs one hundred pounds, or two hundred, or even more, does not anticipate by even one span the arrival on the ground … [of one] weighing half as much when dropped from a height of 200 braccia.
—GALILEO GALILEI, DIALOGUES CONCERNING TWO NEW SCIENCES
The above quotation concerns a realization by Galileo that has become fundamental in mechanics: objects acting under the force of gravity have an acceleration independent of their mass. If we take two cannonballs and drop them from a high tower, they will hit the ground at almost exactly the same time, even though their weights may differ by an enormous amount. (This is ignoring any effects that air resistance may have on them.)
Here’s a thought experiment: in many amusement parks there are “drop towers”, rides in which the people are lifted to some large height above the ground and then dropped toward the ground, only slowed to a stop scarily close to the ground. Now, imagine going on one of these rides with an apple in your pocket. As you are lifted up, remove the apple and then, once the fall begins, release it from your hand.
When you let it go, it falls toward the ground, of course. However, you are also falling to the ground with exactly the same acceleration as the apple; from your point of view, the apple simply hangs in the air. If you push on the apple, it moves away from you on what seems to be a straight-line path. This is from your point of view; to someone standing on the ground, it appears to move in a parabolic arc. You doesn’t feel the force of gravity acting on you because all objects around you are falling with exactly the same acceleration. What we think of as the force of gravity isn’t really the force of gravity: the heaviness we feel standing up is simply the force of the floor pushing up on us, preventing us from falling through the floor. A man falling off a roof doesn’t feel his own weight, nor do astronauts in a space station that is continually falling under the influence of gravity.
7.3.2 Why a Falling Object Doesn’t Have to Hit the Ground
Let’s say we take a cannonball, drop it from a high tower, and measure how far it falls during the time it drops. We’ll find that it falls a distance y in time t, given by the formula
![]()
where g = 9.8 m/s2 is the acceleration of gravity near the surface of the Earth. In 1 second the cannonball will fall a distance ![]() . We then try throwing the cannonball as we drop it so that our throw is perfectly horizontal—that is, the cannonball travels parallel to the surface of the Earth. Surprisingly, the distance it falls is exactly the same no matter how fast we throw it, as long as the throw is perfectly horizontal. This is because there is nothing pushing or pulling it parallel to the surface of the Earth; the only pull is toward the center of the planet. Most people have an intuitive idea that the faster the horizontal motion is, the less far it will fall in a given time, like Wiley Coyote running off a cliff in the Road Runner cartoons. Like most cartoon physics, this isn’t the way things work.
. We then try throwing the cannonball as we drop it so that our throw is perfectly horizontal—that is, the cannonball travels parallel to the surface of the Earth. Surprisingly, the distance it falls is exactly the same no matter how fast we throw it, as long as the throw is perfectly horizontal. This is because there is nothing pushing or pulling it parallel to the surface of the Earth; the only pull is toward the center of the planet. Most people have an intuitive idea that the faster the horizontal motion is, the less far it will fall in a given time, like Wiley Coyote running off a cliff in the Road Runner cartoons. Like most cartoon physics, this isn’t the way things work.
If the Earth were flat, as it appears to be over short distances, then the cannonball would simply hit the ground after a while. But because the Earth is a sphere, the ground is dropping away a bit as the cannonball flies out. Move horizontally far enough and you’ll find yourself above the surface of the Earth. How far does the cannonball have to move horizontally before it is 4.9 m above the surface? We can find this through a little geometry: let x be the distance the cannonball moves horizontally and y be the distance from the surface of the Earth, while R is the radius of the Earth (6,400 km, or 6.4 × 106 m). From the Pythagorean theorem,
![]()
Eliminating R2 on both sides of the equation, we are left with
![]()
using the fact that y is much smaller than R. From this,
![]()
Therefore, if the cannonball travels a horizontal distance of 7,920 m in 1 second, it will drop a distance equal to the distance that the horizon “dips.” Another way of saying this is that if any projectile near the surface of the Earth is given a horizontal velocity of 7,920 m/s, it will never hit the Earth. In 1 second it will fall exactly the same distance that the Earth’s surface falls away as it moves horizontally. The motion of the projectile will be circular, with a radius the same as that of the Earth.
Note that this tells us exactly the same information that equation (6.3) did; in fact, it tells us a little bit less than that equation did, because we have specified what the acceleration of gravity is. However, with a little work, the reasoning given above can be used to derive the circular velocity for a satellite at any distance from the planet.
7.4 ARTIFICIAL “GRAVITY” ON A SPACE STATION
A Space Shuttle astronaut has told me that the first few days in space feel like falling on an endlessly long roller coaster. He referred to this as the “inertia of the viscera,” which results from the small displacement of one’s internal organs owing to the absence of apparent weight. This is one of the medical effects of weightlessness, and astronauts who spend long periods of time in space tend to have medical problems associated with weightlessness, as the human body evolved in a world with a relatively high gravitational field.
You will find the idea in old science fiction novels, such as Robert Heinlein’s The Moon Is a Harsh Mistress, that low gravity or weightlessness will confer longer life on humans because the heart doesn’t need to pump as hard to move blood around the body [119]. This doesn’t seem to be true; prolonged life in free fall leads to loss of appetite, lengthening of the body by a few inches, immune system problems, muscle and bone atrophy, and increased flatulence. None of the effects is serious in the short term, but we don’t know what living in free fall for many years at a stretch will do. It’s better if we can provide some sort of artificial gravity for the inhabitants of the space station.
The easiest way to do this is to spin the space station. The TV show Babylon 5 was set on board a rotating space station five miles long in the shape of a cylinder. It rotated on its long axis to provide the sensation of gravity, as do many other space stations in science fiction. Among these are the already mentioned Venus Equilateral, the space station in Robert Heinlein’s juvenile novel Space Cadet, and many, many others. Let’s say that the space station is a long, hollow cylinder spinning around its (long) axis. If the cylinder radius is R and the rotational speed of the cylinder is v, the acceleration of an object moving around in the circle is
![]()
The acceleration is centripetal, that is, directed toward the center (see appendix 1). The acceleration must be caused by a force; for a person of mass M, the force is equal to Mv2/R and is supplied by the push of the station’s hull against her feet.
Another way to look at this is to view this force from the point of view of someone inside the station, tied the rotating station. From her point of view, there is a “force” pushing her to the outside of the station: the force produces an acceleration outward from the center of the station whose magnitude is
![]()
She will attribute this to a (fictitious) “centrifugal” force pushing her outward against the hull. Therefore, a person in a rotating space station will seem to feel her normal weight if we rotate the station fast enough. If the station has a radius R = 1 km, we need a rotational speed
![]()
to have an “acceleration of gravity” on the station the same as at the surface of the Earth. Using these numbers, the time for one complete rotation of the station is just over one minute. However, all is not quite the same as on Earth.
7.4.1 Thrown for a Loop
There are effects resulting from the rotation of the station that are interesting and produce different results from what one would see on Earth. The rotation gives rise to two “pseudo-forces”: in addition to the centrifugal force pushing against the hull, there is also a Coriolis force acting on moving objects. The Coriolis force also exists on Earth but is relatively weak compared to the force of gravity; its major action is on very large dynamical systems such as hurricanes, which it causes to spin one way north of the equator, and in the opposite direction south of it.1 On a space station, however, the Coriolis effect is very strong because of the relatively small size of the station and the relatively large spin rate.
Imagine standing inside the station holding an apple in your hand and letting it go. From your point of view (rotating along with the station), the apple falls to the “ground” right next to your feet because of this “centrifugal” force pushing outward. However, to someone outside the station looking in, the situation is very different. Let’s take two people, Susan and Mike. The names of our two observers are taken from the 1990s TV show Babylon 5, set in the eponymous O’Neill colony; Mike Garibaldi was the head of security for the station and Susan Ivanova was the second in command. To date, it remains one of the few TV shows that have tried to get the space science reasonably accurate, apart from having sound in space. Let’s say that Susan is in a space suit floating in space outside the station, looking in. Mike is inside holding an apple; he is about to open his hand and let the apple “fall.” What he sees the apple doing is very different from what she sees the apple doing. This is because if the station is large enough, Mike will not notice the rotation.
Mike and the apple are rotating along with the rest of the station. When Mike lets go of the apple, however, it doesn’t rotate; instead, because of its inertia, it moves in a straight-line path tangent to the circle it was just moving on with the speed it had just before being released. This is the key point in going from one point of view to the other: the reason why Mike sees the apple fall is that his rotation along with the rest of the station means that the apple is moving. When it is free from his hand, Newton’s first law takes over and it moves in a straight-line path. It hits the hull near the point where Susan’s feet are rotated to as it falls. To Susan, it moves in a straight-line path at constant speed; to Mike, in the rotating spaceship, it appears to drop from his hand and accelerate to the hull. The apparent acceleration is due to the fact that he isn’t moving along a straight line.
But because the apple is in Mike’s hand when it is released, it is slightly closer to the axis of the space station than his feet are. It travels on a smaller circle around the center of the station than his feet do, but it travels that smaller circle in the same amount of time. Because it moves less far in the same time, it is moving with a slightly smaller speed than his feet are. If he drops it from a height of 2 m, it is traveling 0.2% more slowly than his feet. This means that it won’t hit the station hull exactly where his feet are but slightly in the direction opposite the direction in which the station is spinning.
This is the Coriolis effect. (I caution that we are discussing only one aspect of the Coriolis effect here. For a complete description, see any advanced mechanics textbook, such as Classical Mechanics, by Herbert Goldstein [99, pp. 177–183].) If the station is rotating in a clockwise sense, Mike seems to see a force deflecting the falling apple counter clockwise as it falls. For objects that are either moving quickly inside the space station or projected up near the central axis, the trajectories are just plain odd. If you throw a ball inside the station, it seems to act under the influence of an external force. This is illusion! Once it leaves your hand, no forces act on it. From the point of view of someone outside the station looking in, it moves in a straight line. The appearance of force comes only from the rotated motion of someone inside the station. Now, even though the spin is supposed to simulate gravity, there are big differences between the trajectories of particles in the rotating reference frame and in a real gravitational field.
Because the Earth rotates, there is a Coriolis force that acts on missiles. The mathematics describing it is complicated because of the shape of the Earth and the presence of a real force (gravity) acting on the missile. A rotating space station is much simpler: the geometry is simple enough that it is very clear what is happening to the ball, but the trajectories inside the rotating frame are extremely counterintuitive.
When Michael Garibaldi throws a baseball in the garden section of Babylon 5, he seems to see two distinct forces act on it: the centrifugal force, which pushes the ball toward the hull of the station (mimicking gravity), and a Coriolis force, which doesn’t appear in a true gravitational field [51][63][212]. The Coriolis force is known to be appreciable when the spacecraft diameter is small. It acts only on a moving object; the magnitude of the force is proportional to the velocity and the direction of the force is perpendicular to it. However, most science fiction authors ignore the Coriolis effect when talking about rotating spacecraft; the assumption seems to be that in large enough spacecraft, the effect will be negligible [189, pp. 122–124]. Also, the treatment of this Coriolis effect in rotating spacecraft in science fiction and speculative science has been confined entirely to the description of objects moving parallel to the spin axis of the ship; objects thrown down the long axis of an O’Neill colony, for example, appear to be deflected antispinward from the thrower. If you throw an object perpendicular to the spin axis, the general idea seems to be that you can ignore the Coriolis effect. Not so! For stations less than a kilometer or so in diameter, the effects are large and very strange. Let’s look at what happens when someone in a rotating station decides to start throwing a ball around.
Consider a cylindrical spacecraft with radius R, length L. The ship rotates about the axis of the cylinder at angular frequency Ω. We set up a coordinate system x, y, z with z pointing along the cylindrical axis of the ship. In this book, we will only be concerned with motion in the xy plane. Because x and y are coordinates fixed to the ship, they do not form an inertial coordinate system. We define a coordinate system at rest with respect to the fixed stars, X, Y, a frame that is coincident with x, y at time t = 0. At any time t, the transformation between the two coordinate systems is given by
![]()
![]()
So, Mike stands inside the station at a point x = 0, y = −y0 (see fig. 7.1) and throws a ball with velocity vector (vx, vy) in the x, y frame. What is the trajectory of the ball?
To Susan floating in the inertial frame X, Y, the trajectory is very simple. The ball travels on a straight line with velocity vector (vx − Ωy0, vy), because once it leaves Mike’s hand it is no longer under the influence of any forces. (Like all good physicists everywhere, we ignore air resistance.) To Susan, the ball travels on a straight line given by the equations
![]()
![]()
To Mike, it’s not at all that simple.
Let’s consider a few cases:
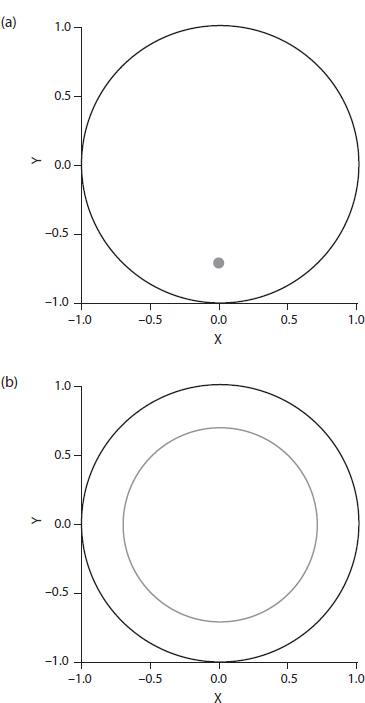
1. The “circular orbit”: vx = Ωy0, vy = 0. Mike throws the ball in an antispinward direction at exactly his rotation velocity. To Susan, the ball stops moving, because it has zero velocity in the inertial frame. Mike, however, is rotated under the stopped ball: he sees the ball follow a circular orbit around the center of the station. Figure 7.1 shows the trajectory of the ball in both the inertial and rotated reference frames. The arrow indicates the direction of the throw in the non inertial coordinate system.
This is not an unreasonable velocity for a thrown ball. Consider the cases of Babylon 5 and another fictional spacecraft, the Discovery, from 2001: A Space Odyssey. The diameter of the rotating section of Discovery is 11 m [58]. If Discovery is rotated to produce 1 g, the hull velocity is ∼7 m/s. In the episode “The Fall of Night,” Susan Ivanova mentions that Babylon 5’s garden section rotates at 60 mph (that is, 28 m/s), which is about right, if we remember that the space station is roughly 0.8 km in diameter and the acceleration of gravity in the garden section is 1/3 g. Even in an Island One structure as proposed by O’Neill, the hull velocity is only about 45 m/s, the speed of a fastball thrown by a major league pitcher [189].
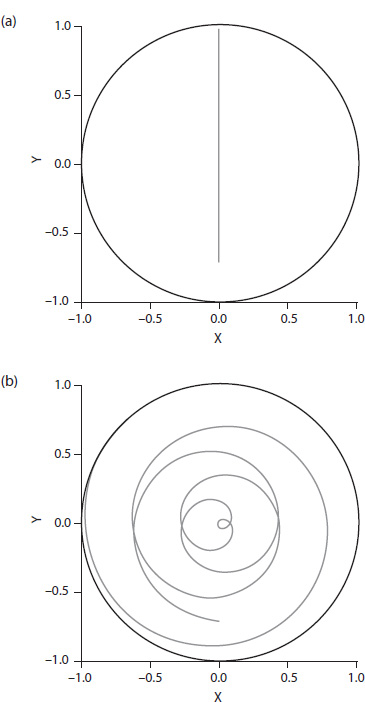
2. “Over the shoulder” trajectory: vx = Ωy0, vy = vx (fig. 7.2). Throwing the ball at an angle of 45 degrees in the air antispinward causes the ball to loop back over the thrower’s head and land behind him. In the inertial frame, the ball travels along the y-axis until its trajectory intersects the hull. Because vy is relatively large, the trajectory intersects the hull before it has enough time to make one complete rotation.
3. The “Wiley Coyote” trajectory: vx = Ωy0, vy = 2Ωy0/π (fig. 7.3). This trajectory is so named because the ball hits the thrower in the head, from the back. In this case, the y velocity is slow enough that the station makes a complete rotation before the ball intersects the hull again. One gets the feeling that golf would be a wild and rather dangerous sport on Babylon 5.
4. The “Etch-a-Sketch” trajectory: vx = Ωy0, vy = 0.08Ωy0 (fig. 7.4). The smaller the y velocity, the larger the number of times the station rotates beneath it before it intersects the hull. Here is a very complicated trajectory whose initial conditions are very close to those for a circular orbit. Unlike the problem of two-body orbits under the influence of gravity, the circular orbit of figure 7.4 is unstable to small perturbations in the initial velocity.
The laws of mechanics as observed by a traveler on a small rotating spaceship or space station are so bizarre as to be cartoonlike. In the novel Orphans of the Sky, Robert Heinlein writes of a generation ship traveling between the stars, on a journey so long that its inhabitants forget that an outside universe exists [117]. One wonders what laws of nature that world’s Galileo or Newton would invent to describe it.
In previous chapters we discussed satellites in low Earth or geosynchronous orbit. These two orbits are common settings for space stations in science fiction novels, but there is a third setting that is at least as common as the other two: the L4 and L5 Lagrange points of the Earth-Moon system.
So far we’ve discussed satellite orbits that are solutions to the two-body problem: orbits in which only the gravitational attraction of the satellite to the Earth is important. The Lagrange points are solutions to the far more difficult three-body problem, or problems involving the gravitational interactions of three separate masses. While such problems can be solved on the computer, there are almost no solutions that lead to periodic orbits as in two-body problems.
The major exceptions are the Lagrange points, first discovered by the physicist Joseph-Louis Lagrange. These are solutions to the three-body problem under the restricted assumption that one of the three is much less massive than the other two, that is, that the orbit of the other two points can be described only by considering of their own masses and motions. The five Lagrange points are shown in figure 7.5.
The points L1 through L3 are easily enough explained by considering the balance of forces acting on them. Essentially, the force of gravity due to the Earth balances out the gravitational attraction of the Moon and the “centrifugal” force resulting from the rotation of the satellite about the Earth (or, more precisely, the center of gravity of the Earth-Moon system). However, these points are unstable: objects placed in these points will tend to drift away from them over long periods of time. They are still useful, as the time periods can be large compared to the duration of any missions. For example, the proposed orbit of the James Webb Space Telescope (the successor to the Hubble Space Telescope) is at the Earth-Moon L2 point. We can approximately calculate the position of the L1 point by balancing the force of gravity from the Earth acting on the station with that of the Moon acting on the station:


Figure 7.5. Lagrange Points of the Earth-Moon System.
In this equation,
• r is the distance from the center of the Earth to the station;
• R is the distance from the center of the Earth to the center of the Moon (= 3.84 × 108 m);
• M is the mass of the Earth (= 5.97 × 1024 kg);
• m is the mass of the Moon (= 7.35 × 1022 kg); and
• G is the universal gravitational constant (= 6.67 × 10−11 Nm2/kg2).
One can solve this, to find
Because the mass of the Earth is much bigger than the mass of the Moon, the L1 point will be located much closer to the Moon than the Earth—by my calculations, nine-tenths of the distance between Earth and Moon. This is not quite all, however: there is also a centrifugal force, owing to the rotation of the space station around the center of the Earth, that seems to push the station away from the center of the Earth. (It’s the same pseudo force that we use to generate “gravity” on the space station.) Because it tends to offset the force of Earth’s gravity (for this point) its effect is to move the position of the L1 point about 5% closer to Earth, at least by my calculations. The effect of the centrifugal force is relatively small for the L1 point but must be taken into account to get the position of the L2 point. The L2 point is located beyond the Moon, so the centrifugal force must balance out the gravitational attraction of both Earth and Moon. The L3 point is located on the opposite side of the Moon orbiting the Earth: again, one must balance the “centrifugal force” against the attraction of both Earth and Moon. There are a number of science fiction stories involving “counter-Earths” circling the Sun exactly opposite Earth (essentially at the Earth-Sun L3 point); we wouldn’t see it because the Sun would block our view of it all the time. I think that this is the position of the Bizarro world of the Superman comics but cannot find a reference for this.
The L4 and L5 points are respectively 60 degrees ahead of and behind the Moon in its orbit around the Earth. They are equidistant from Earth and Moon; O’Neill proposed them as useful places for building these colonies [189]. The reason why an object that is placed there will continue to orbit is subtle; the force vector due to the resultant forces acting on it from the Moon and the Earth points toward the center of mass of the system. If the satellite’s rotational speed matches the rotational speed of the Moon around the center of mass, the satellite will stay in the orbit [232].
Orbits at the L4 and L5 points are quasi-stable: if the station is pushed out of the orbit, it tends to orbit around the Lagrange point rather than be pushed away from it, as with L1 through L3 [232]. However, these points are far from both Earth and Moon, and hence energetically expensive to reach and to place satellites in. Why choose them?
His original papers show that O’Neill chose these points because he was thinking big. Remember that he projected that by the middle of the twenty-first century, more people would be living off-Earth than on it. He therefore needed room for extremely large structures, further envisioning that a network of these structures could one day be transformed into a Dyson shell, a truly huge structure surrounding the Sun that a very advanced civilization could use to harvest all the Sun’s available power [188]. O’Neill also considered that mining the Moon for raw materials would be needed to build these colonies; the Lagrange points are in some sense a compromise position between building a colony in orbit around the Earth or building one in orbit around the Moon. He pointed out, sensibly, that because escape velocity on the Moon is only about one-third the escape velocity from the Earth, the energy costs of lifting objects to the Lagrange points from the Moon is much less expensive than from the Earth. However, this requires the ability to mine the moon for raw materials, something he assumed would be possible relatively quickly post-Apollo but has not yet happened (if it ever will).
7.6 OFF-EARTH ECOLOGY AND ENERGY ISSUES
Now that we have supplied our colony with gravity and found a place in the heavens for it, what other needs do its inhabitants have? Food, air, an ambient temperature between about 0°C and 30°C: these can serve as basic human needs. However, we can’t go about supplying them in the same way that we supply these needs for the Space Shuttle astronauts; this is supposed to be a self-sustaining colony, ready for longterm human habitation, not a trip lasting a few days to a week. This implies that the station needs some sort of ecology: the people on board need to grow their own food and recycle waste products so that they can live as independently of Earth as possible. Otherwise there is no way that the station can become economically viable, given the cost of transporting food and other goods into space. Building such an artificial environment will not be easy, especially when one considers the size and interdependence of ecosystems on spaceship Earth.
7.6.1 Food
An adult male with a mass of about 80 kg needs approximately 2,000 kcal of food energy to sustain him for one day; an adult female with a mass of about 70 kg needs about 1,800 kcal. (The kilocalorie, or food calorie, is a unit of energy equal to 4,200 J.) Other nutritional needs aside, this can be supplied in a variety of ways: meat is the most energy-dense food, with a caloric content of about 3,000 kcal/kg, while fruits and vegetables are less energy dense (about 600 kcal/kg). Meat, however, is very expensive in energy terms to produce, as one must raise the food animals on other food, usually some type of grain. It is about ten times more expensive to grow the grain to feed the animal, which is then slaughtered to feed the people, than it is to feed the people directly on the grain. Agricultural land on a space station will be some of the most expensive real estate anywhere, leading me to believe that very little of it will be used to raise beef, pork, or chicken. Assuming that the inhabitants are essentially vegetarian, they will need about 3 kg of grain and vegetables to eat per person per day, or about 30,000 kg/day for a station with a population of 10,000. This works out to about 107 kg of food per year.
At this point the choices available to the science fiction writer branch out exponentially. Will the food be grown traditionally, meaning that somehow the station must have room for acres of soil spread out and access to sunlight, or will it be grown hydroponically? Will plants be fertilized naturally or artificially? If artificially, how is the fertilizer to be produced? As an aside, the creation of ammonia via the Haber-Bosch process is very expensive, energetically speaking: modern-day agriculture depends strongly on this process, which is estimated to use some 1%–2% of all the energy used in the world [105]. Heinlein’s novel Farmer in the Sky explores some of these issues. The novel is set on a colony on Ganymede rather than a space station. It goes into the ecology of farming in detail. The farming is done in a very low-tech manner, with topsoil being generated from Ganymedan rocks fertilized with bacteria and other organisms brought from Earth, mostly by hand and stoop labor [111]. This is an odd decision by Heinlein given the level of technology exhibited in the book, which includes limitless energy generation through the conversion of matter to antimatter.
One way to clear out these complications is by considering the conservation of energy and the idea that in growing and eating food, we are transforming energy from one form to another. At every step of the way, some fraction of the energy is lost. The prospective author must work through all the details, but let’s make some assumptions and see what we get.
• First, let’s assume that the space station is in orbit around the Earth or the Moon, or is in one of the Lagrange points. This is probably the most common assumption in science fiction, but it lets us assume that the flux of sunlight reaching the station is about the same as that reaching Earth from the Sun.
• Second, let’s assume we have a large station to feed, and one that is somehow self-sustaining. Whether it is perfectly self-sustaining is another issue, but let’s assume that most of the food consumed on the station is somehow produced on the station. Further, we’ll assume that the station has some 10,000 people on board, again about the size of the station in Babylon 5.
• Third, let’s assume that the energy to grow the crops is coming from the Sun. Again, we need not assume this, but this is the energy source of agriculture on Earth, and is free. We need not assume that we are shining the Sun directly on the growing plants; we could use solar power to generate electricity to run lights for hydroponic tanks. The solar constant above Earth’s atmosphere is 1,360 W/m2, meaning that every square meter of the (projected area) of the station (turned toward the Sun) is illuminated by 1,360 J of energy every second.
• Finally, let’s assume a food consumption of 2,500 kcal/day per person. This is an energy usage rate; in metric units, it works out to about 120 W, or a relatively bright light bulb, per person. This means that 120 W per person × 104 people = 1.2 × 106 W must be available in the form of food energy.
Inefficiencies crop up in at least two places in this chain:
• The conversion of solar power into energy stored in a plant; and
• The fact that only a small portion of a plant is edible.
Plants are very roughly 1% efficient in the conversion of solar power into energy stored as biomass via photosynthesis. There are many reasons for this overall efficiency; the maximum possible efficiency is around 13% conversion from basic energetic grounds, but there are larger overall inefficiencies [245]. The second point is that only a small portion of the plant is edible; corn kernels or wheat endosperm make up only a small fraction of the overall mass of the plant, say another 1%. The overall efficiency is therefore only about (10−2)2 = 10−4. Therefore, the total power needed to supply the station for its agricultural needs is 1.2 × 106 W × 104 ≈ 1010 W. Using the solar constant, the total illuminated area therefore is
![]()
It is relatively easy to show that the other power needs for the people on board the station are small compared to the energy required for crop growth (assuming that the energy needs are comparable to that of the average American today). We can account for this by adjusting the area of the station slightly upward, say, to 107 m2. If the station is in the shape of a cylinder 2 km in diameter, then the length of the station must therefore be about 5 km to supply these energy needs.2 This also works out to about 1,000 m2 per person to supply the energy needed to grow the food for their survival. A useful reference for doing these sorts of estimates in detail is the nifty little book The Fire of Life, by Max Kleiber. The subtitle of the book, An Introduction to Animal Energetics, says it all. Table 19.5 of the book is the aptly titled “Area Yielding Food Energy for One Man Per Year”; ignoring the entry on algae (presumably Chlorella), we see that we need between 600 m2 to 1,500 m2 per person, and more if we want meat and eggs. This is right in line with what I estimated [140, p. 341].
Of course, this is only an order-of-magnitude estimate, and there are other options. For example, instead of using natural lighting, one might illuminate the plants by means of white LEDs powered by large solar panels plating the outside the station or floating nearby. Even though the conversion of sunlight into electricity into the light emitted by the LEDs involves energy losses at each step of the way, one might overall do better than by using sunlight alone because much of the solar energy spectrum is useless for photosynthesis, being in the infrared spectrum. By concentrating light in the spectral region where plants can use it, the space station’s inhabitants might be able to boost overall energy efficiency. This is the approach taken by Eric Yam in his design for the space station Asten. He takes a very detailed bottom-up approach, whereas the analysis in this chapter is top down, but his design has a total solar cell area of about 3 × 106 m2, which is pretty close to what I calculate here [11]3.
7.6.2 Atmosphere
Have you heard about the new restaurant on the Moon? Great food, but no atmosphere.
—ANONYMOUS
Earth’s atmosphere is composed of 74% N2, 24% O2, and roughly 2% trace gases. Earth’s mean temperature is about 288 K, or 15°C, and atmospheric pressure at sea level on Earth is about 1 × 105 N/m2. I’m going to assume that we need this mix of atmospheric components on our space station, although this isn’t necessarily true; scuba divers use a different mix of gases (namely, oxygen and helium) when executing deep-sea dives. Oxygen is a highly reactive gas; it is not present in the atmosphere of any other planet in the solar system because it reacts with other gases or solids. On Mars, much of the oxygen is bound up in the soil or in the form of CO2 in the atmosphere. The reason why Earth has so much oxygen in its atmosphere is life. The respiration cycle in which plants take up CO2 and liberate oxygen, which animals then breathe and exhale CO2, is taught in every kindergarten class in the nation. Plant life thus serves a dual use on our station, providing not only food but also oxygen for the inhabitants to breathe. This has long been realized by science fiction writers. For example, in George Smith’s story “Venus Equilateral,” the engineers aboard the station must circumvent disaster when the new station head misguidedly clears out the “weeds” in the station’s air recycling plant, not knowing that they are the air recycling plant(s) [222].
We must imagine that there are enough crops or other vegetation to supply the station with its atmospheric needs. Let’s do a quick, back-of-the-envelope calculation to see if we have enough plants already from agriculture to supply these needs. I’ll assume the following:
• The average adult breathes about 20 times per minute.
• The volume taken in each breath is about 1 liter (= 10−3 m3).
• Oxygen is generated via photosynthesis powered by the Sun at an efficiency of about 1% (as in the previous section).
• The atmosphere on the station is at the same pressure and has the same content (about 74% N2, 24% O2, and 2% trace gases) as Earth’s atmosphere.
• There are 10,000 people on the station.
By my calculations the plants on the station will need to generate about 0.5 kg of oxygen per second to supply the needs of the people. (This is an overestimate, because not all the oxygen in each breath is taken up by the body.) The energy needs to generate this amount of oxygen can be found by considering the chemical reaction for photosynthesis [125, p. 515]:
![]()
It’s an endothermic reaction, meaning that 2,870 kJ of energy in the form of visible light must be supplied to create one mole of glucose from 6 moles of CO2 and water. In the process, 6 moles of O2 are formed, which can be used for human and animal respiration; since each mole of O2 has a mass of 32 grams, oxygen can be supplied by photosynthesis at an energy cost of 1.5×107 J/kg. If again we assume that the overall efficiency of the process is about 1%, it will take about 7.5 × 108 W from sunlight to supply the respiration needs for the colony. This is about 6% of the energy needed for agricultural purposes, so we should be well covered by the agricultural energy budget. Another way to put it is that we need about 50 m2 of plant area per person to provide sufficient oxygen in the atmosphere, but nearly 1,000 m2 to provide food.4 Interestingly enough, in the novel Space Cadet, Robert Heinlein uses what is clearly too low a figure for the area of plants needed to provide oxygen for a human being (10 ft2, or about 1 m2); the figure is off by about two orders of magnitude, as our calculations and other data show [110].
Governmental interest in high-orbital manufacturing stems in part from calculations on its economics. These suggest that a community in space could supply large amounts of energy to the Earth, and that a private, perhaps multinational investment in a first space habitat could be returned several times over in profits.
—GERARD K. O’NEILL, THE HIGH FRONTIER
O’Neill’s space stations were always meant to be economically feasible enterprises. When counting costs he assumed, as did many others at the time, that the dominant expense of putting people into space would be the fuel costs. He therefore studied a number of alternative propulsion systems such as railguns for launching materials from the Earth or Moon. However, fuel costs are not the dominant costs for space travel today. The dominant costs currently are infrastructure costs, which most science fiction writers tended to grossly underestimate when writing their books. I think that O’Neill probably fell into this trap as well; his $30 billion investment to construct the station seems low, even when measured in 1977 currency. Let’s estimate the cost of putting a station into space using numbers appropriate for today’s space program.
Robert Heinlein’s novel Space Cadet featured Terra Station, a large, autonomous space colony in geosynchronous orbit around Earth. In the book, it mentions that the mass of the station is 600,000 tons, or roughly 6×108 kg [110, p. 25]. From chapter 2, the current cost of putting things into space is about $9,000/lb, or nearly $20,000/kg. Let’s assume we can lower this cost by an order of magnitude, or $2,000/kg. Then, the cost of putting the material to make such a station would be about $1.2 trillion.
This seems about right. Eric Yam’s proposal for Asten estimated that the total cost of building it would be $500,000,000, or half a trillion dollars, or roughly a twentieth of the GDP of the United States [11]. This figure strikes me as a low estimate. If you look at Yam’s space station budget, several items are persistently underestimated, such as the construction processes (including mining the Moon for raw materials), which are estimated at only $22 billion. Be that as it may, the proposal is incredibly detailed, and I would certainly like to live on space station Asten if it ever gets built. But let’s play a hard-nosed bean counter who is asked to evaluate whether the station is worth investing in. An investment of $500 billion over 20 years at 5% interest means that ultimately $790 billion will need to be paid back to investors. Since there are 10,000 people aboard the station, each of them is responsible for generating $79 million in revenue these 20 years, or about $3.2 million per year per person. This isn’t impossible. A handful of the top tech companies have similar per capita revenues. Let’s look at Yam’s proposals for the uses of the station Asten, which are similar to ones that other space station designers have proposed:
• Mining the moon for raw materials. This is the biggest proposed use for the station. The minimum energy required to transport 1 kg of materials from geosynchronous orbit to the Moon is 4 × 106 J, as compared to 6 × 107 J from Earth to the Moon. We therefore achieve a ten times reduction in energy costs by building this satellite—more, in fact, once the rocket equation comes into the mix. One question: do we need to mine the Moon? The reason given is to build more space stations and larger spaceships, which sounds suspiciously like a circular argument to me.
• Crystal growing. This has been a mantra of space enthusiasts for the past twenty years: one can grow very large, very defect-free crystals for the computer industry in space. However, there are two problems with this proposal. First, because of improvements in crystal-growing technology on Earth, one can already grow very large, perfect crystals for the computer industry. Second, getting them from geosynchronous orbit down to Earth would cost a lot. Even if we assume something like the space elevator, the costs would still exceed $100/kg, and perhaps exceed $1,000/kg. Crystal growing is estimated as 9% of the space station’s GDP; this implies that it must return a profit of at least $90 billion over the 20-year investment period we assumed, or about $4.5 billion per year. This is comparable to the yearly profits of the entire U.S. semiconductor industry, which seems a stretch for one small space station.
• Building large space telescopes and other space construction projects. Again, we can build and launch them from Earth without the enormous initial price tag.
• Other stuff. Here the Asten proposal gets plain silly. There is a discussion of creating homogeneous mixtures, “perfect spheres,” biomedical research, and so forth, all of which can be done on Earth for a tiny fraction of the price tag. The author is repeating the NASA line that proponents of space station Freedom spouted in the early 1990s. They didn’t make scientific sense then, they don’t make scientific sense now.
The only way to build such a habitat economically is to reduce the costs of transport into space by about two orders of magnitude, to a few hundred dollars per kilogram. Let’s take a look at one suggestion for doing this in the next chapter: the space elevator.
NOTES
1. The idea that water in the toilet spins the way it does because of this effect is an urban myth.
2. I’m assuming that the effective cross-sectional area for light absorption for the station is a rectangle whose length is the length of the station and whose width is equal to its diameter. This takes into account the fact that light will be absorbed obliquely because of its overall shape.
3. Asten won the 2009 Grand Prize for the NASA Space Settlement Design contest. This is a yearly contest open to high school and middle school students to design workable models for space stations. Although I am leery of whether Asten makes any financial sense, the basic physics and engineering underlying it appear to be sound.
4. Another way to do this calculation is to use the fact that in the presence of enough sunlight and a sufficiently high CO2 concentration in the atmosphere, plants can produce a maximum of about 20 × 10−6 moles/m2/s of leaf area, and work from there [245, p. 139, fig. 6.4]. Using this, I estimate 178 m2 of leaf area per person needed to provide the oxygen, which is about three times larger than my back-of-the-envelope calculation. However, the moral is still the same: atmospheric oxygen needs will be provided amply by crops grown for food.