Persi Diaconis
Born: January 31, 1945
THE MATHEMAGICIAN
When Persi Diaconis was fourteen years old, he dropped out of high school and ran away from home to travel with Dai Vernon, a world-class magician. Persi became a professional magician, performing all over the world for more than a decade. One of the things he loved about magic was the math behind some of his tricks. Eventually, Persi decided to go back to school and began studying math at City College of New York, where he got his bachelor’s degree. Later, the head of Harvard’s statistics department, who was also a math-emagician (mathematical magician), arranged for Persi to get into Harvard’s PhD program. Persi has been a math and statistics professor at either Harvard or Stanford ever since.
CARD SHUFFLING
Persi’s mathematical interests include randomness and randomization, especially coin flipping and card shuffling. One of his most famous papers investigated how many times a normal fifty-two-card deck needs to be riffle (standard) shuffled to be considered truly randomized. The paper proved that a deck should be shuffled seven times, and that more shuffles wouldn’t introduce much extra randomness. More than a decade after publishing that paper, a company that makes card-shuffling machines used by casinos asked Persi to check whether their machines were randomizing the decks enough. It turned out that the machines weren’t shuffling well enough, so it’s a good thing they asked Persi to check! The mathematics of card shuffling has applications to turbulence, fluid dynamics, and even how long a vat of cookie dough must be blended to make sure the ingredients are mixed enough.
FLIPPING COINS
Persi has also investigated whether a coin flip is as fair as we think. He found out that a flipped coin is more likely to end up on the same side on which it starts. But he is quick to point out that the coin will only land on the same side about 51 percent of the time, so flipping a coin is still pretty close to fair. That said, you should never agree to flip a coin with Persi, as he has practiced so much that he can flip a coin to come up heads ten times in a row!
DIACONIS THE DEBUNKER
Persi is a well-known skeptic. He famously debunked some prominent psychics who claimed to be experts in extrasensory perception (also known as ESP or sixth sense). Persi believes he is in a better position than most to figure out what is really going on when someone is supposedly practicing telepathy or bending spoons with their mind. Magicians, like psychics, use many psychological tricks, so Persi can better spot them in use. Also, his deep understanding of statistics helps him know when someone is using statistics in a sloppy or intentionally deceptive way.
HONORS AND AWARDS
Persi Diaconis won the MacArthur Fellowship (often called the “genius prize”) in 1982. He and Ron Graham (whom you can also read about in this book) wrote a book called Magical Mathematics: The Mathematical Ideas That Animate Great Magic Tricks. The book won the Euler Book Prize for “an outstanding book in mathematics that is likely to improve the public view of the field.” Persi is very proud to be a member of the National Academy of Sciences, American Philosophical Society, and American Academy of Arts and Sciences.
MATHEMATICAL MAGIC
Did you know that many of the magic tricks magicians do are based on math? Here you’ll learn how to amaze your friends with mathematical magic, just like Persi Diaconis does!
MATERIALS
- A full deck of 52 cards
DIRECTIONS
1 Give your audience a pile of nine cards from your deck.
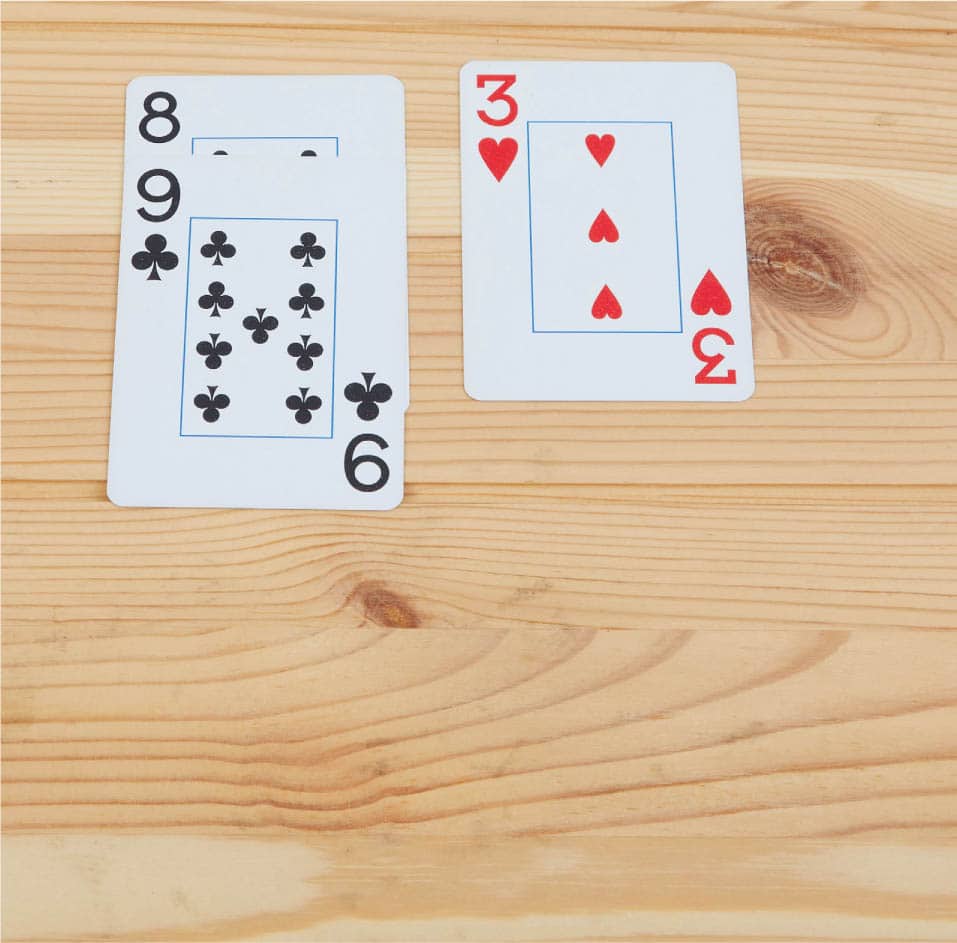
2 Tell your audience, “Here’s a small pile of cards. Pick one, memorize it, then put it on top of the others in a small stack.” Fig. 1.
3 Shuffle the deck, keeping the nine cards from the audience’s pile on the bottom in the same order they gave you the cards. In our example, the ace of diamonds was the chosen card. You can see it is on top of the small pile. When you perform the trick, make sure it is facedown. We only put it faceup in our example so you can see it. Fig. 2.
OPTIONAL: Feel free to tell your audience you aren’t good at shuffling but that it doesn’t really matter. Then you can pretend to shuffle. The only thing that matters is making sure the pile of nine cards you gave them remains on the bottom.
4 Now comes the tricky part! Tell your audience you’re going to count down from ten, flipping over a top card each time, and if the card number matches the number you say, you’ll stop making your pile. Kings, queens, and jacks are worth ten while aces are worth one.
EXAMPLE: First you say, “Ten!” and flip over a card. Here it is an eight. Eight is not ten, the number you said, so you continue. If the card had been a king, queen, jack, or ten, you would have stopped. Fig. 3.
Next, you say, “Nine!” and flip over a card. It is a nine! Since the card you flipped is the same as the number you said, your pile is done! Fig. 4.
5 You’ll make a total of four piles. If you count all the way down to one without a match, put one last card on top of the pile facedown.
EXAMPLE: Here in the second pile you drew a three, so you keep flipping. When you say nine, you draw a ten, then you draw a king when you say eight and a queen when you say seven. The sixth card is a four and the fifth card is a seven, but when you said “Four!” you flipped another four! This means your second pile is done and you start the third pile. Fig. 5 and Fig. 6.
EXAMPLE: In the next pile, you never draw the number you say. So at the end, place a card facedown on top of the pile. The fourth pile also has no match, so you place a card on top of it too. At the end, these are your four piles and what is left of your deck. Fig. 7 and Fig. 8 and Fig. 9.
6 Now add up the numbers that are faceup on your four piles. Then draw that many cards. The last card you reveal will be the audience’s chosen card!
EXAMPLE: The cards we see faceup are the nine and the four: 9 + 4 = 13, so we draw twelve cards and reveal the thirteenth. It is the ace of diamonds, the audience’s card! Fig. 10.
NOTE: If all four piles need a card on top, instead of placing a card on top of the fourth pile, reveal that card. It is your audience’s card.
November 2, 1815 – December 8, 1864 George Boole grew up in a very loving but poor family. His father, John Boole, was a member of the Lincoln Mechanics’ Institute, a group that helped working men further their education. John passed his love of learning and books on to George, who also joined the group. Unfortunately, because his father wasn’t very successful in his career, George had to start working at age sixteen to help support his parents and siblings. He got a junior teacher job in Doncaster, England. George’s first job didn’t pay enough for him to provide for his family, so when he was nineteen, he decided to open his own school in Lincoln. George was an excellent head of school with strong leadership and teaching abilities. Four years later, his great reputation placed him in a position to take over a bigger school in Waddington. Even during his early career running schools and teaching, George made time to study math. He could read multiple languages, which allowed him to read many math books and papers. Mathematician Duncan F. Gregory, who had a more intensive math research education and was the editor of the Cambridge Mathematical Journal, became a good friend and mentor to George, despite being only two years older. Duncan taught George how to write a mathematical paper, allowing George to publish his first math paper in 1840. Publishing math papers led to George becoming the first professor of mathematics at Queen’s College, Cork in Ireland in 1849. Finally, George was able to fulfill his dream of studying math full-time while still earning enough money to provide for his family. While working as a professor, George met Mary Everest (whom you can also read about in this book), the niece of one of his colleagues. The two of them became very close and eventually married. Together they had five daughters. Sadly, George died of pneumonia shortly after the birth of their fifth daughter. Their daughters had amazing careers too, many following in the footsteps of their parents to become academics. One of their children, Alicia Boole Stott, even became a respected mathematician in the field of four-dimensional geometry! George is best known for inventing Boolean logic, also known as Boolean algebra. His innovation was to apply methods from the then-new field of symbolic algebra to logic. Over two thousand years earlier, the famous philosopher Aristotle had laid out rules of logic. George wanted to broaden the rules so they would be useful in more situations and give it a solid mathematical foundation. As is often the case in mathematics, George pursued this work because it was interesting, despite the fact that there were no obvious practical applications. Nearly a hundred years later, mathematician Claude Shannon, who is commonly called “the father of information theory,” realized that Boolean algebra could optimize telephone routing switches. Using electrical switches to process logic is the fundamental concept underlying all modern computers!
Did you know that puzzles are extremely mathematical? Well, they are, and here we’ll explore a special kind—logic puzzles! They have tricky solutions and are super fun to solve. The princess’s dog, Fluffy, was kidnapped! Fluffy is a special dog with purple polka dots and golden eyes. The guards have swept the city and found five dogs matching Fluffy’s description. The problem is that each dog has a child claiming the dog is theirs. Each child—Aaliyah, Bo, Imani, Jaiden, and Marissa—has given a statement. The four who actually own a purple polka-dotted dog with golden eyes all told the truth, and the one who kidnapped the princess’s dog lied. If four are telling the truth and one is lying, figure out who kidnapped Fluffy. Here is what each child said: Aaliyah: “Bo and I got our dogs together three years ago.” Bo: “Marissa didn’t kidnap Fluffy.” Imani: “Aaliyah is telling the truth.” Jaiden: “I didn’t kidnap Fluffy.” Marissa: “I just adopted my dog; her name is Spot.” Let’s make a table. If Aaliyah is lying, the other children would be telling the truth. Is this possible? Check off the names of the people in Aaliyah’s row who could tell the truth if she is lying. Fill out each row of the table, assuming that the kid named in that row is lying. Move on to the next page, once you’re done, for the answer. If Aaliyah is lying . . . Does Aaliyah’s statement make sense? Does Bo’s statement make sense? Does Imani’s statement make sense? Does Jaiden’s statement make sense? Does Marissa’s statement make sense? Does this work? If Bo is lying . . . Does Aaliyah’s statement make sense? Does Bo’s statement make sense? Does Imani’s statement make sense? Does Jaiden’s statement make sense? Does Marissa’s statement make sense? Does this work? If Imani is lying . . . Does Aaliyah’s statement make sense? Does Bo’s statement make sense? Does Imani’s statement make sense? Does Jaiden’s statement make sense? Does Marissa’s statement make sense? Does this work? If Jaiden is lying . . . Does Aaliyah’s statement make sense? Does Bo’s statement make sense? Does Imani’s statement make sense? Does Jaiden’s statement make sense? Does Marissa’s statement make sense? Does this work? If Marissa is lying . . . Does Aaliyah’s statement make sense? Does Bo’s statement make sense? Does Imani’s statement make sense? Does Jaiden’s statement make sense? Does Marissa’s statement make sense? Does this work? Did you figure out who the liar is? Here is how we thought about it: We know that one of the children is lying and the rest are telling the truth. What happens if we assume Aaliyah is lying? If Aaliyah is lying, then she kidnapped Fluffy and all the other kids are telling the truth. That means Bo is correct, and it wasn’t Marissa, which makes sense given what Marissa said. Jaiden simply said it wasn’t him, and that works if Aaliyah is the liar. But there is a problem with Imani’s statement. Imani says Aaliyah is telling the truth, but if she is the liar, that means Imani is lying too! That doesn’t fit the rules of the puzzle, so Aaliyah can’t be the liar. Okay, so what if Bo is the liar? Bo said that Marissa didn’t kidnap Fluffy, but if Bo is lying, then Marissa is the dognapper. Why, then, would Bo lie? So Bo must be telling the truth. Since Bo is telling the truth, Marissa must be too, since Bo said she didn’t kidnap Fluffy. That just leaves two options for the liar: Imani and Jaiden. Imani says Aaliyah is telling the truth, and since we know she must be, that means Imani can’t be lying. That only leaves Jaiden, so Jaiden must be the dognapper! Here’s how we filled out the logic table to help us crack the case. If Aaliyah is lying . . . Does Aaliyah’s statement make sense? Does Bo’s statement make sense? Yes! Does Imani’s statement make sense? Imani must be lying too Does Jaiden’s statement make sense? Yes! Does Marissa’s statement make sense? Yes! Does this work? Doesn’t work If Bo is lying . . . Does Aaliyah’s statement make sense? Yes! Does Bo’s statement make sense? Does Imani’s statement make sense? Yes! Does Jaiden’s statement make sense? Yes! Does Marissa’s statement make sense? Marissa must be lying too Does this work? Doesn’t work If Imani is lying . . . Does Aaliyah’s statement make sense? Aliyah must be lying too Does Bo’s statement make sense? Yes! Does Imani’s statement make sense? Does Jaiden’s statement make sense? Yes! Does Marissa’s statement make sense? Yes! Does this work? Doesn’t work If Jaiden is lying . . . Does Aaliyah’s statement make sense? Yes! Does Bo’s statement make sense? Yes! Does Imani’s statement make sense? Yes! Does Jaiden’s statement make sense? Does Marissa’s statement make sense? Yes! Does this work? Works! If Marissa is lying . . . Does Aaliyah’s statement make sense? Yes! Does Bo’s statement make sense? Bo must be lying too Does Imani’s statement make sense? Yes! Does Jaiden’s statement make sense? Yes! Does Marissa’s statement make sense? Does this work? Doesn’t workGeorge Boole
WORKING YOUNG
CREATING A SCHOOL
BECOMING A MATH PROFESSOR
MATHEMATICAL FAMILY
BOOLEAN LOGIC
THE PRINCESS’S PUZZLES
MATERIALS
DIRECTIONS
PUZZLE 1
HOW TO SOLVE
March 23, 1882 – April 14, 1935 When Amalie Emmy Noether died, Albert Einstein wrote in the New York Times that she was widely considered to be the greatest female mathematician who had ever lived. Emmy earned her PhD in 1907 from the University of Erlangen in Germany, where she was one of only two women enrolled. After she graduated, Emmy taught at Erlangen for seven years without being paid or having an official title. In 1915, two famous mathematicians, David Hilbert and Felix Klein, invited Emmy to join the math department at the University of Göttingen, one of the best math and physics research centers in the world at that time. Various nonmath professors objected to a woman joining the faculty, but David famously responded to the controversy by saying, “I do not see that the sex of the candidate is an argument against her [being a professor]. After all, we are a university, not a bath house.” Nevertheless, Emmy was not allowed to officially join the faculty, so she spent four years teaching at the University of Göttingen by pretending to be David’s assistant. In 1918, Emmy proved what remains today one of the most important theorems in physics! The theorem, which is called Noether’s theorem, states, “Wherever a symmetry of nature exists, there is a conservation law attached to it, and vice versa.” Conservation of momentum, also known as Newton’s third law, and general relativity are both examples of Noether’s theorem. Finally, in 1919, Emmy was allowed to teach under her own name. She quickly became one of the leading members of the Göttingen math department. Her students were sometimes called “Noether boys.” In the second phase of her career, her work changed and guided abstract algebra, an important field of mathematics. In the final chapter of her influential career, Emmy united the previously separate mathematical fields of representation theory and the theory of modules and ideals, which allowed both fields to progress further than they had separately. Unlike most professors, Dr. Emmy Noether did not develop lesson plans but used lecture time to discuss important math questions with her students. Some of her students took very careful notes, which ended up being important, as many of Emmy’s most significant ideas were developed during her lectures. A few of her students even turned their detailed notes into influential textbooks. Teaching was so important to Emmy that she sometimes held classes at her home when the university was closed and once took a class to a coffee shop to deliver a lecture on a holiday. Emmy was extremely generous and sometimes allowed her students or colleagues to take credit for her ideas to help their careers. In 1933, Germany’s Nazi government fired all Jews from university jobs, forcing Emmy and many other famous scholars to flee. Emmy was so well known that she received a grant to move to Bryn Mawr College in the United States. She also taught at the Institute for Advanced Study at Princeton but didn’t feel welcome at “the men’s university, where nothing female is admitted.” On the other hand, she treasured her time at Bryn Mawr, where she had many enthusiastic female collaborators.
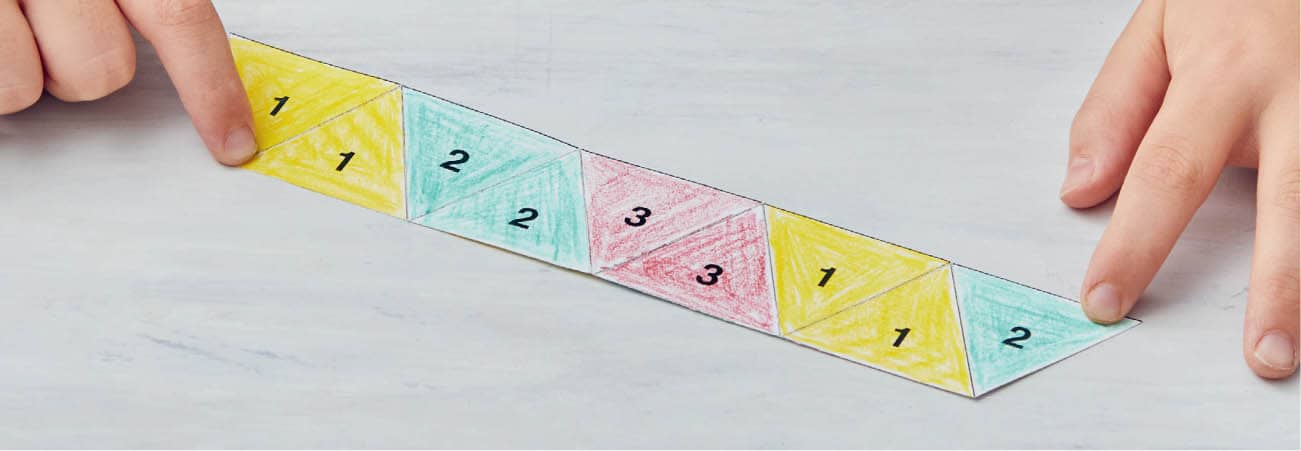
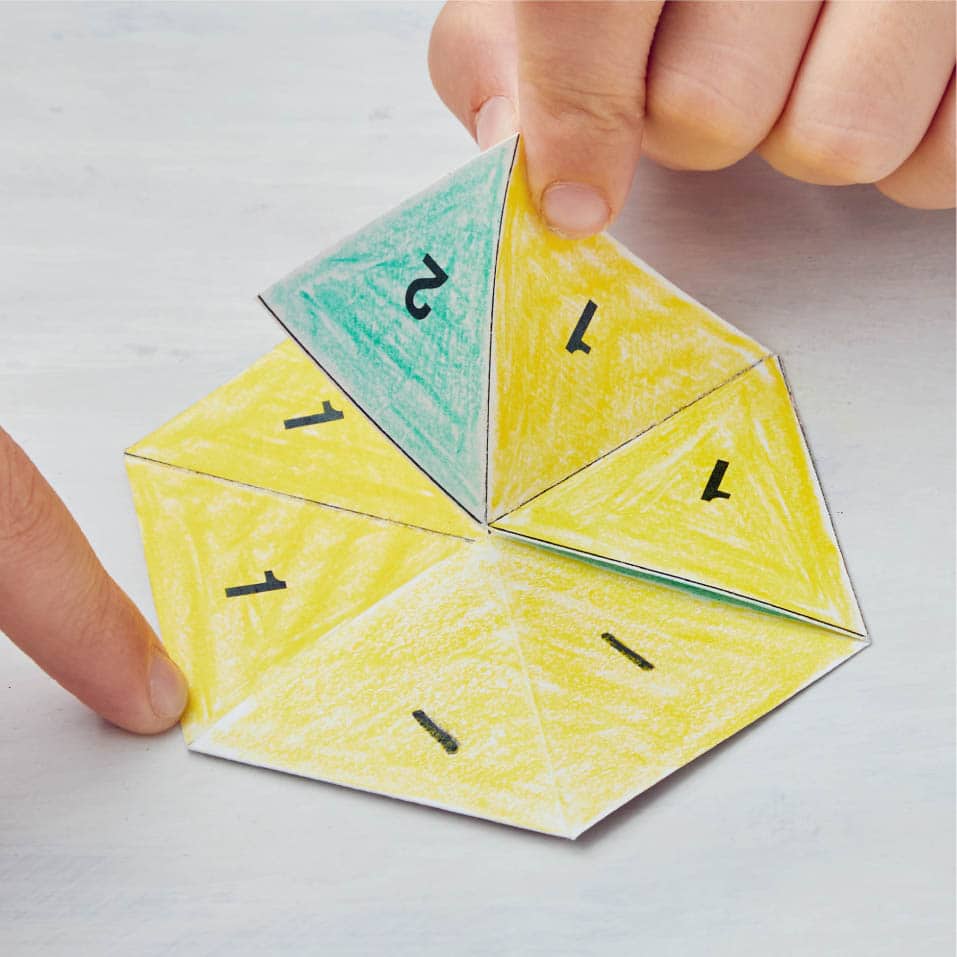
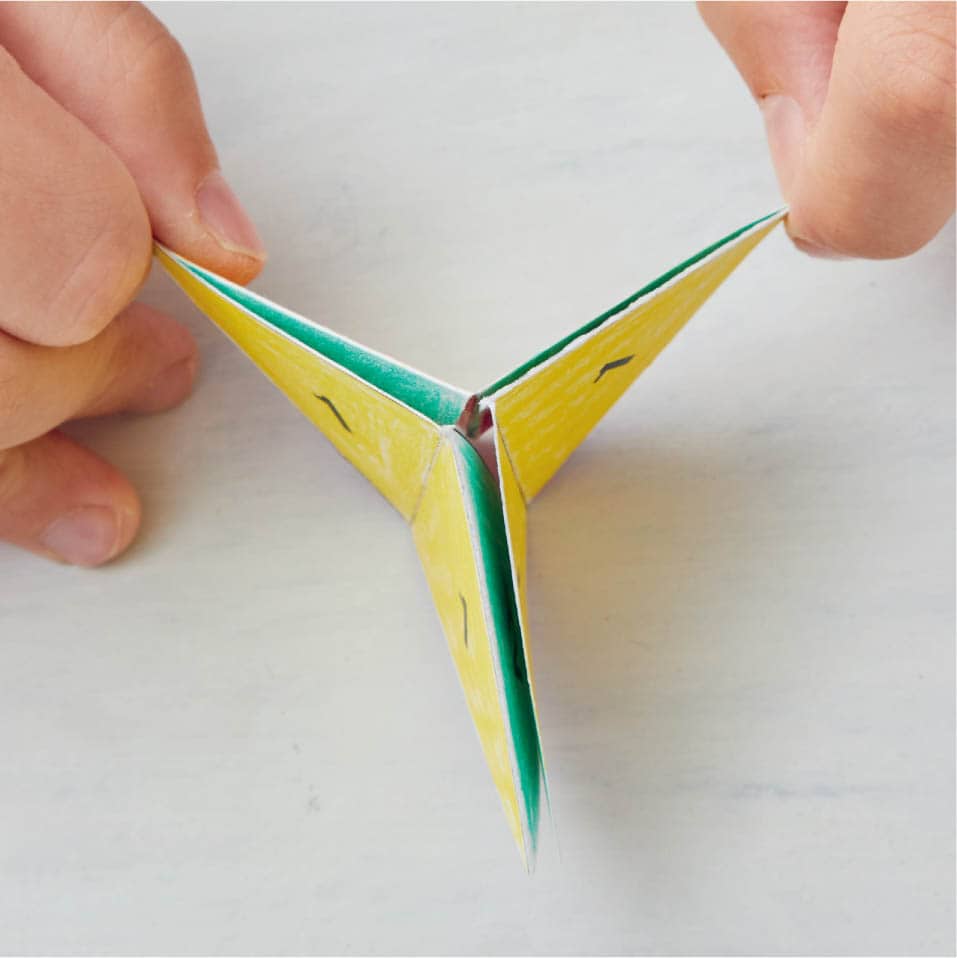
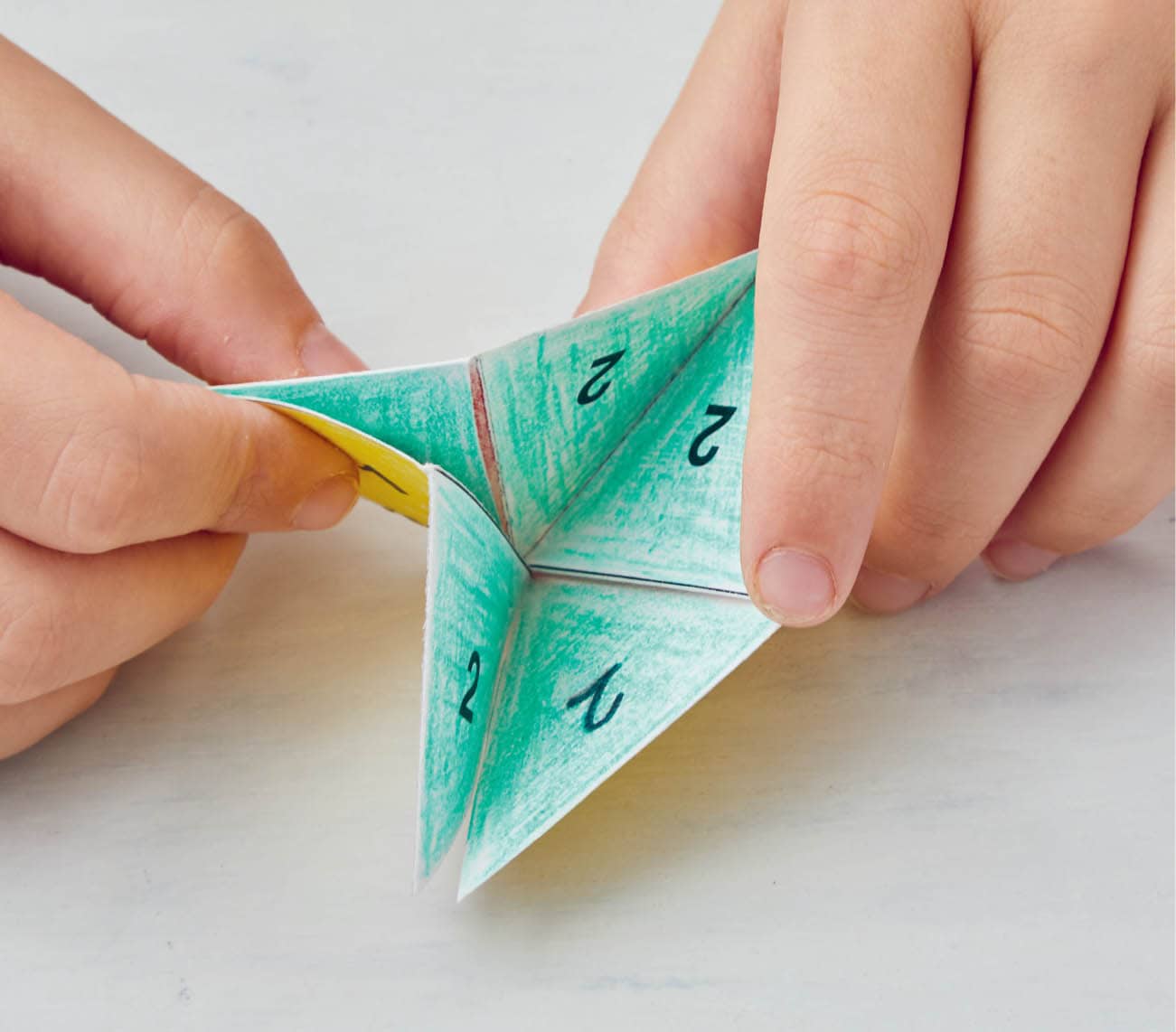
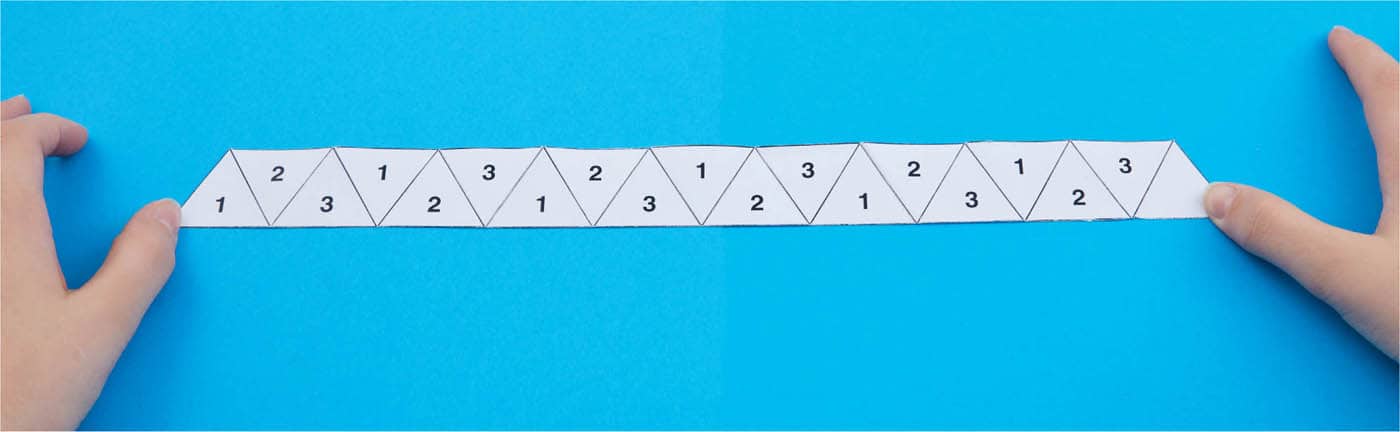
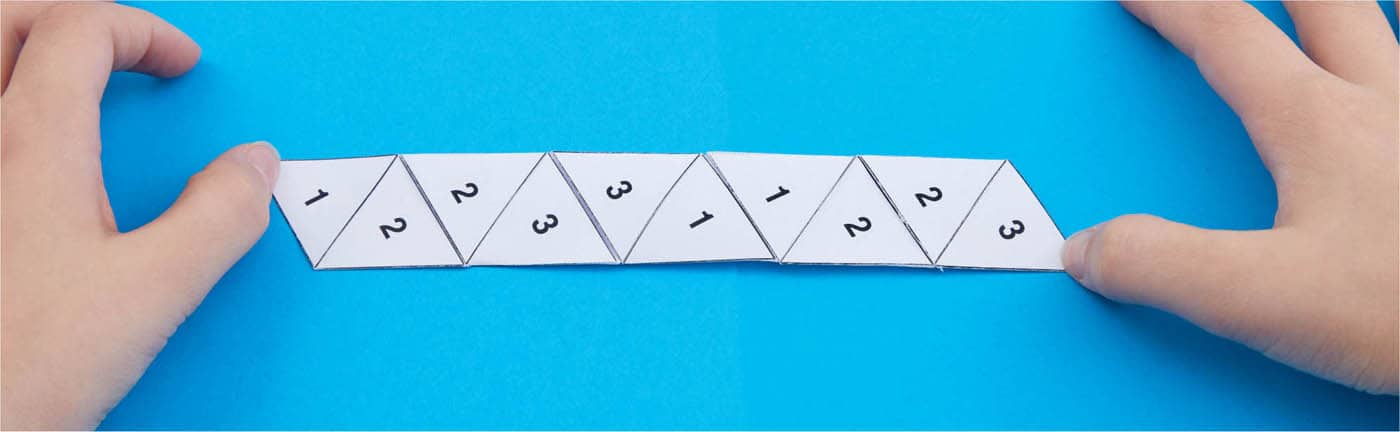
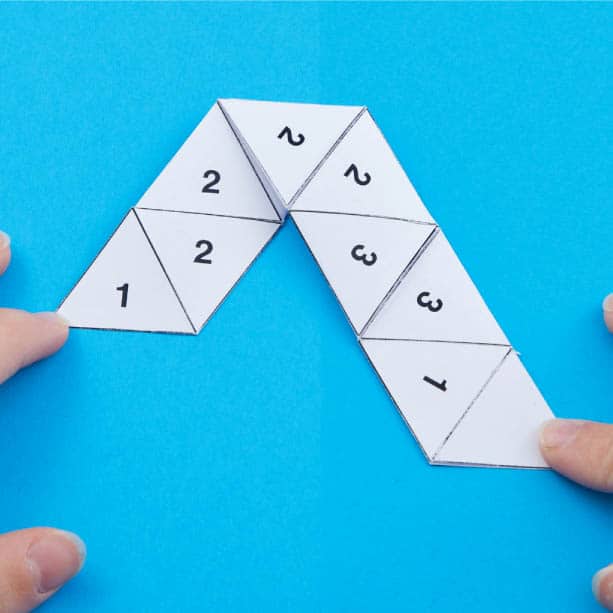
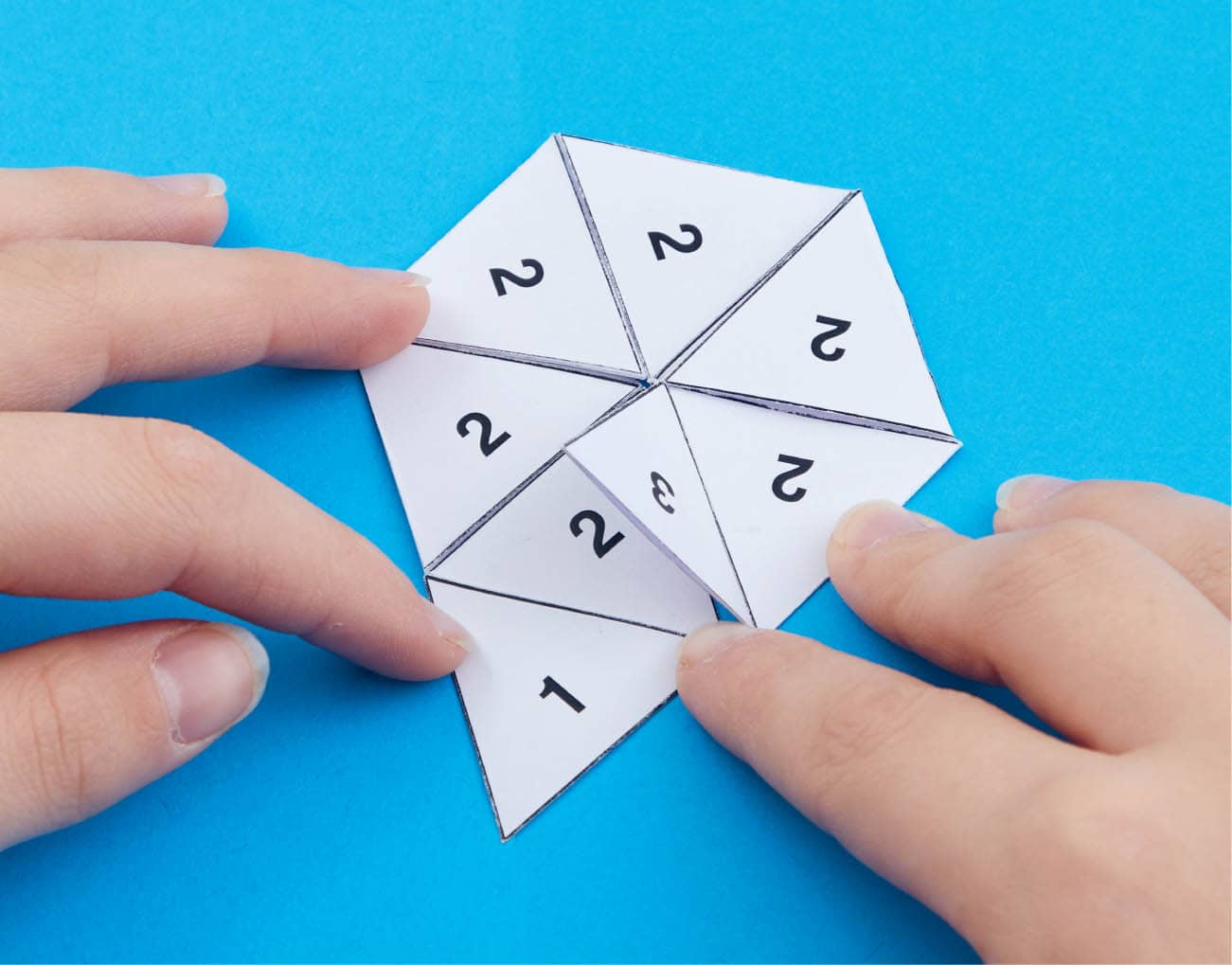
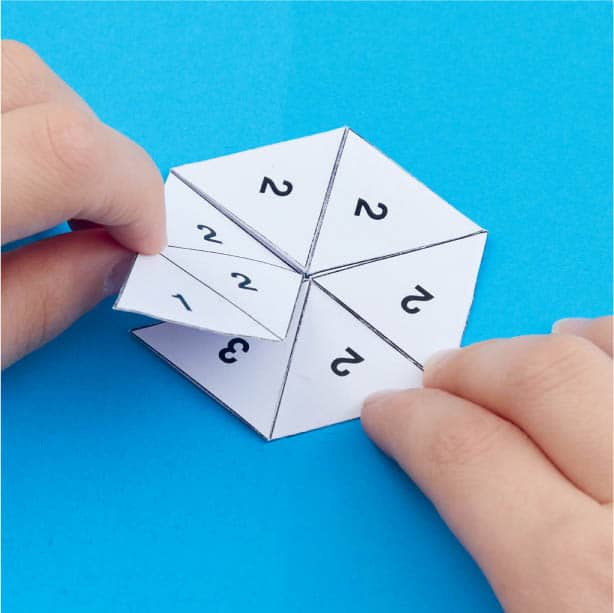
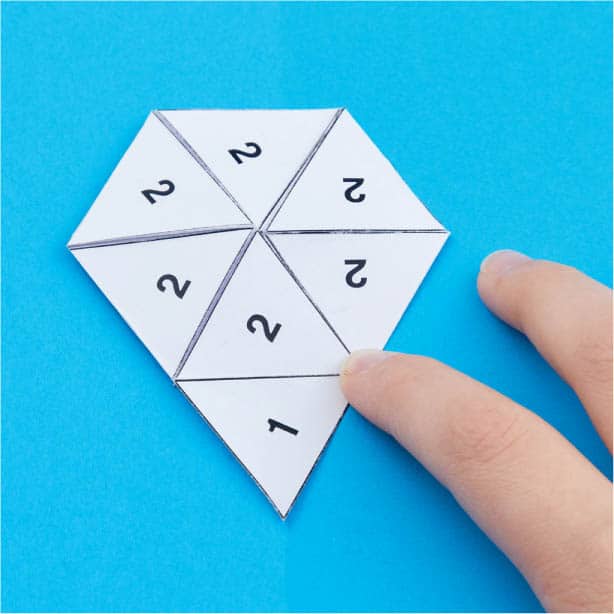
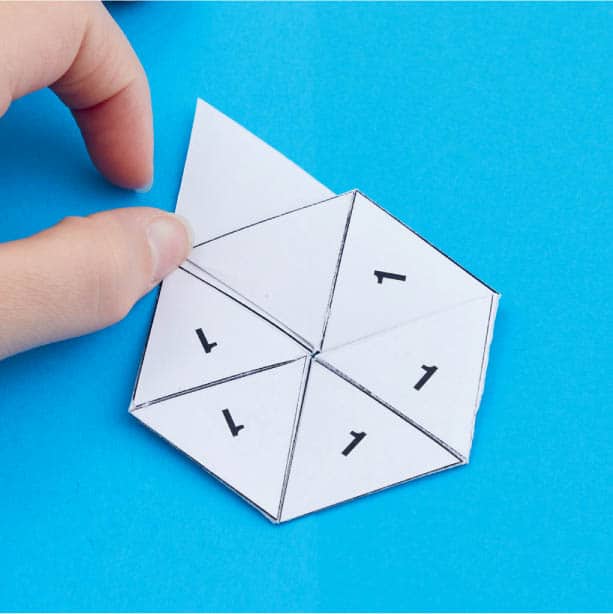
Flexagons are interesting shapes that can be folded and bent to reveal previously hidden aspects. In this activity, we’ll make a trihexaflexagon, which is a shape that looks like a hexagon but has three sides! 1 Copy the Trihexaflexagon template from the book. You’ll get best results with thicker paper or cardstock. (It can also be downloaded from www.mathlabforkids.com and https://quarto.com/files/MathForKids.) 2 With craft knife or scissors, cut out the template. If desired, color all the 1s one color, the 2s a second color, and the 3s a third color. Fig. 1. Fig. 1. Cut out the template and color all the 1s one color, the 2s a second color, and the 3s a third color. 3 Carefully fold along all the lines in both directions. It is important to crease each fold in both directions. If you have a craft knife, you’ll get best results if you have an adult very lightly score along each line using a ruler to keep the lines straight. 4 Turn the strip around and label the triangles 3-3-2-2-1-1-3-3-2 from left to right. Make sure the leftmost 3 has a 2 on the other side and the rightmost 2 has a 1 on its other side. If you colored the triangles in step 2, use the same colors for the numbers you used on the other side. The second part of the template shows you the order to label the other side too. Fig. 2. 5 Flip the strip back over to the printed side, then fold so that you have four 1s next to each other. Fig. 3. 6 Fold the strip so that you can see all six 1s with the 2 on top. Fig. 4. 7 Tuck the triangle labeled 2 behind the triangle labeled 1 that would otherwise be below it. Fig. 5. 8 Tape the top of the triangle labeled 1 to the triangle labeled 2 that is tucked behind it. Fig. 6. 9 You will now have all 1s on the front and all 3s on the back. Fig. 7 and Fig. 8. 1 Now comes the fun part: flexing! Push the creases between the two 1s you labeled by hand and the other two adjacent 1s all toward each other. Fig. 9 and Fig. 10. 2 Pull the tips of the tops of the triangles out from the center. Fig. 11 and Fig. 12 (on this page). 3 You have now revealed the third face of the trihexaflexagon! Fig. 13. 4 You can repeat steps 1 and 2 as often as you want to cycle through all three faces. If after pushing in all the creases as in step 1, the shape won’t open up as in step 2, try pushing in a different set of adjacent triangles instead.Emmy Noether
TRAILBLAZER
CONTROVERSY
NOETHER’S FIRST FAMOUS THEOREM
MORE GROUNDBREAKING WORK
UNUSUAL TEACHING STYLE
MOVE TO THE UNITED STATES
FABULOUS FOLDING FLEXAGONS
MATERIALS
DIRECTIONS
CONSTRUCT A TRIHEXAFLEXAGON
FLEX YOUR TRIHEXAFLEXAGON
October 21, 1914 – May 22, 2010 Martin Gardner never took a college math class and didn’t consider himself a mathematician. Despite that, mathematicians proudly claim Martin as one of their own because he did more than anyone else in the twentieth century (and maybe ever) to popularize and explain math to nonmathematicians. Persi Diaconis (also featured in this book) joked, “Warning: Martin Gardner has turned dozens of innocent youngsters into math professors and thousands of math professors into innocent youngsters.” Martin hung out with some of the most famous people of the twentieth century—writers, artists, Nobel Prize winners, world-famous magicians, and, of course, mathematicians. Martin started as a writer/editor of children’s magazines and particularly enjoyed paper-folding puzzles. He once wrote a column for Scientific American (SA), a widely read popular science magazine, about hexaflexagons. That column was such a success that the editor asked, “Is there enough similar material . . . to make a regular feature?” The answer was a resounding yes! For twenty-five years, Martin wrote a monthly column in SA called “Mathematical Games.” Many famous scientists, such as Albert Einstein, have written articles for SA, but Martin’s column was the magazine’s most popular section for many years. His column was so widely read that he sometimes made people famous just by writing about them. For example, M. C. Escher, now one of the twentieth century’s most famous artists, was basically unknown until Martin included his work in a column. (You can read more about Escher’s work in the section on Jennifer McLoud-Mann.) Martin was fascinated by magic. His first publication, when he was only fifteen years old, was a magic trick in the official magazine of the Society of American Magicians. He helped pay his University of Chicago tuition by performing magic tricks at a department store. Martin’s book The Encyclopedia of Impromptu Magic is highly respected among magicians and his book about mathematical magic tricks, Mathematics, Magic, and Mystery, is still a classic. Magic magazine recognized Martin as one of the “100 Most Influential Magicians of the Twentieth Century.” Fittingly, his last published article was also a magic trick. Martin was a famous skeptic of all forms of pseudoscience. He is credited with launching the modern skeptical movement with his book Fads and Fallacies in the Name of Science. The famed evolutionary biologist and history of science writer Stephen Jay Gould called Martin “The Quack Detector” who “expunged nonsense [and was] a priceless national resource.” Martin wrote or edited more than a hundred books and countless articles, columns, and reviews. In addition to books and articles on math, magic, and skepticism, he wrote two novels. Martin’s most famous and best-selling book, which sold over a million copies, was The Annotated Alice. It included the original illustrations and entire text of Lewis Carroll’s Alice’s Adventures in Wonderland and Through the Looking-Glass, along with extensive commentary explaining mathematical concepts, wordplay, literary references, games, and customs of the time in which Carroll wrote the books. Academics had been writing annotated versions of books meant to be read by other scholars for a long time, but The Annotated Alice was the first annotated book aimed at all readers. It was such a success that it spawned a whole field of annotated books written for the general public. Martin wrote several more, including annotated versions of famous poems “Casey at the Bat” and “The Night Before Christmas.”
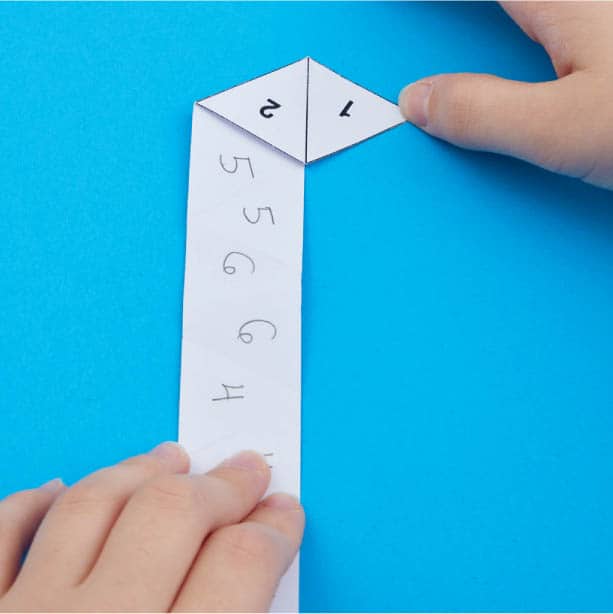
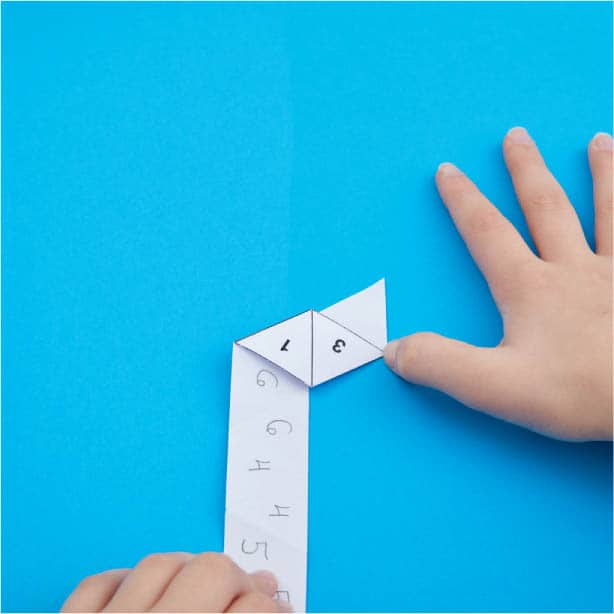
We’ll make an object that will look just like the trihexaflexagons we made earlier but it has six sides instead of three! NOTE: We strongly recommend you do the Fabulous Folding Flexagons activity before you do this one. 1 Copy the template from the book onto paper. It can also be downloaded from www.mathlabforkids.com and https://quarto.com/files/MathForKids. 2 With a craft knife or scissors, cut out the template. If desired, color all the 1s one color, the 2s a second color, and the 3s a third color. The nineteenth triangle doesn’t have a number on it because it won’t be visible in the final hexahexaflexagon, so there is no need to color it. Fig. 1. 3 Carefully fold along all the lines in both directions. It is important to crease each fold in both directions. If you have a craft knife, you’ll get best results if you have an adult very lightly score along each line using a ruler to keep the lines straight. 4 Flip the strip over. This side will have the first triangle blank instead of the last. The rest will be labeled 4-4-5-5-6-6 from left to right repeated three times. Make sure the blank triangle on this side has a 1 on the other side. Make sure the blank triangle on the other side has a 6 on this side. The last part of the template shows you the order to label the other side. If you colored the triangles in step 2, use three new colors for this side so you are using a total of six colors. Fig. 2. 5 Wind the long strip around itself to make a shorter strip by having the back numbers face their partner. Fold the first 4 to face the second 4. Fig. 3 and Fig. 4. 6 Fold the first 5 to face the second 5. Fig. 5. 7 Fold the first 6 to face the second 6. 8 Keep going around until you have a straight strip of ten triangles. On one side it will read 1-2-2-3-3-1-1-2-2-3. On the other side, it will start with a blank triangle, then read 3-1-1-2-2-3-3-1 and then end with a blank triangle. Fig. 6 and Fig. 7. 9 Hold the folded strip with side 1 face up so you see no blank triangles and have a 1 on the left. Fold the 3s that are next to each other (the fourth and fifth triangles from the left) together so you have four 2s visible next to each other. Fig. 6 and Fig. 8. 10 Now fold the two visible 3s on top of each other. Fold so you can see all six 2s with the 3 on top. Fig. 9 (on this page). 11 Tuck the newly visible 3 under the last 2 that is currently under that 3. Fig. 10. 12 You will now see all six 2s and have an extra triangle with a 1 sticking out. Fig. 11. 13 Flip the whole contraption over and you’ll see two blank triangles. Fig. 12. 14 Glue the blank triangles together. You’ll end up with all 1s on one side and all 2s on the other. 15 Try flexing the same way you flexed the trihexaflexagon. There are six sides, but some are easier to find than others. It took us a couple of minutes to find all six sides, so don’t give up! If you can’t find all six sides, try pushing in a different set of adjacent triangles instead. Fig. 13. 16 Decorate your hexahexaflexagon to make it even more interesting!Martin Gardner
MATH POPULARIZER
MATHEMATICAL GAMES
MARTIN THE MAGICIAN
SKEPTIC
OTHER WRITING
A HEAP OF HEXAGONS
MATERIALS
DIRECTIONS