The Jordan Curve Theorem Is Nontrivial
FIONA ROSS AND WILLIAM T. ROSS
1. Introduction
The classical Jordan curve theorem (JCT) says,
Every Jordan curve (a non-self-intersecting continuous loop in the plane) separates the plane into exactly two components.
It is often mentioned just in passing in courses ranging from liberal arts mathematics courses, where it is an illuminating example of an “obvious” statement that is difficult to prove, to undergraduate and graduate topology and complex analysis, where it tends to break the flow. In complex analysis, it is especially given short shrift. There are several reasons for this short shrift. For one, a professor has bigger fish to fry. There are the theorems of Cauchy, Hadamard, Morera, and the like, which comprise the nuts and bolts of complex analysis, and so the Jordan curve theorem appears to be a mere curiosity. Second, there is insufficient time to even outline a proof that an uninitiated student can really appreciate. Third, the result is clearly “obvious,” and professors do not want to put themselves, or their students, through a complicated proof of a theorem which seems to need no proof at all.
A complex analysis teacher who recognizes the need to at the least mention the JCT might hastily draw a circle or an ellipse on the board to point out the interior and exterior regions—knowing full well that the students are not impressed. The slightly more ambitious teacher might make some weak attempt (without practicing this before class) at drawing something more complicated and invite the students to identify the interior and exterior regions. The students always do so quickly and are still not impressed and don’t see the real difficulties. Maybe the teacher gives a slightly more formal “proof” of the JCT:
Start from a point not on the curve and draw a straight line from that point to the outside of the whole drawing. If the line meets the curve an odd number of times, you are on the interior. If the line meets an even number of times, you are on the exterior.
For most curves a (nonartist) teacher might draw in class, this “counting crossings” method is relatively easy, and students still don’t see the real difficulties or why this is not really a valid proof of the JCT. The Jordan curve theorem lesson usually ends with a few mumbled words like, “Well, trust me on this one. Things can get pretty complicated out there, and to make this all exact takes a lot of work. So, let’s move on to …”
We certainly understand the issues mentioned above in teaching the Jordan curve theorem and the need to move on to more relevant topics. We write this note for those who wish to appreciate both the artistic exploration and the history behind the JCT. Thus we unite two lines of discourse on the nature of nontriviality. In our opinion, the JCT is a wonderful result because it exposes us to amazing, pathological, counterintuitive examples, such as nowhere differentiable curves or curves with positive area (see below). So, in a way, not appreciating the JCT is driven by a lack of imagination, in thinking that Jordan curves are nothing more than circles or a couple of wavy lines a teacher hastily draws on a blackboard.
The JCT not only inspires mathematicians to dream up these fantastical examples, which almost mock the technical definition of a curve, but it also inspires artists. The open curve artwork of Mø Morales (see http://virtualmo.com) and the maze art of Berg [1] are well known and much admired. Kaplan [10] and Pedersen and Singh [12] have developed algorithms for computer-generated mazes and labyrinths. Perhaps most relevant are the computer-generated Jordan curve artwork of Bosch [2] and the TSP art of Bosch and Kaplan [3]. Before becoming familiar with these artists’ work, the first author (Fiona) made the drawings described here to show that Jordan curves are not the cold, abstract, boring objects we might think they are. Instead, they can tell a story. They can help mathematicians make a better case to their students, and to themselves, that there is something nontrivial going on here—indeed, something beautiful. We see through some hand-drawn artwork that the interior and exterior regions guaranteed by the JCT are not so easy to identify, and when they are identified, they can be just wonderful to look at.
Just as curves can be used to tell a story, the proof of the JCT is a great story by itself—from the Bohemian monk who had to convince us that the JCT needed a proof, to the Frenchman who gave us an unconvincing one, to the mathematicians who opened up the Pandora’s box of terrifying pathological examples of curves, to the final proof, to Jordan’s redemption. The artwork inspired by the JCT, and how it can inspire mathematicians and teachers, as well as the history of the result, are illuminated here.
2. The Artwork
The black-and-white hand drawings that are Jordan curves drawn by the first author (Figures 1 and 2), were created to give the reader an opportunity to explore the idea of a curve as leading the viewer through a story and also to invite the reader to apply the “proof” of the JCT mentioned earlier (counting the number of crossings needed to exit the drawing) to determine which parts of the figures lie in the interior or exterior. As a visual artist, the first author has always found inspiration in the way curves appear in the structure of the natural world. The curves that appear in geology and biology, such as anafractuous (twisting and turning), concentra, labyrinthine, phyllotaxy (dynamic spirals), vermiculate (wormlike arrangements), and ripple and dune formations have provided a starting point for many of her artworks. In exploring the physical and metaphorical structure of unicursal labyrinths, the first author created a series of self-portraits in landscapes rendered from a single line. The most famous of unicursal, or single path, labyrinths features prominently in the mythological tale of Theseus and the Minotaur. Labyrinth forms are also used as guides to meditation and prayer, giving the person who travels the labyrinth a sense of their very selves as the most complex of labyrinths.
The authors began a discussion of the JCT after the second author (William) saw one of the first author’s unicursal labyrinths (Blue Introverted Unicursal Labyrinth, Figures 3 and 4). In this drawing, the first author made a point of not uniting the curve that formed the labyrinth. Just as there is no metaphorical separation between the journey and the traveler, there was, deliberately, no separation of the interior or exterior of the labyrinth on the paper. Intrigued by the conversations with the first author, who is a mathematician, and by Felix Klein’s statement,

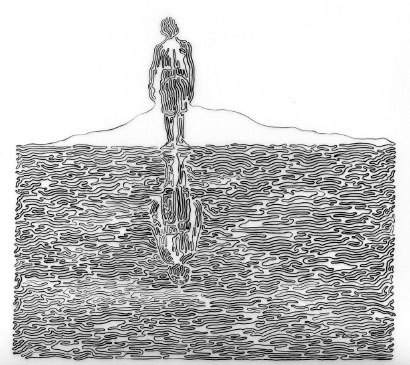
FIGURE 1. Fiona Ross, When We Could Be Diving for Pearls, 9¾″ × 6″, 2011, Micron ink on Denril paper.
Everyone knows what a curve is, until he has studied enough mathematics to become confused through the countless number of possible exceptions,
the first author combined the idea of a curve leading the viewer through a meaningful story together with the mathematical notion of the complexities encountered when trying to prove the JCT. She began a new series of drawings (When we could be diving for pearls (Figure 1) and A thread in the labyrinth (Figure 2) rendered from a single (nonintersecting) curve without beginning or end, i.e., a Jordan curve. In these new drawings, the unicursal labyrinth is no longer open, as in the earlier Blue Introverted Unicursal Labyrinth (Figures 3 and 4), but closed. The interior and exterior spaces in the new drawings are difficult to identify. Making it difficult to identify inside and outside spaces gives the “closed” drawings in Figures 1 and 2 a different meaning than the “open” drawings of Figures 3 and 4. The Jordan curve drawings have a more secretive content. They seem to hold their figurative breath as the inked fingers of the external space unsuccessfully reach in and touch the interior, whereas the non-Jordan curve drawings invite entry into the most remote areas of the work. The first author finds both the forms of content (open and closed) interesting to work with and is grateful to be given the opportunity to point out where art and mathematics intersect. The Jordan curve drawings specifically created for this article (Figures 1 and 2) were hand drawn with Micron ink markers or graphite on Denril drafting film.
FIGURE 2. Fiona Ross, A Thread in the Labyrinth, 6″ × 6″, 2011, Micron ink on Denril paper.

FIGURE 3. Fiona Ross, Blue Introverted Unicursal Labyrinth, 14″ × 8″, 2010, Ink on Yupo paper.
3. A Historical Perspective of the Jordan Curve Theorem
Just as the JCT artwork in the previous section shows that curves can tell a tale, the JCT itself is an interesting story. The JCT says that the plane is separated into two components by a Jordan curve.
We traditionally call the bounded component the “interior” and the unbounded component the “exterior.” Bernard Bolzano [8,13] realized that the problem was nontrivial and officially posed it as a theorem needing a proof. Here is Bolzano’s “prophetic version of the celebrated Jordan curve theorem” (8, p. 285):

FIGURE 4. Blue Introverted Unicursal Labyrinth, detail.
If a closed line lies in a plane and if by means of a connected line one joins a point of the plane which is enclosed within the closed line with a point which is not enclosed within it, then the connected line must cut the closed line.
Bolzano also realized that the current notions of curve at the time were in desperate need of proper definitions! The first proof, and hence the name Jordan curve theorem, was given in 1887 by Camille Jordan in his book Cours d’analyse de l’École Polytechnique [9] but was regarded by many to be incorrect. As an interesting side note, Thomas Hales [7] believes that the charges of incorrectness against Jordan’s original proof were trumped up and that the errors are merely aesthetic. With a few relatively minor changes, he claims to make Jordan’s proof rigorous.
If the Jordan curve is a polygon, one can prove the JCT using the “counting crossings” method mentioned earlier. This proof, once cleaned up a bit to make it completely rigorous, is the standard way to prove the JCT for polygons.
When the curve is not a polygon, all sorts of pathologies can occur. For the student, who may be reminded of defining the interior of the curve by using the rule learned from calculus: “When you travel around the curve, the interior is on your left,” it is important to notice that one is tacitly assuming that there is a continuously turning normal to the curve (your left hand). There are examples of functions f: [0, 1] → ℝ that are continuous everywhere but differentiable nowhere, i.e., Weierstrass’ famous example [5]
![]()
where 0 < a < 1, b > 1 is an odd integer, and ab > 1 + (3π/2) (Figure 5). The graph of f is jagged everywhere and can be used to define a Jordan curve that is also jagged everywhere. Such a curve does not have a well-defined tangent (and is hence normal). These types of jagged curves also serve to point out how our informal proof of the JCT for polygonal type regions (count the number of times you cross the curve) breaks down since you might cross the curve an infinite number of times when exiting the drawing, making the crossing parity (i.e., odd or even) undefined.
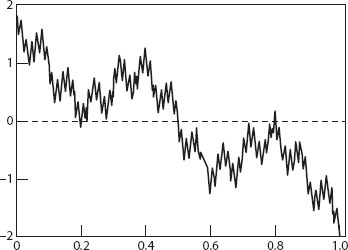
FIGURE 5. A Weierstrass nowhere differentiable function.
Even more pathological is an example of Osgood [11] of a Jordan curve with positive area. Jordan showed that if the curve is rectifiable (of finite length), then it has zero area. These positive-area Osgood curves are quite wild and fascinating, and the reader is directed to a wonderful Mathematica Demonstration by Robert Dickau [4], where one constructs a Jordan curve with any desired (finite) area.
Otto Veblen [15] finally gave what many regard as the first correct proof of the Jordan curve theorem, and others have followed with different proofs, including ones using formal logic [6].
4. Final Thought
In this article, we have seen that a Jordan curve is much more than a circle or ellipse. It can be jagged at every point or perhaps impossible to visually determine the interior from the exterior or perhaps even of positive area, and, as seen through the artwork in this article, can lead the viewer through a story. In fact, the Jordan Curve Theorem is a wonderful story in itself—a seemingly obvious result, which Bolzano had to convince people was not and whose original proof was not convincing and which inspired mathematicians to create pathological, counterintuitive, and fascinating examples. It is then fitting that Jordan curves inspire art and, more importantly, help mathematicians make a better case to the students that the JCT is non-trivial both as a mathematical result and as a work of art.
References
[1] C. Berg, Amazing Art: Wonders of the Ancient World. Harper Collins, New York, 2001.
[2] R. Bosch, Simple-closed-curve sculptures of knots and links, J. Math. Arts 4 (2010), pp. 57–71. http://www.tandfonline.com/doi/abs/10.1080/17513470903459575#.UYQMp0pTn0U.
[3] R. Bosch and C. Kaplan, TSP Art, Bridges Conference Proceedings 2005, 303–310, http://www.cgl.uwaterloo.ca/˜csk/projects/tsp/.
[4] R. Dickau, Knopp’s Osgood Curve Construction, Wolfram Demonstrations Project, http://demonstrations.wolfram.com.KnoppsOsgoodCurveConstruction (user needs the Mathematica player installed to see this demonstration.
[5] B. R. Gelbaum and M. H. J. Olmsted, Counterexamples in analysis, Corrected reprint of the second (1965), Dover Publications Inc., Mineola, N.Y., 2003.
[6] T. C. Hales, The Jordan curve theorem, formally and informally, Amer. Math. Monthly 114 (2007), pp. 882–894.
[7] T. C. Hales, Jordan’s proof of the Jordan curve theorem, Studies in Logic, Grammar and Rhetoric 10 (2007), pp.45–60.
[8] D. M. Johnson, Prelude to dimension theory: the geometrical investigations of Bernard Bolzano, Arch. History Exact Sci. 17 (1977), pp. 262–295.
[9] C. Jordan, Cours d’analyse de l’École Polytechnique, Gauthier-Villars, Paris, 1887.
[10] C. Kaplan, Vortex maze construction, J. Math. Arts 1 (2007), pp. 7–20.
[11] W. F. Osgood, A Jordan curve of positive area. Trans. Amer. Math. Soc. 4(1) (1903), pp. 107–112.
[12] H. Pedersen and K. Singh, Organic labyrinths and mazes, NPAR Conference Proceedings, (2006), 79–86, http://dx.doi.org/10.1145/1124728.1124742.
[13] B. B. Van Rootselaar, Dictionary of Scientific Biography; Vol. II, Charles Scribners Sons, New York, 1970, pp.273–279.
[14] H. Sagan, A geometrization of Lebesgue’s space-filling curve, Math. Intelligencer 15 (1993), pp. 37–43.
[15] O. Veblen, Theory on plane curves in non-metrical analysis situs, Trans. Amer. Math. Soc. 6 (1905), pp. 83–98.