
3.2. ARC LENGTH AND SURFACE AREA 85
Problem 3.18 For each of the following pairs of functions, compute the volume obtained by
rotating the area between the functions about the y-axis.
1. f .x/ D 7x
3
and g.x/ D 28x
2
2. f .x/ D 4x
6
and g.x/ D 64x
2
3. f .x/ D 2x
4
and g.x/ D 18x
2
4. f .x/ D 3x
6
and g.x/ D 3x
2
5. f .x/ D 7x
5
and g.x/ D 21x
4
6. f .x/ D 2x
3
and g.x/ D 8x
Problem 3.19 Using the method of disks, rotating about the x-axis, verify the formula for the
volume of a cone of radius R and height H :
V D
1
3
R
2
H
3.2 ARC LENGTH AND SURFACE AREA
In this section we will learn to compute the length of curves and, having done that, to find the
surface area of figures of rotation.
A piece of a curve is called an arc. e key to finding the length of an arc is the differ-
ential of arc length.
In the past we have had quantities like dx and dy that measure infinitesimal changes in
the directions of the variables x and y.
e differential of arc length is different – it does not point in a consistent direction,
rather it points along a curve and so, by integrating it, we can find the length of a curve.
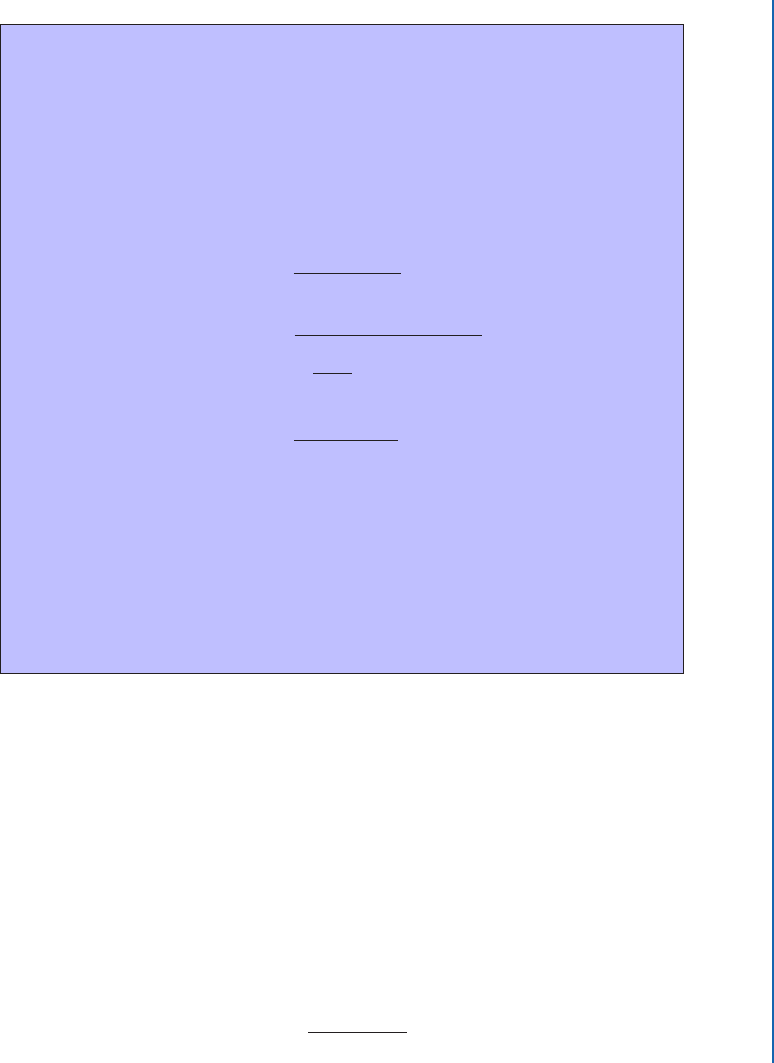
Examine Figure 3.5. e relationship between the change in x and y and the change in the
length of the curve is Pythagorean, based on a right triangle.
If we take this relationship to the infinitesimal scale, we obtain a formula for the differ-
ential of arc length.

86 3. ADVANCED INTEGRATION
4
-1
-2
4
y=f(x)
x
y
s
Figure 3.5: e triangle with sides x, y, and s shows how the change in the length of the
curve is related to the changes in distance in the x and y directions.
3.2. ARC LENGTH AND SURFACE AREA 87
Knowledge Box 3.5
e differential of arc length and arc length
If y D f .x/ is a continuous curve, then the rate at which the length
of the graph of f .x/ changes is called the differential of arc length,
denoted by ds. e value of ds is:
ds
2
D dy
2
C dx
2
ds D
p
dy
2
C dx
2
D
s
dy
2
dx
2
C 1
dx
2
D
q
.
y
0
/
2
C 1 dx:
e length S of a curve (arclength of the curve) from x D a to x D b is:
S D
Z
b
a
ds:
Example 3.20 Find the length of y D 3x
2=3
from x D 1 to x D 8.
Solution:
e first step in an arc length problem is to compute ds.
y D 3x
2=3
y
0
D 2x
1=3
ds D
p
4x
2=3
C 1 dx

88 3. ADVANCED INTEGRATION
is means that the desired length is
S D
Z
8
1
ds
D
Z
8
1
r
4
x
2=3
C 1 dx
D
Z
8
1
s
4 Cx
2=3
x
2=3
dx
D
Z
8
1
p
4 Cx
2=3
dx
x
1=3
Let u D 4 C x
2=3
, then du D
2
3
x
1=3
dx D
2
3
dx
x
1=3
So,
3
2
du D
dx
x
1=3
.
Applying the substitution to the limits we see that the integral goes from u D 5 to u D 8.
Transforming everything to u-space, the arc length is:
S D
Z
8
5
p
u
3
2
du
D
3
2
Z
8
5
u
1=2
du
D
3
2
2
3
u
3=2
ˇ
ˇ
ˇ
ˇ
8
5
D 8
3=2
5
3=2
Š 11:45 units
2
˙
Alert students will have noticed that the function chosen to demonstrate arc length is not one
of our usual go-to functions for demonstration. is is because the formula for ds yields some
very difficult integrals. e next example is one such, but yields a formula we already know how
to integrate.

3.2. ARC LENGTH AND SURFACE AREA 89
Example 3.21 Find the arc length of y D x
2
from x D 0 to x D 2.
Solution:
Since y
0
D 2x, it is easy to find that ds D
p
4x
2
C 1 dx, meaning our integral is:
S D
Z
2
0
p
4x
2
C 1 dx
is is a trig-substitution integral. e triangle for this integral is
p
4x
2
C 1
1
2x
which means our substitutions are:
p
4x
2
C 1 D sec./
2x D tan. /
x D .1=2/ tan. /
dx D .1=2/ sec
2
./ d
So we get:
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
