
108 3. ADVANCED INTEGRATION
D 2
Z
1
0
r
e
r
2
=2
dr
D 2 lim
a!1
Z
a
0
r
e
r
2
=2
dr
Let u D r
2
=2, then du D r dr
D 2 lim
a!1
Z
‹
‹
e
u
du
D 2 lim
a!1
Z
‹
‹
e
u
du
D 2 lim
a!1
e
r
2
=2
ˇ
ˇ
ˇ
a
0
D 2 lim
a!1
e
a
2
=2
1
D 2.0 1/ D 2
If A
2
D 2 then A D
p
2, which is the correct normalizing constant.
˙
3.3.1 MASS AND CENTER OF MASS
e center of mass for an object is the average position of all the mass in an object. is section
demonstrates techniques for computing the center of mass of flat plates with a density function
.x; y/. Density is the rate at which mass changes as you move through an object, which, in
turn, means that the mass of an object is the integral of its density.
3.3. MULTIPLE INTEGRALS 109
Knowledge Box 3.10
Mass of a plate
Suppose that a flat plate occupies a region R with a density function
.x; y/ defined on R. en the mass of the plate is
M D
Z Z
R
.x; y/ dA:
Remember that the function .x; y/ is usually constant, or close enough to constant that we
assume it to be constant, when we have a mass made of a relatively uniform material. e fairly
high variation in the mass functions in the examples and homework problems is intended to give
your integration skills a workout – not as a representation of situations encountered in physical
reality.
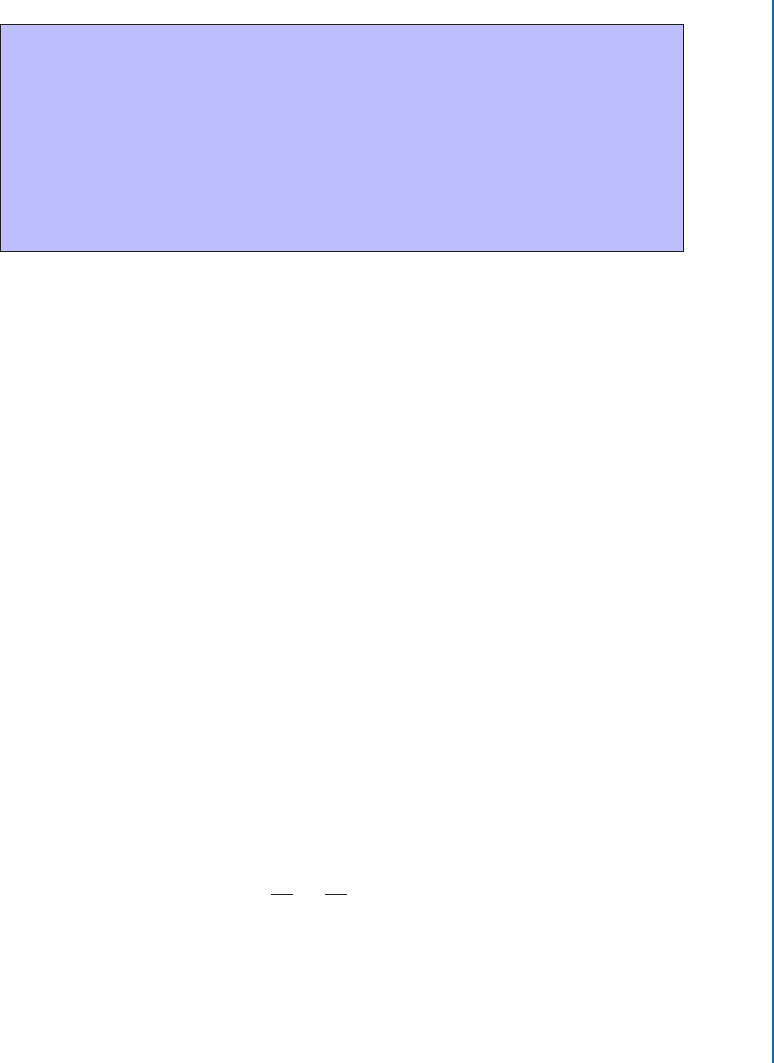
Example 3.43 If a plate fills the triangular region R from Figure 3.8 with a density function
.x; y/ D x C1 grams/unit
2
, find the mass of the plate.
Solution:
Using the mass formula, the integral is
Mass D
Z
2
0
Z
x
0
.
x C 1
/
dy dx
D
Z
2
0
.
xy C y
/
ˇ
ˇ
ˇ
ˇ
x
0
dx
D
Z
2
0
x
2
C x
dx
D
x
3
3
C
x
2
2
ˇ
ˇ
ˇ
ˇ
2
0
D 8=3 C 2 0 0 D 14=3 g
˙
110 3. ADVANCED INTEGRATION
Once we have the ability to compute the mass of a plate from its dimensions and density, we
can compute the coordinates of the center of mass of the plate using a type of averaging integral.
Knowledge Box 3.11
Center of mass
Suppose that a flat plate occupies a region R with a density function
.x; y/ defined on R. en if
M
x
D
Z Z
R
y.x; y/ dA
and
M
y
D
Z Z
R
x.x; y/ dA
the center of mass of the plate is
.x; y/ D
M
y
M
;
M
x
M
:
-2
-2
2
2
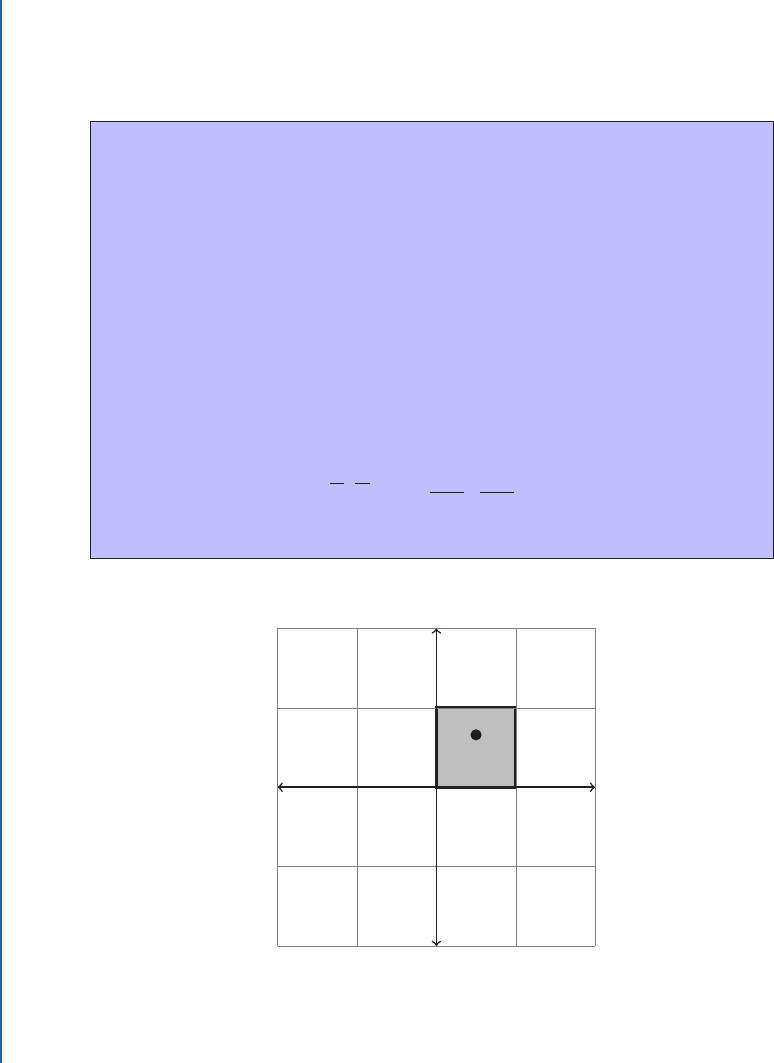
Figure 3.10: Center of mass of square region when .x; y/ D 2y g/unit
2
.

3.3. MULTIPLE INTEGRALS 111
Example 3.44 Suppose that R is the square region
0
x; y
1
and that .x; y/ D 2y g/unit
2
as shown in Figure 3.10.
Find the center of mass.
Solution:
is problem requires three integrals.
M D
Z
1
0
Z
1
0
2y dy dx M
x
D
Z
1
0
Z
1
0
y 2y dy dx M
y
D
Z
1
0
Z
1
0
x 2y dy dx
D
Z
1
0
y
2
ˇ
ˇ
ˇ
ˇ
1
0
dx D
Z
1
0
Z
1
0
2y
2
dy dx D
Z
1
0
xy
2
ˇ
ˇ
ˇ
ˇ
1
0
dx
D
Z
1
0
.1 0/dx D
Z
1
0
2
3
y
3
ˇ
ˇ
ˇ
ˇ
1
0
dx D
Z
1
0
.x 0/ dx
D
Z
1
0
dx D
Z
1
0
2
3
0
dx D
1
2
x
2
ˇ
ˇ
ˇ
ˇ
1
0
D
1
2
D x
ˇ
ˇ
ˇ
ˇ
1
0
D
Z
1
0
2
3
dx
D .1 0/ D 1 gram D
2
3
x
ˇ
ˇ
ˇ
ˇ
1
0
D
2
3
Now that we have the pieces we can use the formula for center of mass:
.x; y/ D
1=2
1
;
2=3
1
D
1
2
;
2
3
˙

112 3. ADVANCED INTEGRATION
PROBLEMS
Problem 3.45 Find the integral of each of the following functions over the specified region.
1. e function f .x; y/ D x Cy
2
on the strip
0 x 4 0 y 1:
2. e function g.x; y/ D xy on the rectangle
1 x 3 1 y 2:
3. e function h.x; y/ D x
2
y Cxy
2
on the square
0 x 2 0 y 2:
4. e function
r.x; y/
D
2x
C
3y
C
1
on the region bounded by
x
D
0
,
y
D
1
, and
y
D
x
.
5. e function s.x; y/ D x
2
C y
2
C 1 on the region bounded by the x axis and the function
y D 4 x
2
.
6. e function q.x; y/ D x C y on the region bounded by the curves y D
p
x and y D x
2
.
7. e function a.x; y/ D x
2
on the region bounded by the curves y D 2x and y D x
2
.
8. e function b.x; y/ D y
2
on the region bounded by the curves y D
3
p
x and y D x for
x 0.
Problem 3.46 Sketch the regions from Problem 3.45.
Problem 3.47 Explain why a density function .x; y/ can never be negative.
Problem 3.48 Find a region R so that the integral over R of f .x/ D x
2
C y
2
is 6 units
3
.
Problem 3.49 Find the square region 0 x; y a so that
Z Z
R
x
3
C y
dA
is 12 units
3
.
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
