
104 3. LIMITS, DERIVATIVES, RULES, AND THE MEANING OF THE DERIVATIVE
Problem 3.49 For which values of x does y D sin.x/ have a horizontal tangent line?
Problem 3.50 Based on the information given in this section what is:
lim
x!1
tan
1
.x/
Problem 3.51 Do either of the functions f .x/ D tan.x/ or g.x/ D tan
1
.x/ have a universal
inverse? Explain your answer.
Problem 3.52 Suppose you take the derivative of
y D sin.x/
104 times. What do you get?
Problem 3.53 Inverses of functions exist over particular parts of their domain—a universal
inverse exists everywhere in the domain. For what largest possible domains does
f .x/ D x
2
C 4x C 4
have inverses. Hint: there are two answers. Find the inverses.
3.4 THE PRODUCT, QUOTIENT, RECIPROCAL, AND
CHAIN RULES
In this section we learn the derivative rules that let us deal with functions built up out of other
functions by both arithmetic and functional composition. Our first rule lets us take the derivative
of a product of two functions. It is called the product rule.
Knowledge Box 3.12
e product rule
.
f .x/ g.x/
/
0
D f .x/g
0
.x/ C f
0
.x/g.x/

3.4. THE PRODUCT, QUOTIENT, RECIPROCAL, AND CHAIN RULES 105
Example 3.54 Find the derivative of h.x/ D x sin.x/.
Solution:
Apply the product rule to the functions f .x/ D x and g.x/ D sin.x/:
h
0
.x/ D x
.
sin.x/
/
0
C
.
x
/
0
sin.x/
D x cos.x/ C 1 sin.x/
D x cos.x/ C sin.x/:
˙
Example 3.55 Find the derivative of r.x/ D ln.x/ cos.x/.
Solution:
Apply the product rule to the functions f .x/ D ln.x/ and g.x/ D cos.x/:
r
0
.x/ D ln.x/
.
cos.x/
/
0
C
.
ln.x/
/
0
cos.x/
D ln.x/ .sin.x// C
1
x
cos.x/
D
cos.x/
x
ln.x/ sin.x/
˙
e next rule is the quotient rule which is used to deal with the ratio of two functions.
Knowledge Box 3.13
e quotient rule
f .x/
g.x/
0
D
g.x/f
0
.x/ f .x/g
0
.x/
g
2
.x/

106 3. LIMITS, DERIVATIVES, RULES, AND THE MEANING OF THE DERIVATIVE
Example 3.56 Find the derivative of h.x/ D
2x C 1
x C 5
.
Solution:
Apply the quotient rule to the functions f .x/ D 2x C 1 and g.x/ D x C 5.
h
0
.x/ D
.x C 5/.2x C 1/
0
.2x C 1/.x C 5/
0
.x C 5/
2
D
.x C 5/ 2 .2x C 1/ 1
.x C 5/
2
D
2x C 10 .2x C 1/
.x C 5/
2
D
9
.x C 5/
2
In general we do not expand the denominator after using the quotient rule. It is often easier to
deal with in factored form.
˙
Example 3.57 Find the derivative of q.x/ D
x
x
2
C 1
.
Solution:
Apply the quotient rule to the functions f .x/ D x and g.x/ D x
2
C 1.

3.4. THE PRODUCT, QUOTIENT, RECIPROCAL, AND CHAIN RULES 107
q
0
.x/ D
x
2
C 1
.x/
0
x
x
2
C 1
0
.
x
2
C 1
/
2
D
x
2
C 1
1 x .2x/
.
x
2
C 1
/
2
D
x
2
C 1 2x
2
.
x
2
C 1
/
2
D
1 x
2
.
x
2
C 1
/
2
˙
Example 3.58 Find the derivative of r.x/ D
e
x
sin.x/
.
Solution:
Apply the quotient rule to the functions f .x/ D e
x
and g.x/ D sin.x/.
r
0
.x/ D
sin.x/.e
x
/
0
e
x
.sin.x//
0
sin
2
.x/
D
sin.x/e
x
e
x
cos.x/
0
sin
2
.x/
D
e
x
.sin.x/ cos.x//
sin
2
.x/
˙
When f .x/ D
1
g.x/
, a simpler version of the quotient rule, called the reciprocal rule, may be
used.
108 3. LIMITS, DERIVATIVES, RULES, AND THE MEANING OF THE DERIVATIVE
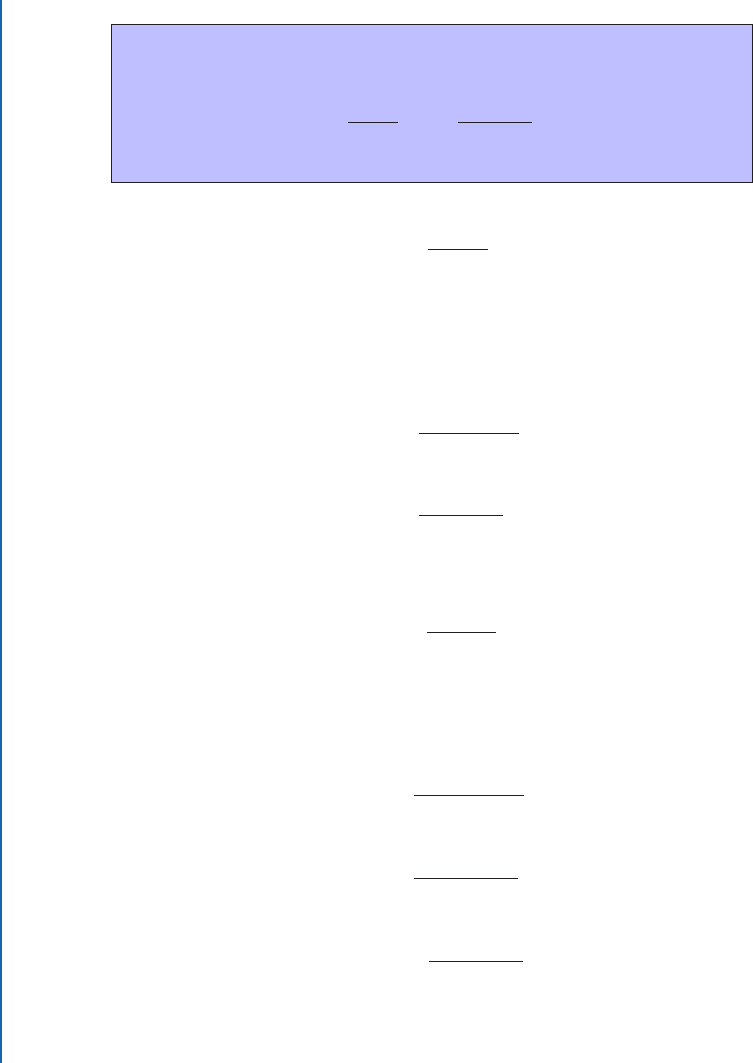
Knowledge Box 3.14
e reciprocal rule
1
f .x/
0
D
f
0
.x/
f
2
.x/
Example 3.59 Find the derivative of h.x/ D
1
x
2
C 1
.
Solution:
Apply the reciprocal rule to the function for which the denominator is f .x/ D x
2
C 1.
h
0
.x/
D
x
2
C 1
0
.
x
2
C 1
/
2
D
2x
.
x
2
C 1
/
2
˙
Example 3.60 Find the derivative of r.x/ D
1
e
x
C x
2
.
Solution:
Apply the reciprocal rule to the function for which the denominator is f .x/ D e
x
C x
2
.
q
0
.x/ D
e
x
C x
2
0
.
e
x
C x
2
/
2
D
.
e
x
C 2x
/
.
e
x
C x
2
/
2
D
e
x
C 2x
.
e
x
C x
2
/
2
˙
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
