
3.5. PHYSICAL INTERPRETATION OF DERIVATIVES 117
3.5 PHYSICAL INTERPRETATION OF DERIVATIVES
So far we have gone from the limit-based definition of a derivative to knowing how to take the
derivative of a pretty large number of different functions. e obvious missing piece here is the
meaning of the derivative. e meaning of a derivative depends a lot on context, of course, but
there is a general principle that covers most of what a derivative means.
Knowledge Box 3.16
What is a derivative?
If f .x/ measures a quantity, then f
0
.x/ is the rate at which that quan-
tity is changing.
We have been using the geometric interpretation of the derivative: it is the slope of the tangent
line to a curve at a point. A line y D ax C b represents something that starts at b and adds
a more per x-unit traversed; a line has a constant rate at which the quantity it is measuring
changes. is explains why “f
0
.x/ is the rate of change of f .x/” and “f
0
.x/ is the slope of the
tangent to the graph at x” are equivalent ideas.
At this point—to permit a number of innovative ways of using the derivative—we introduce a
new notation that acknowledges that the derivative is a rate of change.
Knowledge Box 3.17
Differential notation
Given that y D f .x/, another notation for the derivative is
dy
dx
D f
0
.x/
is is spoken “the differential of y with respect to x.” e new symbols
dy and dx are the differential of y and of x, respectively.
is notion of the derivative as a rate of change leads to a natural application in physics. We will
need one more definition.
Definition 3.3 e derivative of the derivative of a function y D f .x/ is called the second deriva-
tive and is denoted by
d
2
y
dx
2
D f
00
.x/
e second derivative measures the rate at which the rate of change is changing. Ouch. It also
measures the curvature of a graph. An example of this is shown in Figure 3.9; light hash marks
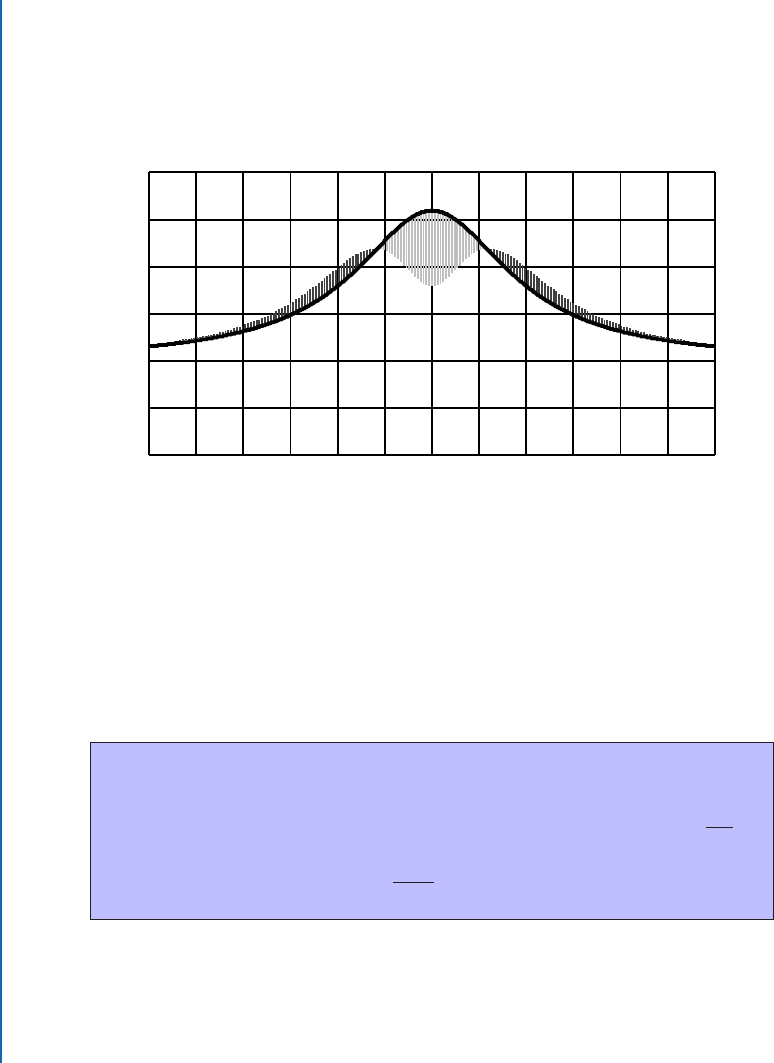
118 3. LIMITS, DERIVATIVES, RULES, AND THE MEANING OF THE DERIVATIVE
show the degree of downward concavity or negative curvature; dark ones document upward
concavity or positive curvature.
-3.0 -2.5 -2.0 -1.5 -1.0 -0.5 0.0 0.5 1.0 1.5 2.0 2.5 3.0
-1.0
-0.5
0.0
0.5
1.0
1.5
2.0
Figure 3.9: Graph of a function annotated to show curvature.
In addition to curvature, there is a straightforward interpretation of the second derivative in
physics—it is acceleration.
Knowledge Box 3.18
Position, velocity, and acceleration
If y D f .t/ measures the position of an object at time t , then
dy
dt
D
f
0
.t/ gives its velocity, while
d
2
y
dt
2
D f
00
.t/ gives its acceleration.
If we can measure position, then the rate of change of position is velocity, and the rate at
which velocity is changing is acceleration. If the unit of position is meters, then velocity is me-
ters/second, and acceleration is meters/second/second. Each successive derivative increases the
number of “per second” qualifiers by one. is is reflected in the differential notation:

3.5. PHYSICAL INTERPRETATION OF DERIVATIVES 119
• y D f .t/ (no denominator)
•
dy
dt
D f
0
.t/ (time in denominator)
•
d
2
y
dt
2
D f
00
.t/ (time squared in denominator)
Example 3.92 Suppose that the distance traveled by a vehicle is given by
s.t/ D 0:02t
2
C 0:5t C 2:
Find the velocity v.t/ and acceleration a.t/ of the vehicle.
Solution:
Take derivatives:
s.t/ D 0:02t
2
C 0:5t C 2 meters
v.t/
D
0:04t
C
0:5
meters/second
a.t / D 0:04 meters/second
2
˙
Velocity and acceleration are not the only rates of change we may be interested in. e next
example shows how to compute the flow out of a tank from the volume of fluid in it.
Example 3.93 A cylindrical tank with an open spigot at the bottom has a volume of water that
is
V .t/ D 1216 72
p
t liters
at a time t. Find the rate of flow of water from the tank.
Solution:
Take derivatives:
V .t/ D 1216 72
p
t L
V .t/ D 1216 72t
1=2
V
0
.t/ D 36t
1=2
V
0
.t/ D
36
p
t
L/sec
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
