Chapter 8: The Foundations of Quantum Mechanics

Search in book...
Toggle Font Controls
Create new playlist

Name your new playlist

Playlist description (optional)
Sign In

Email address

Password

Forgot Password?

or

Continue with Facebook

Continue with Google
Sign Up

Full Name

Email address

Confirm Email Address

Password

or

Continue with Facebook

Continue with Google

chapter 8

The Foundations of Quantum Mechanics

The postulates of quantum mechanics are mathematical prescriptions for using the theory to predict the results of experiments. These postulates can be presented in terms of the state vector or by using the density operator. We discuss both topics and cover material related to the representation of composite quantum states and the Heisenberg picture. We also introduce a useful tool for two-level systems called the Bloch vector.

CHAPTER OBJECTIVES

In this chapter you will

• Learn how physical systems are represented in quantum theory

• Learn how to represent operators in Dirac notation

• understand spectral decomposition of an operator

• Learn about projective measurements

• illustrate these ideas with two-level systems

The Postulates of Quantum Mechanics

In this section the postulates of quantum mechanics are described in terms of the state vector. This formalism works for an isolated physical system.

POSTULATE 1: States of physical systems are represented by vectors.

The state of a physical system is described by a state vector

that belongs to a complex Hilbert space. The superposition principle holds, meaning that if

are kets belonging to the Hilbert space, the linear combination

is also a valid state that belongs to the Hilbert space. States are normalized to conform to the Born probability interpretation, meaning

If a state is formed from a superposition of other states, normalization implies that the squares of the expansion coefficients must add to 1:

Two-Level System Example: State Vectors

A quantum-bit or qubit is a quantum state that is the basic unit of information in a quantum computer. The state space is two-dimensional with orthonormal basis vectors

An arbitrary state

that belongs to this vector space can be written as a superposition of the basis states

where α and β are generally complex numbers. Normalization of the state implies that

The Born rule tells us that the coefficients in the expansion are related to the probability of obtaining a given measurement as follows:

The probability that measurement finds the system in state

POSTULATE 2: Physical observables are represented by operators.

Physically measureable quantities like energy and momentum are known as observables. Mathematically, an observable is a Hermitian operator that acts on state vectors in the Hilbert space. The eigenvectors of Hermitian operators form an orthonormal basis of the state space for the system.

Two-Level System Example: The Pauli Operators

The Pauli operators X, Y, and Z correspond to the measurement of spin (intrinsic angular momentum of a particle) along the x, y, and z axes, respectively. They act on the basis states

as follows:

We see that the basis states are eigenvectors of Z. Let’s remind ourselves how to construct the matrix representation of an operator.

REMINDER: The Matrix Representation of an Operator

In a discrete basis

, an operator is represented by a set of numbers

These numbers are arranged into a square matrix such that A_ij is the element at the ith row and jth column.

EXAMPLE 8-1

The matrix representation of the Pauli operators can be derived using the

basis (remember, we could equally well choose a different basis, giving a different matrix representation). In this basis we form the matrix representation of an operator A by calculating

The basis is orthonormal, and so

Using the action of the X operator described earlier, we find

A similar procedure applied to the other operators shows that

Writing an Operator in Terms of Outer Products

Consider two kets

and

. The quantity formed by their outer product

is an operator. Any operator can be written in this fashion. In terms of some basis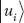

, an operator A can be written as

Looking at the matrix representation of the Pauli X operator

we see that, with respect to the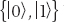

basis, the operator can be written as

EXAMPLE 8-2

A useful operator in quantum information theory is known as the Hadamard gate. This operator can be used to construct superposition states from {|0_, |1_}. In this basis the Hadamard gate has the representation

In outer product notation, the operator is written as

We can use this representation to quickly find the action of H on a state. For example,

We also have

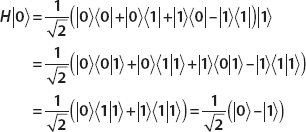
Using these results, we find that for an arbitrary state

An operator can be written in terms of projection operators formed by its own eigenvectors. This is called the spectral decomposition.

Spectral Decomposition

Suppose that an operator A has eigenvalues λ_i and eigenvectors

The spectral decomposition of operator A is a representation of the operator in terms of its eigenvalues and the projector

given by

Two-Level System Example: Spectral Decomposition of Z

A quick exercise shows that the eigenvectors of Z are

with eigenvalues ±1

The spectral decomposition of Z is given by

POSTULATE 3: The possible results of a measurement are the eigenvalues of an operator.

The possible results of a measurement of a physical quantity are the eigenvalues of its corresponding operator.

EXAMPLE 8-3

Elementary particles such as electrons carry an intrinsic angular momentum called spin. Measurement of spin 1/2 along the x axis is represented by the Pauli operator

If spin is measured along the x axis, what are the possible results of measurement?

SOLUTION

The possible results of measurement are found from the eigenvalues of the matrix. The characteristic polynomial is found from

This equation is satisfied by

Therefore the possible results of measurement of spin along the x axis are

According to the measurement postulate, these are the only values of measurement that will ever be found.

Projective Measurements

Projective measurements—the type encountered in standard quantum mechanics—deal with mutually exclusive measurement results. For example, we can ask if a particle is located at position x₁ or position x₂. Another example might be asking if a trapped electron has ground state energy E₁ or excited state energy E₂. Mutually exclusive measurement outcomes such as these are represented by orthogonal projection operators

The statement that two projection operators are orthogonal means that if i ≠ j, then

We can see this by using the spectral decomposition of some operator A:

The eigenvectors of a Hermitian operator are orthogonal, and in fact the practice is to normalize them. Assuming the eigenvectors are orthonormal, we have

Therefore if i ≠ j, we obtain P_iP_j = 0. However, if i = j, then

Two-Level System Example: Projection Operators

In the standard basis, there are two projection operators that correspond to measurements of +1 and −1:

The operator P₀ projects any state onto

while P₁ projects any state onto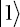

For some arbitrary state

, we have

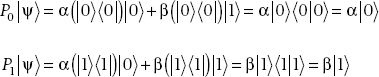
since

. Now we consider the sum of a set of projection operators.

The Completeness Relation

The sum of the projection operators gives the identity

For the two-level system we have been studying, this means that

We can show this by calculating the matrix representations of the outer products

Suppose that an operator A has eigenvalues λ_i and eigenvectors

We can expand a state

in terms of the eigenvectors of A:

The probability of obtaining measurement result λ_i, which is associated with eigenvector , is given by the Born rule:

Probability of finding

Remember, the inner product is a complex number:

Since these are numbers, we can change their order and write the probability in terms of a projection operator:

EXAMPLE 8-4

A quantum system is in the state

where the

constitute an orthonormal basis. These states are eigenvectors of the Hamiltonian operator such that

(a) Is

normalized?

(b) If the energy is measured, what are the probabilities of obtaining E, 2E, and 3E ?

SOLUTION

(a) The bra that corresponds to the state is given by

Therefore the norm of the state is found to be

Notice that we have used the fact that the basis is orthonormal, and so

Since

we conclude the state is normalized.

(b) To find the probability of obtaining each measurement, we use the Born rule. We have

The Born rule tells us that the probability of obtaining measurement result E is

For the next eigenvector, we find

The Born rule tells us that the probability of obtaining measurement result 2E is

Finally, we have

The Born rule tells us that the probability of finding 3E upon measurement is

Although we have already verified that the state is normalized, it is always a good idea to sum the probabilities and verify that they sum to 1, as they do in this case:

EXAMPLE 8-5

An orthonormal basis of a Hamiltonian operator in four dimensions is defined as follows:

A system is in the state

(a) If a measurement of the energy is made, what results can be found and with what probabilities?

(b) Find the average energy of the system.

SOLUTION

First we check to see if the state is normalized. We have

Therefore it is necessary to normalize the state. The normalized state is found by dividing by

. Calling the normalized state

, we obtain

Since the state is expanded in the eigenbasis of the Hamiltonian, the only possible values of measurement are the eigenvalues of the Hamiltonian, which have been given to us as (E, 2E, 3E, 4E). The probabilities of obtaining each measurement are found by application of the Born rule, squaring the coefficients for the normalized state.

(a) The probability of obtaining E is

The probability of obtaining 2E is

The probability of obtaining 3E is

The probability of finding 4E is

A quick check shows these probabilities add to 1:

(b) We can find the average energy from

where p(E_i) is the probability of obtaining measurement result E_i. We find that the average energy is

Aside on Degeneracy

Suppose that an operator A has degenerate eigenvalues corresponding to eigenvectors

Where each of these eigenvectors corresponds to the same eigenvector

This set of eigenvectors constitutes a subspace of the vector space ε. In the case of degeneracy, the probability of obtaining measurement result λ_m is found by summing over the inner products of all the eigenvectors that belong to this subspace:

Probability of finding

POSTULATE 4: The state of a system after measurement.

The reader who studies quantum theory more extensively will find that not all measurements can be described as projective measurements, as we have done here. Nonetheless, in a first exposure to quantum theory it is good to restrict our attention to measurements of this type. We consider the nondegenerate case first. Immediately after a measurement, the state of the system is given by the eigenvector corresponding to the eigenvalue that has been measured. If the state of the system is given by

then suppose a measurement is made and the result λ_i is obtained. The state of the system immediately after measurement is

In terms of projection operators, the state of the system after measurement is

Two-Level System Example: State of the System after Measurement

Recalling the projection operators for the two-state system

and the action on a state

that we found to be

the state after measurement is found by dividing these quantities by

. We have

and

Therefore, if measurement result +1 is obtained, the state of the system after measurement is

and if −1 is obtained, it is

EXAMPLE 8-6

Suppose a Hamiltonian operator has a basis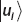

such that

The system is in the state

(a) Write the Hamiltonian operator in outer product notation.

(b) Write the projection operator P that projects a state onto the subspace spanned by

(d) Suppose the energy is measured and found to be E. What is the state of the system after measurement?

SOLUTION

(a) Using the spectral decomposition of H, we find

(b) The projection operator for the subspace spanned by

is found by summing over the individual projection operators for each state:

(c) The reader should verify that the state is normalized. The possible results of measurement are E, 2E, and 4E, corresponding to eigenvectors

, and

, respectively. To calculate the probability of obtaining the measurement result E, which is degenerate, we use

The other probabilities are for nondegenerate eigenvalues and can be calculated immediately by using the Born rule:

(d) If the energy is measured and is found to be E, the state after measurement is

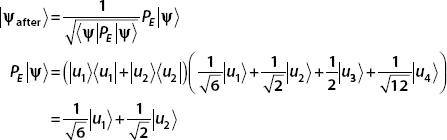
It is easy to show that

and so we have

Therefore, the state after measurement is

Note: The state of the system cannot be further distinguished; with the information given there is nothing we can do to distinguish

from

POSTULATE 5: The time evolution of a quantum system is governed by the Schrödinger equation.

The Schrödinger equation determines how a quantum system changes with time according to

where H is the Hamiltonian and t₀ is some initial time. For an isolated system the Hamiltonian is independent of time. The equation can be integrated in that case, giving

The exponential term defines a time evolution operator, which we denote by U because this operator is unitary:

The infinitesimal operator is found by expanding I in a Taylor series and keeping the first two terms:

Completely Specifying a State with a CSCO

In a previous example, we found that when two energy eigenstates had the same eigenvalue E, and a measurement result turned out to be E, then the state of the system after measurement was

There was no way to distinguish between the states . There are many situations like this that can arise. However, many eigenfunctions of one operator with degenerate eigenvalues turn out to be eigenfunctions of another operator with eigenvalues that can be used to distinguish the states. For example, free particle energy states have degenerate energy eigenvalues, but can be distinguished by momentum ±p. So, to completely distinguish the state, the energy E is not enough; we must also specify a momentum of +p or −p. This notion is formalized by the idea of a CSCO. A CSCO is a complete set of commuting observables and is the minimum set of operators required to completely specify a state. Two or more operators that commute can be simultaneously measured. Suppose that

Now notice what happens when we apply the product AB to the state

and the application of BA gives

and so

Since the state

, we must have

Now suppose that a state

is also an eigenstate of A with the same eigenvalue a, so that this eigenvalue is degenerate. Then a measurement of A cannot distinguish the states. However, if

is also an eigenvector of B such that

then we can distinguish the states by measuring B. In this example, A and B form a CSCO. What if the eigenvalues of B are also degenerate? Then we must find an operator C that commutes with both A and B, but has eigenvalues such that the states can be distinguished. If it turns out that no such set of operators can be found, then the states are physically indistinguishable.

The Heisenberg versus Schrödinger Pictures

In the Schrödinger picture, the time evolution of the system is contained in the state. However, since the physical predictions of quantum mechanics are determined by inner products, the time evolution can be moved from states to operators:

We can then describe time evolution in terms of evolving the operator forward in time according to

The governing equation for time evolution is given by

where H is the system’s Hamiltonian.

Describing Composite Systems in Quantum Mechanics

Sometimes it is necessary to describe a system that is composed of multiple particles, each particle being a system in its own right described by a vector space. A mathematical technique called the tensor product allows us to bring vector spaces that describe individual systems together into a larger vector space. The tensor product between vector spaces V and W is denoted by writing

Dimension of a Vector Space Formed by a Tensor Product

If V is a vector space of dimension p and W is a vector space of dimension q, then the vector space

has dimension pq.

Let

be two vectors. Then the vector

. Alternative shorthand notations for the vector

include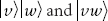

If A is an operator that acts on the vector space V and B is an operator that acts on the vector space W, then the operator formed by the tensor product given by

acts on vectors in the following way:

We now consider the norm of a state that belongs to

. Suppose that

Then the norm is

The Matrix Representation of a Tensor Product

Let A be an m × n matrix and B be a p × q matrix. The tensor product

is a matrix with mp rows and nq columns. The elements of this matrix are constructed by the submatrices

that is, multiply B by each component of A and then arrange these submatrices into a larger matrix. The entire matrix representing the tensor product is

A column vector in two dimensions has two rows and one column. Since the tensor product between an m × n matrix and a p × q matrix has mp rows and nq columns, the tensor product between two 2 × 1 column vectors has 2 × 2 = 4 rows and 1 × 1 = 1 column. The way to compute this is

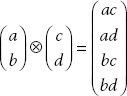
Now consider the tensor product of two 2 × 2 matrices. This tensor product will be a matrix with 2 × 2 = 4 rows and 2 × 2 = 4 columns. If we let

then the tensor product of these two matrices is

EXAMPLE 8-7

Consider the two Pauli matrices Y and Z. Compute the tensor product

SOLUTION

Recalling that

we have

The Tensor Product of State Vectors

We can use the tensor product to compute the tensor product of a state with itself n times. This is represented by the notation

EXAMPLE 8-8

Let

where

. Find

SOLUTION

It is helpful to write the state as a column vector:

So we obtain

The tensor product

EXAMPLE 8-9

Consider a composite system of qubits. The first qubit is denoted by A and the second by B. The spin-singlet state is formed from a linear superposition:

The operator X_A applies the X operator only to states belonging to subsystem A. Suppose that the operator X_A acts on the state. What is the resulting state of the system?

SOLUTION

The operator X is

and it acts as follows:

Therefore, when X_A acts on the composite state, we find

EXAMPLE 8-10

Suppose that a two-qubit system is in the state

Find

SOLUTION

Recalling that 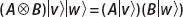, we have

and so

The Density Operator

So far our studies of quantum systems have involved the examination of a single state. This state can be expanded in terms of basis kets:

Such an expansion is referred to as a coherent superposition of the 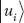states. The task has been to find out what the possible measurement results are on the system and to calculate the probability of obtaining each possible result.

Now consider an ensemble of quantum states that is prepared as a classical statistical mixture. The members of the ensemble are the states , and the probability of finding each state is given by p₁, p₂, . . . , p_n. We can describe this type of mixture of states with a density operator, which is usually represented by the symbol ρ.

The density operator representing a collection of states

If the state of a system is known exactly, we say it is a pure state. This is a state where a single p_i = 1 and all others are zero. This means that the density operator for a pure state

can be written as

This is a projection operator, so in the case of a pure state, the density operator satisfies ρ² = ρ. A mixed state is a collection of different pure states, each occurring with a given probability.

Properties of the Density Operator

• The density operator is Hermitian, ρ = ρ^†.

• The trace of any density matrix is equal to 1: Tr(ρ) = 1.

• For a pure state, since ρ² = ρ, Tr(ρ²) = 1.

• For a mixed state Tr(ρ²) < 1.

• The eigenvalues of a density operator satisfy 0 ≤ λ_i ≤ 1.

• The expectation value of an operator A can be calculated using

We can use a density operator to make all the physical predictions of quantum mechanics. Let a measurement result m be represented by a projection operator 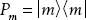. The probability of obtaining measurement result m is

Density operators can be represented by matrices. The off-diagonal elements of a density matrix represent the ability of the system to exhibit quantum interference. Consider, once again, a state formed by a superposition:

This type of state is a pure state. In this case the density operator is found from the outer product

It is not too hard to show that this is

We see that the density operator for this pure state can be split into two parts. The terms in the first part tell us

which is the probability of finding the system in state .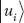

To determine the meaning of the second part of the density operator, given by the sum

first recall that the expansion coefficients are complex numbers. We can write any complex number in polar form, that is, z = re^iΦ;. In this case we have

So, with respect to the second summation, we have

The phase difference in the exponential expresses the coherence or capability of terms in the state to interfere with one another. This is a characteristic of a pure quantum state, and these terms are represented by off-diagonal elements in the density matrix. Mixed states are classical statistical mixtures and therefore have no terms of this form.

The off-diagonal elements of a mixed state will be zero, while those of a pure state—a state with coherences—will be nonzero. Note, however, that the presence of the off-diagonal terms is basis-dependent. Always check purity by computing the trace Tr(ρ²).

A completely mixed state is one for which the probability of each state is equal to all others. For example,

is a completely mixed state with a 50% probability of finding the system in state

The Density Operator for a Completely Mixed State

In an n-dimensional Hilbert space, the density operator can be expressed in terms of the identity matrix, and

A completely mixed state represents one extreme possibility—a statistical mixture with no interference terms. A pure state represents the other extreme—a quantum state in a coherent superposition. A measure of purity can be expressed by computing the trace of ρ² and setting it in between these bounds:

A state that falls in this range without being pure Tr(ρ²) = 1 or completely mixed for which

is called partially coherent. Testing purity by checking the trace of the square of the density matrix is a basis-independent concept, unlike the values of the off-diagonal elements of the matrix, which depend on the basis you choose to express the density operator.

EXAMPLE 8-11

In this example we compare pure versus mixed states for a three-dimensional quantum system. We consider two orthonormal bases

The second basis can be expressed in terms of the first, using the relations

Now consider the pure state

The state

is a coherent superposition of states

. Notice that in this state the probabilities of finding states

are

The state is pure because if we make a measurement in the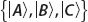

basis, the probabilities of obtaining each measurement result are

Now, using the expansion of in the {

} basis, we can write the density operator

Now we consider another state, a state that is a statistical mixture of the

states. Let

Recalling that a density operator is a summation of the form

where p_i is the probability of finding state

, we see that in the state q_m the probabilities of finding each of the states are

These are the same probabilities we found for the state , and so this seems to describe the same state. However, what are the probabilities of finding the system in the states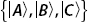

when the system is in the state described by ρ_m? We can answer this question by considering the projection operators

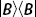, and

and using

. The probability of finding when the system is described by ρ_m is

Now

and so

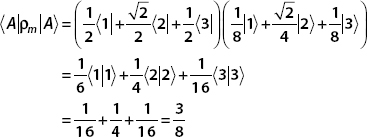
This is quite different from the pure state where we found prob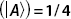

. A similar procedure using the expansion of the state

in the

basis shows that the probability of finding

in the state ρ_m is

This is certainly different from what we found for ρ_A, where the probability was zero. In conclusion, the predictions of ρ_m are identical to those of ρ_A for measurements in the , basis, but they are quite different for the basis.

Now let’s consider the matrix representations of these density operators in the

basis. We have

Notice that

as it should for a density matrix.

An exercise shows that the density operator representing ρ_A in this basis is

Notice that the mixed state has zero off-diagonal elements and the pure state has nonzero off-diagonal elements. While this is a characteristic property, it depends on the basis chosen to represent the density operator (try writing the matrix for ρ_A in the basis). We also have

Now consider the square of this matrix. A calculation shows

telling us that this is a pure state. Now consider

Therefore, this density operator represents a mixed state (as we knew already). Recalling that the dimension n = 3 of this Hilbert space, the completely mixed state would be represented by the density operator

The matrix representation in this basis is

We see that

and so

This is the completely mixed state for three dimensions. And so we have the bounds

where ρ_A is a pure state. The state ρ_m is a partially coherent state.

EXAMPLE 8-12

We work in a two-dimensional vector space with basis

. Consider an ensemble of systems prepared in the following way. We have two quantum states

In the preparation of the ensemble, three-fourths of the states are prepared in state

and one-fourth of the states are prepared in state

(a) Write down the density operator for each individual pure state and

(b) Write down the density operator for the ensemble.

basis.

(d) Compute the trace.

(e) If a particle is drawn from the ensemble and a measurement is performed, what is the probability it is found in state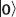

? What is the probability it is found in state

(f) Suppose there is another measurement with basis states

. A particle is drawn from the ensemble, and a measurement is made in this basis. What is the probability of finding

and what is the probability of finding

SOLUTION

(a) The density operators for each pure state are

(b) The density operator for the ensemble can be found from the formula

We are told that three-fourths of the systems are prepared in state and one-fourth of the systems are prepared in state

. Therefore in this case we have

Now

Adding these terms gives

basis is

(d) We find the trace by summing the diagonal elements

as it should be for a density matrix.

(e) The probabilities that a particle drawn is found in the state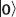

or the state

are found from the elements along the diagonal of the matrix. The probability the particle is found to be in state

is 63/80 = 0.79. The probability the particle is found to be in state

is 17/80 = 0.21.

(f) We can find the probability that a particle is found in the state

by calculating

. The column vector representation of

We can calculate the probability using matrix multiplication:

The probability that the particle is found in the state

EXAMPLE 8-13

A density matrix is given by

In quantum information theory, a measurement of information content is given by the entropy. The entropy is given by

where the λ_i, are the eigenvalues of the density matrix and the log is to base 2. Higher entropy means greater disorder (and less information) in a system. Find the entropy for the system described by this density matrix.

SOLUTION

The eigenvalues of this matrix are {0.933, 0.067}. The entropy is

S = −0.933log(0.933) − 0.067log(0.067) = 0.25

The maximum entropy for the basic unit of quantum information theory, a qubit, is S = 1.

EXAMPLE 8-14

Suppose the density matrix for some system is

(a) Show that this is a mixed state.

(b) Find

for this state.

SOLUTION

(a) We calculate the square of the matrix

The trace is

Therefore this is a mixed state.

(b) We calculate the matrix product

The expectation value can be found from

For Z we have

and the expectation value is given by

A Brief Introduction to the Bloch Vector

For a two-level system, we can write the density operator in the following form:

The vector is called the Bloch vector. We can use the Bloch vector to determine if a state is a pure state or a mixed state.

The magnitude of the Bloch vector is less than or equal to 1 (with equality only for a pure state):

The components of the Bloch vector are calculated from

where X, Y, Z are the Pauli operators.

EXAMPLE 8-15

A density operator for some system is given by

(a) Find the components of the Bloch vector.

(b) Is this state mixed or pure?

(c) A measurement of spin is made in the z direction. What is the probability that the measurement result is spin-down? What is the probability that the measurement is spin-up?

SOLUTION

(a) The components of the Bloch vector are

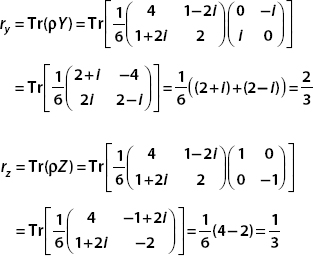
(b) The magnitude of the Bloch vector is

Since the magnitude is less than 1, this density operator represents a mixed state.

(c) The probability of obtaining a given result is given by Tr(ρP), where P is a projection operator corresponding to the given measurement. The projection operator for spin-down is

The probability is found from the trace, which is

For spin-up, the projection operator is

The product of the matrices is

and so the probability of finding spin-up for this state is

Summary

In this chapter we learned the fundamental postulates of quantum mechanics and how to apply them using a two-state system. We also learned the modern concepts of the density operator which is important in many applications such as quantum computing.

QUIZ

1. Using matrix representation, show that the Hadamard matrix