12

Process Costing

12.1 INTRODUCTION

In this chapter another costing method is discussed: process costing. Process costing is used by organizations when a number of production processes are involved and the output of one process is the input of a later process; this continues until the final product is obtained. Examples of industries where process costing might be applied are food processing, chemicals and brewing industries.

Process costing is an accounting methodology that traces and accumulates direct costs, and allocates indirect costs of a manufacturing process. Costs are assigned to products, usually in a large batch, which might include an entire month's production. Eventually, costs have to be allocated to individual units of product. Process costing assigns average costs to each unit and is the opposite extreme of job costing, which attempts to measure individual costs of production of each unit.

Process costing is a type of operation costing that is used to ascertain the cost of a product at each process or stage of manufacture. Chartered Institute of Management Accountants defines process costing as follows: ‘The costing method applicable where goods or services result from a sequence of continuous or repetitive operations or processes. Costs are averaged over the units produced during the period.’ Process costing is suitable for industries producing homogeneous products and where production is a continuous flow. A process can be referred to as the sub-unit of an organization specifically defined for cost collection purposes.

Process costing is a type of costing system that is used for uniform, or homogeneous, products. Process costing averages the costs over all units to come to the cost per unit. This is in contrast to other types of costing systems, such as job order costing that is used for products that are in differentiated batches. Unlike job order costing, process costing is tracked using a work-in-process account for each department, rather than through subsidiary ledgers.

The method for determining the total unit cost of the output of a continuous production run (such as in food processing, petroleum and textile industries) in which a product passes through several processes (or cost centres) involves the following steps: (1) The ‘total cost per process’ is computed by estimating the number of products passing through each process in a given period; (2) the ‘unit cost per process’ is computed by dividing the ‘total cost per process’ by the number of units passing through the process in the given period; and (3) the ‘unit cost per process’ is charged to each unit as it passes through each process, so that at the end of the production cycle each product will have received an appropriate charge for each process through which it has passed.

It is a method in which costs are collected according to processes and the cost of each process is divided by the quantity of production to arrive at the cost per unit. This method is used to ascertain the cost of the product at each process. Therefore, it is known as process costing.

12.2. ADVANTAGES AND DISADVANTAGES OF PROCESS COSTING

12.2.1 Advantages of process costing

The advantages of process costing are as follows:

1. In process costing, the product is of uniform type; so the computation of average cost is easier.
2. The cost is calculated periodically and not at the completion of each job as is done in the case of job costing.
3. Clerical efforts and costs are less as compared to job costing.

The cost of each process and that of finished products can be determined at short intervals, weekly or daily. Cost control and control over production are more effective because of uniform output and use of predetermined costs as budgeted or standard costs.

12.2.2 Disadvantages of process costing

The disadvantages of process costing are as follows:

1. Since process costs are average costs, they are not always accurate.
2. Since process costs are collected at the end of a given period, they are in the nature of historical costs.
3. Computation of average cost becomes more difficult when more than one type of product is manufactured.
4. There is a lack of commonly accepted criterion for the allocation of joint costs among different types of products.

12.3 INDUSTRIES WHERE PROCESS COSTING IS APPLIED

The major industries where process costing is applied are as follows:

 

Chemical works

Oil refining

Soap making

Textiles, weaving and spinning Paper mills

Food products

Biscuits works

Meat products factory, milk dairy

12.4 CHARACTERISTIC FEATURES OF PROCESS COSTING

The characteristic features of process costing can be summarized as follows:

1. Production is continuous.
2. Processes and products are standardized.
3. The output of one process is the input of the next process.
4. There may be process losses of input.
5. There may be abnormal gain.
6. Two or more products may be produced simultaneously.

12.5 ACCOUNTING PROCEDURE OF PROCESS COSTING

The accounting procedure of process costing can be explained as follows:

1. Process account is prepared with both unit and amount columns.
2. Material, labour and overhead are debited to process account.
3. Normal loss, abnormal loss and finished stock are credited to process account.
4. Output of one process is considered as the raw material of the next process.
5. Generally, normal loss has no value unless mentioned.
6. Cost due to normal loss is borne by the good units.
7. Cost due to abnormal loss is not borne by the good units; they are treated as good units.
8. Abnormal gain is valued in the same manner as abnormal loss, but debited to process account.
9. Amount realized from scrap is credited to process account.
10. Formula for abnormal loss is as follows:

     

    

     

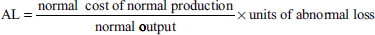
11. Formula for abnormal gain is as follows:

     

    

     

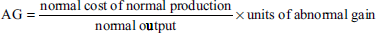
12. Normal cost of normal production refers to the total of material labour and overhead in the debit side of process account.
13. While calculating normal cost of normal production, normal loss (scrap) value should be deducted.

12.6 NORMAL LOSS

Loss that cannot be avoided or controlled is called normal loss, for example, evaporation of petrol and diesel spirit. Losses of this type should he absorbed by good units. Any value realizable on normal loss is credited to process account. Normal losses are unavoidable as they are losses arising due to the nature of the material or the process. The reasons for such losses in output can be the following:

• Evaporation
• Breakage
• Scrap due to the need for high quality
• Rejection on inspection
• Defective units
• Loss inherent in large-scale manufacturing
• Chemical change
• Residue material
• Examples of normal losses are metal turnings, off-cuts, metal borings, edges, shreddage and ends.
• The quantity of normal loss is anticipated based on past experience and the material specification.
• The cost of normal loss is absorbed by the completed output.
• The value of scrap of normal loss units is deducted from direct material cost. Normal loss never receives a share of the process cost.

12.7 ABNORMAL LOSS

Loss that can be avoided or controlled is called abnormal loss. Abnormal loss is valued just like good units and transferred to an account called the abnormal loss account.

12.8 ABNORMAL GAIN

When the actual loss is less than the estimated loss, it is known as abnormal gain. It is possible that the workers’ efficiency goes up all of a sudden thereby causing reduction in normal loss. In such a circumstance, abnormal gain increases. That is why abnormal gain is also known as effectiveness.

12.9 TREATMENT OF NORMAL PROCESS LOSS, ABNORMAL PROCESS LOSS AND ABNORMAL GAIN

Loss of material is inherent in processing operations. The loss of material under different processes may be due to reasons like evaporation or a change in moisture content. Process loss is defined as the loss of material occurring during the course of a processing operation, and it is equal to the difference between the input quantity of the material and the output. There are two types of material losses:

1. Normal process loss: It is defined as the loss of material that is inherent in the nature of work. Such a loss can be reasonably anticipated from the nature of the material, nature of operations, past experience and technical data. Good units produced under the process absorb the cost of normal process loss in practice. Amount realized by the sale of normal process loss units should be credited to process account.
2. Abnormal process loss: It is defined as the loss in excess of predetermined loss. This type of loss may occur due to the carelessness of workers, a bad plant design or a bad operation. Such a loss cannot obviously be estimated in advance. But it can be kept under control by taking suitable measures. The cost of abnormal process loss units is equal to the cost of a good unit. The total cost of abnormal process loss is credited to the process account from which it arises. Cost of abnormal process loss is not treated as a part of the cost of the product. In fact, the total cost of abnormal process loss is debited to costing profit and loss account.

Abnormal gain: Sometimes, loss under a process is less than the anticipated normal figure. In other words, the actual production exceeds the expected figures. Under such a situation, the difference between actual and expected losses or actual and expected production is known as abnormal gain. So, abnormal gain may be defined as the unexpected gain in production under normal conditions. The process account under which an abnormal gain arises is debited with the abnormal gain. The cost of abnormal gain is computed on the basis of normal production.

12.10 JOB COSTING VERSUS PROCESS COSTING

Job costing basically refers to the costs that are encountered in businesses related to manufacturing goods.

Process costing refers to the methodology involved in calculating the costs that are incurred while performing a particular task.

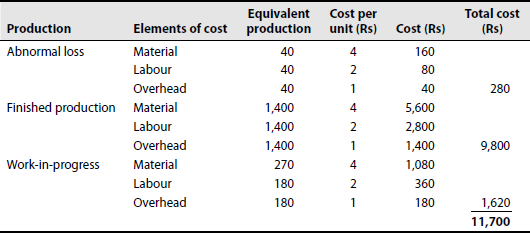
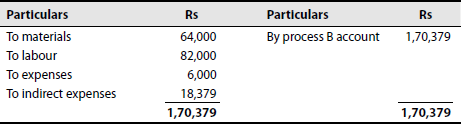
Illustration 1

A product passes through two processes. Prepare process accounts from the following information:

	Process A (Rs)	Process B (Rs)
Materials	64,000	18,000
Labour	82,000	34,000
Expenses	6,000	14,000

Indirect expenses, quantity apportioned to Rs 26,000, are to be appointed on the basis of labour.

Prepare process accounts.

Solution: Process A Account

 

Process B Account

 

 

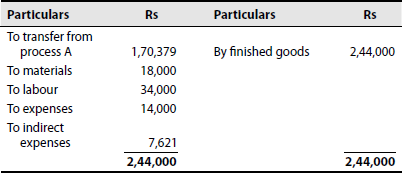
Illustration 2

Prepare process accounts from the following information:

 

 

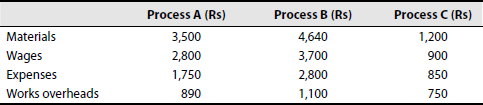
Other expenses of Rs 1,400 should be allocated based on wages.

Solution: Process A Account

 

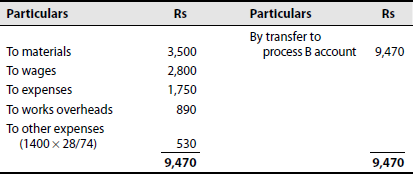
Process B Account

 

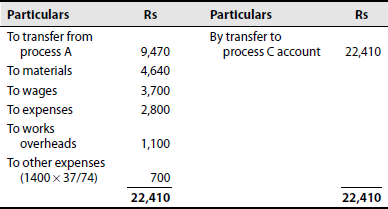
Process C Account

 

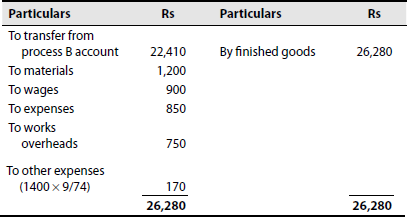
No losses but hints given.

Illustration 3

A product passes through three processes A, B and C for its completion. As finished goods 6,000 units are produced.

 

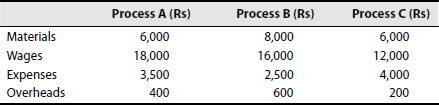
Raw material is issued at Rs 45,000. Prepare process accounts.

Solution: Process A Account

 

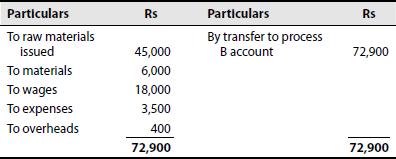
Process B Account

 

Process C Account

 

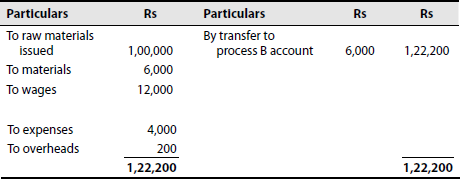
Illustration 4

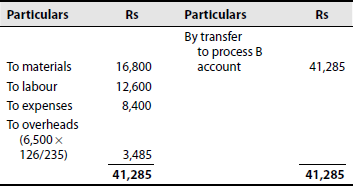
A product passes through two processes. Prepare process accounts.

	Process A (Rs)	Process B (Rs)
Material	16,800	7,200
Labour	12,600	10,900
Expenses	8,400	6,750

Overheads accounting to Rs 6,500 is allocated on the basis of labour. Units produced were Rs 650.

Solution: Process A Account

 

Process B Account

 

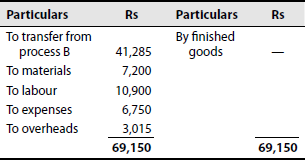
Illustration 5

A product passes through two processes. Prepare process accounts.

	Process A (Rs)	Process B (Rs)
Direct materials	40,000	50,000
Direct wages	50,000	30,000
Output in units	6,000	7,000
Opening stock (in units)	—	3,000
Closing stock (in units)	—	1,000

Solution: Process A Account

 

 

Process B Account

 

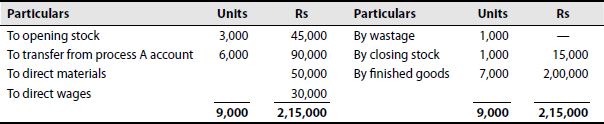
Illustration 6

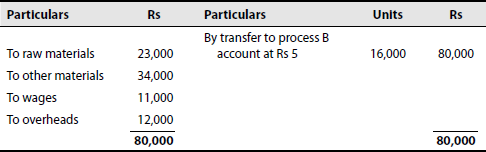
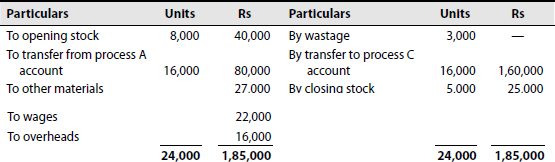
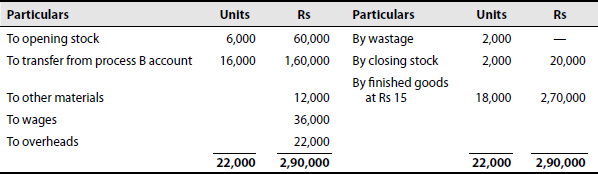
A product passes through three processes. Calculate cost of the product at each stage:

 

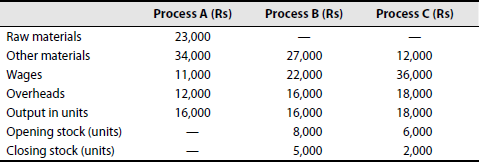
Solution: Process A Account

 

Process B Account

 

 

Process C Account

 

Normal loss with scrap value.

Illustration 7

The following expenditure is incurred for producing some articles:

Materials (200 units)	Rs 7,000
Labour	Rs 4,000
Indirect expenses	Rs 3,000

Normal wastage is 7% of the input. One unit of wastage is sold at Rs 20 each. Prepare process account.

Solution: Process A Account

 

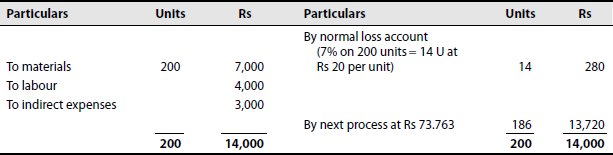
Illustration 8

A product passes through three processes A, B and C. To process A, 10,000 units at Re 1 per unit were issued. The other direct expenses are as follows:

 

 

The wastage of process A was 5%, process B 4% and process C 5%. The wastage of process A was sold at Re 0.25 per unit, that of B at Re 0.50 per unit and that of C at Re 1 per unit. The overhead charges were 168% of direct labour. The final product was sold at Rs 10.0 per unit, fetching a profit of 20% on sale. Prepare process accounts and finished goods account.

Solution: Process A Account

 

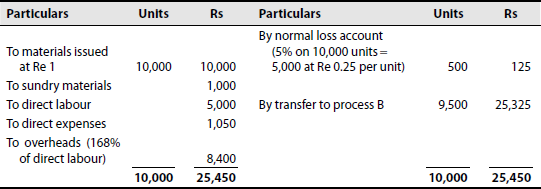
Process B Account

 

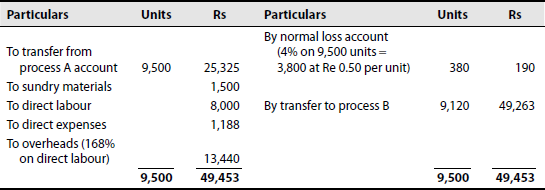
Process C Account

 

Finished Goods Account

 

Illustration 9

Prepare process account from the following:

 

Materials issued = 1,500 kg at Rs 110

Wages = Rs 48,000

Overheads = Rs 10,000

Normal loss = 5% of input

Solution: Process A Account

 

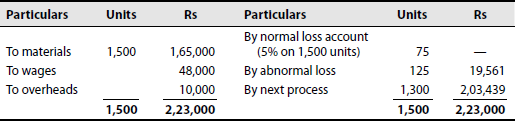
Note:

 

Normal output = 1,500 kg − 75 kg = 1,425 kg

Normal cost of normal output = Rs 2,23,000

Cost per unit of normal output = Rs 2,23,000/1,425 = Rs 156.49

Abnormal loss amount = Rs 156.49 × 125 kg = Rs 19,561

Or

 

Illustration 10

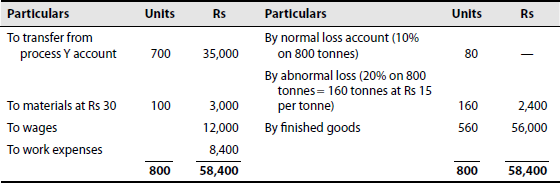
A product passes through two processes X and Y before it is finished and transferred to stock. In both the processes, 10% of the weight put in is lost. An additional 20% is scrapped, which realizes Rs 10 per tonne and Rs 15 per tonne, respectively, from processes X and Y. The following data is obtained for the month of November 1996

	Process X	Process Y
Material consumed	1,000 tonnes	100 tonnes
	Rs	Rs
Cost per tonne of material	20	30
Wages	10,000	12,000
Works expenses	7,000	8,400

Prepare process accounts showing cost of the output of each process and the cost per tonne.

Solution: Process X Account

 

 

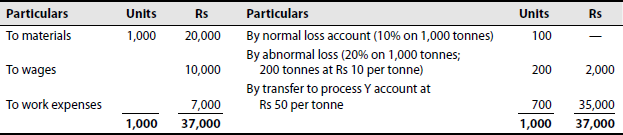
Process Y Account

 

 

12.11 INTERPROCESS PROFITS AND THEIR ACCOUNTING PROCEDURE

12.11.1 Interprocess profits

The output of one process is transferred to the next process. That is, the output of one process becomes the input of the next process. The output is transferred not at the cost price but at the market price, that is, with a certain percentage of profit. The difference between cost and transfer price is known as interprocess profit.

The advantages of interprocess profits are as follows:

1. Comparison between the cost of output and its market price at the stage of completion is facilitated.
2. Each process is made to stand by itself as to profitability.

The disadvantages of interprocess profits are as follows:

1. The use of interprocess profits involves complication.
2. The system shows profits that are not realized because of stock not sold out.

The objectives of this method are as follows:

1. To show whether cost of production competes with market price
2. To make each process stand on its own efficiency and economics
3. To find out the profitability of each process individually
4. To assist in decision making

12.11.2 Accounting procedure for interprocess profit

1. Process account is prepared with three columns: cost, profit and total.
2. Closing stock is deducted and shown in the debit side of process account.
3. Profit on closing stock is calculated.
4. Formula for calculation of closing stock at cost:

    

   

    

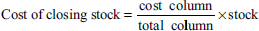
Illustration 11

A certain product passes through three processes before it is completed. The output of each process is charged to the next process at a price calculated to give a profit of 20% on transfer price (that is, 25% on cost price). The output of process III is charged to the finished stock account on a similar basis. There was no work-in-progress at the beginning of the year and overheads have been ignored. Stock in each process has been valued at prime cost of the process. The following data are obtained at the end of 31 March 2001:

 

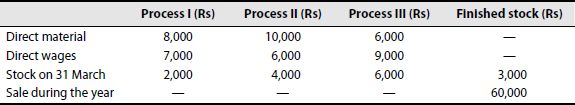
From this information, prepare

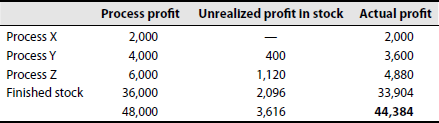
1. Process cost accounts showing the profit element at each stage
2. Actual unrealized profits
3. Stock valuation as it would appear in the balance sheet

Solution: Process I Account

 

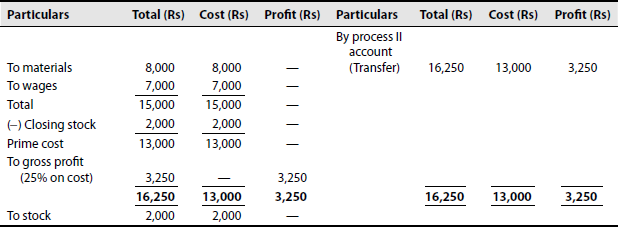
Process II Account

 

 

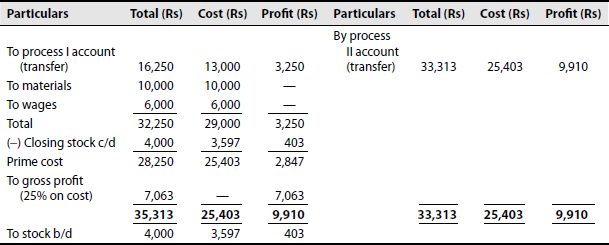
Process III Account

 

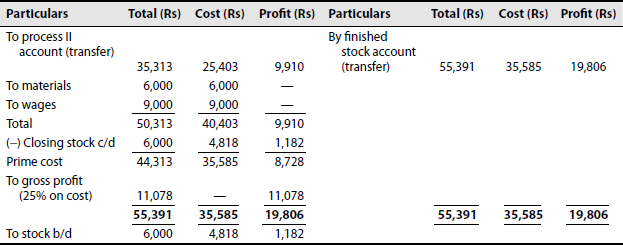
Finished Stock Account

 

Formula for calculation of profit on closing stock:

 

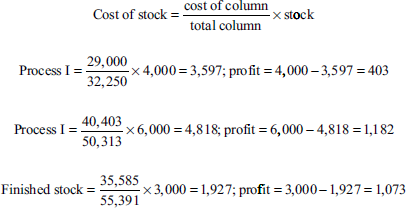
Actual profit unrealized:

 

 

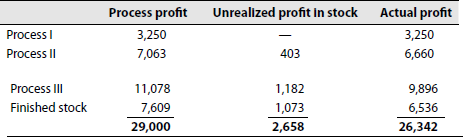
(c) Stocks for balance sheet:

Process I	2,000
Process II	3,597
Process III	4,818
Finished stock	1,927
Total	12,342

Illustration 12

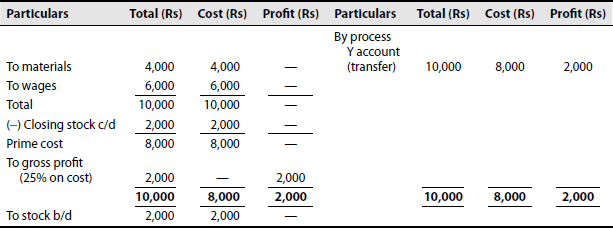
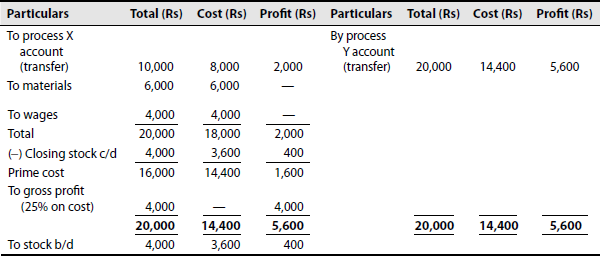
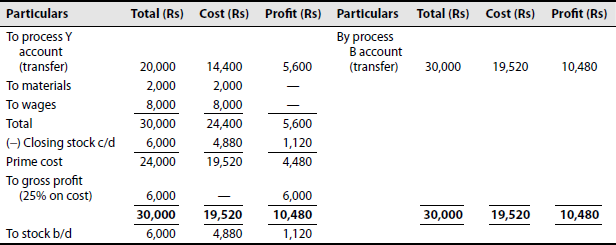
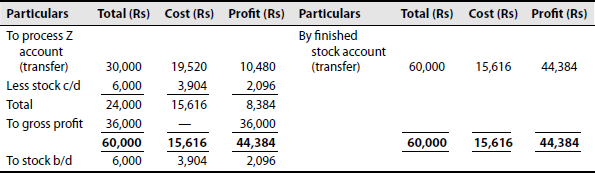
A product passes through three processes to reach completion. These process are X, Y and Z. The output of each process is charged to the next process at a price calculated to give a profit of 20% on transfer price. The output of process Z is charged to the finished stock on a similar basis. There was no partly finished work in any process on 31 December, on which date the following information was obtained:

 

 

Stocks in each process were valued at price cost to the process. There was no stock in hand on 1 January and the question of overhead was ignored. Of the goods passed into finished stock Rs 6,000 remained in hand on 31 December, and the balance was sold for Rs 60,000. Show process accounts and calculate reserve for unrealized profits.

Solution: Process X Account

 

Process Y Account

 

 

Process Z Account

 

Finished Stock Account

 

Formula for calculation of profit on closing stock:

 

 

 

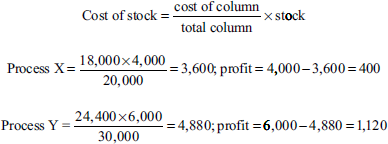
Actual profit unrealized:

 

Stocks for balance sheet:

 

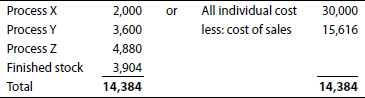
Illustration 13

A product passes through three processes before it is completed. The output of each process is charged to the next process at a price calculated to give a profit of 20% on transfer price. The output of process III is charged to the finished stock account on a similar basis. There was no work-in-progress at the beginning of the year and overheads have been ignored. Stocks in each process have been valued at prime cost of the process. The following data are obtained at the end of 31 March 1998:

 

 

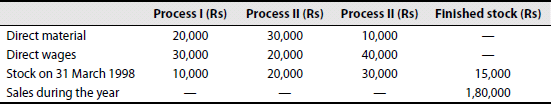
From this information, prepare

1. Process cost accounts showing profit element at each stage
2. A statement showing actual realized profit
3. A statement showing stock valuation for the purpose of balance sheet

Solution: Process I Account

 

 

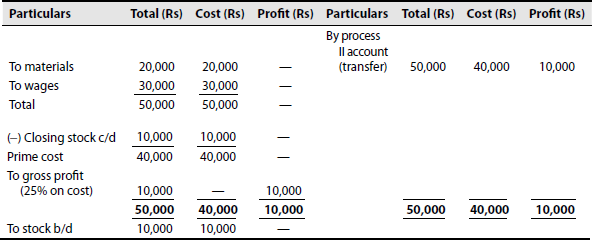
Process II Account

 

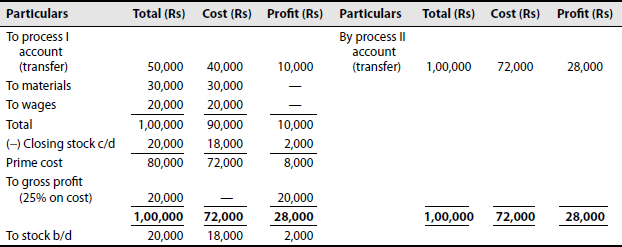
Process III Account

 

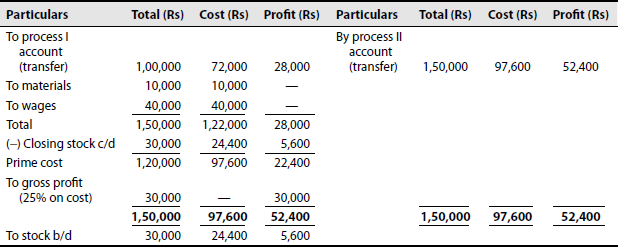
Finished Stock Account

 

 

Actual profit unrealized:

 

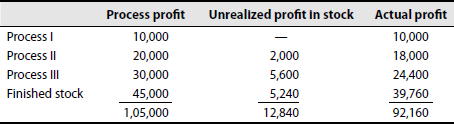
(c) Stocks for balance sheet:

Process I	10,000
Process II	18,000
Process III	24,400
Finished stock	9,760
Total	62,160

Illustration 14

From the following information, prepare process accounts:

 

 

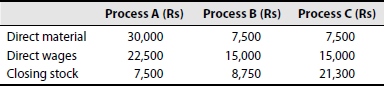
Finished goods were sold for Rs 1,30,000. The closing finished stock was Rs 5,612. It is the policy of the company to charge 20% on transfer price while transferring the goods from each process.

Solution: Process A Account

 

 

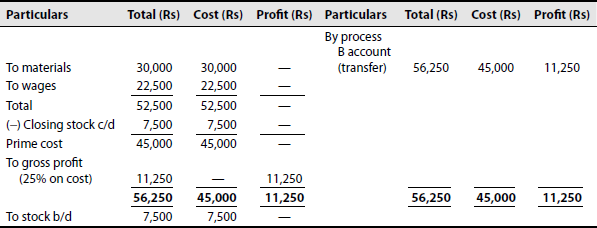
Process B Account

 

Process C Account

 

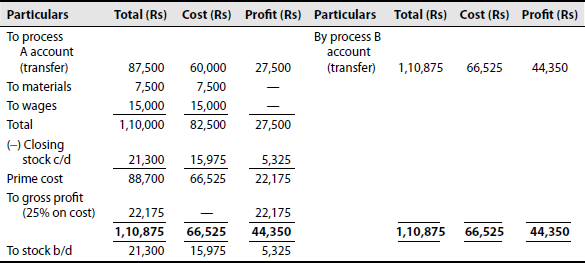
Finished Stock Account

 

 

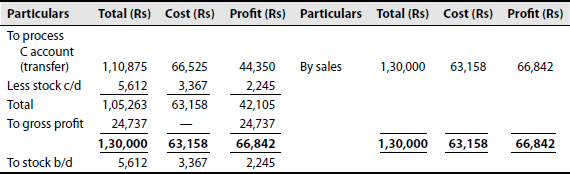
Actual profit unrealized:

 

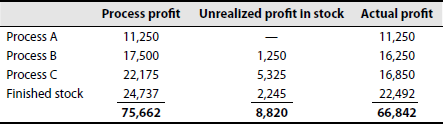
Stocks for balance sheet

Process A	7,500
Process B	7,500
Process C	15,975
Finished stock	3,367
Total	34,342

With opening and closing stock.

Illustration 15

The product of a manufacturing company passes through three processes to reach completion. Costing records give the following information for March 1998:

 

 

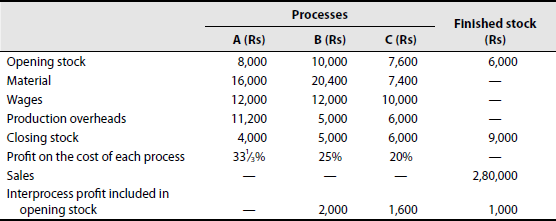
Stocks in the processes are valued at prime cost, and finished stock is valued at the price at which it is received from process C. Show the process accounts, finished stock account, realized profit and closing stock as shown in the balance sheet.

Solution: Process A Account

 

Process B Account

 

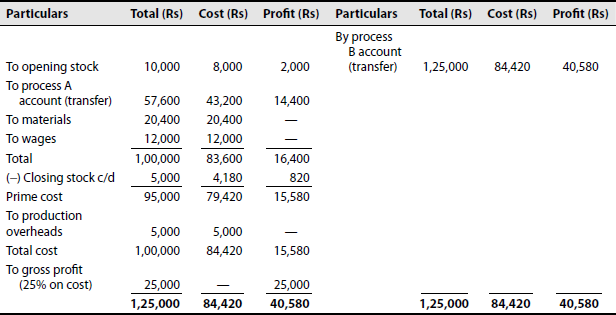
Process C Account

 

 

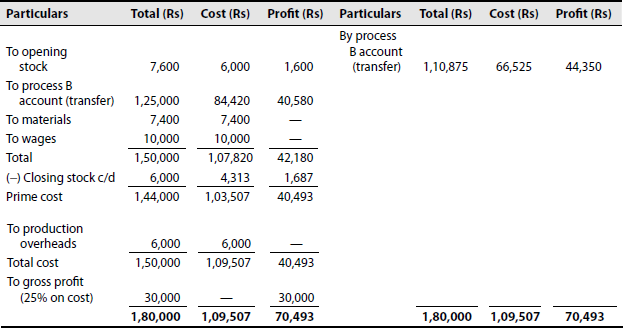
Finished Stock Account

 

Statement showing realized gross profit:

 

 

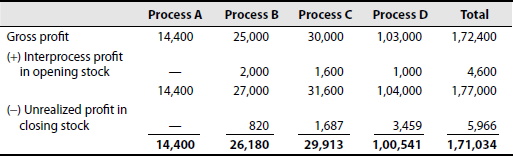
Stocks for balance sheet:

Process A	4,000
Process B	4,180
Process C	4,313
Finished stock	5,541
Total	18,034

Illustration 16

The manufacturing operations of S.K. Ltd involve two distinct processes before the product is completed and transferred to finished stock. The following data relate to December 1994:

 

 

The output of process I is transferred to process II at 33⅓% profit on the transfer price. The output of process II is transferred to finished stock at 20% profit on the transfer price. Stocks in processes are valued at prime cost. Finished stock is valued at the price at which it is received from process II. Sales during the month is Rs 14,000. Prepare process accounts and finished stock account showing the profit at each stage.

Solution: Process I Account

 

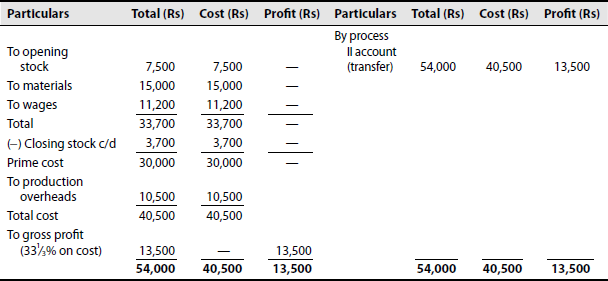
Process II Account

 

 

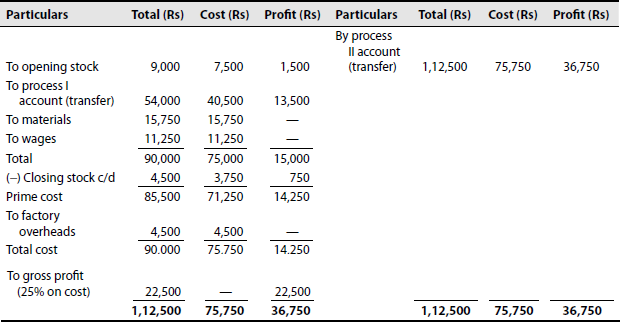
Finished Stock Account

 

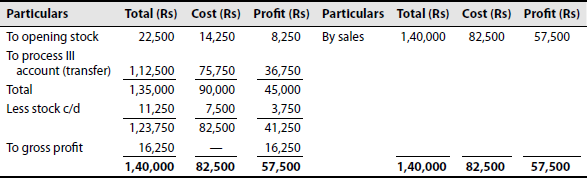
Statement showing realized gross profit:

 

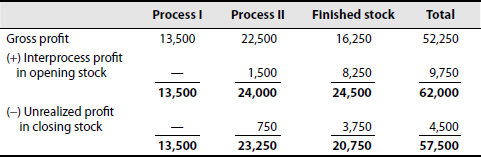
Stocks for balance sheet:

Process I	3,700
Process II	3,750
Finished stock	7,500
Total	14,950

12.12 EQUIVALENT PRODUCTION

Equivalent units are mainly used in process accounting systems, but the method can also be used in a job order system. Equivalent unit calculations are used at the end of a month to prepare monthly production reports. They are also used at the end of the year to determine ending inventory values. The equivalent unit concept has to do with costs incurred, in the form of materials, labour and overhead.

In process industries, production is on a continuous basis and the problem of work-in-progress in processes is quite common. Problems arise about the valuation of work-in-progress or unfinished units in the process. These problems can be solved by calculating equivalent production. Equivalent production represents the production in terms of completed units. It means converting incomplete production into its equivalent of completed units. In other words, equivalent production represents the output of a process expressed in terms of completed units.

Rarely are all units placed in production during the month completed and sent to the next department by the end of the month. In most cases, there are beginning and ending inventories of work-in-process at different stages of completion each month.

To allocate costs when inventories of partially finished goods exist, all units (beginning work-in-process inventory and ending work-in-process inventory) must be expressed in terms of completed units. This is done by means of a common denominator, known as equivalent units of production or equivalent production. By using the equivalent production figure, the unit cost for a month would include the cost of completing any work-in-process at the beginning of the month and the cost to date of work-in-process at the end of the month.

Two separate equivalent production computations are usually needed, one for direct materials and another for direct labour and factory overhead, known as conversion costs. There are two principal methods for costing work-in-process inventories: average costing and first-in first-out (FIFO) costing. There are minor differences in cost report format or procedure for the two methods; the major difference relates to the way in which the work-in-process inventories are treated.

The accounting procedure of equivalent production is as follows:

1. FIFO method: This method is based on the assumption that a material in process moves on a FIFO basis, which means that unfinished work on the opening stock is completed first before the new materials put into the process are taken up. It is assumed that no units from opening work-in-progress are left incomplete.

   For example, production data of process X for the month of July is given as follows:

   Units started in production = 60,000

   Opening inventory = 5,000 units (70% complete)

   Closing inventory = 7,500 units (60% complete)

   Calculate the equivalent units under FIFO method work-in-process.

   

2. Average cost method: Weighted average method blends together units and costs from the current period with units and costs from a prior period. In a weighted average method, the equivalent units of production for a department are the number of units transferred to the next department of finished goods plus the equivalent units in the department's ending work-in-process inventory.

   In this method, the cost of opening work-in-process is not kept separate but is averaged with the additional costs incurred during the period. The average process cost is obtained by adding the cost of beginning work-in-process to the cost put into the process during the period and dividing the total by total equivalent units. Solving the above sum.

   

In process industries, production is on a continuous basis and the problem of work-in-progress in processes is quite common. The problem arises about the valuation of work-in-progress or unfinished units in the process. This problem can be solved by calculating equivalent production.

Equivalent production represents the production in terms of completed units. It means converting the incomplete production into its equivalent of completed units.

In other words, equivalent production represents the output of a process expressed in terms of completed units.

Illustration 17

During January, 2,000 units were introduced into process I. The normal loss was estimated at 10% on input. By the end of the month, 1,400 units had been produced and transferred to the next process, 360 units were incomplete and 140 units had been scrapped. It was estimated that incomplete units had reached a stage in production as follows:

Material	75% completed
Labour	50% completed
Overheads	50% completed
The cost of 2,000 units was Rs 5,000.
Direct material introduced during the process amounted to Rs 2,040.
Direct wages amounted to Rs 3,240.
Production overheads incurred were Rs 1,620.
Units scrapped realized Re 1 each.
Units scrapped passed through the process, so they were 100% completed in terms of material, labour and overhead.

Find out (a) equivalent production, (b) cost per unit; and (c) show the necessary accounts.

Ans:

1. Statement of equivalent production

    

   

    

2. Statement of cost

    

   

    

3. Statement of evaluation

    

   

    

Process I Account

 

Illustration 18

Prepare statement of (a) equivalent production, (b) statement of cost and (c) process account from the following information:

Units introduced	7,600
Output (units)	6,000
Process cost
Materials	Rs 14,560
Labour	Rs 21,360
Overhead	Rs 14,240
Degree of completion of closing work-in-progress:
Material	80%
Labour	70%
Overhead	70%

Solution:

1. Statement of equivalent production

    

   

    

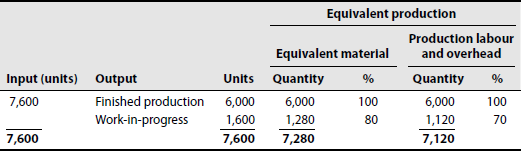
2. Statement of cost

    

   

    

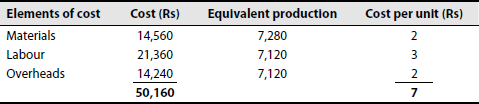
3. Statement of evaluation

    

   

    

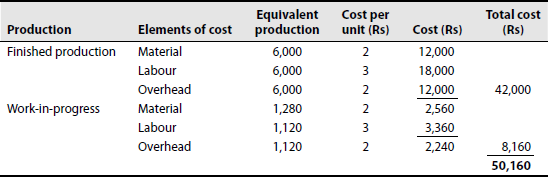
Illustration 19

From the following data, calculate (a) equivalent production, (b) cost per unit of equivalent production and (c) prepare the necessary accounts

Number of units introduced in the process = 4,000
Number of units completed and transferred to process B = 3,200
Number of units in process at the end of the period = 800
Stage of completion:
Material	80%
Labour	70%
Overheads	70%
Normal process loss at the end of the process = 200 units
Value of scrap = Re 1 per unit
Value of raw materials = Rs 7,480
Wages = Rs 10,680
Overheads = Rs 7,120

Solution:

1. Statement of equivalent production

    

   

    

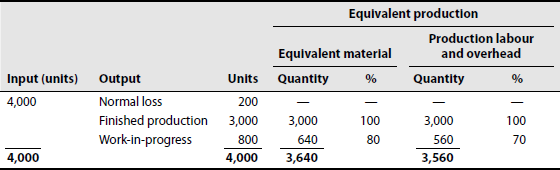
2. Statement of cost

    

   

    

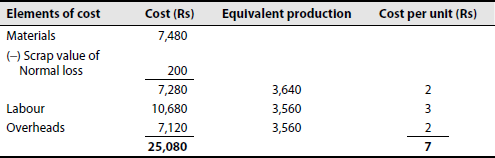
3. Statement of evaluation

    

   

    

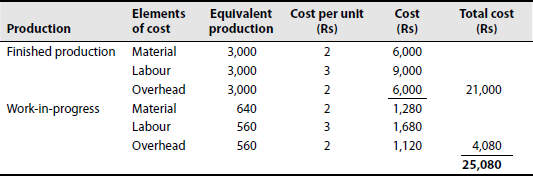
Process B Account

 

 

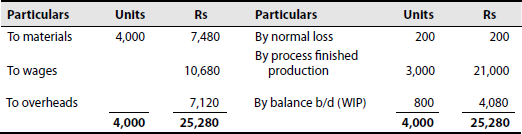
Illustration 20

From the following data, calculate (a) equivalent production, (b) cost per unit of equivalent production and (c) prepare the necessary accounts.

Number of units introduced in the process = 4,000
Number of units completed and transferred to process B = 3,200
Number of units in process at the end of the period = 800
Stage of completion:
Material	80%
Labour	70%
Overheads	70%
Normal process loss at the end of the process = 200 units
Value of scrap = Re 1 per unit
Value of raw materials = Rs 11,120
Wages = Rs 10,680
Overheads = Rs 14,240

Solution:

1. Statement of equivalent production

    

   

    

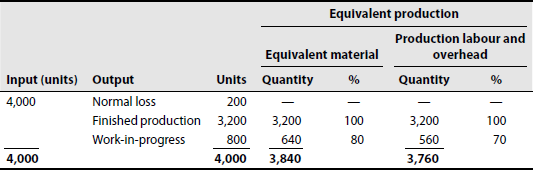
2. Statement of cost

    

   

    

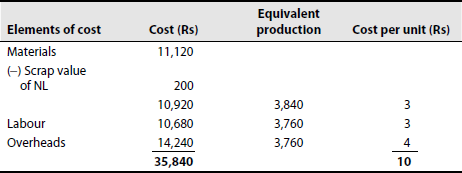
3. Statement of evaluation

    

   

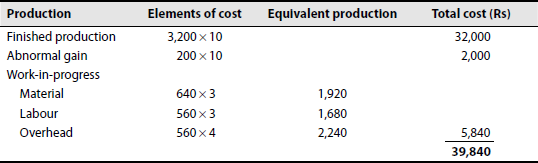
   Process A Account

    

   

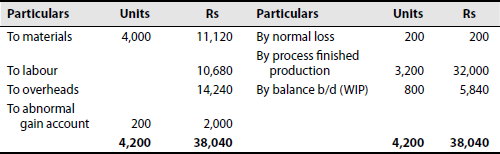
   Normal Loss Account

    

   

   Abnormal Gain Account

    

   

    

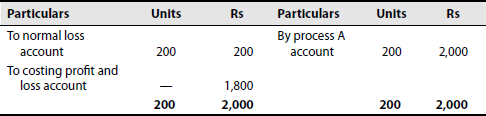
Illustration 21

From the following information, prepare

1. Statement of equivalent production
2. Statement of cost
3. Process II account

   Opening stock: 600 units amounting to Rs 1,050
   Degree of completion:
   Materials	80%
   Labour	60%
   Overheads	60%
   Transfer from process I: 11,000 units at Rs 5,500
   Transfer to process III: 8,800 units
   Direct materials added in process II amount to Rs 4,820.
   Direct labour amounts to Rs 9,540.
   Production overhead incurred amounts to Rs 9,540.
   Units scrapped: 1,200
   Degree of completion:
   Materials	100%
   Labour	70%
   Overhead	70%
   Closing stock:	1,600
   Degree of completion:
   Materials	70%
   Labour	60%
   Overhead	60%

   There was a normal loss in the process of 10% of production. Units scrapped were realized at 50 paise per unit.

Solution:

1. Statement of equivalent production

    

   

    

    

   

    

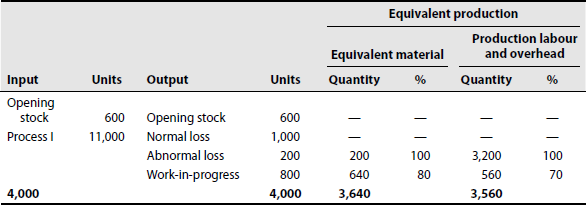
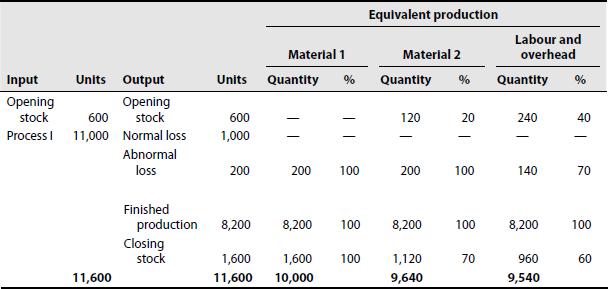
   Note: Material 2 indicates the material added in process II.

2. Statement of cost

    

   

Valuation of Production

 

 

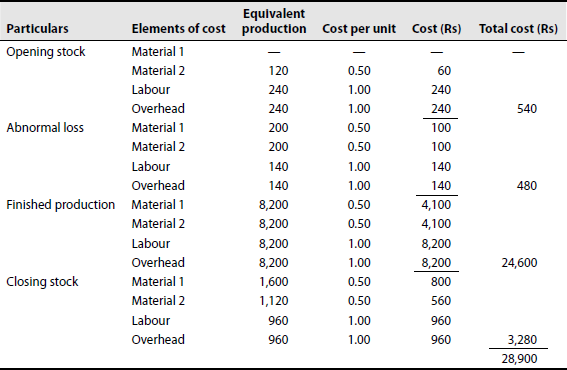
Process II Account

 

 

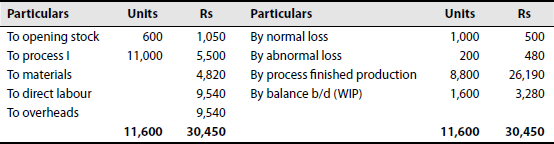
Illustration 22

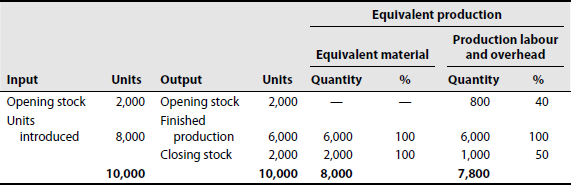
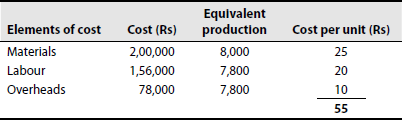
From the following details, prepare (a) statement of equivalent production, (b) statement of cost and (c) statement of evaluation.

Opening work-in-progress	2,000 units
Material (100% complete)	Rs 15,000
Labour (60% complete)	Rs 6,000
Overhead (60% complete)	Rs 3,000
Units introduced into the process = 8,000
There are 2,000 units in the process and the stage of completion is estimated to be
Material: 100%
Labour and overheads: 50%
8,000 units are transferred to next process.
The process costs for the period are as follows:
Material	Rs 2,00,000
Labour	Rs 1,56,000
Overheads	Rs 78,000

Solution:

1. Statement of equivalent production

    

   

    

2. Statement of cost

    

   

   Valuation of Production

    

   

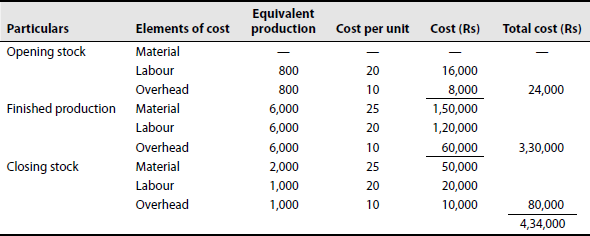
   Process II Account

    

   

    

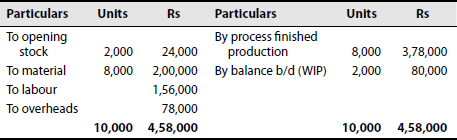   8,000 units at Rs 55 = Rs 3,30,000 2,000 units = Rs 2,48,000 (24,000 + 24,000) = Rs 3,78,000

Illustration 23

Calculate equivalent production for process I during October 1998 from the information furnished:

Opening stock = 4,000 units
Percentage of completion:
Material	75%
Labour	50%
Overhead	50%
Number of units introduced into the process = 50,000
Units scrapped, considered as normal = 5,000
Closing stock units = 6,000
Percentage of completion:
Materials	80%
Labour	60%
Overhead	60%

Solution: Statement of equivalent production

 

Illustration 24

From the following data, find equivalent production by applying FIFO method:

Opening work-in-progress = 2,000 units

Degree of completion:

Materials: 80%; labour: 60%; overhead: 60%; units introduced: 10,000

Closing work-in-progress = 3,000 units

Degree of completion:

Materials: 90%; labour: 70%; overhead: 70%

There is no process loss.

Solution: Statement of equivalent production

 

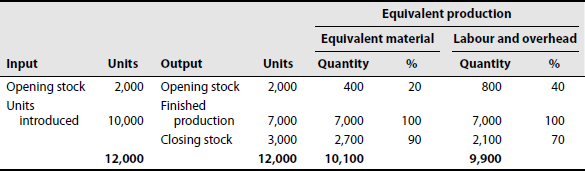
Illustration 25

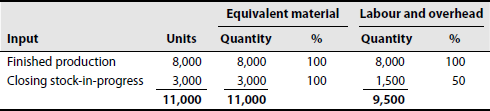
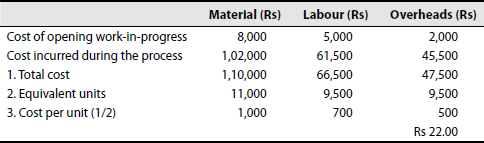
From the following details, (a) prepare statement of equivalent production, (b) statement of cost and (c) statement of evaluation and process account by applying average cost method

Opening work-in-progress = 3,000 units
Materials (100% complete)	Rs 8,000
Labour (60% complete)	Rs 5,000
Overhead (60% complete)	Rs 2,000
Units introduced into the process = Rs 8,000
There are 3,000 units in process, and the stage of completion is estimated to be
Material	100%
Labour	50%
Overheads	50%
It must be noted that 8,000 units are transferred to the next process.
The process costs for the period are as follows:
Material	Rs 1,02,000
Labour	Rs 61,500
Overheads	Rs 45,500

Solution:

1. Statement of equivalent production

    

   

    

2. Statement of cost

    

   

    

3. Statement of evaluation

   	Rs
   1. Value of output transferred (8,000 units at Rs 22)	1,76,000
   2. Value of closing WIP	48,000
   Material (3,000 at Rs 10) = Rs 30,000
   Labour (1,500 at Rs 7) = Rs 10,500
   Overhead (1,500 at Rs 5) = Rs 7,500
   	2,24,000

   Process A Account

    

   

    

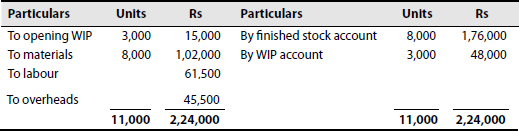
Illustration 26

Opening work-in-progress = 4,000 units
Material (100% complete): Rs 3,000
Labour (60% complete): Rs 5,000
Overheads (60% complete): Rs 8,000
Units introduced into the process: 9,000
Material completed	100%
Labour completed	50%
Overheads completed	50%
9,000 units are transferred to the next process.
Process cost for the month includes
Material: Rs 1,00,000
Labour: Rs 50,000
Overheads: Rs 80,000

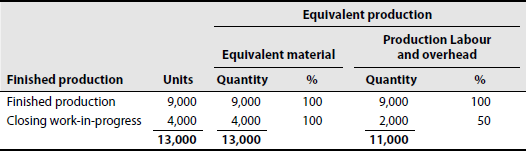
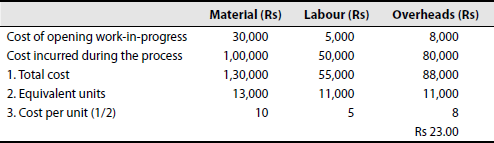
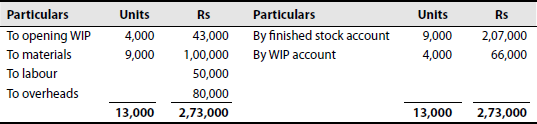
Prepare (a) equivalent production, (b) statement of cost, (c) statement of evaluation and (d) process account. Apply average cost method.

Solution:

1. Statement of equivalent production

    

   

    

2. Statement of cost

    

   

    

3. Statement of evaluation

   	Rs
   1. Value of output transferred (9,000 units at Rs 23.00)	2,07,000
   2. Value of closing WIP	66,000
   Material (4,000 at Rs 10) = Rs 40,000
   Labour (2,000 at Rs 5) = Rs 10,000
   Overhead (2,000 at Rs 8) = Rs 16,000
   	2,73,000

4. Process Account

    

   

    

12.13 JOINT PRODUCTS AND BY-PRODUCTS

Joint products are products produced simultaneously by a common process or series of processes, with each product processing more than a nominal value in the form in which it is produced. The term by-product is generally used to denote one or more products of relatively small total value that are produced simultaneously with a product of greater total value. The meaning of joint products and by-products are as follows: Agricultural product industries, chemical process industries, sugar industries and extractive industries are some industries where two or more products of equal or unequal importance are produced either simultaneously or in the course of the processing operation of a main product.

In all such industries, managements are faced with problems such as valuation of inventory, pricing of products and income determination and problem of making decisions in matters of further processing of by-products and/or joint products after a certain stage.

In fact, the various problems relate to (1) apportionment of common costs incurred for various products and (2) aspects other than mere apportionment of costs incurred up to the point of separation. Before taking up the aforementioned problems, we first define the various necessary concepts.

When two or more products of equal importance are simultaneously produced from the same raw material, such products are regarded as joint products.

12.13.1 Features of joint products

The major features of joint products are as follows:

1. Produced from the same raw material
2. Have comparatively equal importance
3. Produced simultaneously by a common process
4. Require further processing after their point of separation

In dairy industry, milk, butter, cheese, cream and ghee are joint products. In petroleum industry, petrol, diesel and kerosene are joint products.

12.13.2 By-products

By-products are defined as ‘products recovered from material discarded in a main process or from the production of some major products, where the material value is to be considered at the time of severance

from the main product’. Thus, by-products emerge as a result of the processing operation of another product, or they are produced from the scrap or waste of materials of a process. In short, a by-product is a secondary or subsidiary product that emanates as a result of manufacture of the main product. Examples of by-products are molasses, obtained in the manufacture of sugar; tar, ammonia and benzole, obtained in the carbonization of coal; and glycerin, obtained in the manufacture of soap.

By-products are products of comparatively small value that are produced incidental to the main product. They are jointly produced with other major products and remain inseparable up to the point of separation. For example, in sugar industry sugar is the main product and molasses are the by-product. In a rice mill, rice is the main product and husk is the by-product.

12.13.3 Difference between by-products and joint products

Major differences between by-products and joint products are as follows:

1. If products are of equal value, they are called joint products. If products are not of the same value, then the products of lesser value are known as by-products.
2. Additional expense is needed on joint products to make them finished goods.
3. Additional expense is not needed on by-products to make them finished goods as they already are finished goods.

12.14 SPLIT-OFF POINT/SEPARATION POINT

Joint products cannot be identified as separate products up to a certain stage in manufacturing. This stage is known as the split-off point or separation point. At this stage, joint products acquire separate identities. Costs incurred prior to this point are common costs, and any costs incurred after this point are separable costs.

12.15 JOINT COSTS

Costs prior to the split-off stage are known as joint costs. These costs cannot be identified with a particular joint product. Joint products incur common costs until they reach the split-off point.

12.16 SEPARATION COSTS

Costs after the split-off stage are known as subsequent costs. Costs before the split-off stage have to be distributed to each product.

Joint products and co-products are used synonymously in common parlance, but strictly speaking a distinction can be made between the two. Co-products may be defined as two or more products that are contemporary but do not necessarily emerge from the same material in the same process. For instance, wheat and gram produced from two separate farms with separate processes of cultivation are co-products. Similarly, timber boards made from different trees are co-products.

12.17 DIFFICULTIES IN COSTING POSED BY-PRODUCTS AND JOINT PRODUCTS

By-products and joint products are difficult to cost because a true joint cost is indivisible. For example, an ore might contain both lead and zinc. In the raw state of the ore these minerals are joint products, and until

they are separated by reduction of the ore the cost of finding mining and processing is a joint cost; neither lead nor zinc can be produced without the other prior to the split-off point.

Illustration 27

A company produces 600 units of product A, 150 units of product B and 250 units of product C by same process. The cost up to the separation point is Rs 40,000. Apportion the joint cost to all the products using average unit cost method.

Solution:

Apportionment of joint costs among products:

 

Product A = 600 × 40 = Rs 24,000

Product B = 150 × 40 = Rs 6,000

Product C = 250 × 40 = Rs 10,000

Total = Rs 40,000

Illustration 28

X Ltd produces three products from a joint process. Joint expenses are Rs 95,000. Units produced are 2,500; 1,000; and 1,500, respectively. Apportion the joint cost to all the products using average unit cost method.

Solution:

 

Apportionment of joint costs among products:

 

Product A = 2,500 × 19 = Rs 47,500

Product B = 1,000 × 19 = Rs 19,000

Product C = 1,500 × 19 = Rs 28,500

Total = Rs 95,000

PHYSICAL UNITS METHOD

Illustration 29

Product X produces four joint products A, B, C and D per tonne of X. Material: Rs 2,500; labour and overhead: Rs 3,000. Joint products yielded are A—600 units, B—400 units, C—300 units and D—200 units. Apportion the total cost to all the joint products. Apportion the joint cost under physical units method.

Solution: Statement showing apportionment of joint costs

 

 

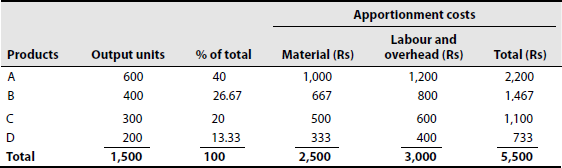
Illustration 30

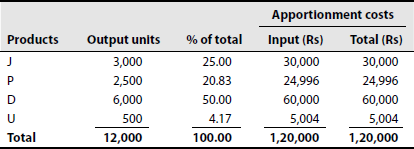
A company produces the following products by putting 6,000 units at Rs 20 per unit into a common process:

 

J: 3,000 units

D: 6,000 units

P: 2,500 units

U: 500 units

Apportion the joint cost under physical unit method.

Solution: Statement showing apportionment of joint costs

 

 

REVERSE COST/NET REALIZABLE VALUE METHOD

Illustration 31

A company produces three joint products A, B and C at a total cost of Rs 52,800. The subsequent costs of the products are Rs 5,000, Rs 10,000 and Rs 6,000, respectively.

 

Sales of A: Rs 60,000, profit margin of 25% on sales

Sales of B: Rs 50,000, profit margin of 20% on sales

Sales of C: Rs 80,000, profit margin of 30% on sales

Apportion the joint costs on the basis of net realizable value.

Solution: Statement showing apportionment of joint costs

 

 

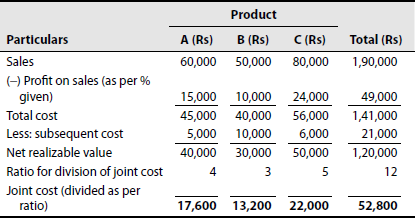
Illustration 32

Main product is X. By-product is Y.

 

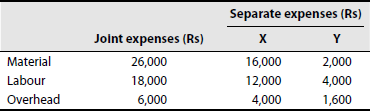
Units produced: X—800 kg and Y—200 kg. Selling price of Y is Rs 280 per kg and profit is 20% on selling price. Calculate the share in joint expenses and cost per unit.

Solution: Main Product X Account

 

By-product Y Account

 

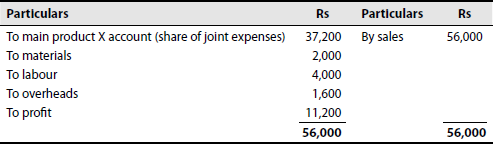
Working Note:

Calculation of joint cost of by-product Y

Sales value (200 × 280)	56,000
Less: profit at 20% on selling price	11,200
Cost of sales	44,800
Less: separate expenses (2,000 + 4,000 + 1,600)	7,600
Joint expenses of by-product Y	37,200

Illustration 33

In a manufacturing concern, a certain product A yields by-products B and C. The joint expenses of manufacture are as follows:

	Rs
Materials	12,500
Labour	10,000
Overheads	17,500

The subsequent expenses are as follows:

	A (Rs)	B (Rs)
Materials	600	700
Labour	1,300	900
Overhead	100	800
	2,000	2,400

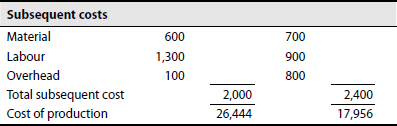
Selling prices are A—Rs 40,000 and B—Rs 32,000. Estimated profits on selling price are A—30% and B—30%. Show how you would apportion the joint costs of manufacture and prepare the products accounts.

Solution: Statement showing apportionment of joint cost

 

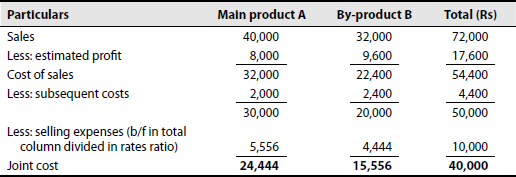
Statement showing cost of production of A and B

 

 

Total joint cost

 

 

CHAPTER SUMMARY

From this chapter, one is able to understand the relevance of process costing in fixing the cost of products. One is also able to understand the different stages of fixing the costs of products.

EXERCISE FOR YOUR PRACTICE

Objective-Type Questions

I. State whether the following statements are true or false

1. Process costing is one aspect of operation costing.
2. Process costing is applied in garment industry.
3. Process costing is applied in chemical works.
4. Normal loss does not increase the cost per unit of usual production.
5. Abnormal loss is spread on good units of production.
6. Abnormal gain should reduce normal loss.
7. In process costing, ordinarily no distinction is made between direct and indirect materials.
8. The cost of abnormal process loss is not included in the cost of a process.
9. The method of costing applied in biscuit industry is process costing.
10. When actual loss is more than estimated loss, the difference between the two is considered as abnormal gain.

[Ans: 1—true, 2—false, 3—true, 4—false, 5—false, 6—true, 7—true, 8—true, 9—false, 10—false]

II. Choose the correct answer

1. In process costing, cost follows
   1. Finished goods
   2. Product flow
   3. Price rise
   4. Price decline
2. Which of the following methods of costing can be used in a large oil refinery?
   1. Job costing
   2. Unit costing
   3. Process costing
   4. Operating costing
3. The type of process loss that should not affect cost of inventories is
   1. Standard loss
   2. Seasonal loss
   3. Normal loss
   4. Abnormal loss

4. Individual products, each of a significant value, produced simultaneously from the same raw material are known as
   1. By-products
   2. Joint products
   3. Main products
   4. Co-products
5. Credit is given to a process account at a predetermined value of the by-product under
   1. Points value method
   2. Sales value method
   3. Standard cost method
   4. Opportunity cost method
6. A bakery producing cakes, biscuits and breads should be treated as
   1. Joint product
   2. Main product
   3. By-product
   4. Co-product
7. Process costing is adopted by
   1. Paper mills
   2. Chemical industries
   3. Textile mills
   4. All the above
8. Avoidable losses arising from the nature of a productive process is termed as
   1. Normal loss
   2. Abnormal loss
   3. Net loss
   4. Gross loss
9. Products that cannot be produced separately are known as
   1. By-products
   2. Co-products
   3. Joint products
   4. Main product
10. The method of accounting for joint product cost that will produce the same gross profit for all the products is
    1. Reverse cost method
    2. Opportunity cost method
    3. Sales value method
    4. Other income method

    [Ans: 1. (b), 2. (c), 3. (d), 4. (b), 5. (c), 6. (a), 7. (d), 8. (a), 9. (c) 10. (c)]

DISCUSSION QUESTIONS

1. Discuss the features of process costing.
2. Name some industries where process costing is applied?
3. Write notes on
   1. Normal loss
   2. Abnormal loss
   3. Abnormal gain
4. State the differences between joint products and by-products.
5. What is the meaning of the term split-off point?
6. What are known as separate expenses and joint expenses?
7. Write a note on interprocess profits.
8. What do you mean by equivalent production?

PROBLEMS

1. Calculate the cost of each process and the total cost of production from the following data:

    

   

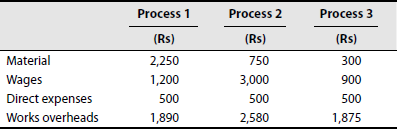
   Other indirect expenses of Rs 1,275 should be apportioned on the basis of wages.

   (Bharathidasan University, 1998)

   [Ans: Process 1—Rs 6,140; process 2—Rs 13,720; process 3—Rs 17,520]

2. A particular brand of scent passed through three important processes. During the week ending on 15 January 1987, 600 bottles were produced. The costbooks show the following information:

    

   

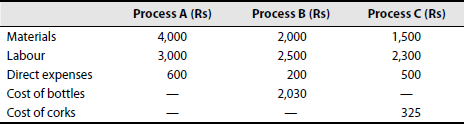
   The indirect expenses for the period were Rs 1,600 (indirect expenses are charged on labour basis). The by-products were sold for Rs 240 (process B). The residue was sold for Rs 125.50 (process C). Prepare the account with respect to each process, showing its cost and cost of production of the finished product per bottle.

   (Calicut, B.Com., April 1991)

   [Ans: Cost of production: process A—Rs 8,215; process B—Rs 15,218; process C—Rs 20,190. Cost per bottle: process A—13.69; process B—25.36; process C—33.65. Indirect expenses: process A—615; process B—513; process C—472]

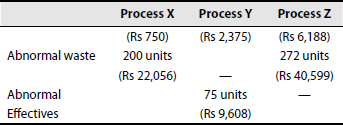
3. A product passes through three processes X, Y and Z. The normal waste of each process was 3%, 5% and 8% for X, Y and Z, respectively. The waste of process X was sold at Rs 2.50 per unit, that of Y at Rs 5 per unit and that of Z at Rs 8.50 per unit. 10,000 units were issued to process X on 1 July at a cost of Rs 100 per unit. The other expenses were as follows:

    

   

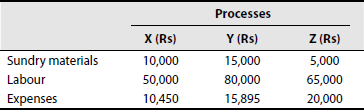
   The actual outputs were 9,500 units; 9,100 units and 8,100 units for X, Y and Z, respectively. Prepare process accounts assuming that there are no opening or closing stocks.

   (Madras, 1998)

   Ans:

   

    

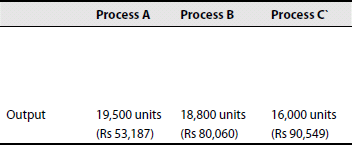
4. The product of a company passes through three distinct processes to reach completion. From past experience it is ascertained that wastage is incurred in each process as under process A—2%, process B—5% and process C—10%. The wastage of processes A and B is sold at Rs 10 per 100 units and that of process C at Rs 80 per 100 units. Following is information regarding the production of March 1994:

    

   

   20,000 units have been issued to process A at a cost of Rs 20,000. The output of each process is as under process A—19,500 units; process B—18,800 units; and process C—16,000 units. There was no stock or work-in-progress in any process in the beginning and the end of March. Prepare process account.

   (Madras, 1987)

   Ans:

   

    

5. From the following information, prepare process cost accounts and normal loss, abnormal loss or gain accounts:

   	Process A (Rs)	Process B (Rs)
   Material	30,000	3,000
   Labour	10,000	12,000
   Overheads	7,000	8,600
   Input (units)	20,000	17,500
   Normal loss	10%	4%
   Sale of waste per unit (Rs)	1	2
   Final output from process B (units)	—	17,000

   (Madras, 1989)

   [Ans: Process A: abnormal loss—units 500, value Rs 1,250; transfer—17,500 units at Rs 2.5 each = Rs 43,750. Process B: abnormal gain—units 200, value Rs 785; transfer—17,000 units at Rs 3.9256 = Rs 66,735]

6. A product passes through three process I, II and III. From the following information, prepare the process accounts assuming that there are no opening or closing stocks:

    

   

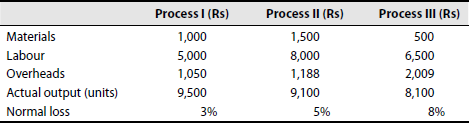
   The wastage of process I was sold at 25 paise per unit, that of process II at 50 paise per unit and that of process III at Re 1 per unit. Raw materials of 10,000 units were introduced into process I in the beginning at a cost of Re 1 per unit.

   (Madras, 1984)

   [Ans: Process I: abnormal loss—units 200, value Rs 350; transfer to process II—9,500 units at Rs 1.75 each = Rs 16,625. Process II: abnormal gain—units 75, value Rs 225; transfer to process III—9,100 units at Rs 3 each = Rs 27,300. Process III: abnormal loss—units 272, value Rs 1,156; transfer to finished stock—8,100 units at Rs 4.25 each = Rs 34,425]

7. The product of a company passes through three distinct processes to reach completion. They are A, B and C. From past experience it is ascertained that loss is incurred in each process as follows: Process A—2%, process B—5%, process C—10%.

   In each case, the percentage of loss is computed on the number of units entering the process concerned. The loss of each process possesses a scrap value. The loss of processes A and B is sold at Rs 5 per 100 units and that of process C at Rs 20 per 100 units.

    

   

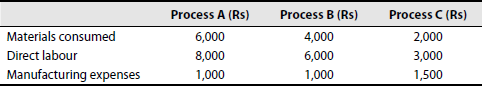
   20,000 units have been issued to process A at a cost of Rs 10,000. The output of each process is as under process A—19,500 units; process B—18,800 units; and process C—16,000 units. There is no work-in-progress in any process. Prepare process accounts. Calculations should be made to the nearest rupee.

   (Madras, 1991)

   [Ans: Process A: abnormal loss—units 100, value Rs 127; transfer to process B—19,500 units at Rs 1.2745 each = Rs 24,853. Process B: abnormal gain—units 275, value Rs 532; transfer toprocess C = 18,800 units at Rs 1.9327 each = Rs 36,336. Process C: abnormal loss—units 920, value Rs 2,309; transfer to finished stock—16,000 units at Rs 2.5093 each = Rs 40,151]

8. A product passes through three processes. The following data relate to the three processes during September 1998:

    

   

   500 units at Rs 4 per unit were introduced in process I. Production overhead is absorbed in the ratio of labour. Prepare process accounts, abnormal loss and abnormal gain accounts.

   (Madras, 1986)

   [Ans: Process I: transfer to process II—450 units at Rs 20 each = Rs 9,000. Process II: abnormal loss—units 20, value Rs 1,000; transfer to process III—340 units at Rs 50 each = Rs 17,000. Process III: abnormal gain—units 15, value Rs 1,200; transfer to finished stock—270 units at Rs 80 each = Rs 21,600. Abnormal loss transferred to profit and loss account—Rs 920; abnormal gain transferred to profit and loss account—Rs 1,125]

9. In a factory, the product passes through two processes A and B. A loss of 5% is allowed in process A and 2% in process B, nothing being realized by disposal of the wastage. During April, 10,000 units of material costing Rs 6 per unit were introduced into process A. The other costs were as follows:

   	Process A (Rs)	Process B (Rs)
   Materials	—	6,140
   Labour	10,000	6,000
   Overheads	6,000	4,600

   The output was 9,300 units from process A; 9,200 units were produced by process B, which were transferred to a warehouse. 8,000 units of the finished product were sold at Rs 15 per unit, the selling and distribution expenses being Rs 2 per unit. Prepare process accounts and a statement of profit or loss of the firm for April, assuming there are no opening stocks of any type.

   (B.Com., Karnataka)

   [Ans: Process A: 9,300 units at Rs 8 per unit; process B: 9,200 units at Rs 10 per unit; profit on sales: Rs 24,000; final profit: Rs 24,000 − 1,600 + Rs 860 = Rs 23,260]

10. The product of a manufacturing concern passes through two processes A and B and then to finished stock. It is ascertained that in each process normally 5% of the total weight is lost and 10% is scrap from which processes A and B realizes Rs 80 per tonne. The following are the figures relating to both the processes:

    	Process A (Rs)	Process B (Rs)
    Materials in tonnes	1,000	70
    Cost of materials in rupees per tonne	125	200
    Wages in rupees	28,000	10,000
    Manufacturing expenses in rupees	8,000	5,250
    Output in tonnes	830	780

    Prepare process cost accounts showing cost per tonne of each process. There was no stock of work-in-progress in any process.

    (Madras, 1995)

    [Ans: Process A: 830 units at Rs 180; abnormal loss: 20 units; process B: 780 units at Rs 210; abnormal gain: 15 units]

11. A product passes through three processes, processes I, II and III. 15,000 units of crude material were introduced into process I at Re 1 per unit. Additional information is as follows:

     

    

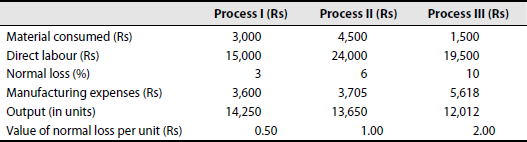
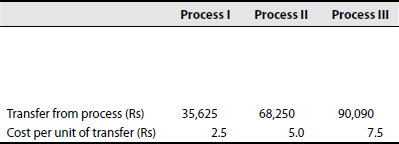
    Prepare process cost accounts and normal loss, abnormal loss and abnormal gain accounts.

    (Calicut, 1994)

    Ans:

    

     

12. A product passes through three processes A, B and C. The details of expenses incurred on the three processes during the year 1992 are as follows:

     

    

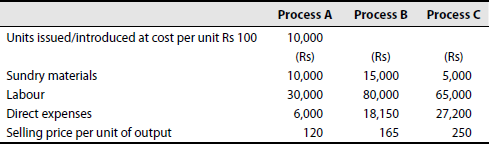
    Management expenses during the year were Rs 80,000 and selling expenses were Rs 50,000. These are not allocable to the processes. The actual outputs of processes A, B and C were 9,300 units; 5,400 units; and 2,100 units, respectively. Two-thirds of the output of process A and one half of the output of process B were passed on to the next process and the balance was sold. The entire output of process C was sold. The normal losses of the three processes, calculated on the inputs of processes, were as follows: process A—5%, process B—15% and process C—20%. The loss of units in process A was sold at Rs 2 per unit, that of B at Rs 5 per unit and that of process C at Rs 10 per unit. Prepare process accounts and profit and loss accounts.

    (B.Com., Karnataka)

    [Ans: Process A: abnormal loss—200 units at Rs 110 per unit, value Rs 22,000; transfer to process B—6,200 units at Rs 110 each = Rs 6,82,000; transfer to finished stock for sale—3,100 units, Rs 3,41,000. Process B: abnormal gain—130 units, value Rs 19,500; transfer to process C—2,700 units at Rs 150 each = Rs 4,05,000; transfer to finished stock for sale—2,700 units, Rs 4,05,000. Process C: abnormal loss—60 units, value Rs 13,800; transfer to finished stock for sale—2,100 units at Rs 230 each = Rs 4,83,000. Loss shown in profit and loss account—Rs 16,500 excluding abnormal loss and gain]

13. M/s. XYZ Co. has a single process.

    Work-in-progress (opening) = 8,000 units

    		Rs
    Cost:	Materials	29,600
    	Wages	6,600
    	Overheads	5,800

    During the period, the input was 32,000 units. Additional cost data is as follows:

    	Rs
    Materials	1,12,400
    Wages	33,400
    Overheads	30,200

    At the end of the year, 28,000 units were fully processed and 12,000 units were still in progress. The value of closing stock included the full cost of materials as well as one-third of the cost of wages and overheads. Tabulate the production and cost figures to give quantities, unit values and total values of the completed output and the detailed values of the closing work-in-progress.

    (Madras, 1997)

    [Ans: Equivalent units: materials—Rs 40,000; labour and overheads—Rs 32,000 each; and costs per unit of Rs 3.55, Rs 1.25 and Rs 1.13 as per average method used]

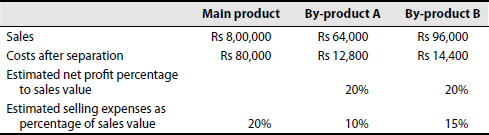
14. In manufacturing the main product, a company processes the incidental waste into two by-products A and B. From the following data relating to the products, you are required to prepare a comparative profit and loss statement showing individual costs and other details. The total cost up to separation point was Rs 3,10,400.

     

    

    (Madras, 1987)

    [Ans: Profit: main product = Rs 3,20,000; by-product A = Rs 12,800; by-product B = Rs 12,800]

JOINT PRODUCTS AND BY-PRODUCTS

I. Methods of Apportioning Cost to Joint Products

15. Sujatha Industries produces three products X, Y and Z from a joint processing operation. The cost before separation amounted to Rs 1,25,000. The outputs of X, Y and Z were 5,000; 6,000; and 1,500 units, respectively. Apportion the joint cost among products on the basis of average unit cost method.

(B.Com., Delhi)

[Ans: Average unit cost = Rs 10. Apportioned joint costs for X is Rs 50,000; for Y is Rs 60,000; and for Z is Rs 15,000]

16. A coke manufacturing company produces the following products by putting 5,000 tonnes of coal at Rs 25 per tonne into the common process:

Coke: 3,500 tonnes

Tar: 1,200 tonnes

Sulphate: 52 tonnes

Benzol: 48 tonnes

Apportion the joint cost among the products on the basis of physical units method.

[Ans: Apportioned joint cost—coke: Rs 91,146; tar: Rs 31,250; sulphate: Rs 1,354; benzol: Rs 1,250]

17. The following data have been extracted from the books of M/s. East India Coke Company Ltd:

	Yield (Rs) of recovered products per tonne of coal
Coke	1,420
Coal tar	120
Benzol	22
Sulphate of ammonia	26
Gases	412
Total	2,000

The price of coal is Rs 80 per tonne. Direct labour and overhead costs to the point of split-off are Rs 40 and Rs 60, respectively, per tonne of coal. Calculate material, labour overhead and total costs of each product on the basis of weight.

[Ans: Apportioned joint cost: coke—Rs 127.80; coal tar—Rs 10.80; benzol—Rs 1.98; sulphate of ammonia—Rs 2.34; gases—Rs 37.08]

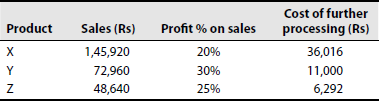
18. A company producing product X yields two by-products Y and Z. The following particulars relate to a particular period of operation in which the joint cost amounted to Rs 1,40,000:

 

The company apportions selling expenses to X, Y and Z in the ratio 10:1:7. Calculate the profit from the sales of each product and the joint cost applied to each product.

(B.Com., Calicut)

[Ans: Profit: X—Rs 29,184; Y—Rs 21,888; Z—Rs 12,160. Selling expenses: X—Rs 6,100; Y—Rs 610; Z—Rs 4,270. Total: Rs 10,980 (balancing figure and total cost). Joint cost: X—Rs 74,620; Y—Rs 39,462; Z—Rs 25,918]

19. Vasanth Ltd manufactures product A, which yields two by-products B and C. The actual joint expense of manufacture for a period was Rs 8,000. Subsequent expenses and other data are as follows:

 

Prepare a statement showing apportionment of joint costs to the main product and the by-products.

(B.Com., Calicut)

[Ans: Apportioned joint cost: A—Rs 3,571; B—Rs 2,548; C—Rs 1,881. Ratio of apportionment: 3,750:2,675:1,975]

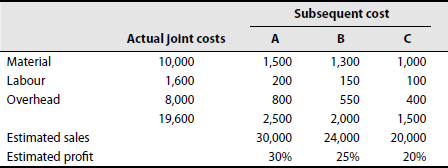
20. A factory produces an article A; this process yields B and C as by-products. The costs are as follows:

 

Show how you would apportion the joint cost of manufacture.

 

[Ans: Apportioned joint cost: A—Rs 7,400; B—Rs 6,400; C—Rs 5,800. Ratio of apportionment = 18.5:16:14.5]

21. A factory producing an article A also produces a by-product B, which is further processed into finished product. The joint cost of manufacture is given as follows:

Material	50,000
Labour	30,000
Overheads	20,000

Subsequent costs are given as follows:

	A	B
	(Rs)	(Rs)
Material	30,000	15,000
Labour	14,000	10,000
Overheads	6,000	5,000
	50,000	30,000
Selling prices	1,60,000	80,000

Estimated profits on sales are 25% for A and 290% for B. It is assumed that selling and distribution expenses are in proportion to sales prices. Show how you would apportion joint costs of manufacture and prepare a statement showing the cost of production of A and B.

 

[Ans: Joint cost of manufacture: A—Rs 67,333; B—Rs 32,667. Selling and distribution expenses: Rs 4,000; cost apportioned: A—Rs 2,667; B—Rs 1,333]

22. A factory producing an article P also produces a by-product Q, which is further processed into finished product. The joint cost of manufacture is as follows:

	Rs
Materials	5,000
Labour	3,000
Overheads	2,000
Total	10,000

Subsequent costs are as follows:

	P	Q
	(Rs)	(Rs)
Material	3,000	1,500
Labour	1,400	1,000
Overheads	600	500
	5,000	3,000

Selling prices are as follows: P—Rs 16,000 and Q—Rs 8,000. Estimated profits on selling prices are 25% for P and 20% for Q. Assume that selling and distribution of expenses are in proportion to the sales price. Show how you would apportion joint cost of manufacture, and prepare a statement showing the cost of production of P and Q.

(Bharathidasan, B.Com., April 1991)

[Ans: Cost of production of product P is Rs 11,733 and that of product Q is Rs 6,267]

23. AB Co. Ltd manufactures product A, which yields two by-products B and C. The actual joint expenses of manufacturing for a period were Rs 8,200. The profits on each product as a percentage of sales are 33⅓%, 25% and 15%, respectively. Subsequent expenses are as follows:

 

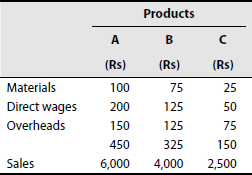
Show how you would apportion the joint expenses of manufacture. (CA)

[Ans: Share of joint expenses: A—Rs 3,550; B—Rs 2,675; C—Rs 1,975]

24. Calculate the estimated costs of production of by-products X and Y at the point of separation from the main product.

	By-product	By-product
	X	Y
Selling price per unit	Rs 12	Rs 24
Cost per unit after separation from the main product	Rs 3	Rs 5
Units produced	500	200

Selling expenses amount to 25% of total works cost, that is, including both pre-separation and post-separation works costs. Selling prices are arrived at by adding 20% of total cost, that is, the sum of works cost and selling expenses.

(Madras, B.Com., (ICE) May 1999)

[Ans: Pre-separation cost: by-product X—Rs 5 per unit or total Rs 2,500; by-product Y—Rs 11 per unit or total Rs 2,200]

25. During a month, 2,000 units of raw materials at a cost of Rs 9,500 were issued to process A. At the end of the month 1,500 units had been produced; 300 units were still in process; and 200 units had been scrapped. A normal wastage of 5% is allowed. The work-in-progress is complete:

100% with respect to raw materials

75% with respect to other materials

50% with respect to labour and overheads

The total costs incurred were (in addition to raw materials) as follows:

	Rs
Materials	1,825
Direct wages	3,500
Overheads	2,725

A scrapped unit realizes Re 1. Prepare the process account.

 

[Ans: Equivalent units: materials—1,900; other materials—1,825; labour and overheads—1,750 each. Costs per unit: Rs 4.94, Re 1, Rs 2, Rs 1.56]

26. During January 2,000 units were introduced into process I. The normal loss was estimated at 5% on input. At the end of the month 1,400 units had been produced and transferred to the next process. 460 units were incomplete and 140 units had been scrapped. It was estimated that the incomplete units had reached a stage in production as follows:

Material: 75% completed

Labour: 50% completed

Overhead: 50% completed

The cost of 2,000 units was Rs 5,800. Direct materials introduced during the process amounted to Rs 1,440. Direct wages amounted to Rs 3,340. Production overheads incurred were Rs 1,670. Units scrapped realized Re 1 each. The units scrapped had passed through the process; so they were 100% complete with respect to material, labour and overhead. Prepare a statement of equivalent production, a statement of cost and the process I account.

(I.C.W.A. Inter, June 1993)

[Ans: Equivalent units: materials—Rs 4; overheads—1,670. Cost per unit: materials—Rs 4; labour—Rs 2; overheads—Re 1. Value of finished units—Rs 9,800; closing work-in-progress—Rs 2,070; abnormal loss—Rs 280; total of process account—Rs 12,250]

27. From the following details, prepare a statement of equivalent production and a statement of cost, and find the value of the following:

Output transferred

Closing work-in-progress, by the average cost method

Opening work-in-progress	2,000 units
Materials (100% complete)	Rs 7,500
Labour (60% complete)	Rs 3,000
Overheads (60% complete)	Rs 1,500
Units introduced into the process	8,000

There are 2,000 units in process and the stage of completion is estimated to be as follows: materials—100%, labour—50% and overheads—50%. 8,000 units are transferred to next process. The process costs for the period are as follows:

materials—Rs 1,00,000; labour—Rs 78,000; overheads—Rs 39,000.

[Ans: Equivalent units: materials—10,000; labour—9,000; overheads—9,000. Cost per unit: material—Rs 10.75; labour—Rs 9; overheads—Rs 4.50. Value of finished units transferred to next process—Rs 1,94,000. Value of closing work-in-progress—Rs 35,000]

28. Calculate equivalent production from the following data:

20,000 units of work-in-progress were there in process I on 1 January 1997 and it was 60% complete.

50,000 units were introduced into the process during January 1997.

56,000 units were fully finished and transferred to process II.

14,000 units of work-in-progress, completed to the extent of 40%, were there in the process on 31 January 1997.

Assume that FIFO method is followed in the process.

 

[Ans: Equivalent production: 49,600 units (8,000 + 36,000 + 5,600]

29. In process I, opening work-in-progress in February 1989 was 200 units, 40% complete. 1,050 units were introduced during the period. 1,100 completed units were transferred to process II and 150 units remained as closing work-in-progress, 70% complete. Compute equivalent production and apportion the total process cost of Rs 2,250 to production and work-in-progress inventories under the FIFO method.

[Ans: Equivalent production: 1,125 units; opening work-in-progress: 120 units; units completely processed: 900 units; closing work-in-progress: 105 units; cost of closing work-in-progress: Rs 210; cost of finished output: Rs 2,040]

30. The following information is given for process I for B Ltd. The average method of pricing work-in-progress is used.

Work-in-progress in January:

	Rs
Materials on 500 units	900
Labour on 500 units	1,000
Factory overheads on 500 units	400
Total	2,300

Production costs for January:

	Rs
Materials	7,800
Labour	9,150
Factory overheads	6,125
Cost for January	23,075

Production completed during January:

500 units from 1 January, work-in-progress

2,000 units from products received during January

2,500 total units completed

Work-in-progress on 31 January: 400 units, 25% completed as to material, labour and overhead.

Calculate process I account for January.

(Madras, B.Com., C & M, Oct. 1990)

[Ans: Equivalent production (WIP) of 400 units = 100 units at Rs 9.75 = Rs 975]