7 Topics in Analytic Geometry
In This Chapter
Planets, asteroids, and some comets revolve around the Sun in elliptical orbits
A Bit of History Hypatia is the first woman in the history of mathematics about whom we have considerable knowledge. Born in Alexandria (circa 370 CE), she was renowned as a mathematician, philosopher, and prophetess. Her life and untimely death at the hands of a fanatical mob are romanticized in an 1853 novel by Charles Kingsley (Hypatia, or New Foes with Old Faces, Chicago: W. B. Conkey, 1853). Among her writings is On the Conics of Apollonius, which popularized Apollonius' work on curves that can be obtained by intersecting a cone with a plane: the circle, parabola, ellipse, and hyperbola. With the close of the Greek period, interest in the conic sections waned and, after Hypatia, study of these curves was neglected for over 1000 years. In the seventeenth century, Galileo Galilei (1564-1642) showed that in the absence of air resistance the path of a projectile follows a parabolic arc. About the same time, the astronomer, astrologer, and mathematician Johannes Kepler (1571-1630) hypothesized that the orbits of planets about the Sun are ellipses with the Sun at one focus. This was later verified by Newton, using the methods of the newly discovered calculus. Kepler also experimented with the reflecting properties of parabolic mirrors; these investigations sped the development of reflecting telescopes. The Greeks had known little of these practical applications. They had studied the conics for their beauty and fascinating properties.
In this chapter, we examine both the ancient properties and the modern applications of these curves.
7.1 The Parabola
Hypatia
Introduction As mentioned in the introduction to this chapter, the Greek mathematician Hypatia popularized Apollonius' (200 BCE) work on curves that can be obtained by intersecting a double-napped cone with a plane: the circle, ellipse, parabola, and hyperbola. See FIGURE 7.1.1.
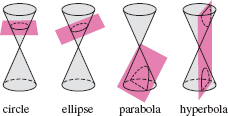
Conic sections
But the Greeks knew little of the practical applications of these conic sections. They had studied the conics for their beauty and fascinating properties. In the first three sections of this chapter, we will examine both the ancient properties and the modern applications of these curves. Rather than using a cone, we will see how the parabola, ellipse, and hyperbola are defined by means of distance. Using a rectangular coordinate system and the distance formula, we obtain equations for the conics. Each of these equations will be in the form of a quadratic equation in variables x and y:
Solar system
where A, B, C, D, E, and F are constants. We have already studied the special case y = ax2 + bx + c of the foregoing equation in Section 4.3.
FIGURE 7.1.2 A parabola
A parabola is shown in FIGURE 7.1.2. The line through the focus perpendicular to the directrix is called the axis of the parabola. The point of intersection of the parabola and the axis is called the vertex, denoted by the symbol V in FIGURE 7.1.2.
Parabola with Vertex (0, 0) To describe a parabola analytically, we use a rectangular coordinate system where the directrix is a horizontal line y = - c, where c > 0, and the focus is the point F(0, c). Then we see that the axis of the parabola is along the y-axis, as FIGURE 7.1.3 shows. The origin is necessarily the vertex, since it lies on the axis c units from both the focus and the directrix. The distance from a point P(x, y) to the directrix is
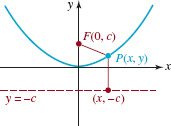
FIGURE 7.1.3Parabola with vertex (0, 0) and focus on the y-axis
Using the distance formula, the distance from P to the focus F is
From the definition of the parabola it follows that d(P, F) = y + c, or
By squaring both sides and simplifying, we obtain
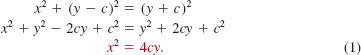
or
Equation (1) is referred to as the standard form of the equation of a parabola with focus (0, c), directrix y = -c, c > 0, and vertex (0, 0). The graph of any parabola with standard form (1) is symmetric with respect to the y-axis.
Equation (1) does not depend on the assumption that c > 0. However, the direction in which the parabola opens does depend on the sign of c. Specifically, if c > 0, the parabola opens upward as in FIGURE 7.1.3; if c < 0, the parabola opens downward.
If the focus of a parabola is assumed to lie on the x-axis at F(c, 0) and the directrix is x = - c, then the x-axis is the axis of the parabola and the vertex is (0, 0). If c > 0, the parabola opens to the right; if c < 0, it opens to the left. In either case, the standard form of the equation is
The graph of any parabola with standard form (2) is symmetric with respect to the x-axis.
A summary of all this information for equations (1) and (2) is given in FIGURE 7.1.4 and FIGURE 7.1.5, respectively. You may be surprised to see in Figure 7.1.4(b) that the directrix above the x-axis is labeled y = - c and the focus on the negative y-axis has coordinates F(0, c). Bear in mind that in this case the assumption is that c < 0 and so -c > 0. A similar remark holds for Figure 7.1.5(b).
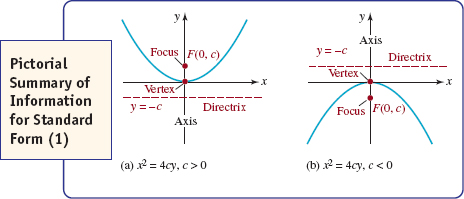
Summary of information for standard form (1)
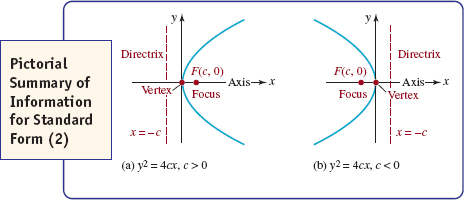
Summary of information for standard form (2)
7.1 The Parabola
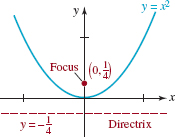
Graph of equation in Example 1
Graphing tip for equations (1) and (2). 
EXAMPLE 1 The Simplest Parabola
We first encountered the graph of y = x2 in Section 3.2. By comparing this equation with (1) we see
and so 4c = 1 or c = 
. Therefore the graph of y = x2 is a parabola with vertex at the origin, focus at (0, ), and directrix y = -. These details are indicated in the graph in FIGURE 7.1.6.
Knowing the basic parabolic shape, all we need to know to sketch a rough graph of either equation (1) or (2) is the fact that the graph passes through its vertex (0, 0) and the direction in which the parabola opens. To add more accuracy to the graph it is convenient to use the number c determined by the standard form equation to plot two additional points. Note that if we choose y = c in (1), then x2 = 4c2 implies x = ±2c. Thus (2c, c) and (-2c, c) lie on the graph of x2 = 4cy. Similarly, the choice x = c in (2) implies y = ±2c, and so (c, 2c) and (c, -2c) are points on the graph of y2 = 4cx.The line segment through the focus with endpoints (2c, c), (-2c, c) for equations with standard form (1), and (c, 2c), (c, -2c) for equations with standard form (2) is called the focal chord. For example, in FIGURE 7.1.6, if we choose y = , then x2 = implies x = ±
. Endpoints of the horizontal focal chord for y = x2 are (-, ) and (, ).
EXAMPLE 2 Finding an Equation of a Parabola
Find the equation in standard form of the parabola with directrix x = 2 and focus (-2, 0). Graph.
Solution In FIGURE 7.1.7 we have graphed the directrix and the focus. We see from their placement that the equation we seek is of the form y2 = 4cx. Since c = -2, the parabola opens to the left and so
As mentioned in the discussion preceding this example, if we substitute x = c, or in this case x = -2, into the equation y2 = -8x we can find two points on its graph. From y2 = -8(-2) = 16 we get y = ±4. As shown in FIGURE 7.1.8, the graph passes through (0, 0) as well as through the endpoints (-2, -4) and (-2, 4) of the focal chord.
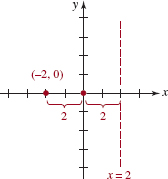
FIGURE 7.1.7 Directrix and focus in Example 2
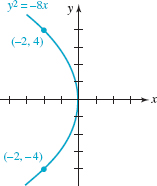
FIGURE 7.1.8 Graph of parabola in Example 2
Parabola with Vertex (h, k) Suppose that a parabola is shifted both horizontally and vertically so that its vertex is at the point (h, k) and its axis is the vertical line x = h. The standard form of the equation of the parabola is then
Similarly, if its axis is the horizontal line y = k, the standard form of the equation of the parabola with vertex (h, k) is
The parabolas defined by these equations are identical in shape to the parabolas defined by equations (1) and (2) because equations (3) and (4) represent rigid transformations (shifts up, down, left, and right) of the graphs of (1) and (2). For example, the parabola
(x + 1)2 = 8(y - 5)
has vertex (- 1, 5). Its graph is the graph of x2 = 8y shifted horizontally 1 unit to the left followed by an upward vertical shift of 5 units.
For each of the equations, (1) and (2) or (3) and (4), the distance from the vertex to the focus, as well as the distance from the vertex to the directrix, is | c |.
EXAMPLE 3 Find an Equation of a Parabola
Find the equation in standard form of the parabola with vertex (-3, - 1) and directrix y = 3.
Solution We begin by graphing the vertex at (-3, - 1) and the directrix y = 3. From FIGURE 7.1.9 we can see that the parabola must open downward, and so its standard form is (3). This fact, plus the observation that the vertex lies 4 units below the directrix, indicates that the appropriate solution of |c| = 4 is c = -4. Substituting h = -3, k = -1, and c = -4 into (3) gives
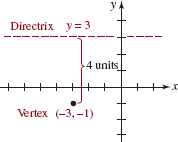
FIGURE 7.1.9 Vertex and directrix in Example 3
EXAMPLE 4 Find Everything
Find the vertex, focus, directrix, intercepts, and graph of the parabola
Solution In order to write the equation in one of the standard forms we complete the square in y:
Thus the standard form of equation (5) is (y - 2)2 = 8(x + 4). Comparing this equation with (4) we conclude that the vertex is (-4, 2) and that 4c = 8 or c = 2. Thus the parabola opens to the right. From c = 2 > 0, the focus is 2 units to the right of the vertex at (-4 + 2, 2) or (-2, 2). The directrix is the vertical line 2 units to the left of the vertex, x = -4 - 2 or x = -6. Knowing the parabola opens to the right from the point (-4, 2) also tells us that the graph has intercepts. To find the x-intercept we set y = 0 in (5) and find immediately that x = -
-
. The x-intercept is
. To find the y-intercepts we set x = 0 in (5) and find from the quadratic formula that 
or y ≈ 7.66 and y ≈ -3.66. The y-intercepts are 
. Putting all this information together we get the graph in FIGURE 7.1.10.
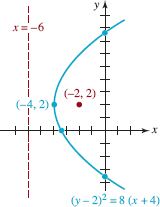
FIGURE 7.1.10Graph of equation in Example 4
Searchlights
200 inch reflecting telescope at Mt. Palomar
Applications of the Parabola The parabola has many interesting properties that make it suitable for certain applications. Reflecting surfaces are often designed to take advantage of a reflection property of parabolas. Such surfaces, called paraboloids, are three-dimensional and are formed by rotating a parabola about its axis. As illustrated in FIGURE 7.1.11(a), rays of light (or electronic signals) from a point source located at the focus of a parabolic reflecting surface will be reflected along lines parallel to the axis. This is the idea behind the design of searchlights, some flashlights, and on-location satellite dishes. Conversely, if the incoming rays of light are parallel to the axis of a parabola, they will be reflected off the surface along lines passing through the focus. See Figure 7.1.11(b). Beams of light from a distant object such as a galaxy are essentially parallel, and so when these beams enter a reflecting telescope they are reflected by the parabolic mirror to the focus, where a camera is usually placed to capture the image over time. A parabolic home satellite dish operates on the same principle as the reflecting telescope; the digital signal from a TV satellite is captured at the focus of the dish by a receiver.
TV satellite dish
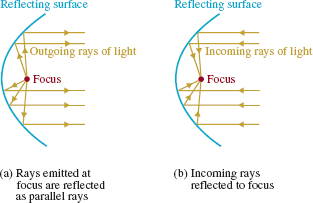
FIGURE 7.1.11 Parabolic reflecting surface
Parabolas are also important in the design of suspension bridges. It can be shown that if the weight of the bridge is distributed uniformly along its length, then a support cable in the shape of a parabola will bear the load evenly.
The trajectory of an obliquely launched projectile-say, a basketball thrown from the free-throw line-will travel in a parabolic arc.
Tuna, which prey on smaller fish, have been observed swimming in schools of 10-20 fish arrayed approximately in a parabolic shape. One possible explanation for this is that the smaller fish caught in the school of tuna will try to escape by “reflecting” off the parabola. See FIGURE 7.1.12. As a result, they are concentrated at the focus and become easy prey for the tuna.
The Brooklyn bridge is a suspension bridge
The ball travels in a parabolic arc
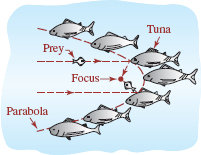
FIGURE 7.1.12 Tuna hunting in a parabolic arc
In Problems 1-24, find the vertex, focus, directrix, and axis of the given parabola. Graph the parabola.
1. y2 = 4x
2. y2 = x
3. y2 = -
x
4. y2 = -10x
5. x2 = -16y
6. x2 = 
y
7. x2 = 28y
8. x2 = -64y
9. (y - 1)2 = 16x
10. (y + 3)2 = -8(x + 2)
11. (x + 5)2 = -4(y + 1)
12.(x - 2)2 + y = 0
13. y2 + 12y - 4x + 16 = 0
14. x2 + 6x + y + 11 = 0
15. x2 + 5x -y + 6 = 0
16. x2 - 2x - 4y + 17 = 0
17. y2 - 8y + 2x + 10 = 0
18. y2 - 4y - 4x + 3 = 0
19. 4x2 = 2y
20. 3(y - 1)2 = 9x
21. -2x2 + 12x - 8y - 18 = 0
22. 4y2 + 16y - 6x - 2 = 0
23. 6y2 - 12y - 24x - 42 = 0
24. 3x2 + 30x - 8y + 75 = 0
In Problems 25-44, find an equation of the parabola that satisfies the given conditions.
25. Focus (0, 7), directrix y = -7
26. Focus (0, -5), directrix y = 5
27. Focus (-4, 0), directrix x = 4
28. Focus (
, 0), directrix x = -
29. Focus (
, 0), vertex (0, 0)
30. Focus (0, -10), vertex (0, 0)
31. Focus (2, 3), directrix y = -3
32. Focus (1, -7), directrix x = -5
33. Focus (-1, 4), directrix x = 5
34. Focus (-2, 0), directrix y =
35. Focus (1, 5), vertex (1, -3)
36. Focus (-2, 3), vertex (-2, 5)
37. Focus (8, -3), vertex (0, -3)
38. Focus (1, 2), vertex (7, 2)
39. Vertex (0, 0), directrix y = -
40. Vertex (0, 0), directrix x = 6
41. Vertex (5, 1), directrix y = 7
42. Vertex (- 1, 4), directrix x = 0
43. Vertex (0, 0), through (-2, 8), axis along the y-axis
44. Vertex (0, 0), through (1, ), axis along the x-axis
In Problems 45-48, find the x- and y-intercepts of the given parabola.
45. (y + 4)2 = 4(x + 1)
46. (x - 1)2 = -2(y - 1)
47. x2 + 2y - 18 = 0
48. y2 - 8y - x + 15 = 0
Miscellaneous Applications
49. Spotlight A large spotlight is designed so that a cross section through its axis is a parabola and the light source is at the focus. Find the position of the light source if the spotlight is 4 ft across at the opening and 2 ft deep.
50. Reflecting Telescope A reflecting telescope has a parabolic mirror that is 20 ft across at the top and 4 ft deep at the center. Where should the eyepiece be located?
51. Light Ray Suppose that a light ray emanating from the focus of the parabola y2 = 4x strikes the parabola at (1, -2). What is the equation of the reflected ray?
52. Suspension Bridge Suppose that two towers of a suspension bridge are 350 ft apart and the vertex of the parabolic cable is tangent to the road midway between the towers. If the cable is 1 ft above the road at a point 20 ft from the vertex, find the height of the towers above the road.
53. Another Suspension Bridge Two 75-ft towers of a suspension bridge with a parabolic cable are 250 ft apart. The vertex of the parabola is tangent to the road midway between the towers. Find the height of the cable above the roadway at a point 50 ft from one of the towers.
54. Drain Pipe Assume that the water gushing from the end of a horizontal pipe follows a parabolic arc with the vertex at the end of the pipe. The pipe is 20 m above the ground. At a point 2 m below the end of the pipe, the horizontal distance from the water to a vertical line through the end of the pipe is 4 m. See FIGURE 7.1.13. Where does the water strike the ground?
FIGURE 7.1.13 Pipe in Problem 54
55. A Bull's-Eye A dart thrower releases a dart 5 ft above the ground. The dart is thrown horizontally and follows a parabolic path. It hits the ground 10 √10 ft from the dart thrower. At a distance of 10 ft from the dart thrower, how high should a bull's-eye be placed in order for the dart to hit it?
56. Path of a Projectile The vertical position of a projectile is given by the equation y = -16t2 and the horizontal position by X = 40t for t ≥ 0. By eliminating t between the two equations, show that the path of the projectile is a parabolic arc. Graph the path of the projectile.
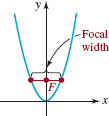
FIGURE 7.1.14 Focal width in Problem 57
57. Focal Width The focal width of a parabola is the length of the focal chord, that is, the line segment through the focus perpendicular to the axis, with endpoints on the parabola. See FIGURE 7.1.14.
a) Find the focal width of the parabola X2 = 8y.
b) Show that the focal width of the parabola X2 = 4cy and y2 = 4cx is 4 | c |.
58. Parabolic Orbit The orbit of a comet is a parabola with the Sun at the focus. When the comet is 50,000,000 km from the Sun, the line from the comet to the Sun is perpendicular to the axis of the parabola. Use the result of Problem 57(b) to write an equation of the comet's path. (A comet with a parabolic path will not return to the solar system.)
For Discussion
59. Reflecting Surfaces Suppose that two parabolic reflecting surfaces face one another (with foci on a common axis). Any sound emitted at one focus will be reflected off the parabolas and concentrated at the other focus. FIGURE 7.1.15 shows the paths of two typical sound waves. Using the definition of a parabola on page 320, show that all waves will travel the same distance. (Note: This result is important for the following reason. If the sound waves traveled paths of different lengths, then the waves would arrive at the second focus at different times. The result would be interference rather than clear sound.)
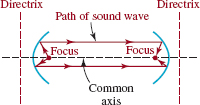
FIGURE 7.1.15 Parabolic reflecting surfaces in Problem 59
60. The point closest to the focus is the vertex. How would you go about proving this? Carry out your ideas.
61. For the comet in Problem 58, use the result of Problem 60 to determine the shortest distance between the Sun and the comet.
7.2 The Ellipse
Introduction The ellipse occurs frequently in astronomy. For example, the paths of the planets around the Sun are elliptical with the Sun located at one focus. Similarly, communication satellites, the Hubble space telescope, and the international space station revolve around the Earth in elliptical orbits with the Earth at one focus. In this section we define the ellipse and study some of its properties and applications.
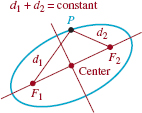
FIGURE 7.2.1 An ellipse
As shown in FIGURE 7.2.1, if P is a point on the ellipse and if d1 = d(F1, P) and d2 = d(F2, P) are the distances from the foci to P, then the preceding definition asserts that
where k > 0 is some constant.
On a practical level, equation (1) suggests a way of generating an ellipse. FIGURE 7.2.2 shows that if a string of length k is attached to a piece of paper by two tacks, then an ellipse can be traced out by inserting a pencil against the string and moving it in such a manner that the string remains taut.
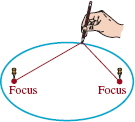
FIGURE 7.2.2 A way to draw an ellipse
Ellipse with Center (0, 0) We now derive an equation of the ellipse. For algebraic convenience, let us choose k = 2a > 0 and put the foci on the x-axis with coordinates F1(-c, 0) and F2(c, 0) as shown in FIGURE 7.2.3. It follows from (1) that
or
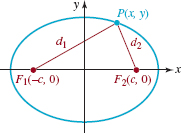
FIGURE 7.2.3 Ellipse with center (0, 0) and foci on the x-axis
We square both sides of the second equation in (2) and simplify,
Squaring a second time gives
or
Referring to FIGURE 7.2.3, we see that the points F1, F2, and P form a triangle. Because the sum of the lengths of any two sides of a triangle is greater than the remaining side, we must have 2a > 2c or a > c. Hence, a2 - c2 > 0.When we let b2 = a2 - c2, then (3) becomes b2x2 + a2y2 = a2b2. Dividing this last equation by a2b2 gives
Equation (4) is called the standard form of the equation of an ellipse centered at (0, 0) with foci (-c, 0) and (c, 0), where c is defined by b2 = a2 - c2 and a > b > 0. If the foci are placed on the y-axis, then a repetition of the above analysis leads to
Equation (5) is called the standard form of the equation of an ellipse centered at (0, 0) with foci (0, -c) and (0, c), where c is defined by b2 = a2 - c2 and a > b > 0.
FIGURE 7.2.4 A summary of all this information for equations (4) and (5) is given in FIGURE 7.2.4.
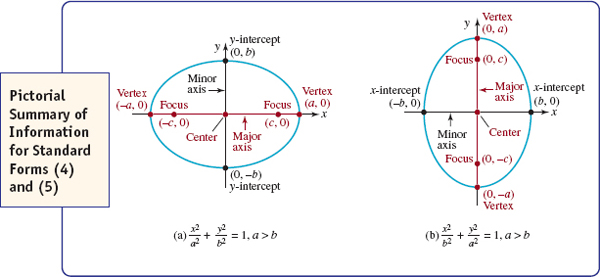
Summary of information for standard forms (4) and (5)
EXAMPLE 1 Vertices and Foci
Find the vertices and foci of the ellipse whose equation is 3x2 + y2 = 9. Graph.
Solution By dividing both sides of the equality by 9 the standard form of the equation is
We see that 9 > 3 and so we identify the equation with (5). From a2 = 9 and b2 = 3, we see that a = 3 and b = √3. The major axis is vertical with endpoints (0, -3) and (0, 3). The minor axis is horizontal with endpoints (-√3, 0) and (√3, 0). Of course, the vertices are also the y-intercepts and the endpoints of the minor axis are the x-intercepts. Now, to find the foci we use b2 = a2 - c2 or c2 = a2 - b2 to write 
. With a = 3, b = √3, we get 
. Hence, the foci are on the y-axis at (0, - √6) and (0, √6).The graph is given in FIGURE 7.2.5.
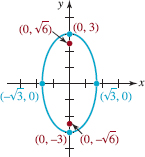
FIGURE 7.2.5 Ellipse in Example 1
EXAMPLE 2 Finding an Equation of an Ellipse
Find an equation of the ellipse with a focus (2, 0) and an x-intercept (5, 0).
Solution Since the given focus is on the x-axis, we can find an equation in standard form (4). Consequently, c = 2, a = 6, a2 = 25, and b2 = a2 - c2 or b2 = 52 - 22 = 21. The desired equation is
Ellipse with Center (h, k) When the center is at (h, k), the standard form for the equation of an ellipse is either
or
The ellipses defined by these equations are identical in shape to the ellipses defined by equations (4) and (5) since equations (6) and (7) represent rigid transformations of the graphs of (4) and (5). For example, the ellipse
has center (1, -3).Its graph is the graph of x2/9 + y2/16 = 1 shifted horizontally 1 unit to the right followed by a downward vertical shift of 3 units.
It is not a good idea to memorize formulas for the vertices and foci of an ellipse with center (h, k). Everything is the same as before, a, b, and c are positive and a > b, a > c. You can locate vertices, foci, and endpoints of the minor axis using the fact that a is the distance from the center to a vertex, b is the distance from the center to an endpoint on the minor axis, and c is the distance from the center to a focus. Also, we still have c2 = a2 - b2.
EXAMPLE 3 Ellipse Centered at (h, k)
Find the vertices and foci of the ellipse 4x2 + 16y2 - 8x - 96y + 84 = 0. Graph.
Solution To write the given equation in one of the standard forms (6) or (7) we must complete the square in x and in y. Recall, in order to complete the square we want the coefficients of the quadratic terms x2 and y2 to be 1. To do this we factor 4 from both x2 and x and factor 16 from both y2 and y:
Then from
we obtain
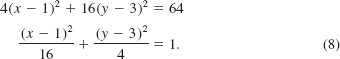
From (8) we see that the center of the ellipse is (1, 3). Since the last equation has the standard form (6) we identify a2 = 16 or a = 4 and b2 = 4 or b = 2. The major axis is horizontal and lies on the horizontal line y = 3 passing through (1, 3). This is the red horizontal dashed line segment in FIGURE 7.2.6. By measuring a = 4 units to the left and then to the right of the center along the line y = 3 we arrive at the vertices (-3, 3) and (5, 3). By measuring b = 2 units both down and up the vertical line x = 1 through the center we arrive at the endpoints of the minor axis (1, 1) and (1, 5). The minor axis is the black dashed vertical line segment in FIGURE 7.2.6. Because c2 = a2 - b2 = 16 - 4 = 12, c = 2√3. Finally, by measuring c = 2√3 units to the left and right of the center along y = 3 we obtain the foci (1 - 2√3, 3) and (1 + 2 √3, 3).
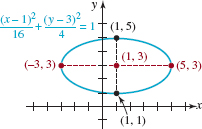
FIGURE 7.2.6 Ellipse in Example 3
EXAMPLE 4 Finding an Equation of an Ellipse
Find an equation of the ellipse with center (2, -1), vertical major axis of length 6, and minor axis of length 3.
Solution The length of the major axis is 2a = 6; hence a = 3. Similarly, the length of the minor axis is 2b = 3, so b = . By sketching the center and the axes, we see from FIGURE 7.2.7 that the vertices are (2, 2) and (2, -4) and the endpoints of the minor axis are (, -1) and (, -1). Because the major axis is vertical, the standard equation of this ellipse is
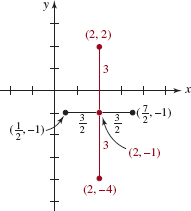
FIGURE 7.2.7 Graphical interpretation of data in Example 4
Eccentricity Associated with each conic section is a number e called its eccentricity. The eccentricity of an ellipse is defined to be
where 
. Since 
the eccentricity of an ellipse satisfies 0 < e < 1.
EXAMPLE 5 Example 3 Revisited
Determine the eccentricity of the ellipse in Example 3.
Solution In the solution of Example 3 we found that a = 4 and c = 2√3. Hence, the eccentricity of the ellipse is e = (2√3)/4 = √3/2 ≈ 0.87.
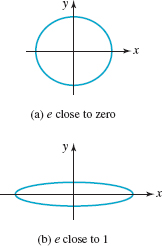
FIGURE 7.2.8 Effect of eccentricity on the shape of an ellipse
Eccentricity is an indicator of the shape of an ellipse. When e ≈ 0 that is, e is close to zero, the ellipse is nearly circular, and when e ≈ 1 the ellipse is flattened or elongated. To see this, observe that if e is close to 0, it follows from 
that 
and consequently a ≈ b. As you can see from the standard equations in (4) and (5), this means that the shape of the ellipse is close to circular. Also, because c is the distance from the center of the ellipse to a focus, the two foci are close together near the center. See Figure 7.2.8(a). On the other hand, if e ≈ 1 or 
then 
and so b ≈ 0 Also, c ≈ a means that the foci are far apart; each focus is close to a vertex. Thus, the ellipse is elongated as shown in Figure 7.2.8(b).
Applications of the Ellipse Ellipses have a reflection property analogous to the one discussed in Section 7.1 for the parabola. It can be shown that if a light or sound source is placed at one focus of an ellipse, then all rays or waves will be reflected off the ellipse to the other focus. See FIGURE 7.2.9. For example, if a pool table is constructed in the form of an ellipse with a pocket at one focus, then any shot originating at the other focus will never miss the pocket. Similarly, if a ceiling is elliptical with two foci on (or near) the floor, but considerably distant from each other, then anyone whispering at one focus will be heard at the other. Some famous “whispering galleries” are the Statuary Hall at the Capitol in Washington, DC, the Mormon Tabernacle in Salt Lake City, and St. Paul's Cathedral in London.
FIGURE 7.2.9 Reflection property of an ellipse
Using his law of universal gravitation, Isaac Newton was the first to prove Kepler's first law of planetary motion: The orbit of each planet about the Sun is an ellipse with the Sun at one focus.
EXAMPLE 6 Eccentricity of Earth's Orbit
The perihelion distance of the Earth (the least distance between the Earth and the Sun) is approximately 9.16 × 107 miles, and its aphelion distance (the greatest distance between the Earth and the Sun) is approximately 9.46 × 107 miles. What is the eccentricity of Earth's orbit?
Solution Let us assume that the orbit of the Earth is as shown in FIGURE 7.2.10. From the figure we see that
Solving this system of equations gives a = 9.31 × 107and c = 0.15 × 107. Thus the
Statuary Hall in Washington, DC
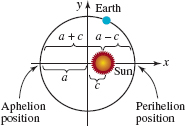
FIGURE 7.2.10 Graphical interpretation of data in Example 6
The orbits of seven of the eight planets have eccentricities less than 0.1 and, hence, the orbits are not far from circular. Mercury is the exception. The orbit of the well-known dwarf planet Pluto has the eccentricity 0.25. Many of the asteroids and comets have highly eccentric orbits. The orbit of the asteroid Hildago is one of the most eccentric, with e = 0.66. Another notable case is the orbit of Comet Halley. See Problem 43 in Exercises 7.2.
In Problems 1-20, find the center, foci, vertices, endpoints of the minor axis, and eccentricity of the given ellipse. Graph the ellipse.
1. 
2. 
3. 
4. 
5. 9x2 + 16y2 = 144
6. 2x2 + y2 = 4
7. 9x2 + 4y2 = 36
8. x2 + 4y2 =4
9. 
10. 
11. 
12. 
13. 4x2 + (y + )2 = 4
14. 36(x + 2)2 + (y - 4)2 = 72
15. 5(x - 1)2 + 3(y + 2)2 = 45
16. 6(x - 2)2 + 8y2 = 48
17. 25x2 + 9y2 - 100x + 18y - 116 = 0
18. 9x2 + 5y2 + 18x - 10y - 31 = 0
19. x2 + 3y2 + 18y + 18 = 0
20. 12x2 + 4y2 - 24x - 4y + 1 = 0
In Problems 21-40, find an equation of the ellipse that satisfies the given conditions.
21. Vertices (±5, 0), foci (±3, 0)
22. Vertices (±9, 0), foci (±2, 0)
23. Vertices (0, ±3), foci (0, ±1)
24. Vertices (0, ±7), foci (0, ±3)
25. Vertices (0, ±3), endpoints of minor axis (±1, 0)
26. Vertices (±4, 0), endpoints of minor axis (0, ±2)
27. Vertices (-3, -3), (5, -3), endpoints of minor axis (1, -1), (1, -5)
28. Vertices (1, -6), (1, 2), endpoints of minor axis (-2, -2), (4, -2)
29. One focus (0, -2), center at origin, b = 3
30. One focus (1, 0), center at origin, a = 3
31. Foci (± √2, 0), length of minor axis 6
32. Foci (0, ±√5), length of major axis 16
33. Foci (0, ±3), passing through (-1, 2√2)
34. Vertices (±5, 0), passing through (√5, 4)
35. Vertices (±4, 1), passing through (2√3, 2)
36. Center (1, -1), one focus (1, 1), a = 5
37. Center (1, 3), one focus (1, 0), one vertex (1, -1)
38. Center (5, -7), length of vertical major axis 8, length of minor axis 6
39. Endpoints of minor axis (0, 5), (0, -1), one focus (6, 2)
40. Endpoints of major axis (2, 4), (13, 4), one focus (4, 4)
41. The orbit of the planet Mercury is an ellipse with the Sun at one focus. The length of the major axis of this orbit is 72 million miles and the length of the minor axis is 70.4 million miles. What is the least distance (perihelion) between Mercury and the Sun? What is the greatest distance (aphelion)?
42. What is the eccentricity of the orbit of Mercury in Problem 41?
43. The orbit of Comet Halley is an ellipse whose major axis is 3.34 X 109 miles long, and whose minor axis is 8.5 X 108 miles long. What is the eccentricity of the comet's orbit?
44. A satellite orbits the Earth in an elliptical path with the center of the Earth at one focus. It has a minimum altitude of 200 mi and a maximum altitude of 1000 mi above the surface of the Earth. If the radius of the Earth is 4000 mi, what is an equation of the satellite's orbit?
Miscellaneous Applications
Elliptical and circular gears in Problem 46
45. Archway A semielliptical archway has a vertical major axis. The base of the arch is 10 ft across and the highest part of the arch is 15 ft. Find the height of the arch above the point on the base of the arch 3 ft from the center.
46. Gear Design An elliptical gear rotates about its center and is always kept in mesh with a circular gear that is free to move horizontally. See FIGURE 7.2.11. If the origin of the xy-coordinate system is placed at the center of the ellipse, then the equation of the ellipse in its present position is 3x2 + 9y2 = 24. The diameter of the circular gear equals the length of the minor axis of the elliptical gear. Given that the units are centimeters, how far does the center of the circular gear move horizontally during the rotation from one vertex of the elliptical gear to the next?
47. Carpentry A carpenter wishes to cut an elliptical top for a coffee table from a rectangular piece of wood that is 4-ft by 3-ft utilizing the entire length and width available. If the ellipse is to be drawn using the string-and-tack method illustrated in FIGURE 7.2.2, how long should the piece of string be and where should the tacks be placed?
48. Park Design The Ellipse is a park in Washington, DC. It is bounded by an elliptical path with a major axis of length 458 m and a minor axis of length 390 m. Find the distance between the foci of this ellipse.
49. Whispering Gallery Suppose that a room is constructed on a flat elliptical base by rotating a semiellipse 180° about its major axis. Then, by the reflection property of the ellipse, anything whispered at one focus will be distinctly heard at the other focus. If the height of the room is 16 ft and the length is 40 ft, find the location of the whispering and listening posts.
50. Focal Width The focal width of the ellipse is the length of a focal chord, that is, a line segment perpendicular to the major axis, through a focus with endpoints on the ellipse. See FIGURE 7.2.12.
(a) Find the focal width of the ellipse x2/9 + y2/4 = 1.
(b) Show that, in general, the focal width of the ellipse x2/a2 + y2/b2 = 1 is 2b2/a.
51. Find an equation of the ellipse with foci (0, 2) and (8, 6) and fixed distance sum 2a = 12. [Hint: Here the major axis is neither horizontal nor vertical; thus none of the standard forms from this section apply. Use the definition of the ellipse.]
52. Proceed as in Problem 51, and find an equation of the ellipse with foci (-1, -3) and (-5, 7) and fixed distance sum 2a = 20.
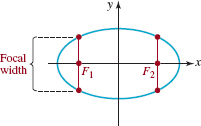
Focal width in Problem 50
For Discussion
53. The graph of the ellipse x2/4 + (y - 1)2/9 = 1 is shifted 4 units to the right. What are the center, foci, vertices, and endpoints of the minor axis for the shifted graph?
54. The graph of the ellipse (x - 1)2/9 + (y - 4)2 = 1 is shifted 5 units to the left and 3 units up. What are the center, foci, vertices, and endpoints of the minor axis for the shifted graph?
55. In engineering the eccentricity of an ellipse is often expressed only in terms of a and b. Show that 
.
7.3 The Hyperbola
Introduction The definition of a hyperbola is basically the same as the definition of the ellipse with only one exception: the word sum is replaced by the word difference.
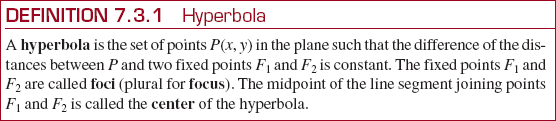
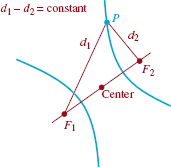
FIGURE 7.3.1 A hyperbola
As shown in FIGURE 7.3.1, a hyperbola consists of two branches. If P is a point on the hyperbola, then
where d1 = d(F1,P) and d2 = d(F2, p).
Hyperbola with Center (0, 0) Proceeding as for the ellipse, we place the foci on the x-axis at F1(-c, 0) and F2(c, 0) as shown in FIGURE 7.3.2 and choose the constant k to be 2a for algebraic convenience. It follows from (1) that
As drawn in FIGURE 7.3.2, P is on the right branch of the hyperbola and so d1 - d2 = 2a > 0. If P is on the left branch, then the difference is -2a. Writing (2) as
we square, simplify, and square again:
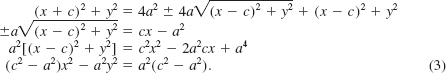
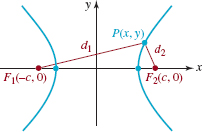
FIGURE 7.3.2 Hyperbola with center (0, 0) and foci on the x-axis
From FIGURE 7.3.2, we see that the triangle inequality gives
or
Using d1 - d2 = ±2a the last two inequalities imply that 2a < 2c or a > c. Since c < a < 0, c2 - a2 is a positive constant. If we let b2 = c2 - a2, (3) becomes b2x2 - a2y2 = a2b2 or, after dividing by a2b2,
Equation (4) is called the standard form of the equation of a hyperbola centered at (0, 0)with foci (-c, 0) and (c, 0), where cis defined by b2 = c2 - a2
When the foci lie on the y-axis, a repetition of the foregoing algebra leads to
Note of Caution
Equation (5) is the standard form of the equation of a hyperbola centered at (0, 0) with foci (0, - c) and (0, c). Here again, c > a and b2 = c2 - a2.
For the hyperbola (unlike the ellipse) bear in mind that in (4) and (5) there is no relationship between the relative sizes of a and b; rather, a2 is always the denominator of the positive term and the intercepts always have ±a as a coordinate.
Transverse and Conjugate Axes The line segment with endpoints on the hyperbola and lying on the line through the foci is called the transverse axis; its endpoints are called the vertices of the hyperbola. For the hyperbola described by equation (4), the transverse axis lies on the x-axis. Therefore, the coordinates of the vertices are the x-intercepts. Setting y = 0 gives x2/a2 = l, or x = 6a. Thus, as shown in Figure 7.3.3(a) the vertices are (-a, 0) and (a, 0);the length of the transverse axis is 2a. Notice that by setting y = 0 in (4), we get - y2/b2 = 1 or y2 = - b2, which has no real solutions. Hence the graph of any equation in that form has no y-intercepts. Nonetheless, the numbers ±b are important.
The line segment through the center of the hyperbola perpendicular to the transverse axis and with endpoints (0, - b) and (0, b) is called the conjugate axis. Similarly, the graph of an equation in standard form (5) has no x-intercepts. The conjugate axis for (5) is the line segment with endpoints (-b, 0) and (b, 0).
This information for equations (4) and (5) is summarized in FIGURE 7.3.3.
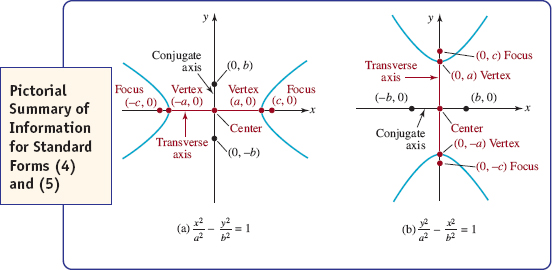
FIGURE 7.3.3 Summary of information for standard forms (4) and (5)
Asymptotes Every hyperbola possesses a pair of slant asymptotes that pass through its center. These asymptotes are indicative of end behavior, and as such are an invaluable aid in sketching the graph of a hyperbola. Solving (4) for y in terms of x gives
As x → -∞ or as x → ∞, a2/x2 → 0, and thus 
. Therefore, for large values of |x|, points on the graph of the hyperbola are close to the points on the lines
By a similar analysis we find that the slant asymptotes for (5) are
Each pair of asymptotes intersect at the origin, which is the center of the hyperbola. Note, too, in Figure 7.3.4(a) that the asymptotes are simply the extended diagonals of a rectangle of width 2a (the length of the transverse axis) and height 2b (the length of the conjugate axis); in Figure 7.3.4(b) the asymptotes are the extended diagonals of a rectangle of width 2b and height 2a. This rectangle is referred to as the auxiliary rectangle.
We recommend that you do not memorize the equations in (6) and (7). There is an easy method for obtaining the asymptotes of a hyperbola. For example, since 
is equivalent to
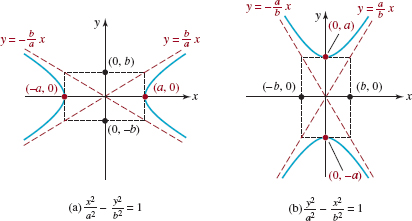
FIGURE 7.3.4 Hyperbolas (4) and (5) with slant asymptotes (red) as the extended diagonals of the auxiliary rectangle (black)
the asymptotes of the hyperbola given in (4) are obtained from a single equation
Note that (8) factors as the difference of two squares:
This is a mnemonic, or memory device. It has no geometric significance.
Setting each factor equal to zero and solving for y gives an equation of an asymptote. You do not even have to memorize (8) because it is simply the left-hand side of the standard form of the equation of a hyperbola given in (4). In like manner, to obtain the asymptotes for (5) just replace 1 by 0 in the standard form, factor y2/a2 - x2/b2 = 0, and solve for y.
EXAMPLE 1 Hyperbola Centered at (0, 0)
Find the vertices, foci, and asymptotes of the hyperbola 9x2 - 25y2 = 225. Graph. Solution We first put the equation into standard form by dividing the left-hand side by 225:
From this equation we see that a2 = 25 and b 2 = 9, and so a = 5 and b = 3. Therefore the vertices are (-5, 0) and (5, 0). Since b2 = c2 - a2 implies c2 = a2 + b2, we have c2 = 34, and so the foci are 
and 
To find the slant asymptotes we use the standard form (9) with 1 replaced by 0:
Setting each factor equal to zero and solving for y gives the asymptotes y =±3 x/5. We plot the vertices and graph the two lines through the origin. Both branches of the hyperbola must become arbitrarily close to the asymptotes as x → ±∞. See FIGURE 7.3.5.
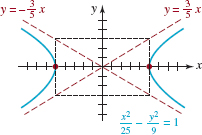
FIGURE 7.3.5 Hyperbola in Example 1
EXAMPLE 2 Finding an Equation of a Hyperbola
Find an equation of the hyperbola with vertices (0, -4), (0, 4) and asymptotes y = -x y =x.
Solution The center of the hyperbola is (0, 0). This is revealed by the fact that the asymptotes intersect at the origin. Moreover, the vertices are on the y-axis and are 4 units on either side of the origin. Thus the equation we seek is of form (5). From (7) or Figure 7.3.4(b), the asymptotes must be of the form y = ±
x so that a/b = 1/2. From the given vertices we identify a = 4, and so
The equation of the hyperbola is then
Hyperbola with Center (h, k) When the center of the hyperbola is (h, k) the standard form analogs of equations (4) and (5) are, in turn,
and
As in (4) and (5) the numbers a2, b2, and c2 are related by b2 = c2 - a2
You can locate vertices and foci using the fact that a is the distance from the center to a vertex, and c is the distance from the center to a focus. The slant asymptotes for (10) can be obtained by factoring
Similarly, the asymptotes for (11) can be obtained from factoring
setting each factor equal to zero and solving for y in terms of x. As a check on your work, remember that (h, k) must be a point that lies on each asymptote.
EXAMPLE 3 Hyperbola Centered at (h, k)
Find the center, vertices, foci, and asymptotes of the hyperbola 4x2 - y2 - 8x - 4y - 4 = 0. Graph.
Solution Before completing the square in x and y, we factor 4 from the two x-terms and factor -1 from the two y-terms so that the leading coefficient in each expression is 1. Then we have
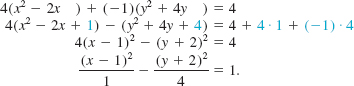
We see now that the center is (1, -2). Since the term in the standard form involving x has the positive coefficient, the transverse axis is horizontal along the line y = -2, and we identify a = 1 and b = 2. The vertices are 1 unit to the left and to the right of the center at (0, -2) and (2, -2), respectively. From b2 = c2 - a2, we have
and so c = √5. Hence the foci are √5 units to the left and the right of the center (1, -2) at (1 - √5, -2) and (1 + √5, -2).
To find the asymptotes, we solve
for y. From y + 2 = ±2(x - 1) we find that the asymptotes are y = -2x and y = 2x - 4. Observe that by substituting x = 1, both equations give y = -2, which means that both lines pass through the center. We then locate the center, plot the vertices, and graph the asymptotes. As shown in FIGURE 7.3.6, the graph of the hyperbola passes through the vertices and becomes closer and closer to the asymptotes as x→±∞;
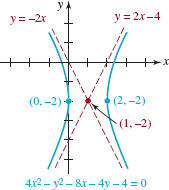
FIGURE 7.3.6 Hyperbola in Example 3
EXAMPLE 4 Finding an Equation of a Hyperbola
Find an equation of the hyperbola with center (2, -3), passing through the point (4, 1), and having one vertex (2, 0).
Solution Since the distance from the center to one vertex is a, we have a = 3. From the location of the center and the vertex, it follows that the transverse axis is vertical and lies along the line x = 2. Therefore, the equation of the hyperbola must be of form (11):
where b2 is yet to be determined. Since the point (4, 1) is on the graph on the hyperbola, its coordinates must satisfy equation (12). From
we find b2 = 
. We conclude that the desired equation is
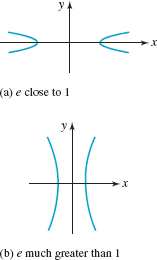
FIGURE 7.3.7 Effect of eccentricity on the shape of a hyperbola
Eccentricity Like the ellipse, the equation that defines the eccentricity of a hyperbola is e = c/a. Except in this case the number c is given by 
. Since 
, the eccentricity of an ellipse satisfies e > 1. As with the ellipse, the magnitude of the eccentricity of a hyperbola is an indicator of its shape. FIGURE 7.3.7 shows examples of two extreme cases: e ≈ 1 and e much bigger than 1.
EXAMPLE 5 Eccentricity of a Hyperbola
Find the eccentricity of the hyperbola 
Solution Identifying a2 = 2 and b2 = 36, we get c2 = 2 + 36 = 38. Thus the eccentricity of the given hyperbola is
We conclude that the hyperbola is one whose branches open widely as in Figure 7.3.7(b).
Applications of the Hyperbola The hyperbola has several important applications involving sounding techniques. In particular, several navigational systems utilize hyperbolas as follows. Two fixed radio transmitters at a known distance from each other transmit synchronized signals. The difference in reception times by a navigator determines the difference 2a of the distances from the navigator to the two transmitters. This information locates the navigator somewhere on the hyperbola with foci at the transmitters and fixed difference in distances from the foci equal to 2a. By using two sets of signals obtained from a single master station paired with each of two second stations, the long-range navigation system LORAN locates a ship or plane at the intersection of two hyperbolas. See FIGURE 7.3.8.
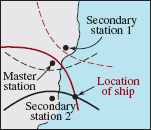
FIGURE 7.3.8 The idea behind LORAN
The next example illustrates the use of a hyperbola in another situation involving sounding techniques.
EXAMPLE 6 Locating a Big Blast
The sound of a dynamite blast is heard at different times by two observers at points A and B. Knowing that the speed of sound is approximately 1100 ft/s or 335 m/s, it is determined that the blast occurred 1000 meters closer to point A than to point B. If A and B are 2600 meters apart, show that the location of the blast lies on a branch of a hyperbola. Find an equation of the hyperbola.
Solution In FIGURE 7.3.9, we have placed the points A and B on the x-axis at (1300, 0) and (-1300, 0), respectively. If P(x, y) denotes the location of the blast, then
From the definition of the hyperbola on page 333 and the derivation following it, we see that this is the equation for the right branch of a hyperbola with fixed distance difference 2a = 100 and c = 1300. Thus the equation has the form
or after solving for x,
With a = 500 and c = 1300, b2 = (1300)2 - (500)2 = (1200)2. Substituting in the foregoing equation gives
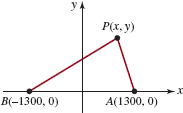
FIGURE 7.3.9 Graph in Example 6
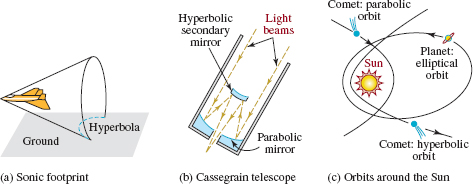
FIGURE 7.3.10 Applications of hyperbolas
To find the exact location of the blast in Example 6 we would need another observer hearing the blast at a third point C. Knowing the time between when this observer hears the blast and when the observer at A hears the blast, we find a second hyperbola. The actual point of detonation is a point of intersection of the two hyperbolas.
There are many other applications of the hyperbola. As shown in Figure 7.3.10(a), a plane flying at a supersonic speed parallel to level ground leaves a hyperbolic sonic “footprint” on the ground. Like the parabola and ellipse, a hyperbola also possesses a reflecting property. The Cassegrain reflecting telescope shown in Figure 7.3.10(b) utilizes a convex hyperbolic secondary mirror to reflect a ray of light back through a hole to an eyepiece (or camera) behind the parabolic primary mirror. This telescope construction makes use of the fact that a beam of light directed along a line through one focus of a hyperbolic mirror will be reflected on a line through the other focus.
Orbits of objects in the universe can be parabolic, elliptic, or hyperbolic. When an object passes close to the Sun (or a planet), it is not necessarily captured by the gravitational field of the larger body. Under certain conditions, the object picks up a fractional amount of orbital energy of this much larger body and the resulting “slingshot-effect” orbit of the object as it passes the Sun is hyperbolic. See Figure 7.3.10(c).
In Problems 1-20, find the center, foci, vertices, asymptotes, and eccentricity of the given hyperbola. Graph the hyperbola.
1. 
2. 
3. 
4. 
5. 4x2 - 16y2 = 64
6. 5x2 - 5y2 = 25
7. y2 - 5x2 = 20
8. 9x2 - 16y2 + 144 = 0
9. 
10. 
11. 
12. 
13. 25(x - 3)2 - 5(y - 1)2 = 125
14. 10(x + 1)2 - 2(y - )2 = 100
15. 8(x + 4)2 - 5(y - 7)2 + 40 = 0
16. 9(x - 1)2 - 81(y - 2)2 = 9
17. 5x2 - 6y2 - 20x + 12y - 16 = 0
18. 16x2 - 25y2 - 256x - 150y + 399 = 0
19. 4x2 - y2 - 8x + 6y - 4 = 0
20. 2y2 - 9x2 - 18x + 20y + 5 = 0
In Problems 21-44, find an equation of the hyperbola that satisfies the given conditions.
21. Foci (±5, 0), a = 3
22. Foci (±10, 2), b = 2
23. Foci (0, ±4), one vertex (0, -2)
24. Foci (0, ±3), one vertex (0, - )
25. Foci (±4, 0), length of transverse axis 6
26. Foci (0, ±7), length of transverse axis 10
27. Center (0, 0), one vertex (0, ), one focus (0, -3)
28. Center (0, 0), one vertex (7, 0), one focus (9, 0)
29. Center (0, 0), one vertex (-2, 0), one focus (-3, 0)
30. Center (0, 0), one vertex (1, 0), one focus (5, 0)
31. Vertices (0, ±8), asymptotes y = ±2x
32. Foci (0, ±3), asymptotes y = ±x
33. Vertices (±2, 0), asymptotes y = ±x
34. Foci (±5, 0), asymptotes y = ±
x
35. Center (1, -3), one focus (1, -6), one vertex (1, -5)
36. Center (2, 3), one focus (0, 3), one vertex (3, 3)
37. Foci (-4, 2), (2, 2), one vertex (-3, 2)
38. Vertices (2, 5), (2, -1), one focus (2, 7)
39. Vertices (±2, 0), passing through (2 √3, 4)
40. Vertices (0, ±3), passing through (
, 5)
41. Center (-1, 3), one vertex (-1, 4), passing through (-5, 3 + √5)
42. Center (3, -5), one vertex (3, -2), passing through (1, -1)
43. Center (2, 4), one vertex (2, 5), one asymptote 2y - x - 6 = 0
44. Eccentricity √10, endpoints of conjugate axis (-5, 4), (-5, 10)
45. Three points are located at A (-10, 16), B(-2, 0), and C(2, 0), where the units are kilometers. An artillery gun is known to lie on the line segment between A and C, and using sounding techniques it is determined that the gun is 2 km closer to B than to C. Find the point where the gun is located.
46. It can be shown that a ray of light emanating from one focus of a hyperbola will be reflected back along the line from the opposite focus. See FIGURE 7.3.11. A light ray from the left focus of the hyperbola x2/16 -y2/20 = 1 strikes the hyperbola at (- 6, - 5). Find an equation of the reflected ray.
47. Find an equation of the hyperbola with foci (0, -2) and (8, 4) and fixed distance difference 2a = 8. [Hint: See Problem 51 in Exercises 7.2.]
48. Focal Width The focal width of a hyperbola is the length of a focal chord, that is, a line segment, perpendicular to the line containing the transverse axis and through a focus, with endpoints on the hyperbola. See FIGURE 7.3.12.
(a)Find the focal width of the hyperbola x2/4 - y2/9 = 1.
(b)Show that, in general, the focal width of the hyperbola x2/a2 - y2/b2 = 1 is 2b2/a.
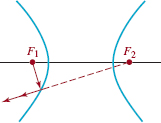
FIGURE 7.3.11 Reflecting property in Problem 46
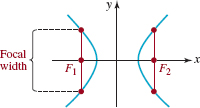
FIGURE 7.3.12 Focal width in Problem 48
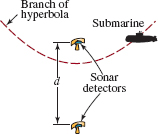
FIGURE 7.3.13 Sonic detectors in Problem 49
For Discussion
49. Sub Hunting Two sonar detectors are located at a distance d from one another. Suppose that a sound (such as a sneeze aboard a submarine) is heard at the two detectors with a time delay h between them. See FIGURE 7.3.13. Assume that sound travels in straight lines to the two detectors with speed v.
(a)Explain why h cannot be larger than d/v.
(b)Explain why, for given values of d, v, and h, the source of the sound can be determined to lie on one branch of a hyperbola. [Hint: Where do you suppose that the foci might be?]
(c)Find an equation for the hyperbola in part (b), assuming that the detectors are at the points (0, d/2) and (0, -d/2). Express the answer in the standard form y2/a2 - x2/b2 = 1.
50. The hyperbolas
are said to be conjugates of each other.
(a)Find the equation of the hyperbola that is conjugate to
(b)Discuss how the graphs of conjugate hyperbolas are related.
51. A rectangular hyperbola is one for which the asymptotes are perpendicular.
(a)Show that y2 - x2 + 5y + 3x = 1 is a rectangular hyperbola.
(b)Which of the hyperbolas given in Problems 1-20 are rectangular?
A. True/False__________________________________________________
In Problems 1-20, answer true or false.
1. The axis of the parabola x2 = -4y is vertical.____
2. The foci of an ellipse lie on its graph.____
3. The eccentricity of a parabola is e = 0.____
4. The minor axis of an ellipse bisects the major axis.____
5. The point (-2, 5) is on the ellipse x2/8 + y2/50 = 1.____
6. The graphs of y = x2 and y2 - x2 = 1 have at most two points in common.____
7. The eccentricity of the hyperbola x2 - y2 = 1 is √2.____
8. For an ellipse, the length of the major axis is always greater than the length of the minor axis.____
9. The vertex and focus are both on the axis of symmetry of a parabola.____
10. The asymptotes for (x - h)2/a2 - (y - k)2/b2 = 1 must pass through (h, k).____
11. An ellipse with eccentricity e = 0.01 is nearly circular.____
12. The transverse axis of the hyperbola x2/9 - y2/49 = lis vertical.____
13. The two hyperbolas x2 - y2/25 = 1 and y2/25 - x2 = 1 have the same pair of slant asymptotes.____
14. The foci of the ellipse 3x2 + 3.1y2 = 9.3 lie on the y-axis.____
15. If P is a point on a parabola, then the perpendicular distance between P and the directrix equals the distance between P and the vertex.____
16. If y = 3x + 8 is an asymptote of a hyperbola, then the slope of the other asymptote is m = -3.____
17. The asymptotes of the hyperbola x2/a2 - y2/a2 = 1 are perpendicular.____
18. The graph of a hyperbola cannot intersect the graphs of its asymptotes.____
19. The hyperbola (x - 1)2 - (y + 1)2 = 1 has no y-intercept(s)._____
20. The eccentricity of the ellipse x2/52 + y2/32 = 1 is e = 
._____
B. Fill in the Blanks
In Problems 1-16, fill in the blanks.
1. An equation in the standard form y2 = 4cx of a parabola with focus (5, 0) is.___________.
2. An equation in the standard form x2 = 4cy of a parabola through (2, 6) is.___________.
3. A rectangular equation of a parabola with focus (1, -3) and directrix y = -7 is.___________.
4. The directrix and vertex of a parabola are x = -3 and (-1, -2), respectively. The focus of the parabola is___________.
5. The focus and directrix of a parabola are (0, ) and y = -, respectively. The vertex of the parabola is___________.
6. The vertex and focus of the parabola 8(x + 4)2 = y - 2 are___________.
7. The eccentricity of a parabola is e =___________.
8. The center and vertices of the ellipse 
= 1 are___________.
9. The center and vertices of the hyperbola y2 - (x - 1) = 1 are___________.
10. The asymptotes of the hyperbola y2 - (x - 1)2 = 1 are___________.
11. The y-intercepts of the hyperbola y2 - (x - 1)2 = 1 are___________.
12. The eccentricity of the hyperbola x2 - y2 = 1 is___________.
13. If the graph of an ellipse is very elongated, then its eccentricity e is close to___________. (Fill in with 0 or 1.)
14. The line segment with endpoints on a hyperbola and lying on the line through its foci is called___________.
15. The graph of the equation x2 + 4x + ay2 = 9 is an ellipse provided a ___________. (Fill in with > 0, < 0, or = 0.)
16. The center of a hyperbola with asymptotes y = -
+ and y = x + 
is___________.
C. Review Exercises_____________________________________________
In Problems 1-4, find the vertex, focus, directrix, and axis of the given parabola. Graph the parabola.
1. (y - 3)2 = -8x
2. 8(x + 4)2 = y - 2
3. x2 - 2x + 4y + 1 = 0
4. y2 + 10y + 8x + 41 = 0
In Problems 5-8, find an equation of the parabola that satisfies the given conditions.
5. Focus (1, -3), directrix y = - 7
6. Focus (3, -1), vertex (0, -1)
7. Vertex (1, 2), vertical axis, passing through (4, 5)
8. Vertex (-1, -4), directrix x = 2
In Problems 9-12, find the center, vertices, and foci of the given ellipse. Graph the ellipse.
9. 
10. 
11. 4x2 + y2 + 8x - 6y + 9 = 0
12. 5x2 + 9y2 - 20x + 54y + 56 = 0
In Problems 13-16, find an equation of the ellipse that satisfies the given conditions.
13. Endpoints of minor axis (0, ±4), foci (±5, 0)
14. Foci (2, -1 ±√2), one vertex (2, -1 + √6)
15. Vertices (±2, -2), passing through (1, -2 + √3)
16. Center (2, 4), one focus (2, 1), one vertex (2, 0)
In Problems 17-20, find the center, vertices, foci, and asymptotes of the given hyperbola. Graph the hyperbola.
17. (x - 1)(x + 1) = y2
18. 
19. 9x2 - y2 - 54x - 2y + 71 = 0
20. 16y2 - 9x2 - 64y - 80 = 0
In Problem 21-26, find an equation of the hyperbola that satisfies the given conditions.
21. Center (0, 0), one vertex (6, 0), one focus (8, 0)
22. Foci (2, ±3), one vertex (2, -)
23. Foci (±2√5, 0), asymptotes y = ±2x
24. Vertices (-3, 2) and (- 3, 4), one focus (-3, 3 + √2)
25. Vertices (1, 0) and (1, 6), one asymptote y = x + 2
26. Center (2, 2), distance to a vertex 3, distance to a focus 4, transverse axis parallel to the y-axis.
27. Find an equation of the ellipse when the center of 4x2 + y2 = 4 is translated to the point (-5, 2).
28. Carefully describe the graphs of the given functions.
29. Distance from a Satellite A satellite orbits the planet Neptune in an elliptical orbit with the center of the planet at one focus. If the length of the major axis of the orbit is 2 × 109m and the length of the minor axis is 6 × 108 m, find the maximum distance between the satellite and the center of the planet.
30. Mirror, Mirror… A parabolic mirror has a depth of 7 cm at its center and the distance across the top of the mirror is 20 cm. Find the distance from the vertex to the focus.
Parabolic mirror