Chapter 5
Sliding-Mode Control for Fractional-Order Nonlinear Systems Based on Disturbance Observer
5.1 Problem Statement
According to the Caputo definition of the fractional derivative (2.17), a class of fractional-order nonlinear systems subjected to external disturbances and control inputs is considered, which can be described as follows:
where ![]() is the fractional order with
is the fractional order with ![]() ,
, ![]() is the state vector of the fractional-order system (5.1),
is the state vector of the fractional-order system (5.1), ![]() is the known function vector,
is the known function vector, ![]() is the control input,
is the control input, ![]() is the external disturbance vector, and
is the external disturbance vector, and ![]() is the system output vector.
is the system output vector.
In this chapter, we design a nonlinear sliding-mode tracking control scheme to track the desired output of the fractional-order nonlinear system (5.1) based on a designed fractional-order SMDO. Under the proposed control scheme, the given desired trajectory ![]() can be followed by
can be followed by ![]() under the effect of the unknown external disturbances. The proposed control scheme will be rigorously shown to guarantee all the signals convergent in the closed-loop system; meanwhile tracking errors converge to zeros.
under the effect of the unknown external disturbances. The proposed control scheme will be rigorously shown to guarantee all the signals convergent in the closed-loop system; meanwhile tracking errors converge to zeros.
To proceed with the design of the sliding-mode control for the fractional-order nonlinear system (5.1) with external disturbances, the following assumptions are required.
5.2 Adaptive Control Design Based on Fractional-Order Sliding-Mode Disturbance Observer
In this chapter, a sliding-mode control scheme is developed based on the fractional-order SMDO for the fractional-order nonlinear system (5.1) in the presence of unknown bounded external disturbances.
5.2.1 Design of Fractional-Order Sliding-Mode Disturbance Observer
According to the fractional-order nonlinear system (5.1), we have
where ![]() is the
is the ![]() th element of
th element of ![]() ,
, ![]() is the
is the ![]() th element of
th element of ![]() ,
, ![]() is the
is the ![]() th element of
th element of ![]() ,
, ![]() is the
is the ![]() th element of
th element of ![]() , and
, and ![]() .
.
Since the disturbance ![]() is unknown in Equation (5.1), the designed tracking controller cannot contain the external disturbance
is unknown in Equation (5.1), the designed tracking controller cannot contain the external disturbance ![]() . To overcome this problem, a fractional-order SMDO is designed to estimate the disturbance.
. To overcome this problem, a fractional-order SMDO is designed to estimate the disturbance.
For the system (5.2), the fractional-order SMDO is designed as follows:
where ![]() is a designed positive constant,
is a designed positive constant, ![]() is the auxiliary sliding-mode surface,
is the auxiliary sliding-mode surface, ![]() and
and ![]() are the state variables of the fractional-order SMDO (5.3),
are the state variables of the fractional-order SMDO (5.3), ![]() is the output of the disturbance observer,
is the output of the disturbance observer, ![]() is the estimate of
is the estimate of ![]() , and
, and ![]() is the sign function.
is the sign function.
According to Equations (5.2) and (5.3), we have
Consider the following Lyapunov candidate function:
where ![]() is a design constant,
is a design constant, ![]() is the estimation error, and
is the estimation error, and ![]() .
.
From Lemma 2.1 and Equation (5.5), we have
In particular, if the Caputo derivative of a constant function is 0, we have
Substituting Equation (5.7) into Equation (5.6), we obtain
Invoking Equation (5.4), Equation (5.8) can be rewritten as

On the basis of Assumption 5.2, we have
From Equation (5.10), we obtain
Furthermore, the adaptive law for ![]() is chosen as
is chosen as
According to Equations (5.3) (5.11), and (5.12), we obtain
From Equation (5.13), we can see that the fractional derivative of the Lyapunov function ![]() is negative definite. Thus, it can be concluded from Lemma 2.2 that the origin of the sliding surface
is negative definite. Thus, it can be concluded from Lemma 2.2 that the origin of the sliding surface ![]() is asymptotically stable. When sliding surface
is asymptotically stable. When sliding surface ![]() is stable, we obtain that
is stable, we obtain that ![]() and
and ![]() . According to Equations (5.3) and (5.4),
. According to Equations (5.3) and (5.4), ![]() can approximate the disturbance
can approximate the disturbance ![]() .
.
This design procedure of fractional-order SMDO can be summarized in the following theorem.
On the basis of these analyses, Theorem 5.1 can be easily proven.
5.2.2 Controller Design and Stability Analysis
In this subsection, the fractional-order SMDO-based sliding-mode tracking control scheme will be studied for the fractional-order nonlinear system (5.1). To design the sliding-mode tracking control scheme for the studied fractional-order nonlinear system (5.1), we define an auxiliary variable as
where ![]() is the
is the ![]() th element of
th element of ![]() .
.
In accordance with Equations (5.2) and (5.3), the Caputo fractional derivative of ![]() is
is
Using the designed SMDO, the fractional-order SMDO-based sliding-mode tracking control scheme is proposed as
where ![]() is a design positive constant.
is a design positive constant.
This development of the sliding-mode control using the fractional-order SMDO for the fractional-order nonlinear system (5.1) is summarized as follows.
5.3 Simulation Examples
In this section, simulation results of two fractional-order nonlinear systems [167] are presented to illustrate the effectiveness of the proposed fractional-order SMDO-based sliding-mode tracking control scheme for the fractional-order nonlinear system with external disturbances.
5.3.1 Example 1
The fractional-order nonlinear system in the presence of external disturbances and control inputs can be described as follows:
where ![]() and
and ![]() are system state variables,
are system state variables, ![]() and
and ![]() are control inputs, and
are control inputs, and ![]() and
and ![]() are external disturbances.
are external disturbances.
For the numerical simulation of the fractional-order system (5.24), to reduce sliding-mode chattering, the saturation function will be used instead of the sign function; this has the following form:
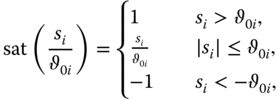

where ![]() and
and ![]() are design parameters.
are design parameters.
In the simulation, the fractional order is chosen as ![]() and the initial condition of the system (5.24) is given by
and the initial condition of the system (5.24) is given by ![]() . The control parameters are chosen as
. The control parameters are chosen as ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and ![]() . The desired trajectories are chosen as
. The desired trajectories are chosen as ![]() and
and ![]() . The external disturbances are assumed as
. The external disturbances are assumed as ![]() and
and ![]() . According to the result of Ishteva [225], we have
. According to the result of Ishteva [225], we have ![]() , where
, where ![]() denotes the square root of minus one and
denotes the square root of minus one and ![]() and
and ![]() are arbitrary numbers. In this simulation, the parameter
are arbitrary numbers. In this simulation, the parameter ![]() and the fractional order
and the fractional order ![]() . Thus, Assumption 5.1 and Assumption 5.2 are satisfied.
. Thus, Assumption 5.1 and Assumption 5.2 are satisfied.
The tracking results of the fractional-order nonlinear system (5.24) with external disturbances are shown in Figure 5.1 under the proposed sliding-control scheme. From the simulation results, the tracking performance of fractional-order nonlinear system (5.24) is satisfactory, as shown in Figure 5.2. Furthermore, the observation performance of the proposed fractional-order SMDO (5.3) is presented in Figure 5.3 and Figure 5.4. It is evident from Figure 5.3 and Figure 5.4 that the disturbance observer is effective and feasible. The control input signals are shown in Figure 5.5. It is concluded from these simulation results that the proposed sliding-mode control technique for the fractional-order nonlinear system using fractional-order SMDO is effective.

Figure 5.1 Output  of fractional-order system (5.24) follows desired trajectory
of fractional-order system (5.24) follows desired trajectory  : (a)
: (a)  and
and 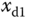 ; (b)
; (b)  and
and 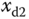 .
.

Figure 5.2 Tracking errors of fractional-order system (5.24): 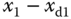 and
and 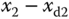 .
.

Figure 5.3 Disturbance  and approximate output of
and approximate output of  for the simulation of fractional-order system (5.24): (a)
for the simulation of fractional-order system (5.24): (a)  and
and  ; (b)
; (b)  and
and  .
.
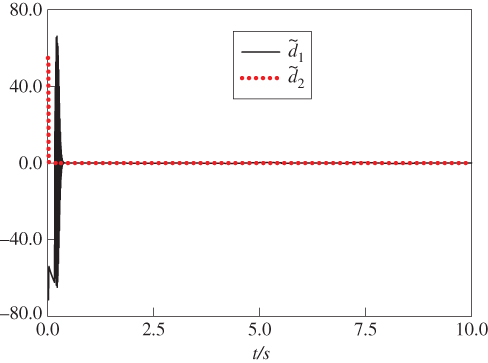
Figure 5.4 Estimation errors  and
and  for disturbances
for disturbances 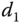 and
and  in fractional-order system (5.24).
in fractional-order system (5.24).
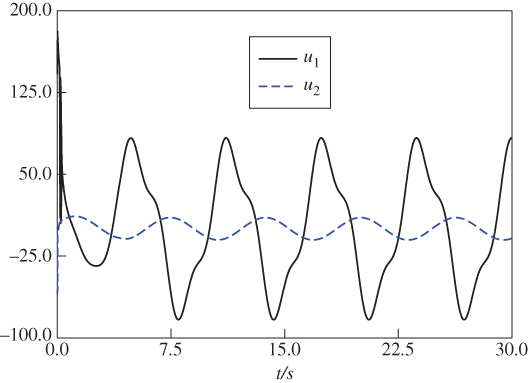
Figure 5.5 Control inputs  and
and  of fractional-order system (5.24).
of fractional-order system (5.24).
5.3.2 Example 2
Consider the following fractional-order nonlinear time-varying system with the following external disturbances and control inputs:
where ![]() and
and ![]() are system state variables,
are system state variables, ![]() and
and ![]() are control inputs, and
are control inputs, and ![]() and
and ![]() are external disturbances.
are external disturbances.
To reduce sliding-mode chattering, the saturation function will be used instead of the sign function; this has the following form:

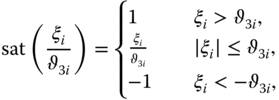
where ![]() and
and ![]() are design parameters.
are design parameters.
For the simulation, the fractional order ![]() , the initial conditions of system (5.27) are chosen as
, the initial conditions of system (5.27) are chosen as ![]() . The control parameters are chosen as
. The control parameters are chosen as ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and ![]() . The desired trajectories are chosen as
. The desired trajectories are chosen as ![]() . The external disturbances are assumed as
. The external disturbances are assumed as ![]() and
and ![]() .
.
On the basis of the proposed sliding-control scheme, the tracking results are shown in Figure 5.6 for the fractional-order nonlinear system (5.27) with external disturbances. We note that satisfactory tracking performance is obtained based on the simulation results. Furthermore, the disturbance estimation results are presented in Figure 5.7 and Figure 5.8. According to Figure 5.7 and Figure 5.8, we can obtain that the disturbance observer is effective and feasible. The control input signals, which are bounded, are shown in Figure 5.9. Therefore, the proposed sliding-mode control technique for the fractional-order nonlinear system using fractional-order SMDO is effective, based on the simulation results.
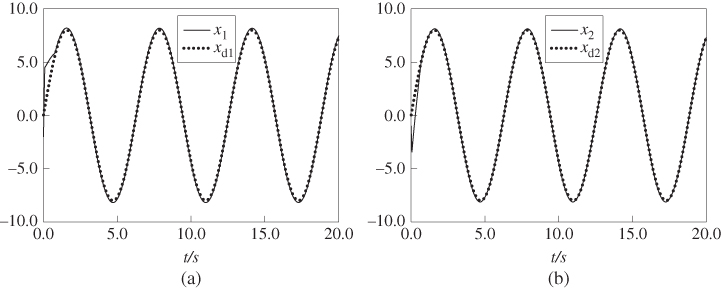
Figure 5.6 Output  of fractional-order system (5.27) follows desired trajectory
of fractional-order system (5.27) follows desired trajectory 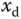 : (a)
: (a)  and
and 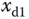 ; (b)
; (b)  and
and  .
.

Figure 5.7 Disturbance estimation results for the simulation of fractional-order system (5.27): (a)  and
and  ; (b)
; (b)  and
and  .
.
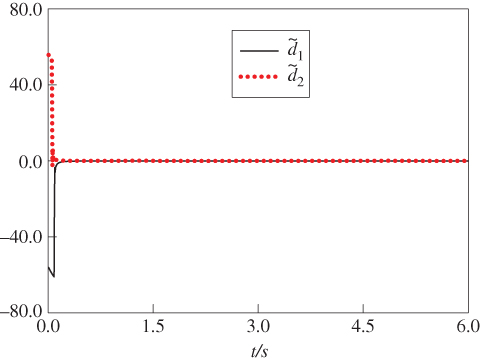
Figure 5.8 Estimation errors  and
and  for disturbances
for disturbances  and
and 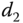 in fractional-order system (5.27).
in fractional-order system (5.27).
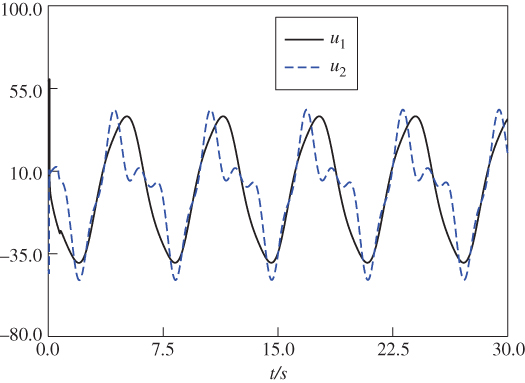
Figure 5.9 Control inputs  and
and  of fractional-order system (5.27).
of fractional-order system (5.27).
5.4 Conclusion
A sliding-mode control scheme has been proposed for a class of fractional-order nonlinear systems using a fractional-order SMDO in this chapter. The SMDO has been designed to guarantee the convergence of the disturbance estimation error. Using the developed disturbance observer, the sliding-mode control scheme has been developed to guarantee the convergence of all closed-loop system signals. Numerical simulation results have been presented to show the effectiveness of the developed fractional-order SMDO-based tracking control scheme under the effect of the unknown disturbances.