Thinking and Comprehending in the Mathematics Classroom

 

DOUGLAS FISHER, NANCY FREY, AND HEATHER ANDERSON

Literacy—reading, writing, speaking, and listening—is a critical foundational skill that provides individuals access to information in all other disciplines and domains. As teachers and researchers, we know that literacy impacts every aspect of a person's life, from success in school and work to living a productive life. As Shanahan (2007) noted in his International Reading Association keynote, low levels of literacy put people at risk in all kinds of ways, from being taken advantage of by scam artists to not understanding health information.

Literacy involves more than learning how to read. Breaking the code and developing fluency are important aspects in the development of a literate life. But that's not our focus in this chapter. We're interested in how literacy, broadly defined to include thinking in words and images, impacts content learning and achievement. This book has examples of successful content literacy initiatives in every subject area. Our focus in this chapter is mathematics and the ways in which literacy and numeracy can be integrated such that students learn more content.

Mathematics Knowledge Is Critical

Failing a year of mathematics is highly correlated with failures in future years of school and difficulty in finding gainful employment (Nichols, 2003; Thompson and Lewis, 2005). Math, specifically algebra, is a gatekeeper course. Haycock (2003) says, “Just as we educators have learned that courses like Algebra II are the gatekeepers to higher education, we must now come to understand that they are gatekeepers to well-paying jobs, as well.”

Failure in mathematics is also a common cause of college dropouts (Heck and Van Gastel, 2006). Colleges spend significant resources remediating students in mathematics, most commonly college-level algebra. The largest higher educational system in the world, the California State University (CSU), has established goals to reduce the number of students who require remedial instruction upon entering college. As part of the CSU effort, an Early Assessment Program (EAP) was developed. This is an optional assessment given to 11th graders that provides students and their families with feedback about readiness for college algebra, the first in a sequence of required mathematics courses for undergraduates. Of 141,648 students who took the math test in 2007, only 77,870 (55%) demonstrated proficiency. Remember that these are the students who chose to take the exam thinking that they were ready for college. Data from the ACT is even worse. Of the 1.2 million students tested, just 40% were ready for their first course in college algebra (ACT, 2004).

Although it can be argued that students are doing better today than they have in the past, there is a clear and immediate need to continue to raise mathematics achievement. Thankfully, there is evidence for how to do this. Before we explore three ideas for using literacy to improve understanding in mathematics, let's recall how many students experience math class. The following comes from Doug's experience in a ninth- grade algebra class.

A Common Experience in Math Class

The day starts like every other so far this semester. As we enter the room, our teacher calls off odd numbers. By now, we know that when we're called on like this, we have to solve the assigned homework problem on the board. Our teacher watches the group of students assigned to complete the problems and offers periodic criticisms and compliments. When everyone is finished and the answers are correctly posted on the board, we check our homework and then pass it forward to the teacher (of course, we've all checked our homework on the bus to make sure we've all got the same answers because homework counts for 25% of the grade).

With the homework review complete, now 15 minutes into the period, our teacher rolls out the overhead projector. It's the kind with rollers on both sides so that the transparency paper slides across as he finishes writing. He solves algebra problems for us for about 15 minutes. It's the last period of the day and his hands have blue stains from the number of times he's spit on them to erase.

Our task during this time is to take notes exactly as he presents them. Our notebooks must have specific page numbers that match his and are worth 25% of our grade. He provides us with a table of contents for our notes during the first week of school, and we are to keep the page numbers current. For example, as noted in Figure 1, page 13 focuses on “inverse of functions.” If we want to take notes on examples, we are told to add pages such as 13a, 13b, etc., so that we do not make mistakes with the numbering system. We do not summarize our notes or organize them in any systematic way; we copy them exactly as they are presented in class.

When he finishes the lecture and note-taking component of the class, we have the remainder of the period available to start our odd-numbered problem set for the day. If we do not finish the problems in class, we are to take them home and complete the rest. If we talk during class, our teacher will call out an even- numbered problem for which there is no answer in the back of the book. As punishment for talking, we have to go to the board and attempt to demonstrate our prowess in front of our peers. Obviously, we quickly learned to be quiet in this class! We also learned to talk with one another outside of the class to check answers and get help.

Every fourth week, we have a test. The tests comprise the remaining 50% of our grade and include long lists of problems to solve, selecting from the correct multiple-choice answer. We never had to explain our thinking, either orally or in writing. We just needed to select the correct answer from the choices provided. As our teacher said many times during the year, “This is what you'll have to do on the standards test, so you might as well get used to it now.'”

You're probably asking yourself, “How will anyone learn anything in this class?” Yet our experiences, and probably yours, bear out the fact that this type of instruction is very common in mathematics classrooms. To analyze the scenario a bit further, it is clear that the teacher values practice—he provides his students with lots of opportunities to engage in independent learning. He also values students having correct information and right answers. He wants their notes to be exact, and he wants students to practice testing formats. Unfortunately, this teacher has no way of understanding his students' thinking and the types of errors they make. Although he explains information, he doesn't let his students in on his thinking—the thinking of an expert.

Figure 1. Sample note page.

With a few adjustments to the structure of the classroom, students would likely develop a deeper understanding of mathematics and begin to see the relevance of this content in their lives. The remainder of this chapter focuses on three areas that we know to be effective ways to engage students in thinking about mathematics (e.g., Fisher and Frey, 2007): modeling, vocabulary development, and productive group work.

Engaging Students in Thinking about Mathematics

MODELING

There exist decades of evidence that teacher modeling positively impacts student performance and achievement (Afflerbach and Johnston, 1984; Duffy, 2003; Olson and Land, 2007). Modeling provides students with examples of the thinking required, as well as the language demands, of the task at hand. In essence, the student gets to peer inside the mind of an expert to see how that person thinks about, processes, and solves a problem.

Unfortunately, there is significant confusion between modeling and explaining. Think of a lecture you've attended. It was probably full of explanations. And explanations aren't all bad. We all need things explained to us sometimes. But we also need modeling, which personalizes the experience for the learner as the teacher uses “I” statements to share his or her thinking. Modeling also provides information about the cognitive process that went on in the mind of the expert; it's the why that we're after here. But as Duffy (2003) pointed out, “The only way to model thinking is to talk about how to do it. That is, we provide a verbal description of the thinking one does or, more accurately, an approximation of the thinking involved” (p. 11).

Accordingly, mathematics teachers must model their thinking by talking and thinking aloud for their students. Some of the common areas of thought that math teachers model include:

• Background knowledge (e.g., “When I see a triangle, I remember that the angles have to add up to 180°.”).

• Relevant versus irrelevant information (e.g., “I've read this problem twice, and I know that there is information included that I don't need.”).

• Selecting a function (e.g., “The problem says 'increased by,' so I know that I'll have to add.”).

• Setting up the problem (e.g., “The first thing that I will do is…because…”).

TABLE 1.
Math signal words

Function	Sample terms	Examples
Words that signal addition	and, made larger, more than, in addition, sum, in excess, added to, plus, add, greater, increased by, raised by	Forty-five and twenty-two are what? Translation: 45 + 22 =
Words that signal subtraction	decreased by, subtract, difference, from, made smaller by, diminished by, reduce, less than, minus, take away	If you take away 3 from 29, what do you have left? Translation: 29 – 9 =
Words that signal multiplication	product, multiplied by times as much, of, times, doubled, tripled, etc., percent of interest on	What is the product of fourteen and sixteen? Translation: 14 × 16 =
Words that signal division	per, quotient, go(es) into, how many, divided by, contained in	How many times can 5 go into 100? Translation: 100/5 =

• Estimating answers (e.g., “I predict that the product will be about 150 because I see that there are 10 times the number.”).

• Determining reasonableness of an answer (e.g., “I'm not done yet as I have to check to see if my answer makes sense.”).

Let's listen in on Heather's modeling of her thinking relative to the algebra problem: The sum of one-fifth p and 38 is as much as twice p. In her words:

“Okay, I've read the problem twice, and I have a sense of what they're asking me. I see the term sum, so I know that I'm going to be adding. I know this because sum is one of the signal words that are used in math problems [for a list of signal words, see Table 1]. I also know that when terms are combined, like one-fifth p, they are related because they make a phrase 'one-fifth of p' so I'll write that l/5p. The next part says and 38, so I know that I'll be adding 38 to the equation. Now my equation reads l/5p 1 38. But I know that's not really an equation. I know from my experience that there has to be an equal sign someplace to make it an equation. Oh, they say as much as, which is just a fancier way of saying equal to. So, I'll add the equal sign to my equation: l/5p 1 38 = . And the last part is twice p. And there it is again, one of those combined phrases like one-fifth p, but this time twice p. So I'll put that on the other side of the equation: l/5p + 38 = 2p. That's all they're asking me to do. For this item, I just need to set up the equation. But I know that I can solve for p, and I like solutions. I know that you can solve for p as well. Can you do so on your dry erase boards?”

Heather clearly understands the task and expectations. But more importantly, she understands her own thinking on the subject. To be effective modelers, teachers have to move beyond their expert blind spots. Gladwell (2005) notes that even brilliant experts have biases and blind spots that prevent them from seeing the problem as it really is. Expert blind spots prevent teachers from recognizing content that would be helpful to students. Too often teachers are unaware that much of their subject matter knowledge, while second nature to them, is very difficult for their students to learn. Nathan and Petrosino (2003) noted in their study of new secondary math teachers that this blind spot was prevalent because they lacked the experience to recall how a new concept is acquired by a novice.

Our experience suggests that there is a good reason for these expert blind spots. The goal of instruction is for students to reach automaticity such that they no longer have to pay conscious attention to every aspect of the problem at hand. As students develop their understanding of mathematics, components become automatic. For example, by mid- elementary school, multiplication facts should have moved from conscious thought to automatic execution. This process frees up working memory such that the brain can work on other parts of the problem. Many math teachers have reached automaticity with mathematics in general and, as a result, have lost the awareness of their cognitive problem-solving processes. The key is to slow down thinking such that the process once again becomes clear. When you know what you think, it's easier to model for students. And simply said, students desperately need to witness experts in action.

VOCABULARY DEVELOPMENT

Returning to the modeling provided by Heather in the example above, it's hard not to notice the vocabulary that she used. In every academic endeavor, words matter. We use words to communicate with one another, and our selection of specific words is intended to convey specific information. The problem is that students often don't know what the words mean, especially in a mathematical context.

In response to this problem, teachers often identify words for students to learn. Of course, learning words requires much more than a list. It is also more than providing definitions for students to memorize, such as, “A polygon is a simple closed figure comprised of line segments.” Although definitional meaning is an aspect of learning a word, when it comes too early in the process it can confound rather than clarify. Consider the vocabulary embedded in that definition—you need to understand what a closed figure is, be able to identify a line segment, and know the meaning of comprised. Students have to engage with the words multiple times to get a sense of their meaning and usage. A number of instructional routines are useful in mathematics word learning (e.g., Fisher and Frey, 2008b), including the following:

• Word walls, on which teachers post 5—10 words on a wall space that is easily visible from anywhere in the room. The purpose of the word wall is to remind teachers to look for ways to bring words they want students to own back into the conversation so that students get many and varied experiences with those words (Ganske, 2006, 2008).

• Word cards, in which students analyze a word for its meaning, what it doesn't mean, and create a visual reminder. A sample card for the word rhombus can be seen in Figure 2.

• Word sorts, in which students arrange a list of words by their features. Word sorts can be open (students are not provided with categories) or closed (students are given categories in which to sort). An example of a word sort in which words could be used more than once can be found in Table 2.

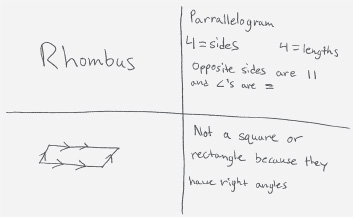
Figure 2. Sample word card.

TABLE 2.
Sample word sort

Circle	Square	Triangle
Radius	Area	Angle
Diameter	Perimeter	Area
Circumference	Quadrilateral	Altitude
Area	Angles	Sides
	Sides
	Length
	Height

• Word games, in which students play with words and their meanings. For example, this might involve a bingo game of sorts (Pat Cunningham calls it Wordo), wherein students write words from the class in various squares and then the teacher randomly draws definitions until someone gets bingo. We also like games such as Jeopardy, Who Wants to Be a Millionaire?, or $25,000 Pyramid because they allow students to review words while having a bitof fun. A great website that provides information about vocabulary games is http://jc-schools.net/tutorials/vocab/ppt-vocab.html.

These instructional routines are useful, especially for technical words, that is, those words that are specific to a discipline or content area. In mathematics, it's important that students learn the accepted meanings of words and phrases such as square root, polygon, linear equation, and Fibonacci sequence. Of course, those aren't the only words students need to know. In addition to technical words, students in mathematics classrooms must learn the intended mathematical meanings of common words. These are known as the specialized vocabulary terms as they tend to change their meaning in different contexts. For example, the word prime has a common meaning related to the best in quality, as in prime beef. However, in mathematics the term takes on a specialized meaning: a number that is only divisible by itself and 1. Our experience suggests that students need these differences made explicit to them, especially English language learners who may know one meaning of a term, but a meaning that does not help them understand the mathematics. For example, one of our students understood the term expression, having heard it in her English language development (ELD) class. That teacher talked about facial expressions and reading social clues through expressions. The newcomers in the ELD class developed an appreciation of the term expression and were able to read nonverbal clues in their new environment. However, when this student enrolled in algebra, the knowledge she had about the term failed her. She had no idea what the teacher meant when he said, “Let's write an expression for the information we have” or “Evaluate the expression 5 × z + 12 when z = 3.”

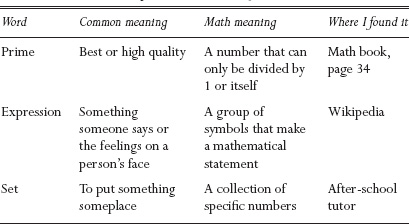
The best way we've found to teach and reinforce specialized vocabulary is through a mathematics journal such as the one in Table 3. Of course this requires that teachers notice the specialized vocabulary in their speaking and in the texts they use. It also requires that teachers take the time to provide instruction on the difference between the common meaning of the word and the math-specific definition or usage. But as the student who did not understand the mathematical use of expression taught us, focusing on specialized vocabulary terms is time well spent.

The first two areas that we've presented have focused a lot on the teacher: The teacher provides expert modeling, the teacher determines which words are worthy of instruction, and then the teacher provides time for students to engage effectively with the words. The final area of attention focuses on the role of students.To significantly raise achievement in mathematics, students have to have opportunities to interact with one another in regard to content.

TABLE 3.
Sample mathematics journal

PRODUCTIVE GROUP WORK

Unlike the classroom scenario that we used to start this chapter, classrooms that work well are filled with student talk, student interaction, and meaningful work. Simply listening to math instruction and then doing math problems will not result in learners who understand and use mathematical concepts in their daily lives, much less in their college classrooms. As noted in the opening scenario, students want, and need, to talk about the content. Our experience suggests that the productive group-work phase of instruction allows students to consolidate their thinking about the content. In that respect, it's a critical aspect of learning. Importantly, we have evidence that students use the information modeled by the teachers when they are working with their peers. And even more importantly, independent work is of higher quality when students have first had an opportunity to collaborate with others. In fact, this aspect is so important that it is one of the foundational principals of Working on the Work (Schlechty, 2002), a reform effort with the aim of improving student achievement by focusing on the tasks students are asked to complete.

The following five features should be considered in any productive group-work task (Fisher and Frey, 2008a; Johnson, Johnson, and Smith, 1991).

1. Positive interdependence. Members must see how their efforts contribute to the overall success of the group. The task cannot be one that individuals could have completed independently. Rather, the task has to have at least an aspect of interdependence such that students need each other to complete their work successfully.

2. Face-to-face interaction. As part of the task, group members must have time to interact live. While they can also interact in virtual and electronic worlds, our experience suggests that the opportunity to interact on the physical level encourages accountability, feedback, and support.

3. Individual and group accountability. As we have noted, productive group work is not simply a matter of having a group of students complete a task in parallel with peers that they could have done alone. Having said that, we also know that the risk of productive group work lies in participation. In nearly every group, there are likely members who would allow their peers to complete the required tasks. To address this issue, each member of the group must be accountable for some aspect of the task. Of course, this is a perfect opportunity to differentiate based on students' needs and strengths. In addition to the individual accountability, the group must be accountable for the overall product. This also ensures that overly involved students will not monopolize the conversations during productive group work.

4. Interpersonal and small-group skills. One of the opportunities presented during productive group work is social skill development. Wise teachers are clear about their expectations related to interpersonal skills and communicate these to students. For example, during a group brainstorming session about ways to represent the concept of slope, Heather reminds her students that “put-downs for ideas are not allowed, especially during a brainstorming session.”

5. Group processing. As part of the learning associated with productive group work, students need to learn how to think about, and discuss, their experiences. The goal of the discussion is for students to consider ways in which they can improve their productivity and working relationships.
 

Following her modeling in which she thinks aloud about a problem and its solution, Heather provides groups of three students (triads) with a problem. Each triad has the same problem as one other triad, and the two triads with the same problem will discuss their results at the end of the class session. Before doing so, students in each triad must solve the problem with words, numbers, and pictures. They are working on reasonableness of their answers. One of the groups received the problem: If Esme cuts an apple into 8 equal pieces and gives Kaila 1 piece, how much of the apple is left? Is it reasonable to suggest that there is more than 50% of the apple left?

The group members go into action, first talking about the problem. Andrew asks how Esme got the pieces to be equal. Maria responds, “That's extraneous to the problem. We can ask that as a follow-up, but first we have to solve the problem.” Their individual accountability is widened by their separate ability to explain the solution to their teacher and another group. The group accountability includes a presentation of the solution in words, numbers, and a picture. This group decides that each member will take one of the required representations and work alone for a minute before sharing the results with the team.

Maria takes writing, Jamal takes numbers, and Andrew takes the picture, and they begin to work. As they finish, they trade papers for a quick review and an opportunity to ask clarifying questions. Jamal suggests that Maria add a sentence about subtracting fractions with common denominators. Andrew asks Jamal if the answer is reasonable, “You have the problem worked out, but I think you forgot the second part. Is it reasonable to suggest that there is more than 50% of the apple left?”

The students then discuss the answer and the various ways that they solved the problem. Using a timer, they each get 60 seconds to explain their thinking. When the 3 minutes are up, triads with the same problem meet. They each explain their thinking and their solutions to the members of the other triad that solved the same problem while Heather listens in on the conversations. Naturally, there are a number of ways that students solved their assignment problems and they're having a chance to hear about alternatives.

Returning to their triads, the students talk about what they learned from the experience. Heather reminds them to “Talk about what you learned about problem solving and also what you can do to make your triad more effective.” Maria talks about adding numbers to her writing like Sophia did. Andrew talks about how all six of them got the answer right, but in different ways. He says, “I like Michael's picture better than mine, but we got the same answer.” In response to increasing the productivity of the group, Jamal suggests that next time they each solve the problem two different ways (writing, numbers, or pictures) and then compare all of the different ways, “so that we'll know more ways to figure out what we have to do.”

The amount of student talk and student engagement in Heather's class is significant. Students know what is expected of them from the models she provides. They also learn a lot of words and have opportunities to use those words in context with their interactions with peers. And students in Heather's class collaborate on productive tasks that allow them to consolidate their understanding of mathematics. It's no wonder that Heather's students do so well on state assessments—they know the content very well because of the structures in place in the classroom.

Conclusion

Importantly, we are not suggesting that mathematics instructors become reading teachers any more than we are suggesting that reading specialists become math educators. However, we do recognize the value that modeling, vocabulary, and productive group work play in learning mathematics content, regardless of the level of mathematics being taught. Mathematics instructors, K—12, can improve student achievement through the use of key “literacy” instructional routines. We put the word literacy in quotations because the ideas presented in this chapter are not owned by reading teachers; they are ways to get students to think about and understand the content. And that's the goal of every teacher—to ensure that comprehension occurs.

References

ACT, Inc. (2004). National data release. Iowa City, Iowa: ACT.

Afflerbach, P., and Johnston, P. (1984). On the use of verbal reports in reading research. Journal of Reading Behavior, 16, 307–22.

Duffy, G. G. (2003). Explaining reading: A resource for teaching concepts, skills, and strategies. NewYork: Guilford Press.

Fisher, D., and Frey, N. (2007). A tale of two middle schools: The role of structure and instruction. Journal of Adolescent and Adult Literacy, 51, 204–11.

Fisher, D., and Frey, N. (2008a). Better learning through structured teaching: A framework for the gradual release of responsibility Alexandria, Va.: Association for Supervision and Curriculum Development.

Fisher, D., and Frey, N. (2008b). Word wise and content rich: Five essential steps to teaching academic vocabulary Portsmouth, N.H.: Heinemann.

Ganske, K. (2006). Word sorts and more: Sound, pattern and meaning explorations K–3. New York: Guilford Press.

Ganske, K. (2008). Mindful of words: Spelling and vocabulary explorations 4–8. NewYork: Guilford Press.

Gladwell, M. (2005). Blink: The power of thinking without thinking. NewYork, Little Brown.

Haycock, K. (2003). Foreword. In A. P. Carnevale and D. M. Desrochers, Standardsfor what? The economic roots of K–16 reform. Princeton, N.J.: Educational Testing Service.

Heck, A., and Van Gastel, L. (2006). Mathematics on the threshold. International Journal of Mathematical Education in Science and Technology, 37(8), 925–45.

Johnson, D. W., Johnson, R. T., and Smith, K. A. (1991). Active learning: Cooperation in the college classroom. Edina, Minn.: Interaction Book.

Nathan, M. J., and Petrosino, A. (2003). Expert blind spot among preservice teachers. American Educational Research Journal, 40, 905–28.

Nichols, J. D. (2003). Prediction indicators for students failing the state of Indiana high school graduation exam. Preventing School Failure, 47(3), 112–20.

Olson, C. B., and Land, R. (2007). A cognitive strategies approach to reading and writing instruction for English language learners in secondary school. Research in the Teaching of English, 41(3), 269–303.

Schlechty, P. C. (2002). Working on the work: An action plan for teachers, principals, and superintendents. San Francisco, Calif.: Jossey-Bass.

Shanahan, T. (2007, May 15). Presidential address for International Reading Association, Toronto, Canada.

Thompson, L. R., and Lewis, B. F. (2005). Shooting for the stars: A case study of the mathematics achievement and career attainment of an African American male high school student. High School Journal, 88(4), 6–18.