Chapter 9
Stick Solids

Construct geometric solids with toothpicks and bits of flip-flops.
Most Timorese women know how to weave several katupa designs. (See Chapter 5 to find out about the wonders of the katupa.) I know how to weave three. They took me hours to learn, and many more hours to relearn after I subsequently forgot them, again and again, as time passed and I didn’t make them on a regular basis.

Katupa are also woven in the miniature with dyed strips and hung around the house as colorful decorations. The one at the top here is in the shape of a bird.

One day I was appreciating one of these hanging decorations and I realized it contained mini-katupas of two of the Platonic solids. These five solids are formed entirely from edges of a constant length and faces of a single geometric figure. The ancient Greeks found them somewhat mystic, and I do too. They are symmetric around any vertex, and it can be proven that only the five exist. Fairly regular versions of the octahedron and tetrahedron were represented in the beautiful little handicraft decoration I was viewing. Here is a close-up of three green octahedron mini-katupas in a hanging decoration.

I’m not sure the Timorese women who wove these Platonic katupas understood their profound mathematical relevance, but I’ve taught many students, and they are generally filled with wonder. It’s but another example of the submerged mathematics that exists in the daily life of every culture and society. Nobody calls it math, but it indisputably is.
These solids and others can be constructed through several simple methods, and then you can analyze their mystical properties. I’ll show you a couple of other methods for making them at the end of this chapter, but the way we make them here is with toothpicks or kabob sticks and small cubes of old flip-flop rubber, or alternatively small cubes of potato, chayote, or young papaya. These food-based cubes are easier to prepare but often don’t last as long; they turn into mold experiments unless you dry them super well.
Instead of showing you how to construct these gems, I’ll lead you to construct them by following basic principles. Each of the five can be constructed by putting together uniform sticks at vertices following only two rules: the shape on each face must be always the same, and the number of sticks coming together at each vertex must also be the same. Start the table here to eventually include all the info and show more mystical aspects of these solids. Now, DON’T LOOK AHEAD! Instead prepare your sticks and cubes and start going.
Gather stuff
- Cubes of old flip-flop or potato, 1 to 1.5 cm on a side
- Toothpicks
- Bamboo skewers
- Thread
Gather tools
- Knife
- Scissors
| Shape on the faces | Number of sticks joining at each vertex | Number of faces | Number of vertices | Number of edges | Name | |
| 1 | Triangle | 3 | ||||
| 2 | Square | 3 | ||||
| 3 | Triangle | 4 | ||||
| 4 | Pentagon | 3 | ||||
| 5 | Triangle | 5 |
Tinker
You can use sticks of whatever length for this, although if you use kabob sticks they become unwieldy for solids #4 and #5. To use the kabob sticks, you have to sharpen the non-pointy end in order to stab it into another cube. Toothpicks have two ends nicely sharpened.
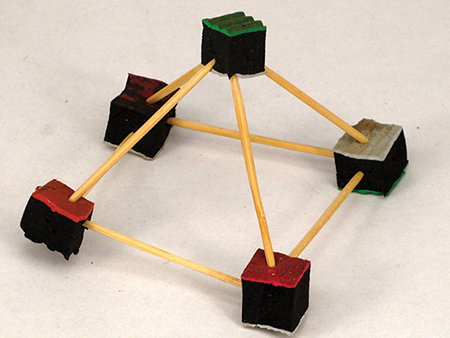
For solid #1, stab three sticks into a cube to form a corner.

Then put more cubes on the other ends to form triangles. Here’s the first one:
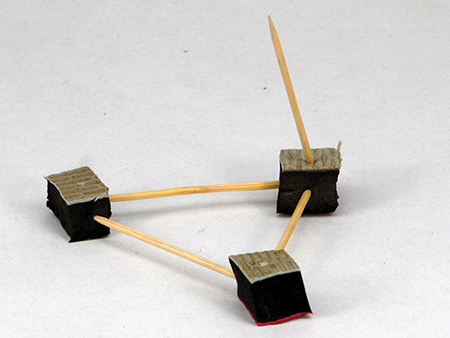
Now continue linking sticks to cubes until you have a complete solid. To get the full challenge, try to do this without looking at the photo that follows. Just keep following the two rules:
- Always form triangles
- Always have three sticks coming together at a corner.
How’d you do? Did you get a little pyramid thingy like this?

Turn it around and be sure it looks the same from each side and each vertex.

There’s your first Platonic solid: the tetrahedron. It’s a pyramid with a triangle base. You can make many other pyramids with triangular bases that are not like this one—that is, they have longer or shorter sticks on one or more sides, making different angles at the corners, and thus don’t have the super symmetry of this baby.
Now count the faces, vertices, and edges, and fill in the table:
| Shape on the faces | Sticks at each vertex | faces | vertices | edges | Name | |
1 | Triangle | 3 | 4 | 4 | 6 | Tetrahedron |
Now you’re ready for the next. Again, try to cover up the following images and figure it out just from these rules! The two rules for this one are
- Always form squares.
- Always have three sticks coming together at a corner.
Start in the same way, but know that the angles will be a bit different on this, as it will turn out to be a different solid.

Now make squares on each side.
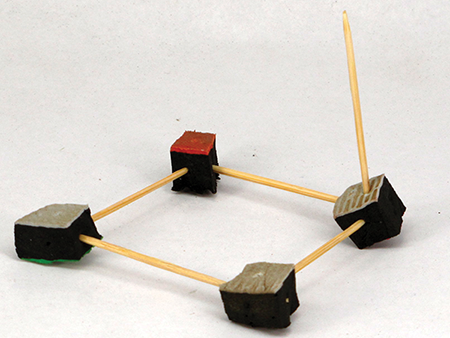
And continue making squares from three-stick vertices!
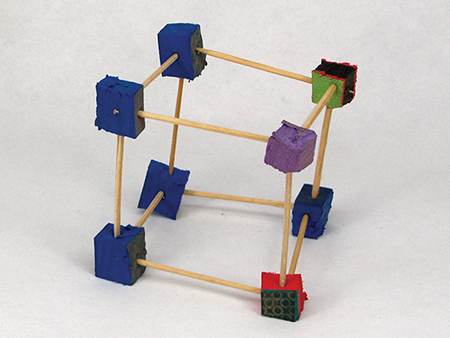
Ta-daaa! That looks familiar, eh? Technically speaking it’s a hexahedron, but you can just call it a cube. Make sure it’s symmetric from all faces and corners. Then fill in your table.
| Shape on the faces | Sticks at each vertex | faces | vertices | edges | Name | |
1 | Triangle | 3 | 4 | 4 | 6 | Tetrahedron |
| 2 | Square | 3 | 6 | 8 | 12 | Hexahedron (Cube) |
Now you begin to see the complexity of these things. Each of the number columns went up, but two doubled while one went by half. Who knows what lies ahead?
Continue with this process to construct the next three. On the next page are the starter hints followed by the answers. Cover the answers and try to work out the shapes just with the hints!!
 3 Always form triangles. Always have four sticks coming together at a corner. |
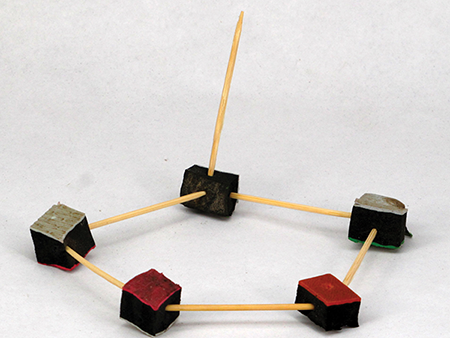 4 Always form pentagons. Always have three sticks coming together at a corner. |
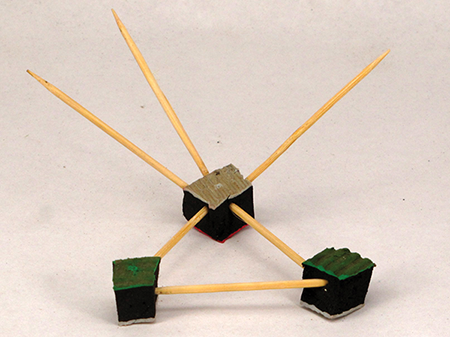 5 Always form triangles. Always have five sticks coming together at a corner. |

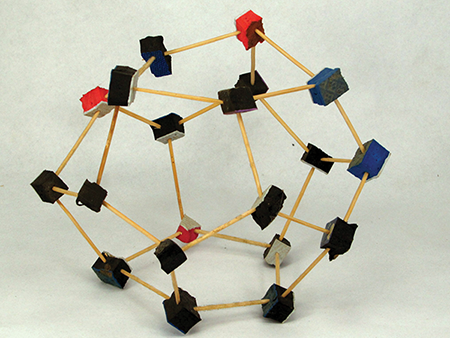
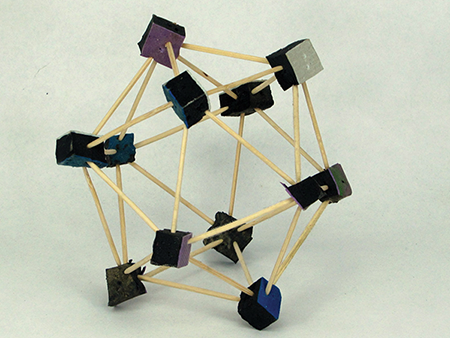
There they stand in all their glory. You know eventually you gotta have these hanging from your ceiling, but first fill in the table, then check it with ours:
| Shape on the faces | sticks at each vertex | faces | vertices | edges | Name | |
1 | Triangle | 3 | 4 | 4 | 6 | Tetrahedron |
2 | Square | 3 | 6 | 8 | 12 | Hexahedron (Cube) |
3 | Triangle | 4 | 6 | 12 | Octahedron | |
4 | Pentagon | 3 | 12 | 20 | 30 | Dodecahedron |
5 | Triangle | 5 | 12 | 30 | Icosahedron |
That’s a lot to chew on. First check out those names. If they seem Greek, it’s because they are. Check out the Greek prefixes for the numbers in that table:
| 3 | tri |
| 4 | tetra |
| 5 | penta |
| 6 | hexa |
| 8 | octo |
| 10 | deca |
| 12 | dodeca |
| 20 | icosa |
For geometric concerns, English uses the Greek prefixes and various other Greek language structures. Hedron means 3D shape, as you probably guessed.
Now compare the results in the number columns. You might have thought that as things grow more complex, the numbers in each column would increase, but in several cases, they decrease. And in some cases they swap directly. I put some arrows in there to emphasize that. Also note that the edge number remains the same for two pairs, which have the same background color in the table above. Cosmic.
What’s Going On?
Polyhedra are the subject of intense academic study and have been since the time of old Plato, around 2,400 years ago. Plato referred to this set of solids in a philosophical piece about the fundamental elements of the universe, and suggested that our universe is formed from these polyhedra.
A contemporary of Plato named Theaetetus did the original proof that there are but five. You can gain some insight by trying (futilely) to create another one. Just choose a regular shape (in geometry, “regular” means all the edges are of equal length) and try to make a solid with it! Go for it! Ask your Uncle Google if you want the details.
A few words on the elegance and symmetry of the characteristics in the table: It just so happens that there is an intimate relationship between the two pairs: cube and octahedron, Dodecahedron and icosahedron. They are known as “dual polyhedra,” which means that one’s faces correspond to the other’s vertices.
What about the lonely tetrahedron? If you morph its vertices into faces and faces into vertices you get another tetrahedron, the evil twin, technically called its “dual.” Your good Aunt Wikipedia has got some nice animations of the metamorphosis between the dual polyhedra.
Did you notice that the ones with triangles are rock solid, sort of unmovable, whereas the cube and especially the dodecahedron felt kind of gangly, unsolid, and ready to collapse? That’s the magic of the triangle, and why it’s essential in construction. In the three solids with triangles, the triangles are holding the angles steady, whereas in the cube and dodecahedron, we rely on the flip-flop cube to hold the angles steady.
You don’t have to stop at the Platonic solids. There also exist 13 Archimedean solids, which relax the rule about a single shape for a face. You’ll learn more about Archimedes in Chapter 20. Here are two of his solids:
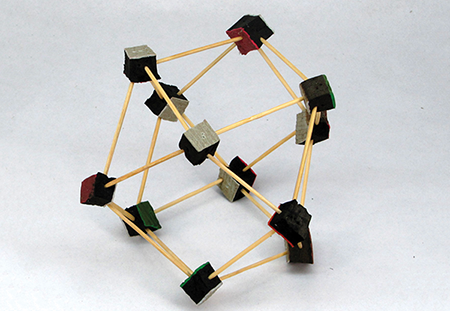
That’s a cuboctahedron, made with triangles and squares. There’s a different one you can make with just squares and triangle; I’ll let you tinker it out yourself.

That’d be an icosidodecahedron, made of triangles and pentagons, and it’s worth making if only to have an opportunity to say that word. These names are not arbitrary Greek mishmashes; they have connections to other solids that can be truncated or morphed to become these solids. If you count, you can find all 20 triangles of the icosahedron and all 12 pentagons of the dodecahedron right here in this baby. And in that previous one, all 6 squares of a cube and all 8 triangles of an octahedron. Radical, eh?
I have found the icosidodecahedron on soccer balls here, which makes sense, because it’s close to a sphere. The following photo shows a bag of balls hanging in a local shop.

But look closely: those triangles are each painted on the inside of a hexagon! The painted design is different from actual lines on the ball! The actual design there is the Buckyball, which is found on most soccer balls. It’s named after the mathematical architect Buckminster Fuller, who built domes from this and other shapes and called them “geodesic.” The Buckyball is an Archimedean solid formed from hexagons and pentagons. It’s also called the truncated icosahedron, because it is formed by chopping each vertex off an icosahedron. You can make it as well from toothpicks and flip-flops!
Check out how this cheap toy soccer ball has got a pentagonal star painted into each pentagon of the Buckyball. Clever!

Then there are the Johnson solids, still demanding a uniform edge length to create regular polygons, but relaxing other symmetry restrictions. The other pyramids are examples of these solids.
Your Aunt Wikipedia has many scrumptious photos and animations of these fabulous forms and will be more than happy to show you all the Shläfli symbols and Coxeter diagrams (haven’t quite figured those out myself), as well as working out the surface area and volume of each. The website http://blazelabs.com/f-p-swave.asp (about the structure of atoms) has a great synopsis of the Platonic and Archimedean solids, all derived from the lowly tetrahedron. Mathisfun.com/geometry has interactive animations of many solids. I’m confident you’ll find perusing these solids on the Internet far more enjoyable than watching another Justin Bieber video.
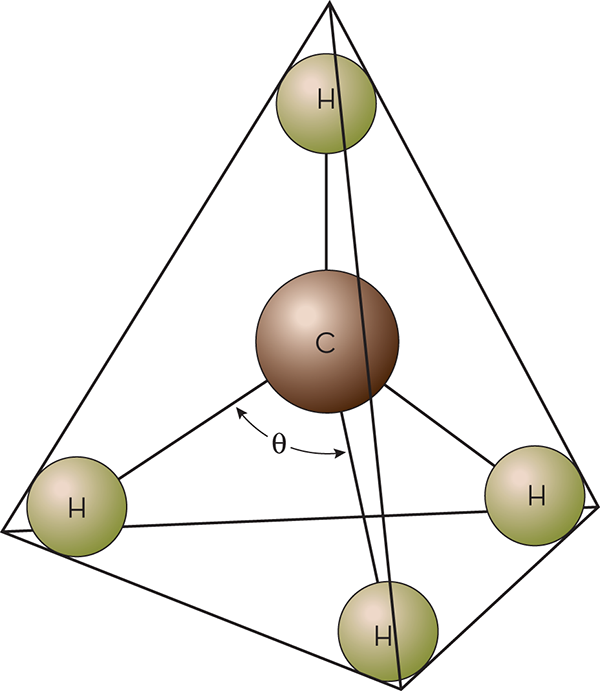
So that was all thrilling and soul quenching, but what’s the point? Is it just abstract mathematics and a treat to the eye? Not at all, my friend. You see, various molecules and crystals have been found in exactly these shapes, including methane, the simplest organic molecule, with four hydrogen atoms positioned at the vertices of a tetrahedron:
And here is the tetrahedron katupa, full sized and all tied up fancy for mathematical analysis (not cooking rice). My clumsy weaving has resulted in a slightly skewed polyhedron, but with effort, this can be made perfectly regular.

Then there is the Circogonia icosahedra, a species of Radiolaria, which are single-celled organisms that make themselves complex exoskeletons. This one is shaped like a regular icosahedron:
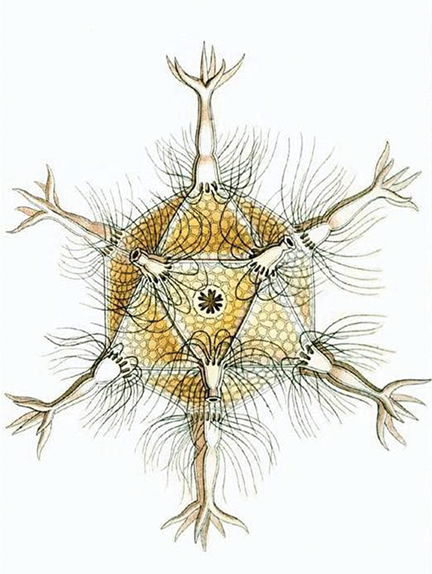
CREDIT Ernst Haeckel’s 1904 Kunstformen der Natur
Basically, the shapes are sacred; they are universal forms, elegant and efficient, with symmetries so strong that nature produces them. In that respect, old Plato was right! Learning them is as enjoyable and important as learning astronomy or microbiology: this is the reality of our universe! Hang them in your room and tell all your friends to come and see!
