
14 1. TOOLS FOR UNDERSTANDING SPACE
• We define the stellar parallax angle in terms of the right triangle made by bisecting the
isosceles parallax triangle, as shown in Figure 1.8. And so relative to this angle, the baseline
is the radius of Earth’s orbit—that is, the astronomical unit itself.
And so we can define a new unit of distance, the parsec, as follows:
A parsec (pc) is the distance an object (such as a star) would need to be in order to
have a stellar parallax of exactly 1 arcsecond.
Notice that the word “parsec” is simply an invented contraction of the words “parallax” and
“second.” Since 1 radian D 206; 265
2
, it is easy to see that the parsec is equivalent to 206,265 AU.
is is a huge distance, approximately equal to 3.26 ly.
Given this definition of the parsec, we can see from Equation (1.11) that the following
very simple relation must hold between the distance, d , to a star as measured in parsecs and its
parallax angle, p, in arcseconds:
d.parsecs/ D
1
p.arcseconds/
: (1.20)
Proxima Centauri is the nearest star to us (other than the Sun of course), and with a
measured parallax of 0:7686
2
, it has a distance of 1.301 pc.
1.3 SCALING AND SCALE MODELS
Most of the distances and sizes in the universe are either too tiny or too large to experience
directly. But even when the numbers themselves are far outside the range of our direct experience,
we can still describe the relationships between those numbers. We may then form a scale model
that preserves the size and distance relationships, while scaling everything together to sizes and
distances that are within the easy reach of human perception.
We will use this technique in Chapter 2 to illustrate the relationship between the sizes
and distances of bodies within the solar system. Let us here, as an introduction, set up a sim-
ple numerical example for a scale model of the three solar-system bodies most important to
humans—Earth, Moon, and Sun.
6
Earth, Moon, and Sun are all (approximately) spheres, each with its own radius. e Moon
orbits Earth at a particular (average) distance, while much farther away the Earth–Moon pair
orbits the Sun. In what follows, I use the standard astrological symbols for Earth (C), Moon
(K), and Sun (@) as subscripts to denote these bodies, placed on R for radius or d for distance
from Earth. And so, for example, R
C
means “radius of Earth.” In SI units of meters, we have
the following data:
R
C
D 6:380 ˆ 10
6
m
R
K
D 1:738 ˆ 10
6
m d
K
D 3:84 ˆ 10
8
m
R
@
D 6:963 ˆ 10
8
m d
@
D 1:496 ˆ 10
11
m .
6
Do not look directly at the Sun!

1.3. SCALING AND SCALE MODELS 15
I have put these numbers all in the same units of meters, and this allows them to be directly
compared to each other. As described in Section 1.1, we can learn a lot simply by comparing the
exponents in the scientific notation. Only a glance is needed to see that Earth and the Moon
have sizes that are within a factor of 10 of each other, but the Sun’s radius is two powers of ten
(a factor of 100) larger. Also notice that the distance to the Moon has the same power of ten as
the radius of the Sun, but the distance to the Sun is three orders of magnitude greater than the
distance to the Moon.
To make a scale model out of this data, we multiply all of the numbers by the same scale
factor. Of crucial importance is that all of the numbers are lengths (as opposed to, for example,
volumes), and that they are all expressed in the same units, before multiplying by the scale factor.
e trick is to choose a scale factor such that all of the scaled lengths are then of a size that is
within the range of direct human experience. If these sizes can be related to familiar objects, so
much the better.
For this particular example, the numbers are all too large for our experience, and so we need
to make them smaller in our scale model. us, we need a scale factor that is a tiny fraction, much
less than unity. Equivalently, we could choose a large scale divisor instead of a factor, dividing all
of our numbers by the same large number. But whether we multiply by a tiny number or divide
by a large one, the goal is to put all of the numbers on a human scale.
We have two rather obvious choices as a starting guess for an appropriate scale factor.
On the one hand, we can try to scale down the smallest-size object (the radius of the Moon in
this example) to the smallest size we can directly experience—a grain of salt, for example. Or
alternatively, we can choose a scale factor such that the largest distance (the distance to the Sun
in this example) is now just barely small enough to experience directly and easily.
Let us make both of these choices and see what we get. And so let us choose on the one
hand a scale factor that puts the Moon down to the size of a grain of salt, scaling all of the other
numbers by that same factor. And for comparison, let us choose a different scale factor that
puts the Earth–Sun distance at, say, 100 m—roughly the length of a soccer pitch or American
football field, a familiar distance that is large, but that one can easily see or walk across.
It is simple to determine the first scale factor example, which I will represent as
s
1
. We
want a value for s
1
such that when it is multiplied by the diameter of the Moon (twice the radius
D 2R
K
), we get a size equivalent to the width of a grain of salt (d
salt
):
2R
K
s
1
D d
salt
(1.21)
s
1
D
d
salt
2R
: (1.22)
If we choose the width of a typical grain of salt to be one third of a millimeter (3:33 ˆ 10
´4
m),
then we have:
s
1
D
3:33 ˆ 10
´4
m
2 ˆ 1:738 ˆ 10
6
m
D 9:58 ˆ 10
´11
: (1.23)
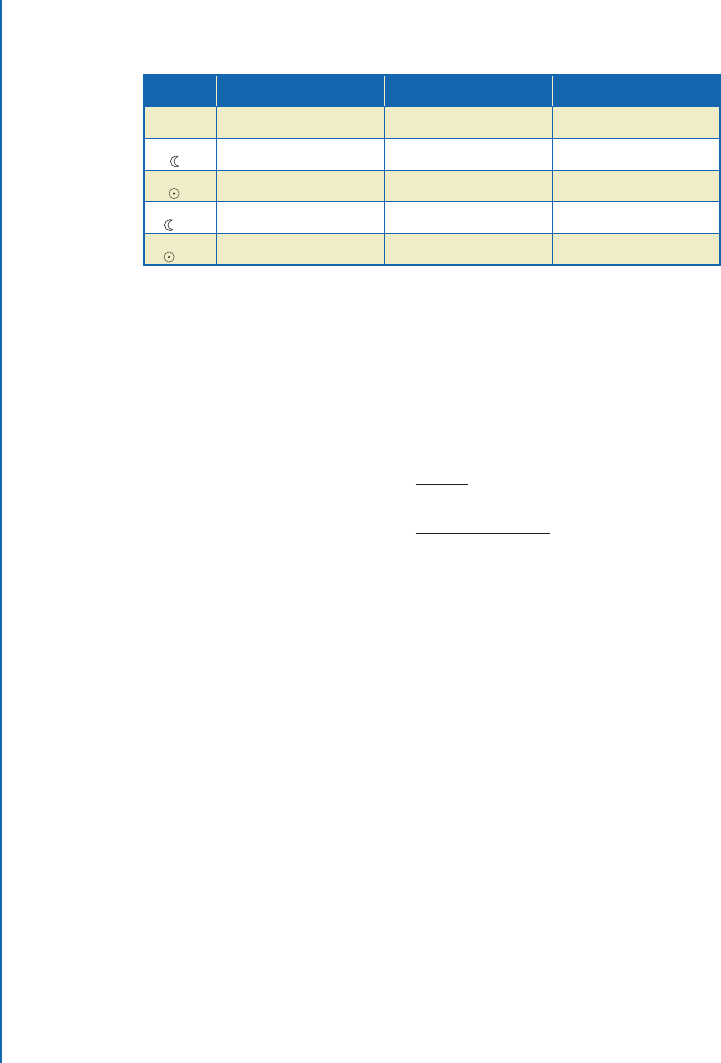
16 1. TOOLS FOR UNDERSTANDING SPACE
Table 1.2: Two examples of scale models for the Earth–Moon–Sun system, using two different
scale factors
Real Value (m)
× s
1
(m) × s
2
(m)
R
⊕
6.380 × 10
6
6.11 × 10
-4
4.26 × 10
-3
R
1.738 × 10
6
1.67 × 10
-4
1.16 × 10
-3
R
6.963 × 10
8
6.67 × 10
-2
0.465
d
3.84 × 10
8
3.68 × 10
-2
0.257
d
1.496 × 10
11
14.3 100
Notice that the units—meters in this case—cancel, leaving a dimensionless number for the scale
factor.
We can do the same for our second attempt at a scale factor, s
2
, using the largest distance
(d
@
) scaled to 100 m:
d
@
s
2
D 100 m (1.24)
s
2
D
100 m
d
@
(1.25)
s
2
D
100
m
1:496 ˆ 10
11
m
(1.26)
s
2
D 6:68 ˆ 10
´10
: (1.27)
We can now multiply these two scale factors by our data to see what we get. e results
can be seen in Table 1.2. Notice that the first scale factor puts the radius of the Moon at half
the width of a grain of salt, and the second scale factor puts the distance to the Sun at 100 m.
e second scale model is bigger than the first, because our second scale factor was larger.
And so which of the two scale models is the most useful? e first puts the Earth–Sun
distance at an easily-manageable 14.3 m—a distance of about 20 steps (assuming a typical step
length of 0.7 m)—and the Moon is nicely placed at 3:68 cm from Earth. e Sun is a little over
13 cm, about the size of a large grapefruit. By design, our scaled Moon is the size of a grain of
salt. Earth, then would be about 1:2 mm across—still very tiny. For an appropriately scaled Earth
for this model, one could purchase a size 16/0 glass seed bead, which is about this size.
7
And so
our scale model of the Earth–Moon–Sun system is a tiny glass bead for Earth, a grain-of-salt
Moon 3:68 cm away, and a grapefruit Sun some 20 steps distant.
For our second scale model, the Moon and Earth are larger and more manageable in size—
2:3 mm across for the Moon and 8:5 mm for Earth. It would be a rather simple and enjoyable
task to wander around with a millimeter scale ruler, looking for suitable Earths and Moons
7
ey are, however, only sold in packs of many hundreds, and that is a lot of extra Earths to give to friends as birthday
presents.

1.3. SCALING AND SCALE MODELS 17
for this scale model. Finding a nearly 1-m diameter ball for the Sun might be somewhat more
difficult. And clearly this model would need more physical space to set up; the scaled Moon is
about 10 in from Earth, but the Sun is a full 100 m distant.
is simple example of a scale model is an easy one because the full range of sizes and
distances is rather limited. But what if one wanted to include not only Earth, Moon, and Sun,
but also Jupiter, Saturn, Uranus, and Neptune? Or what if one were to draw appropriately-sized
craters onto our scaled Moon, or include the star Alpha Centauri in our model? is is where
the hard choices come to play, because the scale model can easily extend beyond the range of
our direct human experience, thus missing the point of making a scale model in the first place.
Clearly, a powerful microscope would be needed to see scaled-down craters on our grain-of-salt
Moon. And even the nearest star is very far away indeed, even when scaled down so much that
the Moon is, by comparison, only a grain of salt.
One nice thing about a scale model is that angular sizes are preserved. Consider the first
scale model described in Table 1.2; a grapefruit-sized Sun is placed 14:3 m from Earth. We could
imagine shrinking ourselves down onto this tiny Earth, and from that vantage point looking at
the grapefruit Sun.
8
How big would our scale-model Sun appear? at is to say, what is the
angular size of a 13.3-cm diameter grapefruit, when viewed from a distance of 14.3 m? We
calculate the answer with the small angle formula:
D
D
0:133 m
14:3 m
(1.28)
D
D 9:3 ˆ 10
´3
radians (1.29)
D
D 0:53
˝
: (1.30)
And so we see that when viewed from the scale-model Earth, the grapefruit sun appears exactly
the same size as does the real Sun in the real sky. is result holds in general for scale models.
Since angular sizes are ratios of lengths, the angular sizes of objects in a scale model are the same
as in real life.
1.3.1 SCALING RATIOS
Any particular scale model is only useful to portray a limited chunk of the universe. We can,
however, use different scale models for different parts of the universe, and find ways to put them
together so as to compare them to each other. A starting point for this task is to calculate simple
comparisons, in the form of numerical ratios, of chosen pairs of sizes and distances. ese ratios
are the same whether one uses the physical numbers or their scale-model versions. And so for
example, one can easily calculate from the numbers in Table 1.2 that Earth has a radius 3.67
times greater than that of the Moon. is is true in real life, but it is also true for any (correctly
made) scale model of the Earth–Moon system.
8
It is generally considered safe to look at a grapefruit without eye protection.
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
