Process control in ring and rotor spinning
Abstract:
Control of process parameters is extremely important in ring and rotor spinning to ensure production of yarns with the desired quality at the highest possible speed and with minimum waste. The chapter discusses the importance of different key process parameters and their influence on process performance and quality of yarns. It also discusses the effect of different factors on those key process parameters.
8.1 Introduction
Ring and rotor spinning are the final stages of yarn formation. Any defect in processing in this final stage may lead to the formation of defective yarns and also higher end breakage. It is therefore extremely important to keep the spinning process under control to deliver yarns with the desired quality at the highest possible speed and with minimum waste. The key parameters which need to be under control for both ring and rotor spinning are:
Effective control of these parameters will ensure both yarn quality and the productivity and economy of the spinning process.
8.1.1 Understanding spinning tension in ring spinning
Control of spinning tension on the ring frame is extremely important in controlling yarn characteristics as well as spinning performance. If tension is too high or too low, it will cause higher end breakage leading to poor productivity, higher wastage and ultimately making the process uneconomical. It is important to understand both the theory involved and the practical issues involved in controlling spinning tension.
Tension in different zones
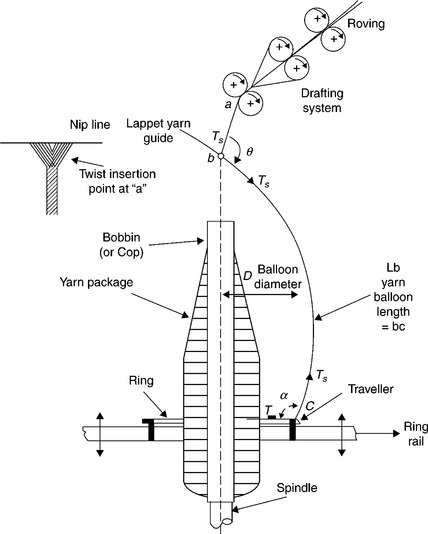
Figure 8.1 illustrates a typical ring spinning system. In ring spinning, the traveller and the balloon length, Lb, must move around a common axis in order to wind the yarn onto the spinning bobbin. Work must therefore be performed against the frictional force of the ring on the traveller, the traveller on the yarn, and against the air drag on the traveller and balloon length. The combination of these forces causes tension in the yarn. This work is additional to the effort needed to overcome the friction of the spindle bearings and air drag on the forming yarn package.
Tension develops in the yarn in three zones:
In the winding zone, the length of yarn between traveller and forming package develops winding tension, TW. In the balloon zone, tension occurs between the traveller and lappet guide (often referred to as the balloon tension). This tension, at a given point on the balloon length, varies with amplitude (i.e. the radius of the point) measured from the common axis. The yarn formation zone is the area between the pigtail lappet guide and the front rollers of the drafting system, where yarn tension is referred to as spinning tension, TS.
Theoretical models of ring spinning
A proper understanding of the theory of ring spinning is essential for effective control of the process. The yarn rotates around the inner circumference of the lappet guide at almost the same speed as the traveller. The radius of the lappet guide is sufficiently small for any central forces generated to be inconsequential. The motion of yarn between the lappet and front drafting rollers is therefore principally related to the velocity along its length, that is the thread line velocity. Consequently, the forces of interest are the air drag along the yarn length, the tension at the lappet guide, and the resistance to bending around the guide. The air drag is proportional to the square of the thread line velocity, but this velocity is usually small in comparison to the rotational velocity of the yarn. The force caused by air drag along the yarn length is thus assumed to be negligible. The bending resistance, due to the flexural rigidity, of the yarn is many times smaller than T0 and requires no further consideration. T0 is therefore the only effective force governing Ts and, as a result, analysis of the forces present in ring spinning is usually concerned with the remaining two zones.
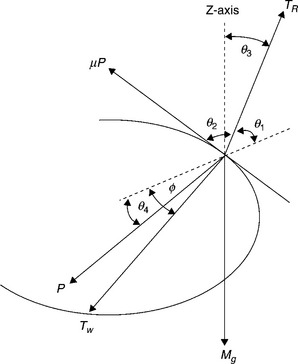
In steady running conditions, the traveller presses against the bottom of the internal flange of the ring. The forces acting on the traveller at the point of contact with the ring are shown in Fig. 8.2, where
C = centripetal force [MR ω2] needed to keep the traveller circulating around the ring
μ = friction coefficient between ring and traveller
η = the yarn-lappet coefficient of friction
θ and α = the angles shown in Fig. 8.1
P = reaction force of the ring and traveller
m = angular velocity of the traveller
θ1 …. θ4 and ϕ = angles indicated in Fig. 8.2
T0, TR = the tensions in the balloon length at the lappet guide and at the ring and traveller, respectively
Strictly, Tw is not the true winding tension. This is because centripetal, Coriolis, and air-drag forces act on the mass of the yarn length from the traveller to the ring bobbin. It can be reasoned that the latter two effects negate each other and are therefore of little relevance. The effect of the centripetal force is to change the path of the yarn; rather than forming a tangent from the package to the traveller, it becomes a curve. The change is a minor one, however, and for the sake of simplicity this centripetal force can also be ignored.
The causes of tension in the balloon zone relate to the forces acting on each elemental length, where r denotes the distance of an element of length from the z-axis. The forces present are as follows:
• centripetal and Coriolis forces associated with yarn motion,
• air-drag forces opposing yarn motion,
• the weight of the element acting vertically downward,
• the resistance to bending due to the flexural rigidity of the element.
Early references to the theory of ring spinning can be found in papers by Ludicke1 in 1881 and by Escher2 in 1883. The theory has since been augmented and developed by various researchers3−14 and, based on these analyses, DeBarr and Catlingi 15 published a monograph on the subject in 1965. Nerli16 attempted to develop a computer-based analysis for calculating forces acting on the balloon and the balloon shape, but this attempt was limited by its dimensional formulation. Batra17−19 summarised theoretical contributions to ring spinning theory and introduced numerical solutions for steady-state balloon shapes.
Lisini20 proposed a non-stationary mathematical model of the ring spinning process. Fraser21, 22 investigated the characteristics of air drag, such as the effects of air drag magnitude on balloon shape, tension and the bifurcation behaviours of the solution. Stump23 developed transient solutions of the nonlinear time dependent partial differential equations and studied the stability of representative quasi-stationary balloons. Zhu24, 25 studied the steady-state response and stability of ballooning strings under the influence of air drag and used the Hopf-like bifurcation to predict the limit cycles of ballooning strings. Fan26 calculated the natural frequencies and mode shapes of a single loop balloon. Tang27−29 estimated the air-drag coefficients on ballooning cotton and wool yarns. Yarn tension and balloon shape were obtained by experimental data.
Rong Yin and Hong-bo Gu30 established a more realistic model by incorporating yarn as a linear elastic component, which has been simplified by most investigators. The equations of motion were derived by following Fraser’s approach,31 and numerical solutions to these equations for small elastic parameter values presented. MATLAB software can be used to develop a program corresponding to the model. The effect of yarn elasticity in a quasi-stationary balloon has been investigated. This model can be used to simulate balloon shape and predict yarn tension under given spinning conditions for a small strain value, and to facilitate further research into reducing yarn breakage in ring spinning.
8.2 Factors affecting spinning tension in ring spinning
This section covers the effect of spindle speed, traveller mass, traveller/ring friction, ring diameter and balloon height.
8.2.1 The effect of spindle speed
Yarn tensions are directly proportional to the square of the traveller or spindle speed. When the spindle speed is increased, the friction work between ring and traveller (and hence the build-up) increases as the 3rd power revolutions-per-minute of the spindle. If the spindle speed is too high then the traveller sustains thermal damage and fails. This speed restriction is felt particularly when spinning high-strength cotton yarns. When spinning 100% cotton, fibre dust from the cotton acts as a lubricant, but not all cottons generate the same amount of lubricating film. Traveller speed is not a limiting factor in cotton combed yarn intended for knitting. Because the yarn TPI is lower, the yarn strand is not strong enough, and so the yarn tension becomes the limiting factor. Yarn strength is not a problem for polyester/ cotton blends and cotton weaving counts, and the limiting factor is traveller speed. For a ring diameter of 40 mm, spindle speeds of up to 19 500 rpm should not be a problem. Rings like Titan (from Braecker), NCN (bergosesia), etc. are able to meet the requirements.
For spindle speeds greater than 20 000 rpm, ORBIT rings or SU-RINGS should be used. Because the area of contact is larger with these rings, with higher speeds and pressure, the heat produced can be easily dissipated. Normal rings and traveller profiles cannot produce good quality yarn if run at speeds greater than 20 000 rpm.
ORBIT rings are very useful in working 100% polyester at high spindle speeds, where high tension generates a lot of heat between the ring and the traveller. Because the yarn strength of polyester is very high, heat dissipation is the only limiting factor. ORBIT rings with higher area of contact will be able to run well at higher spindle speeds.
The maximum spindle speed for a given yarn tension, or the minimum tension for a given spindle speed, can be determined using the following equation32:
where n is the traveller speed in thousands of revolutions-per-minute, T0 is in g-wt, N is the count of the yarn in tex, and H is the balloon height in cm.
A single stable balloon cannot form if the tension is less than the value given in the equations. If, at a given spindle speed, the tension is reduced or the balloon height is increased, at a certain point the balloon collapses and forms a neck. The spinning tension decreases as the diameter of the package increases during the build of a bobbin, so balloon collapse is most likely to occur when the yarn is winding onto the full diameter of the package. Collapse is more likely with longer balloons, even though tension increases with balloon height.
The effect of spindle speed on yarn properties has been observed by many researchers. Anbarasan33 reported that the benefits of advanced speed systems were subsequently extended by the introduction of variable speed systems such as the inverter drive system. This system offers the possibility of selective and continuous speed adjustment for the complete cop build. Optimum spindle speeds can thereby be achieved so that an almost constant spinning tension is maintained throughout the cop build.
8.2.2 The effect of traveller mass
Winding tension increases with traveller mass, and heavier yarns require a greater centripetal force to keep them rotating. Traveller mass is used as a variable to increase tension and generate higher centripetal force for heavier yarns. The traveller mass is usually chosen according to the linear density of the yarn being spun. In fact, all the implications for winding tension apply to tension in the yarn forming zone.
Traveller mass controls winding tension, which in turn produces tension in the lower balloon at the other side of the traveller. Because of yarn/traveller friction, the balloon tension is rather less than half the winding tension. The balloon tension in turn gives rise to the spinning tension between the lappet and the front roller nip; this depends on the winding tension and on the centrifugal force and air drag on the balloon. Within practical limits the coefficient of traveller/ring friction varies inversely with traveller mass, directly with ring diameter, and increases to a maximum at a particular rotational speed.
Heavier travellers are generally used for thicker counts, and the maximum traveller mass consistent with a good spin is chosen, to give an acceptable end breakage rate. As a last resort it may be necessary to reduce the spindle speed in order to control the end breakage rate.
The minimum traveller mass is determined by the need to avoid balloon collapse when winding onto the maximum package diameter and maximum balloon length. The maximum mass is limited by the need to avoid excessive tension, which causes a high end breakage rate. A range of traveller masses can be employed for a given set of conditions, and this range becomes narrower as the spindle speed increases.
The minimum tension required to avoid balloon collapse is proportional to (spindle speed)2, and the tension produced by a given traveller is proportional to f(spindle speed)2, where f is a coefficient that depends on the ring/traveller coefficient of friction, yarn/traveller coefficient of friction, and the angle at which yarn is wrapped around the traveller. The minimum traveller mass would be independent of spindle speed if f remained constant but in practice, although f is constant at low spindle speeds, it decreases at higher speeds. A slightly greater mass is therefore required at higher spindle speeds.
Minimum traveller mass is proportional to the yarn count and to (balloon height)2, but is inversely proportional to ring diameter. If the traveller is too light then the balloon diameter becomes excessive, causing the yarn to beat against the separators. It can also cause traveller ‘flutter’ or ‘chatter’, a vibration with audible impacts between the traveller and the ring, which results in higher peak tensions and increased end breakages as well as traveller and ring wear.
If the traveller mass is greater than the minimum then a firmer package of increased capacity can be produced, and the twist differential across the traveller increased. This may increase yarn strength by parallelising the fibres as they are gathered from the delivery roller nip, and may reduce the end-breakage rate, though a heavier traveller will also increase power consumption.
It is generally assumed that in cotton spinning the tension ratio (mean individual thread breaking load)/(mean spinning tension) must not be less than 14 in order to achieve an acceptable end-breakage rate. Higher tensions with ratios of 4 or 5 are acceptable for polyester/cotton blends, and a ratio of 12 for worsted spinning. Yarn emerging from the front roller nip is only partially twisted and its strength may be only about one-third of the ultimate individual thread breaking load.
The minimum traveller mass for a particular ring frame is that which is just sufficient to prevent balloon collapse during winding on the shoulder of the cop bottom, that is, during winding at the maximum balloon height onto the maximum package diameter. Since both the minimum balloon tension and the traveller-ring frictional force are approximately proportional to the square of the spindle speed, the mass of the lightest traveller that can be used is largely independent of the spindle speed, n. The minimum mass is, however:
where 2R and H are in inches and N is in tex. An approximate value of the minimum traveller weight, M, in grammes is given by the expression
8.2.3 The effect of traveller/ring friction
Rings and travellers are the dominant elements in the ring spinning process. The traveller has, among other duties, the function of regulating the spinning tension. The spinning tension must be high enough to keep the thread balloon stable, but not so high as to cause yarn breakage. Winding tension increases with the frictional coefficient between the ring and the traveller (μRT). It should be noted that the spinning tension in the yarn is directly related to winding tension, so any factor that increases winding tension will also increase tension in the yarn during ring spinning.
Reducing the friction coefficient to the lowest possible level is key to the success of ring spinning. At this point perfectly balanced spinning geometry will have been achieved, meaning that the spinning tension is on a constant balanced level. Friction will be reduced if the rings and travellers establish a symbiosis. The ring/traveller system in short staple spinning operates dry, but at high speeds normal friction systems only work with additional lubricants. The fibres protruding from the yarn body between ring and traveller are crushed and form a steadily regenerating lubricating film in a process known as fibre lubrication. The resulting friction coefficient varies according to the fibre, which may be either dry, wax-containing cotton, or synthetic with added softening agents. The coefficient of friction (μ) can vary in extreme cases from 0.08 to 0.12, explaining why different traveller weights must sometimes be applied in otherwise identical spinning conditions. In order to maintain the same friction or spinning tension with different coefficients of friction, different traveller weights must be used. Dry cotton has a high friction coefficient, while manmade fibres have low to medium coefficients of friction, depending upon the manufacturer.
It has been shown by Catling34 that the effective coefficient of friction between the ring and the traveller is a very important factor in the balloon stability equation, and largely determines the yarn tension. It was found that traveller speeds are, in practice, limited by the fact that at high speeds an abrupt and unpredictable increase in the friction coefficient is apt to occur. In some circumstances this may merely increase the winding tension (and thereby the number of thread breakages) but more usually the increase in friction is accompanied by overheating of the traveller and very rapid wear of both ring and traveller.
8.2.4 The effect of ring diameter
Package capacity is approximately proportional to (ring diameter)2, so a large diameter ring may be thought desirable, but increasing the ring diameter also increases yarn tension. When thicker counts are being spun, frames with larger rings are fitted because the yarn is strong enough to withstand the increased tension and a larger package size is desirable. However, the ring diameter is restricted by yarn tension and the minimum angle of lead, as well as other factors such as power consumption, spindle rpm, and traveller speed limitations.
This is why we do not see ring spinning machines with very large rings and very small bobbins (tubes). Large ring radii and yarn packages are used for coarser and stronger yarns, but for finer yarns both the ring radius and package size must be smaller. Most worsted yarns are spun using rings between 45 and 75 mm in diameter at spindle rotational speeds between 7000 and 12 000 rpm. Short staple yarns are spun with smaller rings, but at about twice the spindle speeds used for worsted yarns.
8.2.5 The effect of balloon height
The balloon does not behave exactly like a tensioned string in ring spinning, because of its relatively large radius and the influence of traveller and ring/ traveller friction. A stable balloon system can be formed for many combinations of balloon height (H), ring radius (R), package radius (R1), spindle speed, yarn count and yarn tension. This is because the length of yarn forming the balloon is not fixed even though the axial balloon height is fixed; the yarn length is self-adjusting so that the balloon shape permits the new horizontal force on each element in the yarn to provide the necessary centripetal force on that element.
For a given value of R and h there is a limiting value of P (as defined in 8.2.1) for which a node can be formed. The limiting value of P decreases as the ratio R/H or R1/H increases; the maximum theoretical value of H/P is π for a ring of infinitely small radius.
To avoid neck formation R/P and H/P must be small and so P must be large. For a given yarn count, this means that either T0 must be large or ω must be small, both of which are undesirable.
A neck will gradually form in the balloon if unfavourable conditions arise, such as increase in H or a reduction in traveller mass. Balloon collapse occurs when the neck makes significant contact with the package, thereby causing a breakdown in the spinning process. Collapse is most likely to occur when yarn is winding onto the maximum package diameter with the longest balloon length, that is, 58 when H is at a maximum and P is at a minimum.
By restricting the maximum balloon radius, and thereby reducing air drag on the balloon, control rings allow the creation of a longer balloon without a neck, and with a much lower yarn tension than would otherwise be possible. Each section of the total balloon then has a smaller value of H. H/P is thus effectively reduced and balloon collapse avoided even though the overall balloon length has increased. By stabilising tension (T0), the benefits from the use of control rings permit larger package length, higher spindle speeds and reduced power consumption.
Yarn tension increases as normalised balloon height increases, particularly when the height is greater than 10 cm. When the ratio of yarn-length-in-balloon to balloon height is greater than 1.2, the yarn tension has a ‘lower’ value and the effect of balloon height on the tension is very weak.
The influence of balloon control rings is quite considerable, especially at long cops. A reduction of the yarn balloon is advantageous and may even be a prerequisite for optimum performance. If balloon control rings are mounted at the correct distance, with the yarn balloon restricted as long as possible during one lift of the ring rail, then a marked increase in performance is possible. Balloon control rings are used to contain the yarn-loop, by reducing the yarn tension and decreasing the balloon flutter instability.35 Flutter instability here refers to uncontrolled changes in a ballooning yarn under dynamic forces, including the air drag. Due to the significant variation in balloon length and radius during the bobbin filling process, the optimal location for the balloon control ring is difficult to pinpoint. In order to address this difficulty, this study35 investigates the variation in the radius of a free balloon, and examines the effect of balloon control rings of various diameters at different locations on yarn tension and balloon flutter stability. The results indicate that the maximum radius of a free balloon and its corresponding position depend not only on the yarn length to balloon height ratio, but also on yarn type and count.
A control ring of suitable radius and position can significantly reduce yarn tension and decrease flutter instability of free single loop balloons. The balloon control rings are usually fixed to, and move in sync with, the ring rail. However, the reported results suggest that, theoretically, a balloon control ring that always remains approximately half way between the yarn-guide and the ring rail during spinning could lead to significant reduction in yarn tension.
8.3 Control of end breakage rate in ring spinning
The end breakage is a critical spinning parameter that not only affects the maximum spindle speed but also indicates the quality of yarn, the mechanical condition of the machines and the quality of raw material. Therefore, control of end breakage rate is the prime requirement for getting better ring frame performance and for achieving higher spindle speed.
8.3.1 Theoretical models
The problem of yarn breakage during spinning is serious and difficult to solve due to the complexity of the breakage mechanisms. Yarn breakage phenomena are complicated because the causes are numerous, including the influences of humidity and temperature, but also because the tension and strength of the yarn always vary. In practice, almost all yarn breakages occur at the exit of the front roller, probably because, at that point, the yarn has not yet been twisted and is still weak. Hori36 has used simple mathematical models of yarn tension and strength variation to calculate the probability of breakages. He concluded that:
1) Variations in yarn strength and tension, and in the average strength and tension, influence the number of the yarn breakages.
2) Above all, the variations in yarn strength are most influential under normal conditions.
Evenness of the yarn is thus more important than lower tension on spinning frames in reducing yarn breakages.
De Barr37 also discussed the problem of end breakage in ring spinning in terms of a simple mathematical model. Absolute values of end breakage rate could not be calculated, but the treatment enabled the relative importance of such factors as tension, regularity of yarn, angle of lead, etc., to be assessed. The results, some of which would be applicable to any process in which yarn breakages are involved, show that the most important factor affecting end breakage rates is yarn regularity.
The method of calculating end breakage rates was based upon that described by Hori,36 but the model used was more realistic, and the treatment extended so as to enable the effect of changes in, for example, angle of lead, to be investigated. The variation of TT/C with angle of lead, α, has been used to calculate the variation of end breakage rate with angle of lead.
From the parameters of the strength and tension distributions, the probability, p, that in any interval, ZS, the mean tension mT1, being greater than the mean strength mS, was calculated by the following equation:
Ghosh et al.38 proposed a statistical model for predicting the end breakage rate based on the following assumptions:
I The yarn is composed of a successive chain of links with different lengths.
II An end break occurs when the spinning tension exceeds the strength of the yarn in the spinning zone.
III The strengths of the links of fibre bundle just issuing from the front rollers are independent variables and follow a normal distribution, that is, a link of high strength may immediately follow one of low strength. The strength is constant within a link but varies from link to link. The strength of a link depends on the number of fibres in the link and the coefficient of variation of the strength of all the links. The coefficient of variation of the strength of the links is equal to the coefficient of variation of mass per 8 mm length of the yarn (Uster CV%). This is based on the fact that the variance-length curves of fibre assemblies run practically horizontal for cut lengths between 1 and 10 mm.39
IV The strength of the yarn in the spinning zone is about one-third of the mean strength of the yarn.
The probability that the tension exceeded the strength of the links just passing from the front roller's nip was found by the relationship
where Z is the standard normal deviation and Φ(Z) can be expressed
8.3.2 Measuring the end breakage rate
Accurate measurement of the end breakage rate can be used in determining operatives’ work allocation, and provide valuable data for quality-control purposes.15 Unfortunately, end breakage rates are particularly difficult to measure with enough accuracy for the results to be useful. Not only are there systematic variations between apparently similar frames at the same time, and for the same frames at different times, but there are also many chance variations resulting from imperfectly understood and randomly occurring phenomena. Useful results can, however, be obtained if care is taken, and the procedure outlined below will be found adequate where the major systematic variations have been eliminated.
Observation of all end breakages occurring on one side of one ring frame during the build of a complete bobbin may be regarded as a 'unit breakage test'. It is usually convenient for one observer to make several unit tests concurrently by observing all the sides attended by one operative, but it is not practicable to extend this by watching more than one operative at a time. Ends gaited after doffing, and those accidentally gaited by the spinner, should be ignored. Multiple breaks on adjacent spindles often have a single cause and should therefore be noted separately.
To reveal trends with time of day, ambient conditions, part of building cycle, etc., records should be made in consecutive constant time intervals of, say, 15 minutes, and ambient atmospheric conditions should be recorded at not less than hourly intervals. After each complete build-cycle, the results should be summarised for each unit test. Trends relative to ambient conditions or stage of build may be revealed by plotting the mean rate for a number of unit tests during consecutive 15 min intervals.
Before making use of the observations, however, it is desirable to determine the reliability of the results in view of chance variations that inevitably occur. Clearly, the greater the number of unit tests comprising the series of observations, the more reliable the results. However, a quantitative procedure is required for determining the number of unit tests needed to ensure that the average is within a particular range of the true breakage rate for each set of conditions.
Suppose the end breakage rates obtained from h unit tests are b1, b2, b3,…, bh; then the average rate is
and the mean of the squares is
is determined, the true breakage rate lies within the range B – 2E and B + 2E, and the number of unit tests required to give a breakage rate reliable to ± q% is given by
8.4 Factors affecting end breakage rates in ring spinning
The mechanism of end breakage in ring spinning is a complex phenomenon involving different factors. The major factors influencing end breakage in ring spinning are yarn strength in relation to mean value of peak spinning tensions, yarn irregularity, yarn twist and relative humidity.
8.4.1 The effects of spinning tension and yarn strength
Most end breaks occur close to the front roller nip, where the fibres are being twisted, and so the strength and tension in this region are of the greatest importance. It is important to note that increasing the spinning tension does not necessarily increase the end breakage rate, because the corresponding increase in twist density in the balloon leads to an increase in yarn strength in the critical nip zone which, in some circumstances, may be sufficient to reduce the end breakage rate. The effect is particularly noticeable when spinning tension is increased without any increase in spinning speeds. The balloon diameter is reduced, and thus the angle at which yarn is wrapped around the traveller is increased. As a result, both the twist differential and the tension differential across the traveller are increased, and, over a substantial range, the end breakage rate is reduced by increasing the spinning tension.
At high speeds and with large packages, however, the interaction of these factors gives rise to operating difficulties. To avoid balloon collapse, limiting spinning speed and use of proper traveller weight are extremely important while winding on the largest diameter and with the largest balloon, that is, just on completion of the cop bottom, particularly when spinning is carried out without a balloon control ring.
At this point in the build, when the balloon is at its largest and the air drag (and therefore the balloon tension) is maximised, the angle of wrap of the yarn round the traveller is minimised, so that both the tension and the twist differentials across the traveller are very small. Maximum tension is transmitted to the nip zone at a time when minimum twist potential is available for consolidation of the newly formed yarn, and consequently end breakages are common.
8.4.2 The effect of yarn irregularities
The weakest part of a forming yarn, where most end breakages occur during ring spinning, is at the point of twist insertion in the spinning triangle. Three factors are therefore of importance40:
(1) The number of fibres in the triangle and the variation of this number
Clearly, where there are a large number of fibres in the cross section of the forming yarn, the yarn will be more able to withstand the spinning tension and tension fluctuations, provided that the mean spinning tension is kept well below the breaking load of the yarn (typically 30% below mean yarn strength). End breakage problems will arise when the number of fibres in the cross section of the fibre ribbon varies significantly and/or the peak value of tension fluctuation is too high. The variation of the number of fibres in the cross section causes thin and thick places in the fibre ribbon. As these pass through the twist insertion point at the apex of the spinning triangle, the thin places are more easily twisted than thick places, so thin parts of the ribbon tend to have more twist than thicker parts. If a very thin part of the ribbon becomes over twisted and weak, the yarn will be susceptible to peak tension fluctuations.
It is a well-established fact that yarn irregularity cannot be eliminated even under the best processing conditions. But there are many conditions that increase the variability of lengths of yarn, and which may lead to excessive spinning breakage rates.
The importance of irregularities in yarn strength in determining the end breakage rate has been noted by Hori,36 and it is clear from findings by De Barr37 that the end breakage rate is more sensitive to variations in irregularity of yarn strength than to any other factor. The effect is so marked that the range between the lowest attainable irregularity and the highest irregularity that can be tolerated is very small.
8.4.3 The effect of twist in the yarn
Most end breaks in ring spinning occur in the material just issuing from the rollers, and the end breakage rate is very dependent upon the twist in the yarn between thread-guide and the roller nip.41 The twist in this zone is always be greater than the equilibrium twist in the yarn on the package, so that the strength of the yarn here is normally greater than might be expected from the known spindle and delivery speeds. Furthermore, although the twist in the yarn in this zone decreases with the twist factor, this reduction will be less than the decrease in twist in the yarn on the package. The end breakage rate in soft-twisted yarns should not be as much greater than that in hard-twisted yarns as might otherwise have been expected. Indeed, the increased twist in the roller nip – thread-guide zone may well be very important in the spinning of soft-twisted yarns.
A reduction in end breakage rate can be achieved by using a traveller that presents a considerable obstacle to the passage of twist across it, but this reduction may be at the expense of yarn quality.
The angle at which yarn is wrapped around the thread-guide is also a factor determining the amount of twist in the yarn issuing from the roller nip. In the usual arrangement, the thread-guide is positioned substantially on the axis of rotation of the spindle, and the spindle-roller geometry is such that the yarn from the roller nip to the thread-guide makes an angle of about 30° with the spindle-axis. This suggests an average angle of wrap of 30° but, under running conditions, the actual angle of wrap will vary cyclically during each revolution of the balloon from 30° + θ0 to 30° – θ0.θ0 is the angle of inclination to the spindle-axis of a yarn element leaving the thread-guide and entering the balloon.
Under most spinning conditions, the instantaneous angle of wrap becomes negligibly small once per revolution and there is effectively a direct path for twist and tension to be transmitted from the yarn balloon to the nip zone. In a system known as vertical spinning, the roller nip is directly above the thread-guide, so that the angle of wrap of the yarn around the thread-guide does not vary appreciably during each revolution of the balloon. It might be expected that the difference between normal and vertical spinning would greatly influence the end breakage rate, but this does not seem to be the case. It may therefore be inferred that the capstan (or angle-of-wrap effect) of the passage of the yarn over the thread-guide is equally effective as a twist barrier and as a tension barrier.
8.4.4 The effect of temperature and humidity
The temperature and humidity of the atmosphere in the mill are important factors in controlling breakage rate.37 While no published data relating to the effect of temperature is available, common experience suggests that low temperatures should be avoided. Some research into humidity has been carried out at the Shirley Institute,37 where it was found that effects in the card room and spinning room were in one respect dissimilar. In the card room it was found that very low humidity, such as is experienced in frosty weather, led to excessive drafting irregularity, especially at the draw frame, which would give rise to higher spinning breakage rates. In the spinning room the breakage rates per l00 spindles per hour were 18, 29 and 28 for percentage relative humidity values of 20, 45 and 65, indicating that very low humidity may be beneficial in reducing spinning breakage rates.
8.5 Control of fly generation and twist variations in ring spinning
Fly generation and twist variations are the two disturbing but unavoidable occurrences in ring spinning. Since it is impossible to prevent the fly from being released and avoid twist variation as it is inherent to the twisting mechanism, exercising control over them will ensure trouble free spinning.
8.5.1 The causes of fly generation
In conventional spinning, it is impossible to collect all the fibres emerging from the front rollers into the forming yarn. Some fibres escape as they emerge from the front rollers, while others are thrown out later by centrifugal acceleration. Many short fibres are lost as fly during the processing of staple fibres on spinning machines, and a considerable quantity of fibre debris and dust is released. Fly and dust are deposited on machine components or are continuously being whipped up and around by rotating and circulating devices such as spindles, drums and drive wheels. They have therefore always caused significant disturbance to service and maintenance, as well as diminishing quality. This problem has intensified further with high production speeds and high drafts. In ring spinning machines, the most fly and dust are released in the main drafting zone and the spinning triangle (up to 85%), while the balloon and travellers account for most of the remainder.
8.5.2 The factors affecting fly generation
For exercising better control over fly generation it is essential to know about the influencing factors. While drafting zone and the spinning triangle are the major sources of fly generation, relative humidity also plays a major role to control it.
The effect of the spinning triangle
During ring spinning, the fibres on the two edges of the spinning triangle must be strongly deflected so that they are bound into the yarn at the convergence point. The deflection is higher with a smaller triangle. Not all fibres are bound into the yarn, particularly those with high rigidity and low cohesion with neighbouring fibres. The end breakage rate also influences the waste and fly. The spinning triangle and spinning angle significantly affect the yarn breakage, because the long and narrow width implies a long weak point and hence causes more end breakages. However, a resulting advantage of the small triangle width is that the edge fibres are better bound in the yarn, which gives smoother (less hairy) yarns and hence less fly generation. According to Klein,42 when the spinning triangle is short, the fibres from the edges must be strongly deflected if they are to be bound into the yarn structure. The quantity of fly waste can be minimised by ensuring that the fibres emerge from the delivery rollers in as narrow a ribbon as possible, and spinning with higher drafts from thicker rovings tends to increase the amount of fly waste.43 With the development of compact spinning systems, where the spinning triangle is practically eliminated, the problem of fly generation is greatly reduced.
The effect of humidity
Atmospheric conditions influence the rate at which machines can be worked. In a very dry atmosphere, the electrical charges generated by friction, if not conducted away, make controlling the yarn more difficult. Dry conditions create static electricity, and unfavourable fibre repulsions occur, causing the fibres to stray from the normal passage. Low humidity also causes fibres to be stiff, resulting in higher fly generation. Correct relative humidity (RH) ensures that yarn can be worked with minimal risk of breakage, reduces static electricity due to the greater electrical conductivity of the yarn, and lowers the amount of fly liberated. If the humidity is too high, static electricity will be doused, causing stickiness. The fibre mass clings together firmly, which makes uniform drafting difficult along with roller laps and piecing conditions. Generally 55–60% RH is maintained for coarser counts (20–40s Ne). For finer counts (60–80 s Ne) a slightly lower range of 50-55%, is maintained.
8.5.3 Mechanisms of twist propagation
Although twist is actually inserted into the region between the thread-guide and the traveller, the yarn between the front roller nip and the thread-guide, and that wound onto the package, is also twisted. Twist must therefore pass both the thread-guide and the traveller. There is, however, an important difference in that the twist passes the traveller in the same direction as the yarn, while across the thread-guide the yarn and twist are moving in opposite directions.
The thread-guide and traveller offer frictional resistance to the passage of yarn, and the tension in the yarn on the downstream side of either must be greater than on the upstream side. In a similar way, the thread-guide and traveller offer resistance to the passage of twist, and the torsional stress in the yarn on the downstream side (in the direction of twist propagation) must be less than on the upstream side. The twist (turns per inch, say) in the yarn in the balloon must therefore be greater than that in the yarn between front roller and thread-guide, or in the yarn as it is wound onto the package. Under steady-state conditions, however, the rate at which the twist passes the traveller must be equal to the rate at which twist is inserted in the balloon zone. It follows that the twist in the yarn in the balloon must be greater than calculated.
The distribution of twist between front roller nip and package was studied by DeBarr and Catling.44 Grandrelle or mock grandrelle yarns were photographed during spinning using the technique described by Axson,45 and the observations confirmed the above predictions. The conclusion of De Barr and Catling does not seem to have been substantiated by direct measurement of twist in the roller nip – thread-guide zone.
Kanai and others46 obtained data on twist in the roller nip–thread-guide zone by direct measurement and concluded that the turns per inch of a yarn between the nip point of rollers and the traveller has been shown to exceed that of a yarn on the bobbin. The twist density of a yarn above the snail wire has been found to be 15% greater than in the yarn below it. On the other hand, Wegener and Landwehrkamp47 found that there was a reduction of about 22% in the twist at the balloon control ring, and another reduction of about 19% at the thread guide. In worsted spinning, Gessner48 found that without a balloon control ring, the twist in the balloon yarn is 123% and 107% below and above the control ring, respectively, and the twist in the roller nip – thread-guide yarn is 78% of the bobbin yarn twist, contradicting the findings of De Barr and Catling. Wegener and Landwehrkamp did not, however, mention whether these results applied to all stages of the doff and to the entire chase cycle. Subramanian and others49 used a simple method for measuring the twist density in the yarn between the roller nip and the thread-guide of a ring spinning frame, which was found to be reliable in comparison to results obtained by high speed photography. The method consisted of plucking the yarn passing between the roller nip and thread guide with the fingers and counting the turns.
Variations in twist flow over a doff
As the yarn package builds up, the traveller adjusts its rotational speed automatically, leading to variation in the twist level within yarn packages. However, this variation is very small and, taking into account the twist added during unwinding, the effect of traveller speed change (as cop buildup) is practically insignificant.
The variation in twist density over a doff has been studied by Subramanian and others,49 by taking a large number of readings from the start to the finish of the doff with the ring rail alternately at the nose and at the shoulder of the chase. The twist in the spinning zone is seen to be, on the whole, higher than the mechanical twist when the ring rail is at the nose of the chase, and always lower than the mechanical twist when the ring rail is at the shoulder of the chase.
Variations in twist flow within a chase
With conventional ring spinning frames, the balloon height and the length of yarn in the balloon vary cyclically according to the type of build being used. Since the twist in the yarn in balloon is greater than that in the yarn wound onto the package, if the length of the yarn in the balloon is changing, the twist in the yarn being wound onto the package is also changing. The magnitude of this twist variation is not dependent upon the change in balloon height but upon the rate of change.
With a roving built package, where the changes in tension accompanying changes in balloon height are small, the twist in the yarn on the package is actually greater when the ring rail is ascending than when it is descending. With cop-build packages, however, tension changes are significant and cause the yarn twist in the balloon to increase as the ring rail is ascending. Variations in the yarn twist on the package throughout the chase depend upon the relative magnitude of the tension and balloon height effects. In general, however, twist is expected to vary during the chase. If the twist density of the yarn in the spinning zone at the shoulder of the chase is less than the mechanical twist, a question arises as to whether the twist density in this zone is only momentarily low just when the ring rail is at the shoulder.
In order to clarify this point, Subramanian et al.4 measured twist density in the yarn in the spinning zone over a doff at four different chase positions. They observed that, over the chase, a periodic variation occurs in the twist density in the yarn in the spinning zone. Starting with a very low value at the shoulder, the twist gradually increases till the nose is reached. It then falls to a low value at the shoulder, so that at any intermediate position of the chase, the value is higher when the ring rail is coming down than when it is going up.
8.5.4 The factors affecting twist variation
Although twist variation is inherent to the mechanism by which twist is being imparted in ring spinning, proper selection of spindle speed, traveller weight and drafting angle will help to provide effective control over it.
The effect of spindle speed
The effect of spindle speed on twist variation has also been studied by Subramanian and others.49 The twist density in the spinning zone was measured at spindle speeds of 6000, 7000 and 9000 rpm. For the same traveller number and yarn count, the twist density in the spinning zone, when winding at the shoulder of the chase, increases as the spindle speed decreases. When winding at the nose, the twist density remains almost unaltered, so that the difference between nose and shoulder twist densities is considerably less at a spindle speed of 6000 rpm than that at 9000 rpm.
The effect of traveller weight
A heavier traveller normally ensures better twist flow in the spinning zone. Even with the heaviest traveller, however, the twist in the spinning zone will be much lower than the mechanical twist when winding at the shoulder.
The effect of varying the traveller weight was studied by Subramanian and others49 at four different stages of the doff. The twist density was always highest when the heaviest traveller was used, at all stages of the doff. The difference in twist densities with a lighter and heavier traveller narrows down towards the end of the doff.
The effect of the drafting angle
The drafting (or roller stand angle) and spinning angles are important parameters controlling twist flow and variation. There are two distinct points of interest for the spinners; the angle at which the yarn is wrapped around the rollers, and the angle of the roller stand. A wrap of a few degrees reduces the tendency of broken ends to lap on the top roller. Too great an angle, however, will impede the twist flow. It is essential that the twist flow run up to the front roller nip to a distance well within the staple length of the raw materials. It is in this context that the angle of the roller stand is important. If the guide eye is vertically below the roller nip, a large roller stand angle is needed to keep the angle of wrap small. When the line thread is vertical, a 60° stand angle results in approximately a 30° wrap, while a 45° stand angle gives angle of wrap of between 10° and 27°.
The best spinning angle is that which gives the best twist flow in the region between the lappet eye and the roller nip. It was once thought that a vertical thread line would give the best results, because it allows twist flow from the bobbin to be absolutely free up to the nip point. This theory appeared to be sound, but in practice it was found not to be entirely correct. The amount of twist generated at the traveller-ring junction is not in fact capable of going beyond the lappet eye in a vertical thread line. When spinning in vertical position, the yarn is in contact with the guide eye at all times, and it tends to hold back the twist in the yarn.
Studies also show that, as the spinning angle increases from the vertical, the pressure between the yarn and the front of the guide eye drops, until at about 35° the yarn is clear of the guide eye for a short time in each revolution of the balloon, and the twist is free to run up to the front roller nip. When the angle increases still further, the yarn remains clear of the guide eye for shorter and shorter periods in each revolution, until finally it is again in constant contact.
8.6 Process control in rotor spinning
As the yarn formation mechanism in rotor spinning is force-restrained rather than position-restrained, proper process control is extremely important to ensure production of yarns with the desired quality at the highest possible speed and with minimum waste.
8.6.1 Control of yarn tension
Yarn tension is much lower in rotor spinning as compared to ring spinning, but controlling yarn tension is still an important factor in avoiding end breakage. This is because the yarn formation mechanism is force-restrained rather than position-restrained in rotor spinning.
Theoretical models
Mack50 originally established the differential equations governing yarn shape and tension inside rotors. The tension, Tr, at a radius r is given by:
where m is the yarn linear density, m the yarn rotational speed, and T0 the tension at the rotor wall. Many authors have followed Stalder52 who showed that T0 is small compared with m ω2b2 (where b is the rotor radius), and have consequently ignored T0. This is convenient because T0 can only be obtained by finding the boundary conditions for the differential equation given by Mack. Arinc53 was the first to determine T0, and found that it is decided by the actual air flow inside the rotor. Arinc also showed that, as the yarn linear density increases, T0 tends towards zero and spinning becomes impossible owing to the change in yarn rotation from forward to backward motion with respect to the rotor. Krause and Soliman54 derived a theoretical relation between yarn-mass variation and the variation of yarn-spinning tension for open-end rotor spinning, and demonstrated experimentally that spinning-tension measurement represents a useful means of assessing yarn irregularity. For an ‘ideal yarn’, the relation between the force variability and mass irregularity was calculated numerically as a function of rotor diameter and mean fibre length. The hypothesis agreed well with experimental results. Practical spinning trials gave proof of the correlation between yarn-mass variability, yarn-spinning tension and yarn strength.
Sampling inspection of the yarn-spinning tension and its variability on open-end spinning machinery could well serve as a useful test for quality-control purposes. Chao and Oxenham55 continued with Arinc’s analysis and were able to show that, as the yarn becomes finer, T0 becomes relatively large compared with the holding-back force against the fibre band at the wall of the rotor. Consequently, as the yarn becomes finer, more and more end breaks will occur owing to the rupture of the fibre band on the rotor wall. Clearly, T0 is important in determining the ultimate linear density limits in rotor spinning, and needs to be calculated as a routine matter, despite the fact that it is much smaller than the yarn tension at the doffing tube. This is rarely done because a computer solution for T0 is needed for each doffing tube, air-flow regime, yarn linear density, and twist factor. Grosberg56 used a simpler method for determining the yarn tension at the rotor wall. He considered that the yarn is situated between the lip of the doffing tube, of radius a, and the rotor wall, of radius b. The tension at b, T0, can be found by imagining the rotor wall removed, and then T0 has to work to overcome air drag on the yarn and friction at the navel. However, the yarn loses energy as it travels from b to a, and this is reflected in the work done by the tension through the force required to overcome the Coriolis acceleration. This method offers an approximate solution, which makes it possible to predict changes in internal yarn tension by a simple closed-form solution as follows:
where t is the twist in the yarn and E is given by:
where C, ρ and d are air drag coefficient, density of air and yarn diameter respectively.
The tension at the rotor wall, as predicted by the above equation, was too large by about 11%. The result is considered useful despite this error because of its simplicity.
8.6.2 The factors affecting yarn tension
Although yarn tension is much lower in rotor spinning as compared to ring spinning, when considering very high speed of rotor and occurrence of twist loss it is extremely important to know in detail about the effect of different factors on yarn tension to keep the end breakage rate within control.
The effect of rotor speed and diameter
In the course of development, rotor speeds have been increased from approximately 30 000 rpm originally to 160 000 rpm today. This has been achieved by reducing rotor diameter. It can be demonstrated that all rotor speeds and diameters introduced in recent decades are governed by the fact that the centrifugal force must be kept within certain limits to avoid end breakage. Assuming that the centrifugal force acting on the thread in the rotor can never exceed yarn tension, this represents a theoretically absolute spinning limit, though this cannot, and is not intended to be reached in practice. Spinning tension must always lie with a sufficient safety margin below normal variations in the inherent strength of the yarn, if the spinning process is to run efficiently.
A minimum possible speed is also allocated to each rotor. There is a risk that the rotor speed (and thus spinning tension) will decline to such an extent that the centrifugal force in the rotor groove is no longer sufficient to generate the twist retention and false-twist effect (between nozzle and rotor groove) on the draw-off nozzle. These twist effects are necessary for spinning stability; if twist integration in the rotor groove is seriously disturbed or interrupted then a thread break occurs. This situation is clearly apparent when calculating the so-called minimum twist multiplier, from which the optimum speed range for each rotor diameter can be derived.
Reducing rotor diameter in the interests of achieving higher rotor speeds and higher output is surprisingly effective. The predicted (lower) limits for rotor diameter have repeatedly been breached by new developments, and nowadays quality yarns are spun (from suitable raw material) with 28 mm diameter rotors operating at speeds of up to 160 000 rpm. The fundamental relationship between rotor diameter and fibre length, although not invalidated, has been decisively modified by such advances in rotor technology.
According to Cheng and Cheng56 a higher rotor speed leads to higher yarn tension. This is the product of frictional force of the yarn at the doffing tube and the centrifugal force acting on the yarn end in the rotor. The tension in the yarn largely results from centrifugal forces, and so is proportional to the square of the product of the rotor speed and diameter. Derichs58 suggested that to maintain an optimal tension with increased rotor speed, the following relationship may be used:
The effect of twist in the yarn
Krause and Soliman54 have shown that specific yarn tension increases with increasing twist factor. This is because a higher axial force is necessary at the spinning point in the rotor to enable a higher twisting rate (and hence a higher yarn torque) to be maintained. The false-twist effect that is usually present in open-end spinning also affects the yarn mass in the radial portion through twist contraction, and thus may influence spinning tension in a different manner for different twist factors.
The effect of navel design
The navel and rotor together form the central spinning unit. It acts both as a twist blocker and a false twister element, turning the yarn gently twice around a solid angle of 90°. Navel design affects the local air compression in the rotor and so has a substantial impact on yarn structure and topography. Other than the number of grooves, the radius of the curvature of the navel is the most obvious design criterion, because the area of the shell increases or decreases with it. The length of the yarn guidance on the navel, the friction path, and the yarn tension, can all be manipulated by this means. It is a far too common mistake to think that a reduction of the radius of the curvature is compellingly linked with a decrease in yarn tension. One has to distinguish clearly between average yarn tension and real yarn tension peaks. Through the changing effects of false twist and twist contraction, the formation of wrapper fibres changes separately from the changing oscillation characteristics of the yarn on the shell. A navel with a smaller radius of curvature and reduced mean values in yarn tension, but higher tension peaks, clearly generates lower yarn elongation values. However, a combination of different design parameters can compensate for this effect. The groove depth substantially affects the retention time of the yarn in the groove and, with it, spin stability. The groove can be used as a vibration agitator to reduce the average frictional force between yarn and spin element, and to improve the propagation of false twist into the rotor groove. There is a fibre-specific optimal average yarn tension, where end breaks are at a minimum.59
The grooves cause the yarn to bounce off the surface of the navel for very brief periods of time. Yarn tensions measured inside the rotor are very close to the theoretical figures, if there are not too many pulses due to yarn riding over the grooves. Unpublished work at NC State University showed that the number of pulses rose with the number of grooves until four grooves were cut. Increasing the number from four to eight gave only four pulses and this was interpreted to mean that the yarn jumped over alternate grooves.60
8.7 Control of end breakage rate and twist loss in rotor spinning
In rotor spinning, the end breakages are broadly classified into three main groups61:
1. Tension yarn breaks. These are found in spun yarn, normally between the take-off nozzle and take-up rollers. The yarn ends on the yarn package have a blunt appearance, and short broken yarn ends are in general found in the rotor groove.
2. Spinning yarn breaks. These occur in the yarn peel-off zone in the rotor groove when continuous fibre spin-in is interrupted.
3. Yarn breaks that result from sliver breaks, or breaks due to similar interference factors outside the spinning box, which can be easily controlled by proper maintenance and good practice.
Das and Ishtiaque62 suggested a new seven-fold method of classifying broken ends in rotor spinning, which is performed by collecting broken ends from the yarn package and by observing the rotor groove after end breakage.
8.7.1 Factors affecting end breakage rate
End breakage in rotor spinning is much lower as compared to ring spinning due to lower yarn tension. Rotor and opening roller speed are the two major factors influencing end breakage in rotor spinning.
The effect of rotor speed
Yarn breakage rate increases as the rotor speed increases. A higher rotor speed causes powerful centrifugal forces on the fibres in the rotor groove,63 resulting in poor spinning stability and an increase in yarn tension, which in turn causes high yarn breakage. Das and Ishtiaque62 observed no clear trend for breakages with a foreign matter embedded end, with an unopened fibrous end, with a seed coat embedded end or with a tapered end, with increase in rotor speed. It was also observed that the proportion of breaks with a tapered end is very high, showing that a large number of yarn breaks occur due to disturbance in the fibrous strand inside the rotor groove. Breaks with a trash particle embedded end and the breaks with a blunt end both consistently increase with rotor speed. At lower speeds, small trash particles are constantly taken away by the yarn, due to the comparatively low centrifugal force acting on these trash particles. They then become entrapped within the yarn structure, and so do not cause breakage. At higher rotor speeds, these small trash particles accumulate inside the rotor groove due to higher centrifugal forces, and after saturation point all the accumulated particles try to come out along with the fibrous strand, causing yarn breakage. Because the spinning tension increases with rotor speed, a rise in blunt ended breakages occurs due to the increase in centrifugal force on the fibre stand and yarn winding speed.
The effect of opening roller speed
As the opening roller speed increases, the carrying factor increases, which in turn increases the opening efficiency of the opening roller.64 The carrying factor is the effective number of wire points per unit of time. Owing to the better opening of fibres, it can be expected that the fibre tufts of smaller size and uniform dimensions are fed into the transport tube and thus into the rotor groove. However, too high an opening roller speed results in higher rotor deposition,65, 66 and fibre orientation inside the transport tube also deteriorates drastically,67 causing higher end breakage.
Das and Ishtiaque62 observed that the proportions of foreign matter embedded in broken ends and blunt broken ends do not show any specific trend with the increase of opening roller speed. The proportion of breaks with unopened fibrous ends increases with the opening roller speed, due to higher rotor deposition and deterioration of fibre orientation (clustering) inside the transport tube. The occurrence of seed coat embedded broken ends shows a decreasing trend with the increase in opening roller speed, because better separation of seed coats from the fibre reduces the deposition of such seed coat particles on the rotor groove. Breaks caused by trash particle embedded ends show an increasing trend with the increase in opening roller speed. This is mainly due to better separation of trash particles, and thus a higher deposition of such particles on the rotor groove. The maximum proportion of broken ends belongs to the tapered end category, and shows a decreasing trend with the increase in opening roller speed. However, the miscellaneous type of break shows a marginally decreasing trend as the opening roller speed increases.
8.7.2 The mechanisms of twist loss in rotor spinning
The machine selected twist in rotor spinning does not accurately correspond to twist measured in the yarn. One reason for this is that surface layer fibres in open-end yarn have a different level of twist from those forming the body of the yarn. A stage will be reached where some fibres are not yet fully twisted while others are already twisting in the opposite direction. These simultaneous opposing twisting effects will cause the measured twist to be lower than the theoretical twist.68
According to Kleinhansl,69 twist loss in the spinning chamber results from torsional stresses in the twisted yarn acting backward past the separation point, and so imparting twist to the strand of fibres in the rotor groove before its full count has been reached. Fibres incorporated into the yarn after that point are not fully twisted and so the total twist is reduced. London and Jordan70 concluded that the difference between measured and machine twist is a function of doffing tube design. According to Lord and Grady,71 the difference between measured and machine twist values, expressed as a twist gradient, is a function of machine design, operating functions and fibre characteristics.
Salhotra72 has put forward a hypothesis to explain twist loss through the incidence of sheath fibres. According to Salhotra the transmission of twisting torque cannot be one hundred percent efficient; there is always a possibility of leakage through fibre ends at the yarn forming point. In the extreme case of a single fibre in the yarn core at yarn formation point, one can reasonably expect a loss of twisting torque in the yarn core.
8.7.3 The factors affecting twist loss
Control of twist loss is extremely important considering its influence on yarn quality and spinning performance. Major factors that influence twist loss are amount of twist and opening roller speed.
The effect of twist factor
The twist properties of rotor yarn have been the subject of several studies. It was mentioned by Audivert7 2 that the difference between adjusted machine twist and measured twist depends on the value of machine twist and yarn linear density, where higher machine twist and higher yarn linear density provides a higher percentage of twist deviation (PTD). Salhotra72 observed that, as the twist factor is increased from 38.3 to 57.4, there is an almost continuous decrease in twist efficiency and an increase in the percentage of sheath fibres. This is obviously due to the well-known increase in the length of the peripheral twist extent (PTE). The higher the machine twist, the higher the twist loss, and so the higher the PTE.
The effect of opening roller speed
The main purpose of the opening roller is to open the fibre sliver to individually aligned single fibres. Well straightened, aligned and parallelised fibres from the opened sliver should make efficiently twisted rotor yarn bodies. Tyagi74 reported a marked increase in twist efficiency with an increase in opening roller speed. The increase in twist efficiency is the result of a decrease in the percentage of sheath fibres. Greater fibre separation at higher opening roller speeds reduces the possibility of fibres becoming wrapped simply because they are entangled with fibres undergoing belt formation. Ülkü75 employed a similar approach, but concluded that there is only a small degree of association between opening roller speed and twist. The slightly increased number of twists for high opening roller speeds can be attributed to the high degree of fibre separation.75 Salhotra72 also studied this effect, and observed that the twist efficiency goes up as the opening roller speed increases.
8.8 Future trends
The main goal of the modern yarn manufacturing industry is to achieve competitive yarn quality and price. Current developments in spinning technology are aimed at maintaining high productivity with effective quality control, by selecting equipment and spinning conditions that suit the raw materials.
The trend towards automation of spinning systems and yarn manufacturing machinery is likely to continue, and automated processes will be increasingly integrated into manufacturing units. Spinning machines that combine carding and drawing functions, and ring frames linked with winders, have already been developed. Research into directly spinning yarns in card and magnetic ring spinning is currently in progress. Production rates are predicted to rise as machines become available with even more spindles, and robot-controlled equipment will become standard.
Improvement in the performance of control strategies will result in more consistent production, facilitating process optimisation, and reducing the need for reprocessing and waste production.
Process models underpin most modern control approaches. Different controllers can be synthesised for different models. Even the prevalent Proportional+Integral+Derivative (PID) algorithm can be utilised, though its performance capabilities are limited. More sophisticated strategies for improving process control include adaptive algorithms and predictive controllers. Statistical process control (SPC) techniques are also experiencing a revival due to the modern emphasis on quality. Attempts are being made to integrate traditional SPC practices with engineering feedback control techniques. Recent interest in the development of practicable nonlinear controllers is of particular significance, because they recognise that many real processes are nonlinear and that adaptive systems may not be able to cope with significant nonlinearities. One approach involves designing control strategies based on nonlinear black box models, for example nonlinear time-series or neural networks. A second method relies on an analytical approach, making use of a physical–chemical model of the process. Cheap powerful computers and advances in the field of Artificial Intelligence are also making an impact. Local controls are increasingly being supplemented with monitoring, supervision and optimisation schemes; roles that were traditionally undertaken by plant personnel.
8.9 References
1. Lüdicke, A. Ein Studie über die ringspindel. Dinglers. Polutech. J.. 1881; 242:334.
2. Escher, R. Theorie der ringspindel. Civil Ing.. 1883; 29:448.
3. Gégauf, C. Analyse Compléte, Théorique et Pratique, Concernant Les Métiers à Filer à Anneaux. Ind. Textile. 1901; 17:273–360. [394, 440].
4. Linder, G. Ballonform, fadenspannung und Läfer-stellung am Ring-spinnmachinen. Leipz. Monatschr. Textil Ind.. 1910; 25(8):123.
5. Baltz, W.E. Ueber die Tatsachliche Ballonform bei der Ringspindel. Milliand Textilber.. 1933; 14:5.
6. Hannah, M. Application of a Theory of the Spinning Balloon. (a). J Textile Inst. 1952; 43:T519. [(b) 1955; 46,T1].
7. Mack, C. Theoretical Study of Ring and Cap Spinning Balloon Curves (With and without Air Drag). J Textile Inst.. 1953; 44:T483.
8. Mack, C., Smart, E. Measurements of the Air-Drag of Textile Thread. J Textile. Inst.. 1954; 45:T348.
9. Crank, J. A Theoretical Investigation of Cap and Ring Spinning System. Textile Res J.. 1953; 23:266.
10. Grishin, P.F., Balloon Control, Platt's Bulletin, 8, 6/161, 8/240, 11/333, 1954.
11. Bracewell, G.M., Greenhalgh, K. Controlled Balloon Spinning. J Textile Inst. 45(T730), 1954.
12. De Barr, A.E. A Descriptive Accounts of Yarn Tensions and Balloon Shapes in Ring Spinning. J. Textile Inst.. 1958; 49:T58.
13. De Barr, A.E. The Role of Air Drag in Ring Spinning. J Textile Inst.. 1961; 52:T126.
14. Mack, C. Theory of the Spinning Balloon. Quart. J. Mech. Appl. Math. 1958; XI:196–207.
15. De Barr, A.E., Catling, H. The Principles and Theory of Ring Spinning, The Textile Institute and Butterworths. Manchester and London; 1965.
16. Nerli, G., Mechanical behaviour of yarn in Certain Textile Manufacture a). Textile Tech Dec. 1984; 56 [(b) 36(Sept. 1985)].
17. Batra, S.K., Ghosh, T.K., Zeidman, M.I. An integrated approach to dynamic analysis of the ring spinning process – Part I: without air drag and coriolis acceleration. Textile Res J. 1989; 59:309–317.
18. Batra, S.K., Ghosh, T.K., Zeidman, M.I. An integrated approach to dynamic analysis of the ring spinning process – Part II: with air drag. Textile Res J. 1989; 59:416–424.
19. Batra, S.K., Ghosh, T.K., Zeidman, M.I. An integrated approach to dynamic analysis of the ring spinning process – Part IV: inherent instability of free balloon. Textile Res J. 1995; 65:417–423.
20. Lisini, G.G. Toni. P., Quilghini, D and Di GiogiCampedelli VL., A comparison of stationary and non-stationary mathematical models for the ring-spinning process. J Textile Inst. 1992; 83:550–559.
21. Fraser, W.B. On the theory of ring spinning. Proc R Soc Lond A. 1993; 342:439–468.
22. Fraser, W.B. Air drag and friction in the two-for-one twister: result from the theory. J Textile Inst. 1993; 84:364–375.
23. Stump, D.M., Fraser, W.B. Transient solutions of the ring spinning balloon equations. J ApplMech. 1996; 63:523–528.
24. Zhu, F., Hall, K., Rahn, C.D. Steady state response and stability of ballooning strings in Air. Int J Non-linear Mech. 1998; 33:33–46.
25. Zhu, F., Rahn, C.D. Limit cycle prediction for ballooning strings. Int J Nonlinear Mech. 2000; 35:373–383.
26. Fan, R., Singh, S.K., Rahn, C.D. Modal analysis of ballooning strings with small curvature. J ApplMech. 2001; 68:332–338.
27. Tang, Z.X., Fraser, W.B., Wang, X. Modelling yarn balloon motion in ring spinning. Appl Math Model. 2007; 31:1397–1410.
28. Tang, Z.X., Wang, X., Fraser, W.B. Simulation and experimental validation of a ring spinning process. Simul Model. 2006; 14:809–816.
29. Tang, Z.X., Wang, X., Fraser, W.B. An investigation of yarn snarling and balloon flutter instabilities in ring spinning. J Textile Inst. 2006; 97:441–448.
30. Rong, Yin, Hong-bo, Gu. Numerical simulation of quasi-stationary ring spinning process linear elastic yarn. Textile Res. J. 2011; 81(i):22–27.
31. Fraser, W.B. The effect of yarn elasticity on an unwinding balloon. J Textile Inst. 1993; 83:603–613.
32. De Barr, A.E. The role of air drag in ring spinning. J Textile Inst. 1961; 52:T126.
33. Anbarsan, M. Optimizing spindle speed. The Indian text. J. 1996; CVI(12):24–30.
34. Catling, H. Traveller-Ring Friction in Spinning. Journal Mechanical Engineering Science. 1962; 4(1):94.
35. Zheng-Xue Tang, W. Barrie, Fraser, Lijing, Wang and Xungai, Wang, Examining the effects of balloon control ring on ring spinning. Fibers and Polymers. 2008; 9(5):625–632.
36. Hori, Y. A Statistical Analysis of Yarn Breakage Rates on a Spinning Frame. J. Text. Mach. Soc. Japan (English Edition). 1955; 1(2):54.
37. De Barr, A.E. End – breakages in ring spinning. J Textile Inst. 1959; 50:T284.
38. Ghosh, A., Ishtiaque, S., Rengasamy, S., Patnaik, A. The Mechanism of end Breakage in ring Spinning: A Statistical model to Predict the End Break in Ring Spinning. AUTEX Research Journal. March 2004; 4(1):19–24.
39. Martindale, J.G. A New Method of Measuring the Irregularity of Yarns with Some Observations on the Origin of Irregularities in Worsted Slivers and Yarns. J Textile Inst.. 1945; 36:T35–T47.
40. Lawrence, C.A., Fundamentals of Spun Yarn Technology. 2002:272–273. [CRC Press].
41. DeBarr, A.E., Catling, H., The Principals and Theory of Ring Spinning. The Textile Institute, Butterworths, 1965:136–137.
42. Klein, W. Spinning Geometry and its significance. Int Text Bull, Yarn Fabric Forming. 1993; 3:22–26.
43. Oxtoby, E., Spun Yarn Technology. Butterworth & Co. (Publishers) Ltd, 1987:111.
44. DeBarr, A.E., Catling, H., The Principals and Theory of Ring Spinning. The Textile Institute and Butterworth & Co. (publishers) Ltd, 1965:129.
45. Axson, M. Observation of the Traveller during Spinning, Letters to Editor. J Textile Inst. 1954; 45:T368.
46. Kanai, K., Kanai, H., Sakata, T., Kamogawa, H. Twist in Ring Spinning and Doubling Part 1: Snail Wire. J. Textile Mach. Soc. Japan. 1960; 13(2):19–23.
47. Gessner, W. Fadenbruchursachen in der Kammgarnspinnerei. Textil-Praxis. January, 1964; Vol-19:58–62.
48. Wegener, W., Hanslandwehrkemp. Die Drehungsverleilung im Fadenbeim Spinnprozess. Textil-Praxis (Ger). October, 1962; Vol-17:995–1000.
49. Subramanian, T.A., Salhotra, K.R., Bhaduri, S.N. Twist Flow in Ring Spinning. Textile Res J. 1995; 37,:195.
50. Mack, C. Quart, Theory of winding in centrifuge spinning. J. Mech. Appl. Math.. 1956; 9:75.
51. Stalder, H., Eidgenossische Technische Hochschule, Zurich, 1968. [Ph.D. Thesis].
52. Arinc, K., Grosberg, P., ‘Open-end Spinning’ in International Textile Seminar, New Delhi, 1973.
53. Krause, H.W., Soliman, H.A. The Significance of Yam Tension in Open-end Spinning. J. Text. Inst. 1976; 67(5):166.
54. Chao, S.N., Oxenham, W.. Proceedings of International Symposium on the Theory and Practice of New Spinning Techniques. InstytutWlokiennictwa, lodz, Poland, 1978:129.
55. Grosberg, P. The Tension Inside the Rotor. J Text Inst. 1984; 75(5):T332.
56. Cheng, Y.S.J., Cheng, K.P.S. Selecting process Parameters that Influence the Strength of Rotor Spun Yarn Formed on the SDL Quickspin System. Textile Res. J. September, 2004; 74(9):792–796.
57. Derichs, J., Theoretical and Practical Limits of Rotor Spinning in the Production of Fine Yarns, Schlafhorst documentation, 1986. [No. 16, a141e-3.86. 1–9].
58. Sonntag, E., Bolze, J., Design of a navel and its influence on yarn structure. Emil BRÖLL GmbH & Co, Austria, 2003.. www.broell.com
59. Lord, R. Handbook of yarn production. Cambridge, England: Woodhead Publishing Ltd.; 2003. [p. 195].
60. Trommer, G. Rotor Spinning. Frankfurt/Main, Germany: Deutscher Fachverlang; 1995.
61. Das, A., Ishtiaque, S.M. End Breakage in Rotor Spinning: Effect of Different Variables on Cotton Yarn End Breakage. AUTEX Research Journal. 4(2), 2004. [June © AUTEX].
62. Ishtiaqe, S.M., Saxena, A.K. Rotor machine variables and yarn structure: part iv – influence of machine variables on configuration of neps and thick places of polyester rotor yarn. Indian J Fibre Text Res. 1998; 23:209.
63. Ishtiaque, S.M., Saxena, A.K. Rotor machine variables and yarn structure: part iii – fibre distribution in yarn in relation to rotor machine variables. Indian J Fibre Text Res. 1998; 23:141.
64. Lawrence, C.A., Tahiroghi, S. Rotor machine variables and yarn structure: part iii – fibre distribution in yarn in relation to rotor machine variables. Indian J Fibre Text Res. 1990; 15:108.
65. Simpson, J., Murray, M.F. Effects of Combing Roller Wire Design and Rotor Speed on open-end Spinning and cotton properties, Textile Res. J. 1979; 49:506.
66. Harlock, S.C., An investigation into feed mechanisms for open-end spinning machines Ph.D thesis. The University of Leeds, 1977.
67. Hunter, L. The Production and Properties of Staple-Fibre Yarns Made by Recently Developed Techniques. Textile Progress. 10(1/2), 1978.
68. Kleinhansl, E. Beitrag zur Drehungsprufung an OE-Garnen. Melliand Textilberichte International (Ger). 1972; 53(1):16–22.
69. London, J.F., Jordan, G.B. Open-End Yarns and Their Characteristics. Knitting Times. 1974; 43(15):44.
70. Lord, P.R., Grady, P.L. Twist Structure of Open End Yarns. Textile Res. J. 1976; 46:123.
71. Salhotra, K.R. The Role of Sheath Fibers in Influencing Twist Loss in Rotor Spinning. Textile Res. J. 1981; 11:710–714.
72. Audivert, R. The Deviation of Measured Twist from Machine Twist in Rotorspun Yarns. J Textile Inst. 1988; 2:333.
73. Tyagi, G.K. Effect of fibre profile, spin finish and opening roller speed on properties of polyester OE rotor-spun yarns. Indian J Fibre Text Res. 2003; 28:411. [December].
74. Ülkü, ![]() ., Acar, M., King, T.G., Özipek, B. Fibre Alignment and Straightening in Opening for Open end spinning. Textile Res J. 1993; 63(6):309.
., Acar, M., King, T.G., Özipek, B. Fibre Alignment and Straightening in Opening for Open end spinning. Textile Res J. 1993; 63(6):309.
75. Ülkü, ![]() ., Özipek, B., Acar, M. Effects of Opening Roller Speed on the Fibre and Yarn Properties in Open-End Friction Spinning. Textile Res J. 1995; 65(10):557.
., Özipek, B., Acar, M. Effects of Opening Roller Speed on the Fibre and Yarn Properties in Open-End Friction Spinning. Textile Res J. 1995; 65(10):557.