Section 1.7 Equations and Inequalities Involving Absolute Value

Before Starting this Section, Review

  1. 1 Properties of absolute value (Section P.1 , page 9)

  2. 2 Intervals (Section P.1 , page 8)

  3. 3 Linear inequalities (Section 1.6 , page 146)

Objectives

  1. 1 Solve equations involving absolute value.

  2. 2 Solve inequalities involving absolute value.

Rescuing a Downed Aircraft

Rescue crews arrive at an airport in Maine to conduct an air search for a missing Cessna with two people aboard. If the rescue crews locate the missing aircraft, they are to mark the global positioning system (GPS) coordinates and notify the mission base. The search planes normally average 110 miles per hour, but weather conditions can affect the average speed by as much as 15 miles per hour (either slower or faster). If a search plane has 30 gallons of fuel and uses 10 gallons of fuel per hour, what is its possible search range (in miles)? In Example 5, we use an inequality involving absolute value to find the plane’s possible search range.

Equations Involving Absolute Value

  1. 1 Solve equations involving absolute value.

Recall that geometrically, the absolute value of a real number a is the distance from the origin on the number line to the point with coordinate a The definition of absolute value is

A summary of the most useful properties of absolute value can be found on page 11 in Section P.1.

Because the only two numbers on the number line that are exactly 2 units from the origin are 2 and −2,−2, they are the only solutions of the equation |x|=2.|x|=2. See Figure 1.12. This simple observation leads to the following rule for solving equations involving absolute value.

Figure 1.12

Distance from the origin

Note that if a=0,a=0, then u=0u=0 is the only solution of |u|=0.|u|=0.

Example 1 Solving an Equation Involving Absolute Value

Solve each equation.

  1. |x+3|=0|x+3|=0

  2. |2x−3|−5=8|2x−3|−5=8

Solution

  1. Let u=x+3.u=x+3. Then, |u|=0|u|=0 has only one solution: u=0.u=0.

    |x+3|=0|u|=0x+3=0u=0x=−3Solve for x.
    |x+3|x+3x===00−3|u|=0u=0Solve for x.

    Now we check the solution in the original equation.

    Check:

    |x+3|=0Original equation|−3+3|?=0Replace x with −3.|0|=0✓Simplify.
    |x+3||−3+3||0|==?=000✓Original equationReplace x with −3.Simplify.

    We have verified that −3−3 is a solution of the original equation.

    The solution set is {−3}.{−3}.

  2. To use the rule for solving equations involving absolute value, we must first isolate the absolute value expression to one side of the equation.

    |2x−3|−5=8|2x−3|=13Add 5 to both sides and simplify.
    |2x−3|−5|2x−3|==813Add 5 to both sides and simplify.

    |u|=13|u|=13 is equivalent to u=13u=13 or u=−13.u=−13. Let u=2x−3;u=2x−3; then |2x−3|=13|2x−3|=13 is equivalent to

    2x−3=13or2x−3=−13.u=13 or u=−132x=162x=−10Add 3 to both sides of each equation.x=8x=−5Divide both sides by 2.
    2x−3=13or2x−3=−13.u=13 or u=−132xx==1682xx==−10−5Add 3 to both sides of each equation.Divide both sides by 2.

    We leave it to you to check these solutions.

    The solution set is {−5, 8}.{−5, 8}.

Practice Problem 1

  1. Solve each equation.

    1. |x−2|=0|x−2|=0

    2. |6x−3|−8=1|6x−3|−8=1

With a little practice, you may find that you can solve these types of equations without actually writing u and the expression it represents. Then you can work with just the expression itself.

Example 2 Solving an Equation of the Form |u|=|v||u|=|v|

Solve: |x−1|=|x+5||x−1|=|x+5|

Solution

If |u|=|v|,|u|=|v|, then u is equal to |v||v| or u is equal to −|v|.−|v|. Because |v|=±v|v|=±v in every case, we have u=vu=v or u=−v.u=−v. Thus,

|u|=|v| is equivalent to u=v or u=−v.|x−1|=|x+5| is equivalent tox−1=x+5orx−1=−(x+5)u=x−1,v=x+5−1=5(False)x=−2Solve for x.
|u||x−1|==|v| is equivalent to u=v or u=−v.|x+5| is equivalent tox−1=x+5orx−1=−(x+5)u=x−1,v=x+5−1=5(False)x=−2Solve for x.

The only solution of |x−1|=|x+5||x−1|=|x+5| is −2,−2, so the solution set is {−2}.{−2}. We leave it to you to check this solution.

Practice Problem 2

  1. Solve: |x+2|=|x−3||x+2|=|x−3|

Example 3 Solving an Equation of the Form |u|=v|u|=v

Solve: |2x−1|=x+5|2x−1|=x+5

Solution

Letting u=2x−1u=2x−1 and v=x+5,v=x+5, the equation |u|=v|u|=v is equivalent to

u=voru=−v2x−1=x+52x−1=−(x+5)2x−1=x+52x−1=−x−5Distributive propertyx=63x=−4Isolate x term.x=6x=−43Solve for x.
u=voru=−v2x−12x−1xx====x+5x+5662x−12x−13xx====−(x+5)−x−5−4−43Distributive propertyIsolate x term.Solve for x.

Check:

x=6orx=−43|2(6)−1|?=6+5|2 (−43)−1|?=−43+5|11|?=11|−83−1|?=−4+153|11|?=11✓ Yes|−113|?=113113?=113✓ Yes
x=6orx=−43|2(6)−1||11||11|=?=?=?6+51111✓ Yes∣∣∣2 (−43)−1∣∣∣∣∣∣−83−1∣∣∣∣∣∣−113∣∣∣113=?=?=?=?−43+5−4+153113113✓ Yes

The solution set of the given equation is {−43, 6}.{−43, 6}.

Practice Problem 3

  1. Solve |3x−4|=2(x−1).|3x−4|=2(x−1).

Inequalities Involving Absolute Value

  1. 2 Solve inequalities involving absolute value.

The equation |x|=2|x|=2 has two solutions: 2 and −2.−2. If x is any real number other than 2 or −2,−2, then |x|<2 or |x|>2.|x|<2 or |x|>2. Geometrically, this means that x is closer than 2 units to the origin if |x|<2|x|<2 or farther than 2 units from the origin if |x|>2.|x|>2. Any number x that is closer than 2 units from the origin is in the interval −2<x<2.−2<x<2. See Figure 1.13(a). Any number x that is farther than 2 units from the origin is in the interval (−∞, −2)(−∞, −2) or the interval (2, ∞),(2, ∞), that is, either x<−2x<−2 or x >2.x >2. See Figure 1.13(b).

Figure 1.13

Graphs of inequalities involving absolute value

This discussion is equally valid when we use any positive real number a instead of the number 2, and it leads to the following rules for replacing inequalities involving absolute value with equivalent inequalities that do not involve absolute value.

Example 4 Solving an Inequality Involving Absolute Value

Solve the inequality |4x−1|≤9|4x−1|≤9 and graph the solution set.

Solution

Rule 2 applies here with u=4x−1u=4x−1 and a=9.a=9.

|4x−1|≤9 is equivalent to−9≤≤4x−1≤9Rule 2, −a≤u≤a1−9≤4x−1+1≤9+1Add 1 to each part.−8≤4x≤10Simplify.−84≤4x4≤104Divide by 4; the sense of theinequality is unchanged.−2≤x≤52Simplify.
|4x−1|−9≤1−9−8−84−2≤≤≤≤≤≤9 is equivalent to4x−1≤94x−1+1≤9+14x≤104x4≤104x≤52Rule 2, −a≤u≤aAdd 1 to each part.Simplify.Divide by 4; the sense of theinequality is unchanged.Simplify.

The solution set is {x|−2≤x≤52};{x∣∣∣−2≤x≤52}; that is, the solution set is the closed interval [−2, 52].[−2, 52]. See Figure 1.14.

Figure 1.14

Practice Problem 4

  1. Solve |3x+3|≤6|3x+3|≤6 and graph the solution set.

Note the following techniques for solving inequalities similar to Example 4.

  1. To solve |4x−1|<9,|4x−1|<9, change ≤≤ to << throughout to obtain the solution (−2, 52).(−2, 52).

  2. To solve |1−4x|≤9,|1−4x|≤9, note that |1−4x|=|4x−1|;|1−4x|=|4x−1|; so the solution is the same as that for Example 4, [−2, 52].[−2, 52].

Example 5 Finding the Search Range of an Aircraft

In the introduction to this section, we wanted to find the possible search range (in miles) for a search plane that has 30 gallons of fuel and uses 10 gallons of fuel per hour. We were told that the search plane normally averages 110 miles per hour but that weather conditions can affect the average speed by as much as 15 miles per hour (either slower or faster). How do we find the possible search range?

Solution

To find the distance a plane flies (in miles), we need to know how long (time, in hours) it flies and how fast (speed, in miles per hour) it flies.

Let x=actualx=actual speed of the search plane, in miles per hour. We know that the actual speed is within 15 miles per hour of the average speed of 110 miles per hour. That is, |actual speed−average speed|≤15 miles per hour.|actual speed−average speed|≤15 miles per hour.

|x−110|≤15Replace the verbal description with an inequality.
|x−110|≤15Replace the verbal description with an inequality.

Solve this inequality for x.

−15≤x−110≤15Rewrite the inequality using Rule 2.110−15≤x≤110+15Add 110 to each part.95≤x≤125Simplify.
−15110−1595≤≤≤x−110≤15x≤110+15x≤125Rewrite the inequality using Rule 2.Add 110 to each part.Simplify.

The actual speed of the search plane is between 95 and 125 miles per hour.

Because the plane uses 10 gallons of fuel per hour and has 30 gallons of fuel, it can fly 3010,3010, or 3 hours. Thus, the actual number of miles the search plane can fly is 3x.

From95≤x≤125,we have3(95)≤3x≤3(125)Multiply each part by 3.285≤3x≤375Simplify.
Fromwe have953(95)285≤≤≤x≤125,3x≤3(125)3x≤375Multiply each part by 3.Simplify.

The search plane’s range is between 285 and 375 miles.

Practice Problem 5

  1. Repeat Example 5 , but let the average speed of the plane be 115 miles per hour and suppose the wind speed can affect the average speed by 25 miles per hour.

Example 6 Solving an Inequality Involving Absolute Value

Solve the inequality |2x−8|≥4|2x−8|≥4 and graph the solution set.

Solution

Rule 4 applies here with u=2x−8 and a=4.u=2x−8 and a=4.

|2x−8|≥4 is equivalent to2x−8≤−4or2x−8≥4Rule 4, u≤−a or u ≥a2x−8+8≤−4+82x−8+8≥4+8Add 8 to each part.2x≤42x≥12Simplify.2x2≤422x2≥122Divide both sides of eachpart by 2.x≤2x≥6Simplify.
|2x−8|≥4 is equivalent to2x−8≤−4or2x−8≥4Rule 4, u≤−a or u ≥a2x−8+82x2x2x≤≤≤≤−4+844222x−8+82x2x2x≥≥≥≥4+8121226Add 8 to each part.Simplify.Divide both sides of eachpart by 2.Simplify.

The solution set is {x|x≤2 or x ≥6};{x|x≤2 or x ≥6}; that is, the solution set is the set of all real numbers x in either of the intervals (−∞, 2](−∞, 2] or [6, ∞).[6, ∞). This set can also be written as (−∞, 2]∪[6, ∞).(−∞, 2]∪[6, ∞). See Figure 1.15.

Figure 1.15

Practice Problem 6

  1. Solve |2x+3|≥6|2x+3|≥6 and graph the solution set.

Example 7 Solving Special Cases of Absolute Value Inequalities

Solve each inequality.

  1. |3x−2|>−5|3x−2|>−5

  2. |5x+3|≤−2|5x+3|≤−2

Solution

  1. Because the absolute value is always nonnegative, |3x−2|>−5|3x−2|>−5 is true for all real numbers x The solution set is the set of all real numbers; in interval notation, we write (−∞, ∞).(−∞, ∞).

  2. There is no real number with absolute value ≤−2≤−2 because the absolute value of any number is nonnegative. The solution set for |5x+3|≤−2|5x+3|≤−2 is the empty set, ∅.∅.

Practice Problem 7

  1. Solve.

    1. |5−9x|>−3|5−9x|>−3

    2. |7x−4|≤−1|7x−4|≤−1

Example 8 Solving an Inequality of the Form |u|<|v||u|<|v|

Solve: |x+1|<3|x−1||x+1|<3|x−1|

Solution

|x+1|<3|x+1|Original inequality|x+1||x+1|<3For x≠1, divide both sides by |x+1|.|x+1x−1|<3|a||b|=|ab|−3<x+1x−1<3|u|<a implies −a<u<a.
|x+1||x+1||x+1|∣∣∣x+1x−1∣∣∣−3<x+1x−1<<<<3|x+1|333Original inequalityFor x≠1, divide both sides by |x+1|.|a||b|=∣∣ab∣∣|u|<a implies −a<u<a.

We solve the two inequalities:

−3<x+1x−1andx+1x−1<30<x+1x−1+30<(x+1)+3(x−1)x−10<2(2x−1)x−10<2x−1x−12x−1x−1>0x+1x−1−3<0(x+1)−3(x−1)x−1<0Combine.−2(x−2)x−1<0Simplify.−(x−2)x−1<0Divide by 2.x−2x−1<0Rewrite.The solution set of this inequalityis S1(Figure 1.16).The solution set of this inequalityis S1(Figure 1.16).
−3<x+1x−1andx+1x−1<300002x−1x−1<<<<>x+1x−1+3(x+1)+3(x−1)x−12(2x−1)x−12x−1x−10The solution set of this inequalityis S1(Figure 1.16).x+1x−1−3(x+1)−3(x−1)x−1−2(x−2)x−1−(x−2)x−1x−2x−1<<<<<00000Combine.Simplify.Divide by 2.Rewrite.The solution set of this inequalityis S1(Figure 1.16).

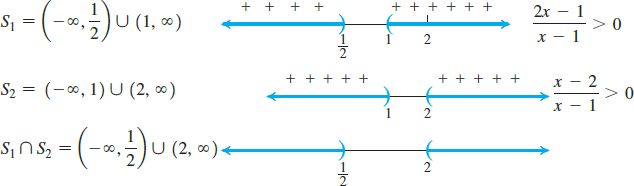
From Figure 1.16, we see that both inequalities are true on S1∩S2.S1∩S2. So the solution set of the given inequality is (−∞, 12)π(2, ∞).(−∞, 12)π(2, ∞).

Figure 1.16

Practice Problem 8

  1. Solve: |x−2|<4|x+4||x−2|<4|x+4|

Side Note

You can use the “test point“ method to verify that the solution set of 2x−1x−1>02x−1x−1>0 is S1S1 and that of x−2x−1>0x−2x−1>0 is S2S2 as shown in Figure 1.16.

Section 1.7 Exercises

Concepts and Vocabulary

In Exercises 1–6, assume that a>0.a>0.

  1. The solution set of the equation |x|=a|x|=a is                        .

  2. The solution set of the inequality |x|<a|x|<a is                        .

  3. The solution set of the inequality |x|≥a|x|≥a is                        .

  4. The equation |u|=|v||u|=|v| is equivalent to u=_u=−−−−−−−−−−−−− or u=_u=−−−−−−−−−−−−−.

  5. True or False. If a<b,a<b, then on the number line point a is to the left of point b.

  6. True or False. The statement “a is nonnegative” can be expressed as an inequality by “a>0a>0.”

  7. True or False. The solution set of |3x−2|<a|3x−2|<a is the same as the solution set of |2−3x|<a.|2−3x|<a.

  8. True or False. If a>0,a>0, the solution set of |3x−2|≤−a|3x−2|≤−a is ∅.∅.

Building Skills

In Exercises 9–36, solve each equation.

  1. |3x|=9|3x|=9

  2. |4x|=24|4x|=24

  3. |−2x|=6|−2x|=6

  4. |−x|=3|−x|=3

  5. |x+3|=2|x+3|=2

  6. |x−4|=1|x−4|=1

  7. |6−2x|=8|6−2x|=8

  8. |6−3x|=9|6−3x|=9

  9. |6x−2|=9|6x−2|=9

  10. |6x−3|=9|6x−3|=9

  11. |2x+3|−1=0|2x+3|−1=0

  12. |2x−3|−1=0|2x−3|−1=0

  13. 12|x|=312∣∣∣x∣∣∣=3

  14. 35|x|=635∣∣∣x∣∣∣=6

  15. |14x+2|=3∣∣∣14x+2∣∣∣=3

  16. |32x−1|=3∣∣∣32x−1∣∣∣=3

  17. 6|1−2x|−8=106|1−2x|−8=10

  18. 5|1−4x|+10=155|1−4x|+10=15 

  19. 2|3x−4|+9=72|3x−4|+9=7

  20. 9|2x−3|+2=−79|2x−3|+2=−7

  21. |2x+1|=−1|2x+1|=−1

  22. |3x+7|=−2|3x+7|=−2

  23. |x2−4|=0∣∣x2−4∣∣=0

  24. |9−x2|=0∣∣9−x2∣∣=0

  25. |1−2x|=3|1−2x|=3

  26. |4−3x|=5|4−3x|=5

  27. |13−x|=23∣∣∣13−x∣∣∣=23

  28. |25−x|=15∣∣∣25−x∣∣∣=15

In Exercises 37–46, solve each equation.

  1. |x+3|=|x+5||x+3|=|x+5|

  2. |x+4|=|x−8||x+4|=|x−8|

  3. |3x−2|=|6x+7||3x−2|=|6x+7| 

  4. |2x−4|=|4x+6||2x−4|=|4x+6| 

  5. |2x−1|=x+1|2x−1|=x+1

  6. |3x−4|=2(x−1)|3x−4|=2(x−1)

  7. |4−3x|=x−1|4−3x|=x−1 

  8. |2−3x|=2x−1|2−3x|=2x−1

  9. |3x+2|=2(x−1)|3x+2|=2(x−1)

  10. |4x+7|=x+1|4x+7|=x+1

In Exercises 47–62, solve each inequality.

  1. |3x|<12|3x|<12

  2. |2x|≤6|2x|≤6

  3. |4x|>16|4x|>16

  4. |3x|>15|3x|>15

  5. |x+1|<3|x+1|<3

  6. |x−4|<1|x−4|<1

  7. |x|+2≥−1|x|+2≥−1

  8. |x|+2>−7|x|+2>−7

  9. |2x−3|<4|2x−3|<4 

  10. |4x−6|≤6|4x−6|≤6

  11. |5−2x|>3|5−2x|>3 

  12. |3x−3|≥15|3x−3|≥15 

  13. |3x+4|≤19|3x+4|≤19 

  14. |9−7x|<23|9−7x|<23

  15. |2x−15|<0|2x−15|<0

  16. |x+5|≤−3|x+5|≤−3

In Exercises 63–72, solve each inequality.

  1. |x−2x+3|<1∣∣∣x−2x+3∣∣∣<1

  2. |x+3x−1|<2∣∣∣x+3x−1∣∣∣<2

  3. |2x−3x+1|≤3∣∣∣2x−3x+1∣∣∣≤3

  4. |2x−13x+2|≤1∣∣∣2x−13x+2∣∣∣≤1

  5. |x−1x+2|≥2∣∣∣x−1x+2∣∣∣≥2

  6. |x+3x−2|≥3∣∣∣x+3x−2∣∣∣≥3

  7. |2x+1x−1|>4∣∣∣2x+1x−1∣∣∣>4

  8. |2x−13x+2|>5∣∣∣2x−13x+2∣∣∣>5

  9. |x−1|≤2|2x−5||x−1|≤2|2x−5|

  10. 2|x−5|≤|2x−3|2|x−5|≤|2x−3|

Applying the Concepts

  1. Varying temperatures. The inequality |T−75|≤20,|T−75|≤20, where T is in degrees Fahrenheit, describes the daily temperature in Tampa during December. Give an interpretation for this inequality, assuming that the high and low temperatures in Tampa during December satisfy the equation |T−75|=20.|T−75|=20.

  2. Scale error. A butcher’s scale is accurate to within ±0.05±0.05 pound. A sirloin steak weighs 1.14 pounds on this scale. Let x=actualx=actual weight of the steak. Write an absolute value inequality in x whose solution is the range of possible values for the actual weight of the steak.

  3. Company budget. A company budgets $700 for office supplies. The actual expense for budget supplies must be within ±$50±$50 of this figure. Let x=actualx=actual expense for the office supplies. Write an absolute value inequality in x whose solution is the range of possible amounts for the expense of the office supplies.

  4. National achievement scores. Suppose 68% of the scores on a national achievement exam will be within ±90±90 points of a score of 480. Let x=ax=a score among the 68% just described. Write an absolute value inequality in x whose solution is the range of possible scores within ±90±90 points of 480.

  5. Blood pressure. Suppose 60% of Americans have a systolic blood pressure reading of 120, plus or minus 6.75. Let x=ax=a blood pressure reading among the 60% just described. Write an absolute value inequality in x whose solution set is the range of possible blood pressure readings within ±6.75±6.75 points of 120.

  6. Gas mileage. A motorcycle has approximately 4 gallons of gas, with an error margin of ±14±14 of a gallon. If the motorcycle gets 37 miles per gallon of gas, how many miles can the motorcycle travel?

  7. Event planning. An event planner expects about 120 people at a private party. She knows that this estimate could be off by 15 people (more or fewer). Food for the event costs $48 per person. How much might the event planner’s food expense be?

  8. Company bonuses. Bonuses at a company are usually given to about 60 people each year. The bonuses are $1200 each, and the estimate of 60 recipients may be off by 7 people (more or fewer). How much might the company spend on bonuses this year?

  9. Fishing revenue. Sarah sells the fish she catches to a local restaurant for 60 cents a pound. She can usually estimate how much her catch weighs to within ±12±12 pound. She estimates that she has 32 pounds of fish. How much might she get paid for her catch?

  10. Ticket sales. Ticket sales at an amusement park average about 460 on a Sunday. If actual Sunday sales are never more than 25 above or below the average and if each ticket costs $29.50, how much money might the park take in on a Sunday?

Beyond the Basics

In Exercises 83–88, solve each equation.

    1. |x2−9|=x−3∣∣x2−9∣∣=x−3

    2. |x2−8|=−2x∣∣x2−8∣∣=−2x

    3. |x2−5x|=6∣∣x2−5x∣∣=6

    4. |x2+3x−2|=2∣∣x2+3x−2∣∣=2

    1. |x2−7|=|6x|∣∣x2−7∣∣=∣∣6x∣∣

    2. |x2−2x|=|5x−10|∣∣x2−2x∣∣=∣∣5x−10∣∣

    3. |2x2−3x+5|=|x2−4x+7|∣∣2x2−3x+5∣∣=∣∣x2−4x+7∣∣

    4. |x2+x+3|=|x2+5x+1|∣∣x2+x+3∣∣=∣∣x2+5x+1∣∣

  3. |2x−3|+|x−2|=4|2x−3|+|x−2|=4

    [Consider three cases:

    1. −∞ <x≤32,−∞ <x≤32,

    2. 32<x≤2,32<x≤2,

    3. 2<x<∞.2<x<∞.]

  4. 2|2x−3|−3|x−2|=52|2x−3|−3|x−2|=5

  5. |x|2−4|x|−7=5|x|2−4|x|−7=5

  6. 2|x|2−|x|+8=112|x|2−|x|+8=11

  7. Show that if 0<a<b0<a<b and 0<c<d,0<c<d, then ac<bd.ac<bd.

  8. Show that if 0<a<b<c0<a<b<c and 0<e<f<g,0<e<f<g, then ae<bf<cg.ae<bf<cg.

  9. Show that if x2<ax2<a and a>0,a>0, the solution set of the inequality x2<ax2<a is {x|−√a<x<√a},{x|−a−−√<x<a−−√}, or in interval notation, (−√a, √a).(−a−−√, a−−√). [Hint: a=(√a)2;a=(a−−√)2; factor x2−a.x2−a.]

  10. Show that if x2>ax2>a and a>0,a>0, then the solution set of the inequality x2>ax2>a is (−∞, −√a)∪(√a, ∞).(−∞, −a−−√)∪(a−−√, ∞).

In Exercises 93–104, write an absolute value inequality with variable x whose solution set is in the given interval(s).

  1. (1, 7)

  2. [3, 8]

  3. [−2, 10][−2, 10]

  4. (−7, −1)(−7, −1)

  5. (−∞, 3)∪(11, ∞)(−∞, 3)∪(11, ∞)

  6. (−∞, −1)∪(5, ∞)(−∞, −1)∪(5, ∞)

  7. (−∞, −5]∪[10, ∞)(−∞, −5]∪[10, ∞)

  8. (−∞, −3]∪[−1, ∞)(−∞, −3]∪[−1, ∞)

  9. (a, b)

  10. [c, d]

  11. (−∞, a)∪(b, ∞)(−∞, a)∪(b, ∞)

  12. (−∞, c]∪[d, ∞)(−∞, c]∪[d, ∞)

  13. Varying temperatures. The weather forecast predicts that temperatures will be between 8°8° and 70°70° Fahrenheit. Let x=temperaturex=temperature in degrees Fahrenheit. Write an absolute value inequality in x whose solution is the predicted range of temperatures.

In Exercises 106–111, solve each inequality for x.

  1. |x−1|≤5|x−1|≤5 and |x|≥2|x|≥2

  2. 1≤|x−2|≤31≤|x−2|≤3

  3. |x−1|+|x−2|≤4|x−1|+|x−2|≤4

  4. |x−2|+|x−4|≥8|x−2|+|x−4|≥8

  5. |x−1|+|x−2|+|x−3|≤6|x−1|+|x−2|+|x−3|≤6

  6. |x−1| − (x+2)x+2≥0|x−1| − (x+2)x+2≥0

Critical Thinking/Discussion/Writing

  1. For which values of x is √(x−3)2=x−3?(x−3)2−−−−−−−√=x−3?

  2. For which values of x is

    √(x2−6x+8)2=x2−6x+8?
    (x2−6x+8)2−−−−−−−−−−−−√=x2−6x+8?

  3. Solve |x−3|2−7|x−3|+10=0.|x−3|2−7|x−3|+10=0.

    [Hint: Let u=|x−3|.u=|x−3|.]

Getting Ready for the Next Section

In Exercises 115–122, simplify each expression.

  1. 2+522+52

  2. −3+72−3+72

  3. −3−72−3−72

  4. (a−b)−(a+b)2(a−b)−(a+b)2

  5. √(5−2)2+(3−7)2(5−2)2+(3−7)2−−−−−−−−−−−−−−−√

  6. √(−8+3)2+(−5−7)2(−8+3)2+(−5−7)2−−−−−−−−−−−−−−−−−−√

  7. √(2−5)2+(8−6)2(2−5)2+(8−6)2−−−−−−−−−−−−−−−√

  8. √(√3−√12)2+(√2+√8)2(3–√−12−−√)2+(2–√+8–√)2−−−−−−−−−−−−−−−−−−−−−−√

In Exercises 123–128, add the appropriate term to each binomial so that it becomes a perfect square trinomial. Write and factor the trinomial.

  1. x2+4xx2+4x

  2. x2−6xx2−6x

  3. x2−5xx2−5x

  4. x2+7xx2+7x

  5. x2+32xx2+32x

  6. x2−45xx2−45x

..................Content has been hidden....................

You can't read the all page of ebook, please click here login for view all page.
Reset
18.224.54.136