1.6. Selection and Adjustment of Grain-Sizes
The ability to select a proper grain-size world for a given problem is fundamental to human intelligence and flexibility. The ability enables us to map the complex world around us into a simple one that is computationally tractable. At the same time, the attributes in question of the world are still preserved. As mentioned before, it is not necessarily true that any classification can achieve the goal.
How to select a proper grain-size is a domain-dependent problem mainly. We'll discuss some general principles below via semi-order spaces.
It has been shown that the compatibility of R and T is the foundation for assuring that  is also a semi-order space. We will discuss below how to adjust R such that it is compatible with T.
is also a semi-order space. We will discuss below how to adjust R such that it is compatible with T.
1.6.1. Mergence Methods
Assume that 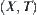 is a semi-order space, where X is a finite set, R is an equivalence relation on X and is incompatible with T.
is a semi-order space, where X is a finite set, R is an equivalence relation on X and is incompatible with T. 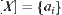 is a quotient space corresponding to R. We will discuss how to adjust R by mergence methods.
is a quotient space corresponding to R. We will discuss how to adjust R by mergence methods.
We define a new partition 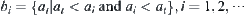 , where ‘<’ is a topology
, where ‘<’ is a topology 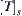 on
on  induced from T. Obviously,
induced from T. Obviously, 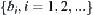 is a partition of X. Let
is a partition of X. Let  be an equivalence relation corresponding to partition
be an equivalence relation corresponding to partition 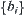 .
.
Proposition 1.9
Proof:
For 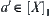 , if
, if 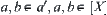 , from the definitions of
, from the definitions of 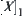 and
and  , we have
, we have 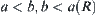 , i.e., any neighborhood of a must contain b and is also the neighborhood of b. Conversely, any neighborhood of b must contain a and is also the neighborhood of a. Thus, the neighborhood systems of a and b are the same. Their common neighborhood system is just the neighborhood system of a′ on
, i.e., any neighborhood of a must contain b and is also the neighborhood of b. Conversely, any neighborhood of b must contain a and is also the neighborhood of a. Thus, the neighborhood systems of a and b are the same. Their common neighborhood system is just the neighborhood system of a′ on 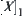 . In other words, if elements on [X] having a common neighborhood system are classified as one category, then [X] is revised as
. In other words, if elements on [X] having a common neighborhood system are classified as one category, then [X] is revised as 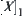 .
.
Now we prove that  is compatible. Assuming
is compatible. Assuming  and
and  , from the above discussion,
, from the above discussion, 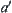 and
and 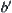 on
on 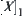 have a common neighborhood system.
have a common neighborhood system. 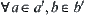 ,
, 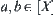 , a and b have a common neighborhood system. From the definition of
, a and b have a common neighborhood system. From the definition of 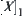 , a and b belong to the same category on
, a and b belong to the same category on 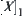 . Thus,
. Thus, 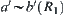 , i.e.,
, i.e.,  is compatible.
is compatible.
Finally, we prove that  is the finest one. Assume that
is the finest one. Assume that 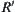 is any partition and
is any partition and 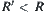 .
.
We will prove below that 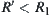 . Let
. Let 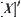 be a quotient space corresponding to
be a quotient space corresponding to 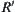 and its projection be
and its projection be 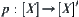 . For
. For 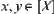 and
and  , let
, let 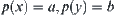 ,
, 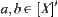 .
.
Assume that 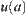 is any neighborhood of
is any neighborhood of  on
on 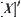 . Since
. Since 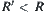 , regarding
, regarding 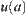 as a set on
as a set on  , it is open, i.e.,
, it is open, i.e., 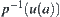 is open on
is open on  . Since
. Since 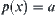 ,
, 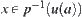 , i.e.,
, i.e., 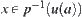 is a neighborhood of x. On the other hand, since
is a neighborhood of x. On the other hand, since  , x and y have the same neighborhood system, i.e.,
, x and y have the same neighborhood system, i.e., 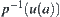 is also a neighborhood of y. Thus,
is also a neighborhood of y. Thus, 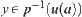 . We have
. We have 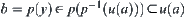 , i.e.,
, i.e., 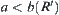 .
.
Similarly, 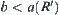 , i.e.,
, i.e., 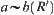 .
.
Finally, 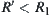 .
.
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
