Problem 1.1

Stability and composition of transfer functions

G. Fernández-Anaya

Departamento de Ciencias Básicas

Universidad Iberoaméricana

Lomas de Santa Fe

01210 México D.F.

México

[email protected]

J. C. Martínez-García

Departamento de Control Automático

CINVESTAV-IPN

A.P. 14-740

07300 México D.F.

México

[email protected]

1 INTRODUCTION

As far as the frequency-described continuous linear time-invariant systems are concerned, the study of control-oriented properties (like stability) resulting from the substitution of the complex Laplace variable s by rational transfer functions have been little studied by the Automatic Control community. However, some interesting results have recently been published:

Concerning the study of the so-called uniform systems, i.e., LTI systems consisting of identical components and amplifiers, it was established in [8] a general criterion for robust stability for rational functions of the form D(f(s)), where D(s) is a polynomial and f(s) is a rational transfer function. By applying such a criterium, it gave a generalization of the celebrated Kharitonov’s theorem [7], as well as some robust stability criteria under - Huncertainty. The results given in [8] are based on the so-called H-domains.¹ As far as robust stability of polynomial families is concerned, some Kharito- nov’s like results [7] are given in [9] (for a particular class of polynomials), when interpreting substitutions as nonlinearly correlated perturbations on the coefficients.

More recently, in [1], some results for proper and stable real rational SISO functions and coprime factorizations were proved, by making substitutions with (s) = (as + b) / (cs + d), where a, b, c, and d are strictly positive real numbers, and with ad−bc ≠0. But these results are limited to the bilinear transforms, which are very restricted.

In [4] is studied the preservation of properties linked to control problems (like weighted nominal performance and robust stability) for Single-Input SingleOutput systems, when performing the substitution of the Laplace variable (in transfer functions associated to the control problems) by strictly positive real functions of zero relative degree. Some results concerning the preservation of control-oriented properties in Multi-Input Multi-Output systems are given in [5], while [6] deals with the preservation of solvability conditions in algebraic Riccati equations linked to robust control problems.

Following our interest in substitutions we propose in section 22.2 three interesting problems. The motivations concerning the proposed problems are presented in section 22.3.

2DESCRIPTION OF THE PROBLEMS

In this section we propose three closely related problems. The first one concerns the characterization of a transfer function as a composition of transfer functions. The second problem is a modified version of the first problem: the characterization of a transfer function as the result of substituting the Laplace variable in a transfer function by a strictly positive real transfer function of zero relative degree. The third problem is in fact a conjecture concerning the preservation of stability property in a given polynomial resulting from the substitution of the coefficients in the given polynomial by a polynomial with non-negative coefficients evaluated in the substituted coefficients.

Problem 1: Let a Single Input Single Output (SISO) transfer function G(s) be given. Find transfer functions G0(s) and H(s) such that:

1.

2. H (s) preserves proper stable transfer functions under substitution of the variable s by H (s), and:

3. The degree of the denominator of H(s) is the maximum with the properties 1 and 2.

Problem 2: Let a SISO transfer function G(s) be given. Find a transfer function G0 (s) and a Strictly Positive Real transfer function of zero relative degree (SPR0), say H(s), such that:

1.

2. The degree of the denominator of H(s) is the maximum with the property 1.

Problem 3: (Conjecture) Given any stable polynomial:

and given any polynomial q(s) with non-negative coefficients, then the poly-nomial:

is stable (see [3]).

3 MOTIVATIONS

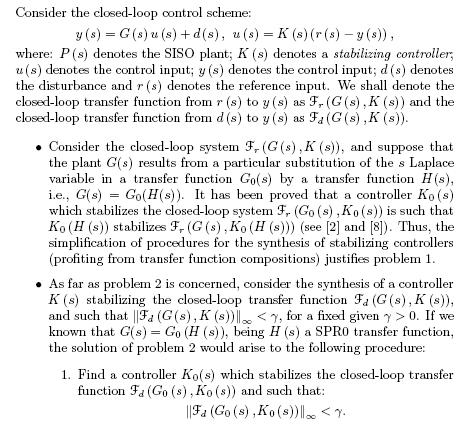
2. The composed controller K (s) = stabilizes the closed-loop system (G(s) ,K (s)) and:

(see [2], [4], and [5]).

It is clear that condition 3 in the first problem, or condition 2 in the second problem, can be relaxed to the following condition: the degree of the denominator of H (s) is as high as be possible with the appropriate conditions. With this new condition, the open problems are a bit less difficult.

• Finally, problem 3 can be interpreted in terms of robustness under positive polynomial perturbations in the coefficients of a stable transfer function.

BIBLIOGRAPHY

[1] G. Fernández, S. Muñoz, R. A. Sánchez, and W. W. Mayol, “Simultaneous stabilization using evolutionary strategies, ”Int. J. Contr., vol. 68, no. 6, pp. 1417-1435, 1997.

[2] G. Fernández, “Preservation of SPR functions and stabilization by substitutions in SISO plants, ”IEEE Transaction on Automatic Control , vol. 44, no. 11, pp. 2171-2174, 1999.

[3] G. Fernández and J. Alvarez, “On the preservation of stability in families of polynomials via substitutions, ”Int. J. of Robust and Nonlinear Control, vol. 10, no. 8, pp. 671-685, 2000.

[4] G. Fernández, J. C. Martínez-García, and V. Kučera, “-Robustness Properties Preservation in SISO Systems when applying SPR Substitutions, ” Submitted to the International Journal of Automatic Control.

[5] G. Fernández and J. C. Martínez-García, “MIMO Systems Properties Preservation under SPR Substitutions, ” International Symposium on the Mathematical Theory of Networks and Systems (MTNS’2002), University of Notre Dame, USA, August 12-16, 2002.

[6] G. Fernández, J. C. Martínez-García, and D. Aguilar-George, “Preservation of solvability conditions in Riccati equations when applying SPR0 substitutions, ” submitted to IEEE Transactions on Automatic Control, 2002.

[7] V. L. Kharitonov, “Asymptotic stability of families of systems of linear differential equations, ”Differential’nye Uravneniya, vol. 14, pp. 2086 2088, 1978.

[8] B. T. Polyak and Ya. Z. Tsypkin, “Stability and robust stability of uniform systems, ”Automation and Remote Contr., vol. 57, pp. 1606-1617, 1996.

[9] L. Wang, “Robust stability of a class of polynomial families under nonlinearly correlated perturbations, ”System and Control Letters, vol. 30, pp. 25-30, 1997.

1 The H-domain of a function f (s) is defined to be the set of points h on the complex plane for which the function f (s) - h has no zeros on the open right-half complex plane.