Problem 2.4
On the convergence of normal forms for
analytic control systems
Wei Kang
Department of Mathematics,
Naval Postgraduate School
Monterey, CA 93943
USA
Arthur J. Krener
Department of Mathematics,
University of California
Davis, CA 95616
USA
1 BACKGROUND
A fruitful technique for the local analysis of a dynamical system consists of using a local change of coordinates to transform the system to a simpler form, which is called a normal form. The qualitative behavior of the original system is equivalent to that of its normal form which may be easier to analyze. A bifurcation of a parameterized dynamics occurs when a change in the parameter leads to a change in its qualitative properties. Therefore, normal forms are useful in analyzing when and how a bifurcation will occur. In his dissertation, Poincaré studied the problem of linearizing a dynamical system around an equilibrium point, linear dynamics being the simplest normal form. Poincaré’s idea is to simplify the linear part of a system first, using a linear change of coordinates. Then the quadratic terms in the system are simplified, using a quadratic change of coordinates, then the cubic terms, and so on. For systems that are not linearizable, the Poincaré-Dulac theorem provides the normal form.
Given a 
dynamical system in its Taylor expansion around x = 0,
As is pointed out in [1], even in cases where the formal series are divergent, the method of normal forms turns out to be a powerful device in the study of nonlinear dynamical systems. A few low degree terms in the normal form often give significant information on the local behavior of the dynamics.
2 THE OPEN PROBLEM
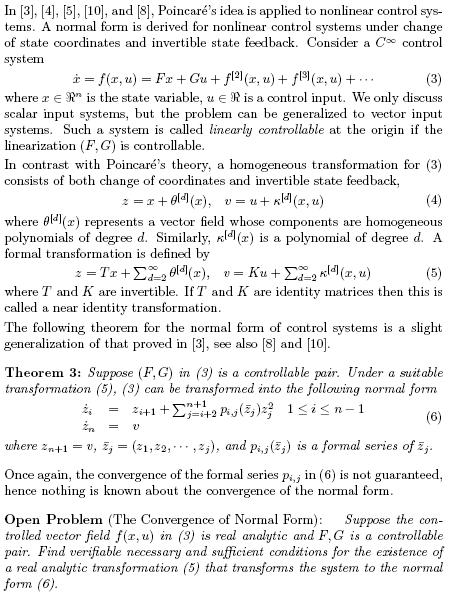
Normal forms of control systems have proven to be a powerful tool in the analysis of local bifurcations and local qualitative performance of control systems. A convergent normal form will make it possible to study a control system over the entire region in which the normal form converges. Global or semi-global results on control systems and feedback design can be proved by studying analytic normal forms.
3 RELATED RESULTS
The convergence of the Poincaré normal form was an active research topic in dynamical systems. According to Poincaré’s Theorem
and Siegel’s theorem, the location of eigenvalues determines the convergence. If the eigenvalues are located in the Poincaré
domain with no resonances, or if the eigenvalues are located in the Siegel domain and are of type (C, ), then the analytic
vector field that defines the system is biholomorphically equivalent to a linear vector field. Clearly, the normal form converges
because it has only a linear part. The Poincaré-Dulac theorem deals with a more complicated case. It states that if the eigenvalues
of an analytic vector field belong to the Poincare domain, then the field is biholomorphically equivalent to a polynomial
vector field. Therefore, the Poinca
re normal form has only finite many terms, and hence is convergent.
As for control systems, it is proved in [5] that if an analytic control system is linearizable by a formal transformation, than it is linearizable by an analytic transformation. It is also proved in [5] that a class of three-dimensional analytic control systems, which are not necessarily linearizable, can be transformed to their normal forms by analytic transformations. No other results on the convergence of control system normal forms are known to us.
The convergence problem for control systems has a fundamental difference from the convergence results of Poincaré-Dulac. For the latter, the location of the eigenvalues are crucial and the eigenvalues are invariant under change of coordinates. However, the eigenvalues of a control system can be changed by linear state feedback. It is unknown what intrinsic factor in control systems determines the convergence of their normal form or if the normal form is always convergent.
The convergence of normal forms is an important problem to be addressed.
Applications of normal forms for control systems are proved to be successful.
In [6] the normal forms are used to classify the bifurcation of equilibrium sets and controllability for uncontrollable systems. In [7] the control of bifurcations using state feedback is introduced based on normal forms. For discrete-time systems, normal form and the stabilization of Naimark-Sacker bifurcation are addressed in [2]. In [10] a complete characterization for the symmetry of nonlinear systems is found for linearly controllable systems.
In addition to linearly controllable systems, the normal form theory has been generalized to larger family of control systems. Normal forms for systems with uncontrollable linearization are derived in several papers ([6], [7], [8], and [10]). Normal forms of discrete-time systems can be found in [9] and [2].
The convergence of these normal forms is also an open problem.