Problem 6.4

Lie algebras and stability of switched

nonlinear systems

Daniel Liberzon

Coordinated Science Laboratory

University of Illinois at Urbana-Champaign

Urbana, IL 61801

USA

[email protected]

1 PRELIMINARY DESCRIPTION OF THE PROBLEM

Suppose that we are given a family of continuously differentiable functions from , parameterized by some index set P. This gives rise to the switched system

where is a piecewise constant function of time, called a switching signal. Impulse effects (state jumps), infinitely fast switching (chatter-ing), and Zeno behavior are not considered here. We are interested in the following problem: find conditions on the functions which guarantee that the switched system (1) is asymptotically stable, uniformly over the set of all possible switching signals. If this property holds, we will refer to the switched system simply as being stable. It is clearly necessary for each of the subsystems to be asymptotically stable—which we henceforth assume—but simple examples show that this condition alone is not sufficient.

The problem posed above naturally arises in the stability analysis of switched systems in which the switching mechanism is either unknown or too complicated to be explicitly taken into account. This problem has attracted considerable attention and has been studied from various angles (see [7] for references). Here we explore a particular research direction, namely, the role of commutation relations among the subsystems being switched. In the following sections, we provide an overview of available results on this topic and delineate the open problem more precisely.

2 AVAILABLE RESULTS: LINEAR SYSTEMS

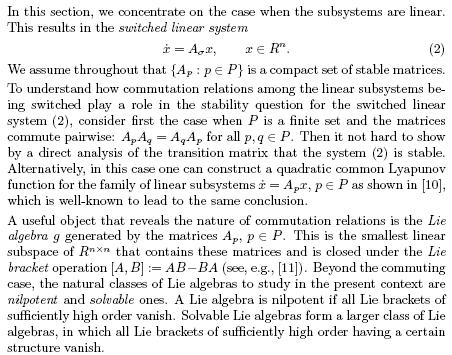
If P is a finite set and g is a nilpotent Lie algebra, then the switched linear system (2) is stable; this was proved in [4] for the discrete-time case. The system (2) is still stable if g is solvable and P is not necessarily finite (as long as the compactness assumption made at the beginning of this section holds). The proof of this more general result, given in [6], relies on the facts that matrices in a solvable Lie algebra can be simultaneously put in the triangular form (Lie’s Theorem) and that a family of linear systems with stable triangular matrices has a quadratic common Lyapunov function.

It was subsequently shown in [1] that the switched linear system (2) is stable if the Lie algebra g can be decomposed into a sum of a solvable ideal and a subalgebra with a compact Lie group. Moreover, if g fails to satisfy this condition, then it can be generated by families of stable matrices giving rise to stable as well as to unstable switched linear systems, i.e., the Lie algebra alone does not provide enough information to determine whether or not the switched linear system is stable (this is true under the additional technical requirement that I ¤ g).

By virtue of the above results, one has a complete characterization of all matrix Lie algebras g with the property that every set of stable generators for g gives rise to a stable switched linear system. The interesting and rather surprising discovery is that this property depends only on the structure of g as a Lie algebra, and not on the choice of a particular matrix represen- tation of g. Namely, Lie algebras with this property are precisely the Lie algebras that admit a decomposition of the kind described earlier. Thus, in the linear case, the extent to which commutation relations can be used to distinguish between stable and unstable switched systems is well understood.

Lie-algebraic sufficient conditions for stability are mathematically appealing and easily checkable in terms of the original data (it has to be noted, however, that they are not robust with respect to small perturbations in the data and therefore highly conservative).

3 OPEN PROBLEM: NONLINEAR SYSTEMS

We shall now turn to the general nonlinear situation described by equation (1). Linearizing the subsystems and applying the results described in the previous section together with Lyapunov’s indirect method, it is not hard to obtain Lie-algebraic conditions for local stability of the system (1). This was done in [6, 1]. However, the problem we are posing here is to investigate how the structure of the Lie algebra generated by the original nonlinear vector fields is related to stability properties of the switched system (1). Taking higher-order terms into account, one may hope to obtain more widely applicable Lie-algebraic stability criteria for switched nonlinear systems.

The first step in this direction is the result proved in [8] that if the set P is finite and the vector fields commute pairwise, in the sense that

then the switched system (1) is (globally) stable. In fact, commutativity of the flows is all that is needed, and the continuous differentiability assumption on the vector fields can be relaxed. If the subsystems are exponentially stable, a construction analogous to that of [10] can be applied in this case to obtain a local common Lyapunov function; see [12].

A logical next step is to study switched nonlinear systems with nilpotent or solvable Lie algebras. One approach would be via simultaneous triangu-larization, as done in the linear case. Nonlinear versions of Lie’s Theorem, which provide Lie-algebraic conditions under which a family of nonlinear systems can be simultaneously triangularized, are developed in [3, 5, 9].

However, as demonstrated in [2], the triangular structure alone is not sufficient for stability in the nonlinear context. Additional conditions that can be imposed to guarantee stability are identified in [2], but they are coordinate-dependent and so cannot be formulated in terms of the Lie algebra. Moreover, the results on simultaneous triangularization described in the papers mentioned above require that the Lie algebra have full rank, which is not true in the case of a common equilibrium. Thus an altogether new approach seems to be required.

In summary, the main open question is this:

Q: Which structural properties (if any) of the Lie algebra generated by a noncommuting family of asymptotically stable nonlinear vector fields guarantee stability of every corresponding switched system? For example, when does nilpotency or solvability of the Lie algebra imply stability?

To begin answering this question, one may want to first address some special classes of nonlinear systems, such as homogeneous systems or systems with feedback structure. One may also want to restrict attention to finite-dimensional Lie algebras.

A more general goal of this paper is to point out the fact that Lie algebras seem to be directly connected to stability of switched systems and, in view of the well-established theory of the former and high theoretical interest as well as practical importance of the latter, there is a need to develop a better understanding of this connection. It may also be useful to pursue possible relationships with Lie-algebraic results in the controllability literature (see [1] for a brief preliminary discussion on this matter).

BIBLIOGRAPHY

[1] A. A. Agrachev and D. Liberzon, “Lie-algebraic stability criteria for switched systems, ” SIAM J. Control Optim., 40:253–269, 2001.

[2] D. Angeli and D. Liberzon, “ A note on uniform global asymptotic stability of nonlinear switched systems in triangular form, ” In: Proc. 14th Int. Symp. on Mathematical Theory of Networks and Systems (MTNS), 2000.

[3] P. E. Crouch, “Dynamical realizations of finite Volterra series, ” SIAM J. Control Optim., 19:177–202, 1981.

[4] L. Gurvits, “Stability of discrete linear inclusion, ” Linear Algebra Appl. , 231:47–85, 1995.

[5] M. Kawski, “Nilpotent Lie algebras of vectorfields‘, ” J. Reine Angew. Math., 388:1–17, 1988.

[6] D. Liberzon, J. P. Hespanha, and A. S. Morse, “Stability of switched systems: a Lie-algebraic condition, ” Systems Control Lett., 37:117–122, 1999.

[7] D. Liberzon, Switching in Systems and Control. Birkhäuser, Boston, 2003.

[8] J. L. Mancilla-Aguilar, “A condition for the stability of switched nonlinear systems, ” IEEE Trans. Automat. Control, 45:2077–2079, 2000.

[9] A. Marigo, “Constructive necessary and sufficient conditions for strict triangularizability of driftless nonholonomic systems‘, ” In: Proc. 38th IEEE Conf. on Decision and Control, pages 2138–2143, 1999.

[10] K. S. Narendra and J. Balakrishnan, “A common Lyapunov function for stable LTI systems with commuting A-matrices, ” IEEE Trans. Automat. Control, 39:2469–2471, 1994.

[11] H. Samelson, Notes on Lie Algebras. Van Nostrand Reinhold, New York, 1969.

[12] H. Shim, D. J. Noh, and J. H. Seo, “Common Lyapunov function for exponentially stable nonlinear systems, ” In: Proc. 4th SIAM Conference on Control and its Applications, 1998.