Problem 7.2

A Hautus test for infinite-dimensional

systems

Birgit Jacob

Fachbereich Mathematik

Universität Dortmund

D-44221 Dortmund

Germany

[email protected]

Hans Zwart

Department of Applied Mathematics

University of Twente

P.O. Box 217, 7500 AE Enschede

The Netherlands

[email protected]

1 DESCRIPTION OF THE PROBLEM

We consider the abstract system

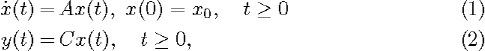
on a Hilbert space H. Here A is the infinitesimal generator of an exponentially stable C0-semigroup and by the solution of (1) we mean which is the weak solution. If C is a bounded linear operator from H to a second Hilbert space Y , then it is straightforward to see that y(.) in (2) is well-defined and continuous. However, in many PDE’s, rewritten in the form (1)-(2), C is only a bounded operator from D(A) to Y (D(A) denotes the domain of A), although the output is a well-defined (locally) square integrable function. In the following, C will always be a bounded operator from D(A) to Y . Note that D(A) is a dense subset of H. If the output is locally square integrable, then C is called an admissible observation operator, see Weiss [11]. It is not hard to see that since the C0-semigroup is exponentially stable, the output is locally square integrable if and only if it is square integrable. Using the uniform boundedness theorem, we see that the observation operator C is admissible if and only if there exists a constant L > 0 such that

Assuming that the observation operator C is admissible, system (1)-(2) is said to be exactly observable if there is a bounded mapping from the output trajectory to the initial condition, i.e., there exists a constant l > 0 such that

Often the emphasis is on exact observability on a finite interval, which means that the integral in (4) is over [0, ] for some > 0. However, for exponentially stable semigroups, both notions are equivalent, i.e., if (4) holds and the system is exponentially stable, then there exists a > 0 such that the system is exactly observable on [0, ].

There is a strong need for easy verifiable equivalent conditions for exact ob-servability. Based on the observability conjecture by Russell and Weiss [9] we now conjecture the following:

Conjecture Let A be the infinitesimal generator of an exponentially stable C0-semigroup on a Hilbert space H and let C be an admissible observation operator. Then system (1)-(2) is exactly observable if and only if

2 MOTIVATION AND HISTORY OF THE CONJECTURE

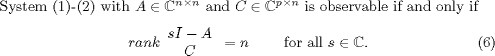
This is known as the Hautus test, due to Hautus [2] and Popov [8]. If A is a stable matrix, then (6) is equivalent to condition (C2). Although there are some generalizations of the Hautus test to delay differential equations (see, e.g., Klamka [6] and the references therein) the full generalization of the Hautus test to infinite-dimensional linear systems is still an open problem.

It is not hard to see that if (1)-(2) is exactly observable, then the semigroup is similar to a contraction, see Grabowski and Callier [1] and Levan [7].

Condition (C2) was found by Russell and Weiss [9]: they showed that this condition is necessary for exact observability, and, under the extra assumption that A is bounded, they showed that this condition also is sufficient.

Without the explicit usage of condition (C1) it was shown that condition (C2) implies exact observability if

Recently, we showed that (C2) is not sufficient in general, [4]. The C0semigroup in our counterexample is an analytic semigroup, which is not similar to a contraction semigroup. The output space in the example is just the complex plane .

3 AVAILABLE RESULTS AND CLOSING REMARKS

In order to prove the conjecture it is sufficient to show that for exponentially stable contraction semigroups condition (C2) implies exact observability.

It is well-known that system (1)-(2) is exactly observable if and only if there exists a bounded operator L that is positive and boundedly invertible and satisfies the Lyapunov equation

From the admissibility of C and the exponential stability of the semigroup, one easily obtains that equation (7) has a unique (non-negative) solution.

Russell and Weiss [9] showed that Condition (C2) implies that this solution has zero kernel. Thus the Lyapunov equation (2) could be a starting point for a proof of the conjecture.

We have stated our conjecture for infinite-dimensional output spaces. However, it could be that it only holds for finite-dimensional output spaces.

If the output space Y is one-dimensional one could try to prove the conjecture using powerful tools like the Sz.-Nagy-Foias model theorem (see [10]). This tool was quite useful in the context of admissibility conditions for contraction semigroups [3]. Based on this observation, it would be of great interest to check our conjecture for the right shift semigroup on .

We believe that exponential stability is not essential in our conjecture, and can be replaced by strong stability and infinite-time admissibility, see [5].

Note that our conjecture is also related to the left-invertibility of semigroups, see [1] and [4] for more details.

BIBLIOGRAPHY

[1] P. Grabowski and F. M. Callier, “Admissible observation operators, semigroup criteria of admissibility, ” Integral Equation and Operator Theory, 25:182–198, 1996.

[2] M. L. J. Hautus, “Controllability and observability conditions for linear autonomous systems, ” Ned. Akad. Wetenschappen, Proc. Ser. A, 72:443–448, 1969.

[3] B. Jacob and J. R. Partington, “The Weiss conjecture on admissibility of observation operators for contraction semigroups, ” Integral Equations and Operator Theory, 40(2):231-243, 2001.

[4] B. Jacob and H. Zwart, “Disproof of two conjectures of George Weiss, ” Memorandum 1546, Faculty of Mathematical Sciences, University of Twente, 2000. Available at http://www.math.utwente.nl/publications/

[5] B. Jacob and H. Zwart, “Exact observability of diagonal systems with a finite-dimensional output operator, ” Systems & Control Letters, 43(2):101-109, 2001.

[6] J. Klamka, Controllability of Dynamical Systems, Kluwer Academic Publisher, 1991.

[7] N. Levan, “The left-shift semigroup approach to stability of distributed systems, ” J. Math. Ana. Appl., 152: 354–367, 1990.

[8] V. M. Popov, Hyperstability of Control Systems. Editura Academiei, Bucharest, 1966 (in Romanian); English trans. by Springer Verlag, Berlin, 1973.

[9] D. L. Russell and G. Weiss, “A general necessary condition for exact observability, ” SIAM J. Control Optim., 32(1):1–23, 1994.

[10] B. Sz.-Nagy and C. Foias, Harmonic analysis of operators on Hilbert spaces, Amsterdam-London: North-Holland Publishing Company. XIII, 1970.

[11] G. Weiss, “Admissible observation operators for linear semigroups, ” Israel Journal of Mathematics, 65:17–43, 1989.

[12] Q. Zhou and M. Yamamoto, “Hautus condition on the exact controllability of conservative systems, ” Int. J. Control, 67(3):371–379, 1997.