Appendix 1B: Retarded Potentials and Review of Potentials for the Static Cases

Search in book...
Toggle Font Controls
Create new playlist

Name your new playlist

Playlist description (optional)
Sign In

Email address

Password

Forgot Password?

or

Continue with Facebook

Continue with Google
Sign Up

Full Name

Email address

Confirm Email Address

Password

or

Continue with Facebook

Continue with Google

1B.1Electrostatics

The basic equation is

(1B.1)

\nabla \times E = 0 .

$\nabla \times E = 0 .$

It is known that the curl of the gradient of any scalar is zero, that is,

(1B.2)

\nabla \times [gradient of any scalar] \equiv 0.

$\nabla \times [gradient of any scalar] \equiv 0.$

Therefore, E can be expressed as the gradient of a scalar ψ (electrostatic potential).

(1B.3)

E = - \nabla ψ,

$E = - \nabla ψ,$

where ψ satisfies Poisson’s equation

(1B.4)

\nabla^{2} ψ = - \frac{ρ_{v}}{ε} .

$\nabla^{2} ψ = - \frac{ρ_{v}}{ε} .$

The solution of Equation 1B.4 at an arbitrary point P is given by

(1B.5)

ψ (r) = \underset{v^{'}}{∭} \frac{ρ_{v} (r^{'})}{4 π ε |r - r^{'}|} d v^{'},

$ψ (r) = \underset{v^{'}}{∭} \frac{ρ_{v} (r^{'})}{4 π ε |r - r^{'}|} d v^{'},$

where the volume charge density ρ_v exists over volume v′ as shown in Figure 1B.1.

1B.2Magnetostatics

The basic equation is

(1B.6)

\nabla \cdot B = 0.

$\nabla \cdot B = 0.$

It is known that the divergence of the curl of any vector is zero, that is,

(1B.7)

\nabla \cdot [curl of any vector] \equiv 0 .

$\nabla \cdot [curl of any vector] \equiv 0 .$

Therefore, B can be expressed as the curl of a vector A as

(1B.8)

B = \nabla \times A .

$B = \nabla \times A .$

The magnetic vector potential A satisfies the vector Poisson’s equation

(1B.9)

\nabla^{2} A = - μ J .

$\nabla^{2} A = - μ J .$

In deriving Equation 1B.9, we use Ampere’s law ∇ × H = J and choose ∇ · A = 0. The solution of Equation 1B.9 at an arbitrary point P is given by

(1B.10)

A (r) = \underset{v^{'}}{∭} \frac{μ J (r^{'})}{4 π |r - r^{'}|} d v^{'}

$A (r) = \underset{v^{'}}{∭} \frac{μ J (r^{'})}{4 π |r - r^{'}|} d v^{'}$

and

(1B.11)

\nabla \cdot A = 0 .

$\nabla \cdot A = 0 .$

1B.3Time-Varying Case

We know

(1B.12)

\nabla \cdot B = 0

$\nabla \cdot B = 0$

and

(1B.13)

B = \nabla \times A,

$B = \nabla \times A,$

but

(1B.14)

\nabla \times E = - \frac{\partial B}{\partial t} .

$\nabla \times E = - \frac{\partial B}{\partial t} .$

From Equations 1B.13 and 1B.14, we obtain

(1B.15)

\nabla \times E + \frac{\partial}{\partial t} (\nabla \times A) = 0,

$\nabla \times E + \frac{\partial}{\partial t} (\nabla \times A) = 0,$

(1B.16)

\nabla \times [E + \frac{\partial A}{\partial t}] = 0.

$\nabla \times [E + \frac{\partial A}{\partial t}] = 0.$

Therefore, from Equation 1B.2, E + (∂A/∂t) can be expressed as the gradient of a scalar ψ (electric potential)

(1B.17)

E = - \nabla ψ - \frac{\partial A}{\partial t} .

$E = - \nabla ψ - \frac{\partial A}{\partial t} .$

Now, we shall derive the equations satisfied by A and ψ and we would like to have these equations without the E and H fields in them. We want the equations for the time varying case corresponding to Equations 1B.4 and 1B.9. Let us start with

(1B.18)

\nabla \cdot D = ρ_{v} .

$\nabla \cdot D = ρ_{v} .$

Using Equations 1B.18, 1B.17, and the relation D = εE, we obtain

(1B.19)

\nabla \cdot ε E = ε \nabla \cdot E = ε \nabla \cdot [- \nabla ψ - \frac{\partial A}{\partial t}] = ρ_{v},

$\nabla \cdot ε E = ε \nabla \cdot E = ε \nabla \cdot [- \nabla ψ - \frac{\partial A}{\partial t}] = ρ_{v},$

(1B.20)

\nabla^{2} ψ + \frac{\partial}{\partial t} \nabla \cdot A = - \frac{ρ_{v}}{ε} .

$\nabla^{2} ψ + \frac{\partial}{\partial t} \nabla \cdot A = - \frac{ρ_{v}}{ε} .$

Similarly, start from the equation

(1B.21)

\nabla \times H = J + \frac{\partial D}{\partial t},

$\nabla \times H = J + \frac{\partial D}{\partial t},$

(1B.22)

\nabla \times B = μ J + μ \frac{\partial D}{\partial t} = μ J + ε μ \frac{\partial E}{\partial t} = μ J + ε μ \frac{\partial}{\partial t} (- \nabla ψ - \frac{\partial A}{\partial t}) .

$\nabla \times B = μ J + μ \frac{\partial D}{\partial t} = μ J + ε μ \frac{\partial E}{\partial t} = μ J + ε μ \frac{\partial}{\partial t} (- \nabla ψ - \frac{\partial A}{\partial t}) .$

Since

(1B.23)

\nabla \times B = \nabla \times (\nabla \times A) = \nabla (\nabla \cdot A) - \nabla^{2} A,

$\nabla \times B = \nabla \times (\nabla \times A) = \nabla (\nabla \cdot A) - \nabla^{2} A,$

(1B.24)

\nabla (\nabla \cdot A) - \nabla^{2} A = μ J - ε μ \frac{\partial^{2} A}{\partial t^{2}} - ε μ \nabla (\frac{\partial ψ}{\partial t}),

$\nabla (\nabla \cdot A) - \nabla^{2} A = μ J - ε μ \frac{\partial^{2} A}{\partial t^{2}} - ε μ \nabla (\frac{\partial ψ}{\partial t}),$

(1B.25)

\nabla^{2} A - ε μ \frac{\partial^{2} A}{\partial t^{2}} - \nabla (\nabla \cdot A + ε μ \frac{\partial ψ}{\partial t}) = - μ J .

$\nabla^{2} A - ε μ \frac{\partial^{2} A}{\partial t^{2}} - \nabla (\nabla \cdot A + ε μ \frac{\partial ψ}{\partial t}) = - μ J .$

Equations 1B.20 and 1B.25 are coupled, that is, in each of these equations, both variables ψ and A appear. In the static case, we had uncoupled equations for ψ and A (Equations 1B.4 and 1B.9). A vector is uniquely defined only if its curl and also its divergence are specified everywhere. In the static case, A is made unique by specifying its curl as B, that is, ∇ × A = B, and its divergence as zero, that is, ∇ · A = 0. In the time-varying case, we have specified curl of A as B, but we have not specified its divergence. It is seen from Equation 1B.25 that if we specify (Lorentz condition)

(1B.26)

\nabla \cdot A + ε μ \frac{\partial ψ}{\partial t} = 0,

$\nabla \cdot A + ε μ \frac{\partial ψ}{\partial t} = 0,$

then Equation 1B.25 becomes uncoupled (ψ does not appear in the equation):

(1B.27)

\nabla^{2} A - ε μ \frac{\partial^{2} A}{\partial t^{2}} = - μ J .

$\nabla^{2} A - ε μ \frac{\partial^{2} A}{\partial t^{2}} = - μ J .$

Furthermore, if we substitute Equation 1B.26 into Equation 1B.20 to eliminate A, then we obtain

(1B.28)

\nabla^{2} ψ + \frac{\partial}{\partial t} (- ε μ \frac{\partial ψ}{\partial t}) = - \frac{ρ_{v}}{ε}

$\nabla^{2} ψ + \frac{\partial}{\partial t} (- ε μ \frac{\partial ψ}{\partial t}) = - \frac{ρ_{v}}{ε}$

that is,

(1B.29)

\nabla^{2} ψ - ε μ \frac{\partial^{2} ψ}{\partial t^{2}} = - \frac{ρ_{v}}{ε},

$\nabla^{2} ψ - ε μ \frac{\partial^{2} ψ}{\partial t^{2}} = - \frac{ρ_{v}}{ε},$

which is also an uncoupled equation.

Equations 1B.27 and 1B.29 are called wave equations. Although Poisson’s equation governs the static cases, time-varying phenomena are governed by the wave equation.

In free space, 1/ε₀μ₀ = (3 × 10⁸)² = c², where c is the velocity of light. Note that c is large but finite. Equation 1B.29 for free space is

(1B.30)

\nabla^{2} ψ - \frac{1}{c^{2}} \frac{\partial^{2} ψ}{\partial t^{2}} = - \frac{ρ_{v}}{ε} .

$\nabla^{2} ψ - \frac{1}{c^{2}} \frac{\partial^{2} ψ}{\partial t^{2}} = - \frac{ρ_{v}}{ε} .$

This tends to Equation 1B.4 if 1/c² → 0, that is, c → ∞. The implication of this statement will be explained later. Consider a charge Q at point A as shown in Figure 1B.2. A comparison between the static and dynamic cases is summarized in Table 1B.1.

FIGURE 1B.2
Point charge at point A for static and dynamic case comparison.

TABLE 1B.1
Comparison of Static and Dynamic Cases for a Point Charge

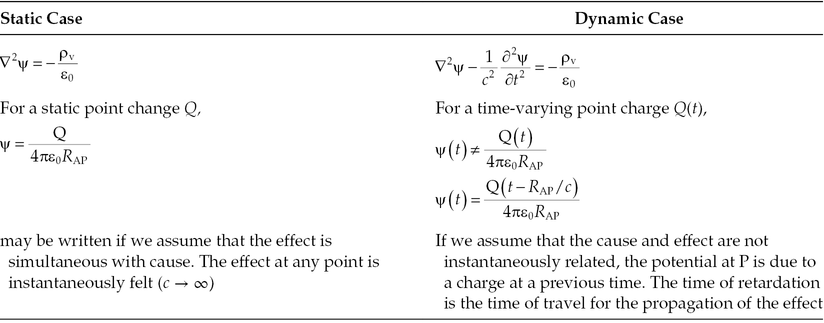
For a volume charge source of density ρ_v, the solution is

(1B.31)

ψ (r, t) = \underset{v^{'}}{∭} \frac{ρ_{v} (t - R_{AP} / c)}{4 π ε R_{AP}} d v^{'} = \underset{v^{'}}{∭} \frac{[ρ_{v}]}{4 π ε R_{AP}} d v^{'},

$ψ (r, t) = \underset{v^{'}}{∭} \frac{ρ_{v} (t - R_{AP} / c)}{4 π ε R_{AP}} d v^{'} = \underset{v^{'}}{∭} \frac{[ρ_{v}]}{4 π ε R_{AP}} d v^{'},$

where

[ρ_{v}] = ρ_{v} (t - \frac{R_{AP}}{c}) .

$[ρ_{v}] = ρ_{v} (t - \frac{R_{AP}}{c}) .$

is the charge density at the retarded time.

Example 1B.1

Let a current filament be short (length = ℓ) and carry a current I = I₀ cos(ωt) as shown in Figure 1B.3.

Then

(1B.32)

A (r, t) = μ \underset{v^{'}}{∭} \frac{[J]}{4 π r} d v^{'} = μ_{0} \int \frac{[I]}{4 π r} d l^{'} .

$A (r, t) = μ \underset{v^{'}}{∭} \frac{[J]}{4 π r} d v^{'} = μ_{0} \int \frac{[I]}{4 π r} d l^{'} .$

For I(t) = I₀ cos ωt,

(1B.33)

[I] = I_{0} cos ω (t - \frac{r}{c}) = Re (I_{0} e^{j ω t} e^{- j ω r / c}),

$[I] = I_{0} cos ω (t - \frac{r}{c}) = Re (I_{0} e^{j ω t} e^{- j ω r / c}),$

(1B.34)

A (r, t) = Re [μ_{0} I_{0} \int \frac{e^{j ω t} e^{- j ω r / c}}{4 π r}] d z^{'} \hat{z} ​,

$A (r, t) = Re [μ_{0} I_{0} \int \frac{e^{j ω t} e^{- j ω r / c}}{4 π r}] d z^{'} \hat{z} ,$

(1B.35)

A (r, t) = Re [μ_{0} I_{0} e^{j ω t} \int \frac{e^{- j ω r / c}}{4 π r} d z^{'}] \hat{z} .

$A (r, t) = Re [μ_{0} I_{0} e^{j ω t} \int \frac{e^{- j ω r / c}}{4 π r} d z^{'}] \hat{z} .$

If ℓ ≪ λ,

(1B.36)

A_{z} (r, t) \approx Re [\frac{μ_{0} I_{0} l e^{j ω t}}{4 π r} e^{- j ω r / c}] .

$A_{z} (r, t) \approx Re [\frac{μ_{0} I_{0} l e^{j ω t}}{4 π r} e^{- j ω r / c}] .$

The phasor Ã_z is given by

{\tilde{A}}_{z} (r) = \frac{μ_{0} I_{0} l}{4 π r} e^{- j ω r / c} .

${\tilde{A}}_{z} (r) = \frac{μ_{0} I_{0} l}{4 π r} e^{- j ω r / c} .$

1B.4One-Dimensional Solution for the Wave Equation

In explaining the retardation effect, we assumed the solution of

(1B.37)

\nabla^{^{2}} ψ - \frac{1}{c^{2}} \frac{\partial^{2} ψ}{\partial t^{2}} = 0,

$\nabla^{^{2}} ψ - \frac{1}{c^{2}} \frac{\partial^{2} ψ}{\partial t^{2}} = 0,$

is a wave propagating with the velocity c. Let us solve Equation 1B.37 for the one-dimensional case, ψ = ψ (z, t), that is,

(1B.38)

\frac{\partial^{2} ψ}{\partial z^{2}} - \frac{1}{c^{2}} \frac{\partial^{2} ψ}{\partial t^{2}} = 0.

$\frac{\partial^{2} ψ}{\partial z^{2}} - \frac{1}{c^{2}} \frac{\partial^{2} ψ}{\partial t^{2}} = 0.$

Let the Laplace transform of ψ be F and it may be written as

(1B.39)

L [ψ] = \int_{0}^{\infty} ψ e^{- s t} d t = F .

$L [ψ] = \int_{0}^{\infty} ψ e^{- s t} d t = F .$

Transforming Equation 1B.37, we obtain

(1B.40)

\frac{d^{2} F (z, s)}{d z^{2}} - \frac{1}{c^{2}} [s^{2} F - s ψ (z, 0) - ψ^{'} (z, 0)] = 0.

$\frac{d^{2} F (z, s)}{d z^{2}} - \frac{1}{c^{2}} [s^{2} F - s ψ (z, 0) - ψ^{'} (z, 0)] = 0.$

If the initial conditions are zero, then

(1B.41)

\frac{d^{2} F}{d z^{2}} - \frac{s^{2}}{c^{2}} F = 0.

$\frac{d^{2} F}{d z^{2}} - \frac{s^{2}}{c^{2}} F = 0.$

Then

(1B.42)

F = F_{1} (s) e^{(- s / c) z} + F_{2} (s) e^{(+ s / c) z} .

$F = F_{1} (s) e^{(- s / c) z} + F_{2} (s) e^{(+ s / c) z} .$

If $f_{1} (t) = L^{- 1} {F_{1} (s)}$ $f_{1} (t) = L^{- 1} {F_{1} (s)}$ and $f_{2} (t) = L^{- 1} {F_{2} (s)}$ $f_{2} (t) = L^{- 1} {F_{2} (s)}$ , then by using shifting theorem, that is, $L [f (t - a)] = e^{- a s} F (s)$ $L [f (t - a)] = e^{- a s} F (s)$ , we get

(1B.43)

ψ (z, t) = f_{1} (t - \frac{z}{c}) + f_{2} (t + \frac{z}{c}) .

$ψ (z, t) = f_{1} (t - \frac{z}{c}) + f_{2} (t + \frac{z}{c}) .$

Equation 1B.43 is the solution to Equation 1B.38.

Consider the case where f₁(t) = δ(t). Then f₁(t − z/c) is the pulse f₁(t) moving with a velocity c in the +z direction as depicted in Figure 1B.4.

FIGURE 1B.4
f₁(t − z/c) is the pulse f₁(t) moving with a velocity v = c in the +z-direction.

..................Content has been hidden....................

You can't read the all page of ebook, please click here login for view all page.

Table of Contents for Appendix 1B: Retarded Potentials and Review of Potentials for the Static Cases

Create new playlist

Sign In

Sign Up

1B.1Electrostatics

1B.2Magnetostatics

1B.3Time-Varying Case

1B.4One-Dimensional Solution for the Wave Equation

Table of Contents for
Appendix 1B: Retarded Potentials and Review of Potentials for the Static Cases