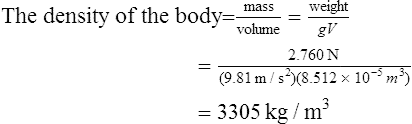Pressure in fluids
Publisher Summary
This chapter discusses pressure in fluids. The pressure acting on a surface is defined as the perpendicular force per unit area of the surface. The unit of pressure is the pascal, (Pa), where one pascal is equal to one Newton per square meter. A fluid is either a liquid or a gas. There are four basic factors governing the pressure within the fluids, including the pressure at a given depth in a fluid is equal in alldirections, the pressure at a given depth in a fluid is independent of the shape of the container in which the fluid is held, pressure acts at right angles to the surface containing the fluid, and when a pressure is applied to a fluid, this pressure is transmitted equally in all directions.
1. The pressure acting on a surface is defined as the perpendicular force per unit area of surface. The unit of pressure is the pascal, (Pa), where 1 pascal is equal to 1 newton per square metre. Thus
where F is the force in newtons acting at right angles to a surface of area A square metres.
When a force of 20 N acts uniformly over, and perpendicular to an area of 4 m2, then the pressure on the area, p, is given by:
2. A fluid is either a liquid or a gas and there are four basic factors governing the pressure within fluids.
(a) The pressure at a given depth in a fluid is equal in all directions, see Figure 44.1 (a).

(b) The pressure at a given depth in a fluid is independent of the shape of the container in which the fluid is held. In Figure 44.1 (b), the pressure at X is the same as the pressure at Y.
(c) Pressure acts at right angles to the surface containing the fluid. In Figure 44.1(c), the pressure at points A to F all act at right angles to the container.
(d) When a pressure is applied to a fluid, this pressure is transmitted equally in all directions. In Figure 44.1 (d), if the mass of the fluid is neglected, the pressures at points A to D are all the same.
3. The pressure, p, at any point in a fluid depends on three factors:
(a) the density of the fluid, ρ in kg/m3;
(b) the gravitational acceleration, g, taken as 9.8 m/s2; and
(c) the height of fluid vertically above the point, h metres.
The relationship connecting these quantities is: p = ρgh pascals.
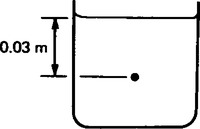
When the container shown in Figure 44.2 is filled with water of density 1000 kg/m3, the pressure due to the water at a depth of 0.03 m below the surface is given by:

4. The air above the earth’s surface is a fluid, having a density, ρ, which varies from approximately 1.225 kg/m3 at sea level to zero in outer space. Since p = ρgh, where height h is several thousands of metres, the air exerts a pressure on all points on the earth’s surface. This pressure, called atmospheric pressure, has a value of approximately 100 kilopascals. Two terms are commonly used when measuring pressures:
(a) absolute pressure, meaning the pressure above that of an absolute vacuum (i.e. zero pressure), and
(b) gauge pressure, meaning the pressure above that normally present due to the atmosphere. Thus:
absolute pressure = atmospheric pressure + gauge pressure
Thus, a gauge pressure of 50 kPa is equivalent to an absolute pressure of (100+50) kPa, i.e. 150 kPa, since the atmospheric pressure is approximately 100 kPa.
Another unit of pressure, used in particular for atmospheric pressures, is the bar.
5. There are various ways of measuring pressure, and these include by:
U-tube manometer
6. A manometer is a device used for measuring relatively small pressures, either above or below atmospheric pressure. A simple U-tube manometer is shown in Figure 44.3. Pressure p acting in, say a gas main, pushes the liquid in the U-tube until equilibrium is obtained. At equilibrium: pressure in gas main, p = (atmospheric pressure, pa) + (pressure due to the column of liquid, ρgh) i.e., p = pa + ρgh.

Thus, for example, if the atmospheric pressure, pa is 101 kPa, the liquid in the U-tube is water of density 1000 kg/m3 and height, h is 300 mm, then

The gauge pressure of the gas is 2.94 kPa.
By filling the U-tube with a more dense liquid, say mercury having a density of 13 600 kg/m3, for a given height of U-tube, the pressure which can be measured is increased by a factor of 13.6.
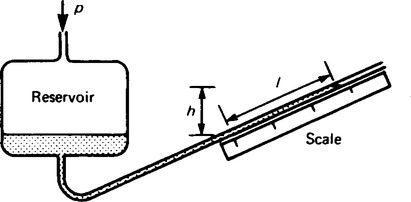
By inclining one limb of the U-tube, as shown in Figure 44.4, greater sensitivity is achieved, that is, there is a larger movement of the liquid for a given change in pressure when compared with a U-tube having vertical limbs. An inclined manometer normally has a reservoir of sufficient area to give virtually a constant level in the left-hand limb. From Figure 44.4, it can be seen that pressure p applied to the reservoir causes a scale change of ‘l’ in the inclined manometer compared with ‘h’ in a normal manometer.
Simple barometer
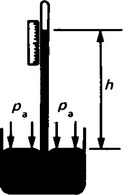
7. A simple barometer consists of a length of glass tubing, approximately 800 mm long and sealed at one end, which is filled with mercury and then inverted in a beaker of mercury, as shown in Figure 44.5. At equilibrium, the atmospheric pressure, pa, is tending to force the mercury up the tube, whilst the force due to the column of mercury is tending to force the mercury out of the tube, i.e. pa = ρgh. As the atmospheric pressure varies, height h varies, giving an indication on the scale of the atmospheric pressure.
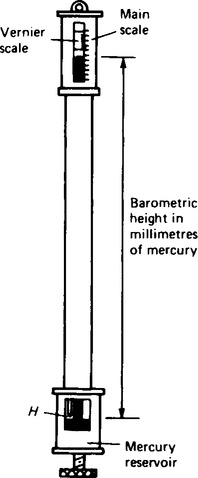
Fortin barometer
8. The Fortin barometer is as shown in Figure 44.6. Mercury is contained in a leather bag at the base of the mercury reservoir, and height, H, of the mercury in the reservoir can be adjusted using the screw at the base of the barometer to depress or relase the leather bag. To measure the atmospheric pressure, the screw is adjusted until the pointer at H is just touching the surface of the mercury and the height of the mercury column is then read using the main and vernier scales. The measurement of atmospheric pressure using a Fortin barometer is achieved much more accurately than by using a simple barometer.
Bourdon pressure gauge
9. The main components of a Bourdon pressure gauge are shown in Figure 44.7. When pressure, p, is applied to the curved phosphor bronze tube, which is sealed at A, it tends to straighten, moving A to the right. Conversely, a decrease in pressure below that due to the atmosphere moves point A to the left. When A moves to the right, B moves to the left, rotating the pointer across a scale. This type of pressure gauge can be used to measure large pressures and pressures both above and below atmospheric pressure. The Bourdon pressure gauge indicates gauge pressure and is very widely used in industry for pressure measurements.

Hydrostatic pressure
10. The pressure at the base of the tank shown in Figure 44.8(a) is:
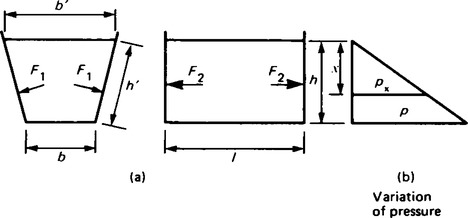
where w is the specific weight, i.e. the weight per unit volume, its unit being N/m3.
The pressure increases to this value uniformly from zero at the free surface. The pressure variation is shown in Figure 44.8(b).
At any intermediate depth x the pressure is
It may be shown that the average pressure on any wetted plane surface is the pressure at the centroid, the centre of area. The sloping sides of the tank of Figure 44.8 are rectangular and therefore the average pressure on them is the pressure at half depth:
The force on a sloping side is the product of this average pressure and the area of the sloping side:
where h’ is the slant height. The pressure and consequently the forces F1 are at right angles to the sloping sides as shown in Figure 44.8.
The average pressure on the vertical trapezoidal ends of the tank is not the pressure at half depth. This is because the centroid of an end is not at half depth — it is rather higher. The depth of the centroid is given by:
(see Figure 44.8)
The average pressure on an end is therefore:
The forces F2 on the vertical trapezoidal ends of the tank are horizontal forces given by the product of this average pressure and the area of the trapezium,
The force on the base of the tank is (ρgh)x (area of base) = ρhlb = whlb
For a tank with vertical sides this is the weight of liquid in the tank.
11. In any vessel containing homogeneous liquid at rest and in continuous contact, the pressure must be the same at all points at the same level. In a U-tube, as shown in Figure 44.9, with the liquid in the lower part at rest, the pressure must be the same on both sides for all levels up to X1X2. The pressure at X1, however, is greater than the pressure at Y1 by an amount p1 = w1h = ρ1gh, where w1,ρ1, ρ1 are the specific weight and density respectively of the liquid, or gas, between X1 and Y1.

Similarly, the pressure at X2 is greater than the pressure at Y2 by an amount given by p2 = w2h = ρ2gh, where w2, ρ2 are the specific weight and density respectively of the liquid in the bottom of the U-tube.
For practical reasons ρ2 must be greater than ρ1 and the pressure at Y1 will exceed that at Y2 by
If the upper limits of the U-tube contain air or any other gas or gas mixture w1 and ρ1 can reasonably be ignored, giving
If the upper limbs contain a lighter liquid, then the pressure difference may be expressed as
A common arrangement is mercury and water, in which case d is the relative density of mercury approximately 13.6. This gives:
ρ and w being respectively, the density and specific weight of water. The pressure difference at Z1Z2 will be the same as at Y1Y2 if both limbs contain the same liquid between these levels. This follows from the fact that the pressure increase from Z1 to Y1 is the same as the increase from Z2 to Y2.
Archimedes’ principle
12. If a solid body is immersed in a liquid, the apparent loss of weight is equal to the weight of liquid displaced. If V is the volume of the body below the surface of the liquid, then the apparent loss of weight is W = Vw = Vρg, where w, ρ are respectively the specific weight and density of the liquid.
If ρ is known and W obtained from a simple experiment, V can be calculated. Hence the density of the solid body can be calculated. If V is known and W obtained, ρ can be calculated.
If a body floats on the surface of a liquid all of its weight appears to have been lost. The weight of liquid displaced is equal to the weight of the floating body.
For example, a body weighs 2.760 N in air and 1.925 N when completely immersed in water of density 1000 kg/m3.
Hence the apparent loss of weight is
This is the weight of water displaced, i.e. Vρg, where V is the volume of the body and ρ is the density of water.
Thus 0.835 N = (V) (1000 kg/m3) (9.81 m/s2)