3.2 Integral Domains and Fields
1.
a. 1, − 4.
c. 0, 1.
3. If e2 = e in a domain, then e(1 − e) = 0 so e = 0 or e = 1. If an = 0, n ≥ 1, then a = 0. For if a ≠ 0 then aan−1 = 0 gives an−1 = 0, . . ., and eventually a = 0, a contradiction.
5. Let 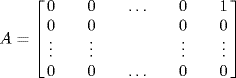 . Then A2 = 0 but A ≠ 0. So Mn(R) is not a domain.
. Then A2 = 0 but A ≠ 0. So Mn(R) is not a domain.
 . Then A2 = 0 but A ≠ 0. So Mn(R) is not a domain.
. Then A2 = 0 but A ≠ 0. So Mn(R) is not a domain.
7. If ab = 0 then (ba)2 = b(ab)a = 0, so ba = 0 by hypothesis.
9. In 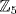 , 12 + 22 = 0; in
, 12 + 22 = 0; in 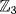 , let a2 + b2 = 0. If either a = 0 or b = 0, the other is 0 (x2 = 0 ⇒ x = 0 in a field). If a ≠ 0, b ≠ 0 then a, b
, let a2 + b2 = 0. If either a = 0 or b = 0, the other is 0 (x2 = 0 ⇒ x = 0 in a field). If a ≠ 0, b ≠ 0 then a, b  {1, 2}. But 12 + 12 ≠ 0, 12 + 22 = 2 ≠ 0, 22 + 22 = 3 ≠ 0.
{1, 2}. But 12 + 12 ≠ 0, 12 + 22 = 2 ≠ 0, 22 + 22 = 3 ≠ 0.
11. The group F∗ = F {0} has order q − 1 so aq−1 = 1 for all a ≠ 0 (by Lagrange's theorem). Thus aq = a if a ≠ 0; this also holds if a = 0.
13. Since |F| = p is prime, (F, +) is cyclic and is generated by 1 (or any nonzero element) by Lagrange's theorem. Hence the map  given by
given by  is an isomorphism of additive groups. It is a ring isomorphism because (km)1 = (k1)(m1) in F.
is an isomorphism of additive groups. It is a ring isomorphism because (km)1 = (k1)(m1) in F.
15. Let Z denote the center of a division ring D. If 0 ≠ z  Z we have zd = 1 = dz for some d
Z we have zd = 1 = dz for some d D ; we must show that d
D ; we must show that d  Z. Given r
Z. Given r  D we have (rz)d = r(zd) = r and (zr)d = d(zr) = (dz)r = r. Hence (rz)d = (zr)d so rz = zr.
D we have (rz)d = r(zd) = r and (zr)d = d(zr) = (dz)r = r. Hence (rz)d = (zr)d so rz = zr.
16.
a. If K is a subfield, let 0 ≠ a  K. If a′ is the inverse of a in K then aa′ = 1. But aa−1 = 1 in F so a′ = a−1 by cancellation. Hence a−1
K. If a′ is the inverse of a in K then aa′ = 1. But aa−1 = 1 in F so a′ = a−1 by cancellation. Hence a−1  K. Conversely, if the condition holds, then the inverse of a in F serves as its inverse in K.
K. Conversely, if the condition holds, then the inverse of a in F serves as its inverse in K.
c. Here |K| = 1, 2, 4, 8, 16, and |K∗| divides 15 so |K∗| = 1, 3, 5, 15. Thus |K| = 2, 4, 8, 16. The common values are |K| = 2, 4, 16. So K = {0, 1}, |K| = 4, or K = F.
17. It is clearly a subring of  . If a = r + si ≠ 0 then
. If a = r + si ≠ 0 then 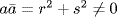 (one of r ≠ 0 or s ≠ 0). Since
(one of r ≠ 0 or s ≠ 0). Since 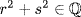 we have
we have 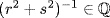 too, so
too, so
![]()
18.
a. It is clearly a subring of 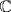 . As in Example 4, if
. As in Example 4, if 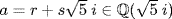 define
define 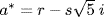 and N(a) = aa∗ = r2 + 5s2. If a ≠ 0 then N(a) ≠ 0 in
and N(a) = aa∗ = r2 + 5s2. If a ≠ 0 then N(a) ≠ 0 in 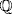 so
so  . Thus
. Thus 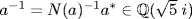 .
.
19. 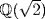 is a subfield of
is a subfield of 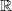 by Example 4, and it contains
by Example 4, and it contains  If F is any subfield of
If F is any subfield of 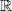 then
then 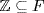 (because
(because  ), and hence
), and hence 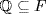 (because
(because 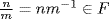 for all n, m ≠ 0 in
for all n, m ≠ 0 in 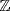 ). If also
). If also 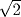
 F, this means
F, this means  for all
for all 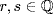 . Thus
. Thus 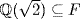 .
.
21.
a. 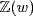 is a subring of
is a subring of 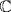 , and so is an integral domain by Example 3.
, and so is an integral domain by Example 3.
c. r∗∗ = r is obvious. If r = n + mw and 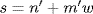 then
then
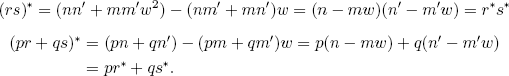
e. If r is a unit in 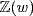 then rr−1 = 1 in
then rr−1 = 1 in  so, by (d) N(r)N(r−1) = N(1) = 1 in
so, by (d) N(r)N(r−1) = N(1) = 1 in 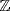 . It follows that N(r) = ± 1. Conversely, if N(r) = ± 1 then rr∗ = ± 1 so r−1 = ± r∗.
. It follows that N(r) = ± 1. Conversely, if N(r) = ± 1 then rr∗ = ± 1 so r−1 = ± r∗.
23. Let R = {r1, r2, . . ., rn} be a domain with n elements. If 0 ≠ a  R, then the elements of aR = {ar1, ar2, . . ., arn} are distinct (because a can be cancelled) so |aR| = n = |R|. Hence aR = R so ab = 1 for some b
R, then the elements of aR = {ar1, ar2, . . ., arn} are distinct (because a can be cancelled) so |aR| = n = |R|. Hence aR = R so ab = 1 for some b  R. Similarly Ra = {r1a, . . ., rna} = R so ca = 1 for some c
R. Similarly Ra = {r1a, . . ., rna} = R so ca = 1 for some c  R. Thus c = c(ab) = (ca)b = b, and this element is the inverse of a.
R. Thus c = c(ab) = (ca)b = b, and this element is the inverse of a.
24.
a.
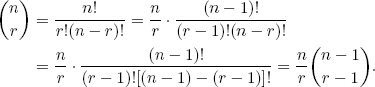
c. If F is a field of characteristic p, the map σ : F → F with σ(a) = ap satisfies σ(1) = 1, σ(ab) = (ab)p = apbp = σ(a) · σ(b), and, using (b), σ(a + b) = (a + b)p = ap + bp = σ(a) + σ(b). Hence σ is a homomorphism. We claim σ is one-to-one. Let a  ker σ, that is σ(a) = 0. Then ap = 0 so a = 0 because F is a field). Since F is finite, σ is also onto, and so is an automorphism of F.
ker σ, that is σ(a) = 0. Then ap = 0 so a = 0 because F is a field). Since F is finite, σ is also onto, and so is an automorphism of F.
25. Given σ : R → R, we have Q = {ru−1  r
r  R, 0 ≠ u
R, 0 ≠ u  R}. Since u ≠ 0 implies σ(u) ≠ 0, if
R}. Since u ≠ 0 implies σ(u) ≠ 0, if 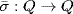 exists it must be given by
exists it must be given by
![]()
So define 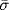 by this formula. If ru−1 = sv−1 then rv = su so
by this formula. If ru−1 = sv−1 then rv = su so
![]()
that is 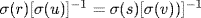 . Hence
. Hence 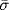 is well defined. Now
is well defined. Now

Similarly 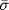 preserves multiplication. If
preserves multiplication. If 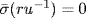 then σ(r)[σ(u)]−1 = 0 so σ(r) = 0. Hence r = 0, ru−1 = 0;
then σ(r)[σ(u)]−1 = 0 so σ(r) = 0. Hence r = 0, ru−1 = 0; 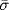 is one-to-one. Finally let sv−1
is one-to-one. Finally let sv−1  Q. Let r = σ−1(s) and
Q. Let r = σ−1(s) and 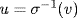 . Then u ≠ 0 and σ(ru−1) = σ(r) · [σ(u)]−1 = sv−1. Thus
. Then u ≠ 0 and σ(ru−1) = σ(r) · [σ(u)]−1 = sv−1. Thus  is onto.
is onto.
26.
a. If 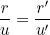 and
and  then ru′ = ur′ and sv′ = vs′, and so
then ru′ = ur′ and sv′ = vs′, and so
![]()
This shows 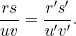
c.
27. Let R ⊆ F where F is a field, and let 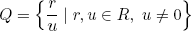 . Define σ : Q → F by
. Define σ : Q → F by 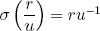 . Then
. Then  , so σ is well defined and one-to-one. We have
, so σ is well defined and one-to-one. We have

Hence R ≅ σ(R) = {ru−1  r, u
r, u  R, u ≠ 0} and σ(R) is a subring of F. It is a subfield because, if ru−1 ≠ 0 then r ≠ 0 so (ru−1)−1 = ur−1
R, u ≠ 0} and σ(R) is a subring of F. It is a subfield because, if ru−1 ≠ 0 then r ≠ 0 so (ru−1)−1 = ur−1  σ(R).
σ(R).
29.
a. If r = i and s = 1 in  , consider a = r + sω in
, consider a = r + sω in 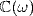 . Then aa∗ = r2 + s2 = 0, but a ≠ 0 and a∗ ≠ 0 in
. Then aa∗ = r2 + s2 = 0, but a ≠ 0 and a∗ ≠ 0 in 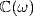 . Thus
. Thus 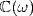 is not a field. In
is not a field. In 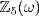 let a = 1 + 2ω. Then aa∗ = 12 + 22 = 0, and a ≠ 0 ≠ a∗. So
let a = 1 + 2ω. Then aa∗ = 12 + 22 = 0, and a ≠ 0 ≠ a∗. So 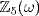 is not a field. However
is not a field. However 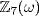 is a field. If a = r + si ≠ 0 in
is a field. If a = r + si ≠ 0 in 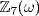 then aa∗ = r2 + s2 and it suffices to show r2 + s2 ≠ 0 in
then aa∗ = r2 + s2 and it suffices to show r2 + s2 ≠ 0 in  . Suppose r2 + s2 = 0. If r = 0 or s = 0 then a = 0, contrary to hypothesis. Thus r ≠ 0 ≠ s. Then 0 = s−1(r2 + s2) = (s−1r)2 + 1 so (s−1r)2 = − 1 in
. Suppose r2 + s2 = 0. If r = 0 or s = 0 then a = 0, contrary to hypothesis. Thus r ≠ 0 ≠ s. Then 0 = s−1(r2 + s2) = (s−1r)2 + 1 so (s−1r)2 = − 1 in 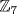 . This is not the case because 02 = 0, 12 = 1 = 62, 22 = 4 = 52, 32 = 2 = 42 in
. This is not the case because 02 = 0, 12 = 1 = 62, 22 = 4 = 52, 32 = 2 = 42 in 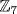 .
.
c. Let a = r + si ≠ 0 in 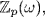 p ≡ 3 (mod 4). Then aa∗ = r2 + s2 so it suffices to show r2 + s2 ≠ 0 (than a−1 = (r2 + s2)−1a∗). Suppose r2 + s2 = 0. Now r ≠ 0 or s ≠ 0 (because a ≠ 0). If s ≠ 0 then 0 = s−2(r2 + s2) = (s−1r)2 + 1. Thus x = s−1r satisfies x2 = − 1 in
p ≡ 3 (mod 4). Then aa∗ = r2 + s2 so it suffices to show r2 + s2 ≠ 0 (than a−1 = (r2 + s2)−1a∗). Suppose r2 + s2 = 0. Now r ≠ 0 or s ≠ 0 (because a ≠ 0). If s ≠ 0 then 0 = s−2(r2 + s2) = (s−1r)2 + 1. Thus x = s−1r satisfies x2 = − 1 in  , contrary to the Corollary to Theorem 8 §1.3. Similarly if r ≠ 0.
, contrary to the Corollary to Theorem 8 §1.3. Similarly if r ≠ 0.
e. If e = r + sω and e2 = e then r2 − s2 = r and 2rs = s. If s = 0 then r2 = r; r = 0, 1 ; e = 0, 1. If s ≠ 0 then 2r = 1, 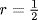 ,
, 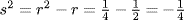 , (2s)2 = − 1.
, (2s)2 = − 1.
30.
a. and c. These are routine calculations.
e. 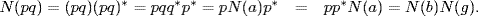
31.
a. and c. are routine verifications.
e. If 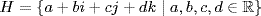 then (b) shows that
then (b) shows that
![]()
where  denotes the conjugate of z. In this form H is easily verified to be a subring of
denotes the conjugate of z. In this form H is easily verified to be a subring of 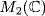 . Since (a) and (d) determine the multiplication, we have
. Since (a) and (d) determine the multiplication, we have 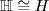 .
.
32. Since R is commutative, Lemma 1 holds in 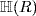 ; the proof is the same.
; the proof is the same.
a. If q is a unit in  then 1 = N(1) = N(qq−1) = N(q)N(q−1), so N(q) is a unit in R. Conversely qq∗ = N(q) shows q−1 = N(q)−1q∗ if N(q)
then 1 = N(1) = N(qq−1) = N(q)N(q−1), so N(q) is a unit in R. Conversely qq∗ = N(q) shows q−1 = N(q)−1q∗ if N(q)  R∗.
R∗.
c. Let q = a + bi + cj + dk in 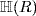 . Then
. Then
![]()
Similarly qj = jq  2a = 2c = 0 and qk = kq
2a = 2c = 0 and qk = kq  2b = 2c = 0. Since
2b = 2c = 0. Since  , qj = jq and qk = kq, the result follows. If R has characteristic 2, then 2r = 0 for all r
, qj = jq and qk = kq, the result follows. If R has characteristic 2, then 2r = 0 for all r  R, so
R, so  , that is
, that is 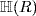 is commutative. Conversely, if
is commutative. Conversely, if 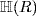 is commutative, (1 + ai)j = j(1 + ai) for all a
is commutative, (1 + ai)j = j(1 + ai) for all a  R, so ak = − ak, 2a = 0. Thus R has characteristic 2. Finally, A2(Z6) = {0, 3} = 3Z6, so
R, so ak = − ak, 2a = 0. Thus R has characteristic 2. Finally, A2(Z6) = {0, 3} = 3Z6, so
![]()
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
