11.1 Wedderburn's Theorem
1. If RM is simple, then M = Rx for any 0 ≠ x  M. Hence α : R → M given by α(r) = rx is an onto R-linear map, so M ≅ R/L where L = ker(α), and L is maximal because M is simple. Conversely, R/L is simple for every maximal left ideal L. Conversely, R/L is simple for every maximal left ideal L again by Theorem 6 §8.1.
M. Hence α : R → M given by α(r) = rx is an onto R-linear map, so M ≅ R/L where L = ker(α), and L is maximal because M is simple. Conversely, R/L is simple for every maximal left ideal L. Conversely, R/L is simple for every maximal left ideal L again by Theorem 6 §8.1.
3. Define σ : Ra → Rb by σ(ra) = rb. This is well defined because ra = 0 implies rbR = r(aR) = 0, so rb = 0 . So σ is an onto homomorphism of left R-modules with σ(a) = b. Finally if σ(ra) = 0 then rb = 0 so (ra)R = r(aR) = r(bR) = 0, so ra = 0 . Hence σ is one-to-one.
5. If L1⊆ L2 ⊆  are left ideals of eRe, then RL1⊆ RL2 ⊆
are left ideals of eRe, then RL1⊆ RL2 ⊆  are left ideals of R so RLn = RLn+1 =
are left ideals of R so RLn = RLn+1 = for some n by hypothesis. If i ≥ n, the fact that Li ⊆ eRe for each i, gives Li = eLi ⊆ eRLi = eRLn = eReLn ⊆ Ln. Hence Ln = Ln+1 =
for some n by hypothesis. If i ≥ n, the fact that Li ⊆ eRe for each i, gives Li = eLi ⊆ eRLi = eRLn = eReLn ⊆ Ln. Hence Ln = Ln+1 =  , as required.
, as required.
7. If RM is finitely generated, then 143 M is an image of Rn for some n ≥ 1 by Theorem 5 §7.1 and its Corollaries. Since Rn is noetherian as a left R-module (Corollary 1 of Lemma 2), the same is true of M by Lemma 2.
8. a. Observe that ann(X) is a left ideal for any X ⊆ M (note that ann (∅) = R) . Hence use the artinian condition to choose ann(X) minimal in
![]()
Then ann(M) ⊆ ann(X) is clear; we prove equality. If a  ann(X), suppose that a ∉ ann(M), say am ≠ 0 with m
ann(X), suppose that a ∉ ann(M), say am ≠ 0 with m  M. Put Y = X ∪ {m} . Then ann(Y) ⊆ ann(X), so ann(Y) = ann(X) by minimality. But a
M. Put Y = X ∪ {m} . Then ann(Y) ⊆ ann(X), so ann(Y) = ann(X) by minimality. But a  ann(Y) and so am = 0, a contradiction. Hence a
ann(Y) and so am = 0, a contradiction. Hence a  ann(M) and we have proved (a).
ann(M) and we have proved (a).
9. Let  and p does not divide m} . If
and p does not divide m} . If 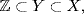 where Y is a subgroup of X, choose
where Y is a subgroup of X, choose 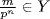 with n maximal (n exists because Y ≠ X,
with n maximal (n exists because Y ≠ X, 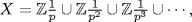 and
and 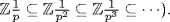 Then
Then 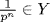 by Theorem 4 §1.2 because m and pn are relatively prime. Hence
by Theorem 4 §1.2 because m and pn are relatively prime. Hence 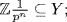 we claim that this is equality. To see this, let
we claim that this is equality. To see this, let 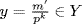 where p does not divide m′ . Then k ≤ n by the maximality of n, so
where p does not divide m′ . Then k ≤ n by the maximality of n, so  whence
whence 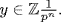
11. a. If x  M then x− π(x)
M then x− π(x)  ker π, and it follows that M = π(M)+ ker π. If y
ker π, and it follows that M = π(M)+ ker π. If y π(M) ∩ ker π write y = π(z), z
π(M) ∩ ker π write y = π(z), z  M. Since y
M. Since y ker π, we have 0 = π(y) = π2(z) = π(z) = y, so π(M)∩ ker π = 0 . This shows that M = π(M)⊕ ker π. (c). We have ker π = (1 − π)(M) because π(x) = 0 if and only if (1 − π)(x) = x. And π(M) = ker(1 − π) because (1 − π)(x) = 0 if and only if x = π(x).
ker π, we have 0 = π(y) = π2(z) = π(z) = y, so π(M)∩ ker π = 0 . This shows that M = π(M)⊕ ker π. (c). We have ker π = (1 − π)(M) because π(x) = 0 if and only if (1 − π)(x) = x. And π(M) = ker(1 − π) because (1 − π)(x) = 0 if and only if x = π(x).
13. a. Suppose that K = K1⊕ K2 ⊕  is an infinite direct sum of nonzero submodules of M. Then K⊃ K2 ⊕ K3 ⊕
is an infinite direct sum of nonzero submodules of M. Then K⊃ K2 ⊕ K3 ⊕  ⊃ K3 ⊕ K4 ⊃
⊃ K3 ⊕ K4 ⊃  contradicts the DCC, and K1⊂ K1 ⊕ K2 ⊂ K1 ⊕ K2 ⊕ K3 ⊂
contradicts the DCC, and K1⊂ K1 ⊕ K2 ⊂ K1 ⊕ K2 ⊕ K3 ⊂  contradicts the ACC.
contradicts the ACC.
15. Here 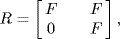 and we consider
and we consider  Then e2 = e and
Then e2 = e and 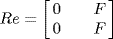 is not simple as
is not simple as 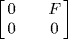 is a submodule. But end
is a submodule. But end 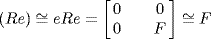 as rings.
as rings.
17. a. We have ker(α)⊆ ker(α2) ⊆ ker(α3) ⊆  so, since M is noetherian, there exists n ≥ 1 such that ker(αn) = ker(αn+1) =
so, since M is noetherian, there exists n ≥ 1 such that ker(αn) = ker(αn+1) =  . If x
. If x  ker(α) then (since αn is onto) write x = αn(y) for some y
ker(α) then (since αn is onto) write x = αn(y) for some y  M. Then 0 = α(x) = αn+1(y), so y
M. Then 0 = α(x) = αn+1(y), so y  ker(αn+1) = ker(αn) . Hence x = αn(y) = 0, proving that ker(α) = 0.
ker(αn+1) = ker(αn) . Hence x = αn(y) = 0, proving that ker(α) = 0.
19.
a. The easy verification that θ is an F-linear homomorphism of additive groups is left to the reader. To show that θ is one-to-one, suppose that θ(r) = 0 ; that is, uir = 0 for each i. If 1 = ∑ iaiui, then r = 1 · r = ∑ iaiuir = 0, so ker θ = 0 and θ is one-to-one. Finally, let θ(s) = [sij] so that 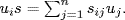 Then:
Then:
![]()
Thus 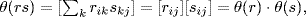 and the proof is complete.
and the proof is complete.
c. 

c. 
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
