14 APPROXIMATE METHODS
1.4 THE INVERSE PROBLEM
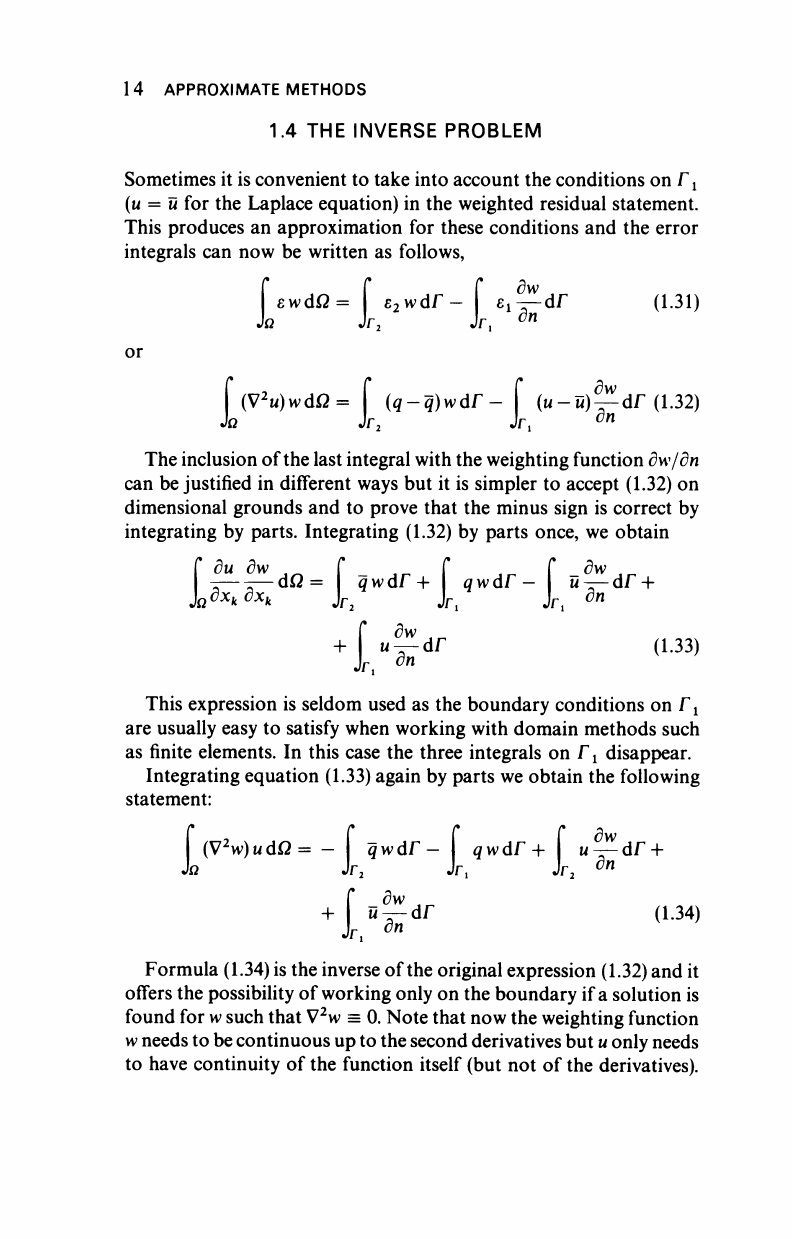
Sometimes it is convenient to take into account the conditions on Γ
ι
(u = ü for the Laplace equation) in the weighted residual statement.
This produces an approximation for these conditions and the error
integrals can now be written as follows,
I ewdQ = I s
2
wdr- I ^i^cir (1.31)
or
(V
2
w)wdfl= (q-q)wdr- (u-ü)-^dr (1.32)
h
Jr
2
Jr,
dn
The inclusion of the last integral with the weighting function dw/dn
can be justified in different ways but it is simpler to accept (1.32) on
dimensional grounds and to prove that the minus sign is correct by
integrating by parts. Integrating (1.32) by parts once, we obtain
J
du dw
1Λ
f ,„ f
tr
^ C dw
fr
,
—-—-άΩ= qwdr+ qwdr - ΰ—-άΓ +
Q
dx
k
dx
k
J
r
J
r
J
r
dn
^df (1.33)
on
This expression is seldom used as the boundary conditions on Γ
χ
are usually easy to satisfy when working with domain methods such
as finite elements. In this case the three integrals on Γ
χ
disappear.
Integrating equation (1.33) again by parts we obtain the following
statement:
^dr + (V
2
w)wdß= - qwdr- qwdr + u^-dr
h Jr
2
Jr, Jr
2
dn
+ I »^dr (1.34)
Formula (1.34) is the inverse of
the
original expression (1.32) and it
offers the possibility of working only on the boundary if a solution is
found for w such that V
2
w = 0. Note that now the weighting function
w
needs to be continuous up to the second derivatives but
u
only needs
to have continuity of the function itself (but not of the derivatives).

APPROXIMATE METHODS
1
5
Here again the weighting function w can be found using the same
functions as for the approximating function u or different functions.
Statements (1.32), (1.33) and (1.34) permit us to establish a
classification of the different approximating techniques in accordance
with the type of statement one uses and the relationship between the
approximating and the weighting functions. Note that the first two
statements usually give the domain methods but the last one can be
used to build boundary solutions.
Figure 1.2 illustrates the different types of approximating tech-
niques some of which have already been explained.
Example 1.6
Consider again the equation,
dx
2
+
u
+ x = 0 in Ω (a)
with boundary conditions u = 0 at x = 0 and at x = 1.
Statement (32) can be written (note all the boundary is of the Γ
χ
type),
lx = 1
0
{^
+ u +
x
)
wdx+
[
(u
-
u
aw
= 0 (b)
JC
= 0
Integrating by parts twice we obtain
Note that now we need to find a solution that satisfies the equation
d
2
w
^
r +
w = 0 (d)
The general solution is,
w = ß
x
cos x + ß
2
sin x (e)
with
dw/dx = - β! sin x + ß
2
cos x (f)

1
st statement
original one
formula (1.22)
Functions for u and w
are the same
Original Galerkin
2nd statement
weak formulations
equation (1.24)
Functions for u and w
are the same
Galerkin-Finite
element techniques
3rd statement
inverse formulation
equation (1.25)
Functions for u and w
are the same
Classical Trefftz
method
Functions for u and w
are different
Method of moments,
finite differences
Functions for u and w
are different
General weak
formulations
Functions for u and w
are different
Singular boundary
solutions and others
Figure 1.2 Different approximating techniques
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
