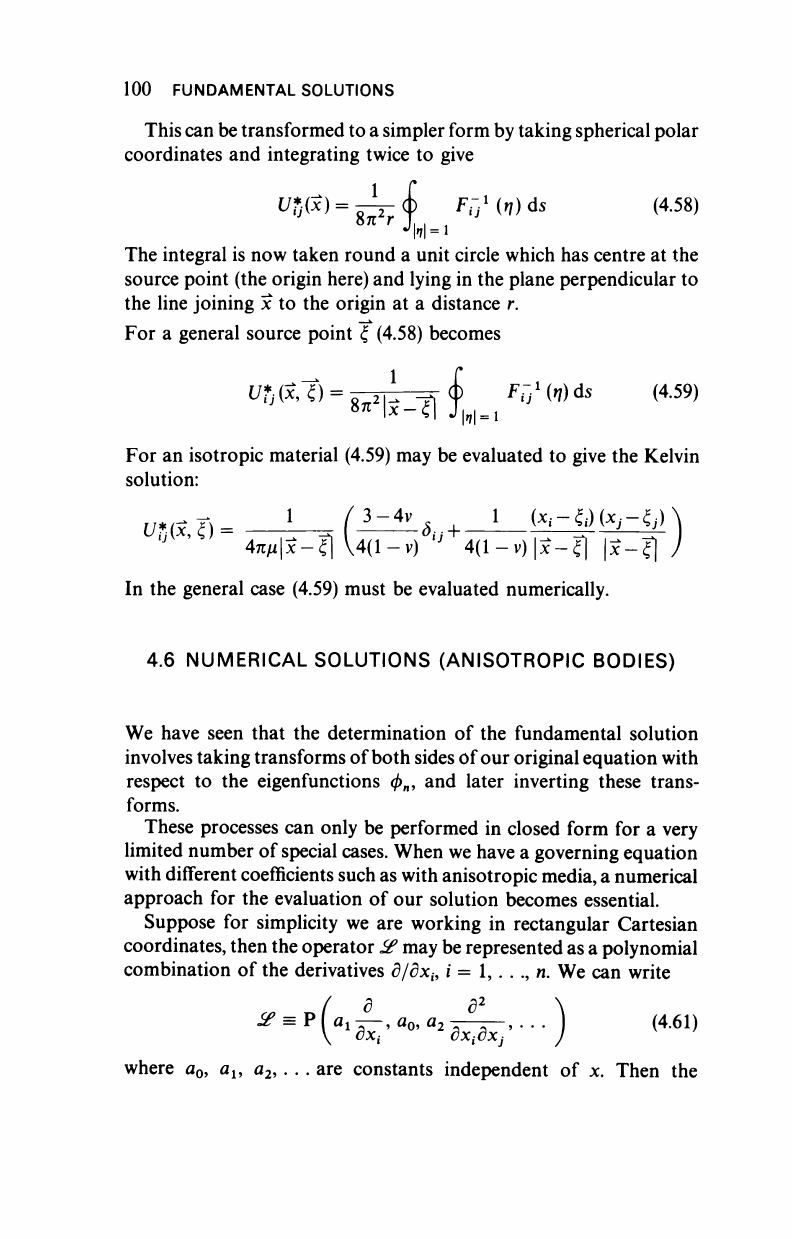
100 FUNDAMENTAL SOLUTIONS
This can be transformed to a simpler form by taking spherical polar
coordinates and integrating twice to give
VTj(x)
= A-& Ρ^(η)ά8
(4.58)
8πΓ
^|
=
ι
The integral is now taken round a unit circle which has centre at the
source point (the origin here) and lying in the plane perpendicular to
the line joining
3c
to the origin at a distance r.
For a general source point ξ (4.58) becomes
Ufj
(3c,
t)
=
-,,1
-?, <j>
FT,
l
(f) ds (4.59)
For an isotropic material (4.59) may be evaluated to give the Kelvin
solution:
„
sft
a__>
(iz*.^-· .<*-«<*-«
4πμ|χ-|1 4(l-v) " 4(1
—
v)
|3c —^|
χ-ξ
In the general case (4.59) must be evaluated numerically.
4.6 NUMERICAL SOLUTIONS (ANISOTROPIC BODIES)
We have seen that the determination of the fundamental solution
involves taking transforms of both sides of our original equation with
respect to the eigenfunctions φ
η
, and later inverting these trans-
forms.
These processes can only be performed in closed form for a very
limited number of special cases. When we have a governing equation
with different coefficients such as with anisotropic media, a numerical
approach for the evaluation of our solution becomes essential.
Suppose for simplicity we are working in rectangular Cartesian
coordinates, then the operator if may be represented as a polynomial
combination of the derivatives d/dx
i9
i = 1, . . ., n. We can write
ΞΡ
(
αι
έ
,α
°'
where a
0
, a
u
a
2
, . . . are constants independent of x. Then the
^
p
i
fl
'^
fl
»·^'-
'
(461)
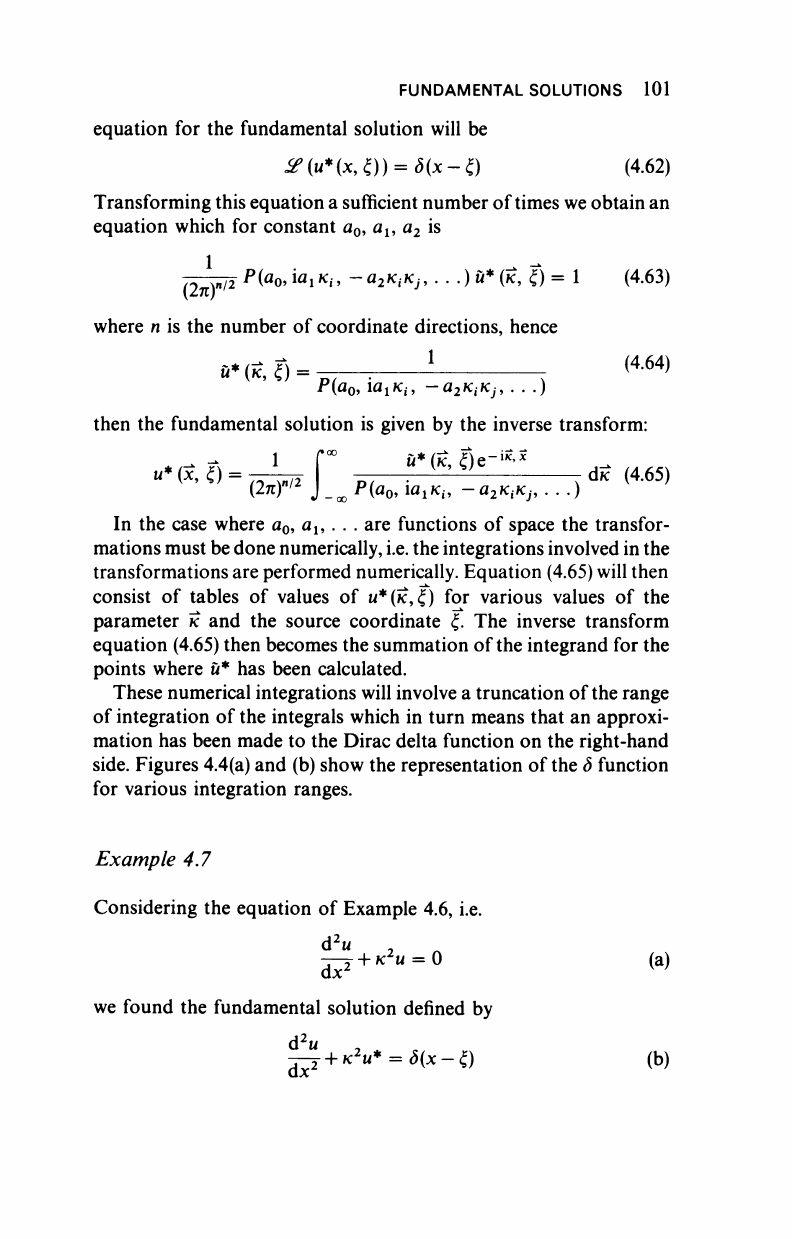
FUNDAMENTAL SOLUTIONS 101
equation for the fundamental solution will be
<?(ι**(χ,ξ)) = δ(χ-ξ) (4.62)
Transforming this equation a sufficient number of times we obtain an
equation which for constant a
0
, a
u
a
2
is
π v./2 P(ßoAaKi, -a
2
KiKj, . . .) ü* (κ, ξ) = 1 (4.63)
(2π)
where n is the number of coordinate directions, hence
VKti-rT-.
Ϊ
(464)
P(a
0
,
ia
x
K
i9
-a
2
KiK
j9
. . .)
then the fundamental solution is given by the inverse transform:
-*
<*»- ί^ ί".
,^ί*-·.;"„■..,
d
«
«
4
·
65
>
In the case where a
0
, a
i9
. . . are functions of space the transfor-
mations must be done numerically, i.e. the integrations involved in the
transformations are performed numerically. Equation (4.65) will then
consist of tables of values of Μ*(Κ,£) for various values of the
parameter κ and the source coordinate ξ. The inverse transform
equation (4.65) then becomes the summation of
the
integrand for the
points where ü* has been calculated.
These numerical integrations will involve a truncation of
the
range
of integration of the integrals which in turn means that an approxi-
mation has been made to the Dirac delta function on the right-hand
side. Figures 4.4(a) and (b) show the representation of the δ function
for various integration ranges.
Example 4.7
Considering the equation of Example 4.6, i.e.
dx
we found the fundamental solution defined by
2
+ K
2
U = 0 (a)
^ +
κ
2
ι**
= δ(χ-ξ) (b)
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
