
22 APPROXIMATE METHODS
Taking into consideration symmetry this reduces to,
l'(
dAv dv
v-z—-Γ-ΔΌ dr = o (f)
0 x
on on
Substituting (d) into (f) we obtain the following equation:
Γ
1
[ift(l + y
2
) (4 -
12y
2
)A(x
+ a(4 - 12y
2
) (1 - 6y
2
+ /)Ja] dy = 0
Jo
(g)
Hence,
[ii»(l +y
2
)+a(l -6y
2
+ /)] (4- 12y
2
)dy = 0 (h)
This gives after integration,
-^ + α^ = 0 (i)
.-.α = ιί4& (j)
Whence the value of v becomes,
v =
<x(x*
-6x
2
y
2
+ y*) =
T^bix*
-6x
2
y
2
+ y*) (k)
and u is given by formula (b).
Note that the magnitude of
u
or
v
may be displaced from the correct
solution by a constant value. In order to determine this value one can
carry out the following integration over the x=l,0<y<l side:
• 1
(v-v-c)dy
= 0 (1)
/o
or,
»1
[-ifc(l+>;
2
)
+ a(l-6>>
2
+ /)-c]d); = 0 (m)
ί
Jo
r
Thus,
c=~mb (n)
Example 1.9
Let us now solve the same problem as in Example 1.8, but using
boundary collocation and starting with equation (9). We can take as
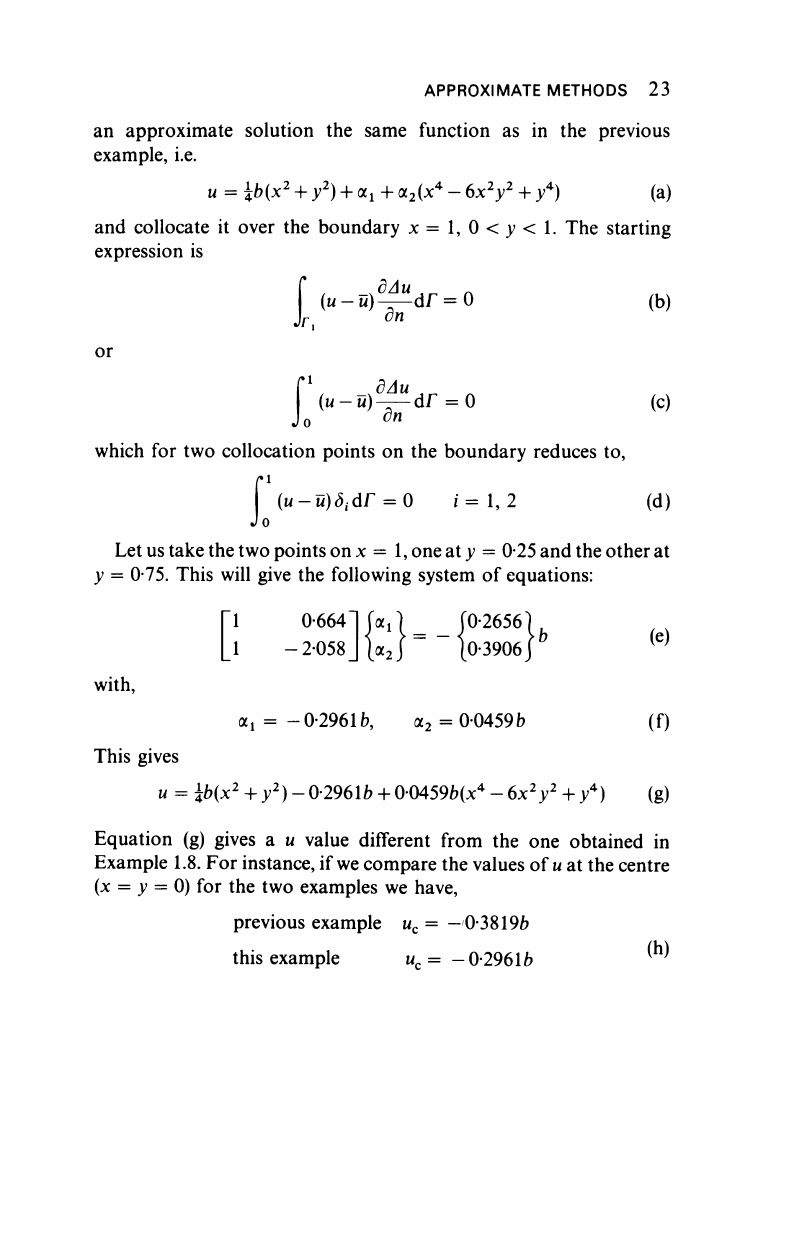
APPROXIMATE METHODS 23
an approximate solution the same function as in the previous
example, i.e.
u = ±b(x
2
+ y
2
) + a
x
+ a
2
(x
4
- 6x
2
y
2
+ /) (a)
and collocate it over the boundary x = 1, 0 < y < 1. The starting
expression is
* 1
(„-Ar-o
on
or
ί
(u-ü)
d
-pdr
= 0
which for two collocation points on the boundary reduces to,
Jo
(u-ü)(5,dr = 0 i = 1, 2
(b)
(c)
(d)
Let us take the two points on x =
1,
one at y = 0-25 and the other at
y = 0-75. This will give the following system of equations:
0-664
-2-058
0-26561
0-3906
J
with,
a, = -0-2961
b,
a
2
= 00459
b
This gives
u = ib(x
2
+y
2
)-02961b+ 00459b(x
4
-6x
2
y
2
+ y*)
(e)
(0
(g)
Equation (g) gives a u value different from the one obtained in
Example 1.8. For instance, if
we
compare the values of
M
at the centre
(x = y = 0) for the two examples we have,
previous example u
c
= —0-3819fc
this example u
c
= —0-29616
(h)
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
