
FUNDAMENTAL SOLUTIONS 89
Note. For real functions the hat denoting complex conjugation
becomes superfluous.
Example 4.2
Considering again our function on the rectangle —a^x
l
^
:
a,
—
b ^ x
2
^ b, we have
^(-r) =
4
-^
n
|
o
exp(^
1
-i
1
))exp(^(x
2
-
i2
))(a)
Let u(x,y) be any function on the rectangle. We may write
u(x
l9
x
2
) = —= X a„exp exp —— (b)
2jabn
= o
a
J
b
)
where a„ are complex constants given by
1 Γ
° Γ / ^ (
ϊπηχ
Λ ( ™*Χι
Α Α
ii(x
1
,x
2
)expl — lexpl — ldx
1
dx
2
2 Jab]
(c)
which in this case is the form of the coefficients in a double Fourier
series in terms of a double 'Fourier transform' of u.
4.3 FUNDAMENTAL SOLUTIONS ON A FINITE REGION
We are now in a position to define the fundamental solution ug(x, ξ)
for a linear operator if in a region Ω by
&(νί(χ,ξ)) = δ
Ω
(χ-ξ) (4.19)
Jz?
involves differentials in the variables x. If we expand
u%
and δ
Ω
in
terms of eigenfunctions: putting
«Ä(*,£)= Σ «.(T)*„(3c)
n = 0

90 FUNDAMENTAL SOLUTIONS
we obtain
/ 00 00 _^
2
Σ «.(?)*„(*) = Σ
Φη(*)$Λξ)
(4-20)
n = 0 / n = 0
with
hence
η = 0 π = 0
X α„(ξμ,Α(ϊ) = X φ„$)$
η
{ξ) (4.21)
This is an identity true for all
3c
and ξ. Hence we may deduce
*Λξ) = ~- (4.22)
This formula may also be deduced by using the orthogonality of our
eigenfunctions. Here
λ„
is assumed not to be a function of x, i.e.
<£
has
constant coefficients.
Hence the fundamental solution u$(x, ξ) is given by
"£(*,£) = Σ ^τ-Φη(χ) (4.23)
n = 0
Λ
π
This function represents the
field
due to a point source at ξ together
with 'image' sources generated by the boundary of the region Ω.
Example 4.3
For our example of Laplace's equation on a rectangle discussed in
Example 4.1 and 4.2 we have
and
_, 1 (ΊπηχΛ (Ίπηχ
2
φ
η
{χ) = —^=exp exp ——- (b)
IJab
a
J b J
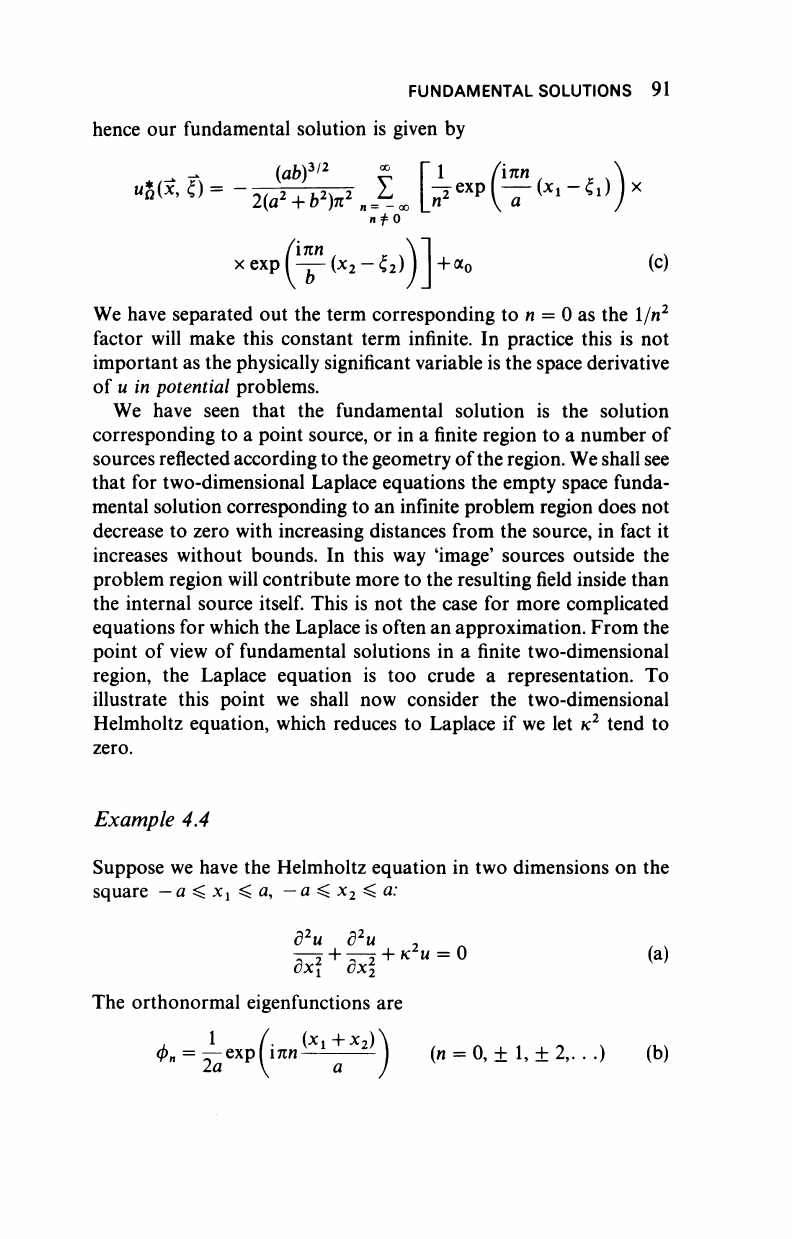
FUNDAMENTAL SOLUTIONS 91
hence our fundamental solution is given by
(ab)
312
« Γΐ (πη ,
e
Λ
xexpi^(x
2
-£
2
)j + α
0
(c)
We have separated out the term corresponding to n = 0 as the 1/n
2
factor will make this constant term infinite. In practice this is not
important as the physically significant variable is the space derivative
of u in potential problems.
We have seen that the fundamental solution is the solution
corresponding to a point source, or in a finite region to a number of
sources reflected according to the geometry of the region. We shall see
that for two-dimensional Laplace equations the empty space funda-
mental solution corresponding to an infinite problem region does not
decrease to zero with increasing distances from the source, in fact it
increases without bounds. In this way 'image' sources outside the
problem region will contribute more to the resulting field inside than
the internal source
itself.
This is not the case for more complicated
equations for which the Laplace is often an approximation. From the
point of view of fundamental solutions in a finite two-dimensional
region, the Laplace equation is too crude a representation. To
illustrate this point we shall now consider the two-dimensional
Helmholtz equation, which reduces to Laplace if we let κ
2
tend to
zero.
Example 4A
Suppose we have the Helmholtz equation in two dimensions on the
square
—
a ^ x
x
^ a,
—
a ^ x
2
^ a:
d
2
u d
2
u
2
e*
+
M
+
KU
=
°
(a)
The orthonormal eigenfunctions are
φ
η
= ^χρ(ίπη
{Χι+
α
Χ2
Λ (η = 0,±1,±2,...) (b)
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
