
FUNDAMENTAL
SOLUTIONS 95
4.5 THE FUNDAMENTAL SOLUTION IN INFINITE SPACE
The fundamental solution
in
infinite space corresponding
to an
isolated point source
at the
point
ξ is
defined
by
Χ(
α
*{χ,ξ)) = δ(χ-ξ)
(4.36)
Expanding u*
and δ in
terms
of
eigenfunctions,
i.e.
putting
«•(3c,
{) =
<
φ(κ, x),
&(κ,
ξ) y
e
(4.37)
and
δ(χ-
ξ) =
<
φ(κ,
5t),
φ(κ, ξ) >-
(4.38)
we write (4.36)
Jä?(
<
φ(κ, x),
a(ic,
ξ) >
g
) =
<
φ(κ, χ), φ(κ, ζ) }
g
(4.39)
.·.
<Χ(φ(κ,
χ)
St(ic,
ξ)
>
jf
= <φ(κ, χ), φ(κ, ξ) >^
(4.40)
.·.
(λ(κ)φ(κ,
χ),
δ(κ,Τ)
>?=
<Φ(κ,
χ), φ(κ, ξ) >-
(4.41)
which can
be
written
<
φ(κ,
5t),
1(κ)οί(κ,
ξ)
}
g
= <φ(κ, χ), φ(κ, ξ) >£
(4.42)
This
is an
identity
for all
3c
and ξ,
hence
we may
write
*
{κ
>
ξ)
=
-χ(ϊΓ
(4
·
43)
We
can compare this directly with equation (4.22) for the discrete case.
Hence
we can
write
our
fundamental solution
"*(x,
0 =
<
Φ(κ, 3c), Φ(κ, ξ)/1(κ)
>^
(4.44)
This procedure indicates the general method
of
finding fundamen-
tal solutions by transform methods. We may also use the fundamental
solution
to
solve problems like
<e{u)
= P
(4.45)
where
P is
some function
of x in our
region.
If we
put
u(x)=
<M*(X,T),/
>
«)>
0(T)
(4.46)

96 FUNDAMENTAL SOLUTIONS
we obtain equation (4.45) by substituting for u using
<?(ιι*&ξ)) = δ(ϊ-ξ)
Hence (4.46) gives the solution of (4.45).
Furthermore if we have
&(u) = Jt(P) (4.47)
where M is some linear differential operator, then
ώ(χ)=^Τ(<ιι*(χ,Γ),Ρ(Γ)>
β
) (4.48)
is the solution of
(4.47) as
can also be verified by substitution. We shall
now give a simple example to illustrate these points.
Example 4.6
Consider the one-dimensional reduced wave equation given by
-, + ^ = 0 (a)
The orthonormal eigenfunctions we choose correspond to our one-
dimensional Cartesian system and are given by
φ(κ x) = -
7
=e"
iKX
(b)
'2π
and the eigenvalues
λ(κ') =
κ
2
-κ'
2
We wish to find the fundamental solution defined by
d
2
u*
dx
2
we may write
+
K
2
U*
=δ{χ-ξ)
(c)
δ(χ-ζ)=(φ(κ,χ),φ(κ,ξ)>
κ
exp [ - ifc(x - f)] d/c (d)
-_L
Γ

FUNDAMENTAL SOLUTIONS 97
and
ιι*(χ,ξ)=(η*(κ
9
ξφ(κ
9
χ)}
κ
ξ)ζ~
ικχ
άκ
(e)
1
Γ
=
-= Ü*(K,
V
27r
J-oc
u*
(/c,
ξ) is the
Fourier transform
of
the fundamental solution given
by
i
Γ
ö*(ic,{)
= —=
u*
(K,
£) e
1K
* die
ν/
2π
J-oo
(f)
from (4.32), which
is the
inverse relationship corresponding
to (e).
The fundamental solution
is now
given
by
ξ)ζ-
{κχ
άκ'
+/c
1
iH^D**'·
, Jin
η*(κξ)ε-
ικχ
άκ'
00
i
Γ
=
—
exp[ifc'(-*
+
£)]
die'
271
J-CC
(g)
(K
2
-K'
2
)M*(K:',
^)e-
iKX
dK'
exp[iic'(
—
χ +
ξ)~άκ'
2π
J
or
(κ
2
-κ'
2
)ΰ*
=e
iK
'i le-
iKX
dK'
= 0
(h)
(i)
Note that the expression in large parentheses is the equation resulting
from taking
the
Fourier transform
of
both sides
of
equation
(c).
Note,
(i) is an
identity
for all
values
of ξ and we may
write simply
(K
2
-K'
2
)Ü*
=
1
,ίκ'ί
(j)
which gives
the
Fourier transform
of the
solution directly.

98 FUNDAMENTAL SOLUTIONS
Alternatively we may exploit the orthonormalisation of our eigen-
functions to retrieve the same result.
We have therefore
(κ
2
-κ'
2
) ^2π
and hence using (f) we can write
u*(xA)
= f
7^2^,2,
exp
|>'(£-x)]
άκ' (1)
which by simple contour integration gives (taking the real part for our
actual solution)
u*(x. ξ) = — sin (κχ-ξ) = — sin(tcr) (m)
where r is the (positive) distance between x and ξ.
The above example demonstrates the procedure used to obtain the
fundamental solution in Cartesian coordinates. The main steps of the
analysis are given below:
1 Transform the equation a sufficient number of times in order to
obtain an algebraic equation in terms of the transformed
function w*(jc, ξ).
2 Solve this equation in terms of ü*.
3 Invert the transformations to obtain the fundamental solution
u*
(3c,
ξ).
In practice it may not be necessary to perform the full number of
transformations as the resulting transformed equation at some point
may have
a
known fundamental solution. The inverse transforms may
then be performed on this function to obtain the fundamental
solution for the original equation.
As an example of the non-trivial use of this technique, the
fundamental solution for a concentrated load P applied at the point
ξ in an anisotropic elastic space will now be deduced starting from
Navier's equations in the form
3,4
^pqrs ™r, sq "
(4.49)
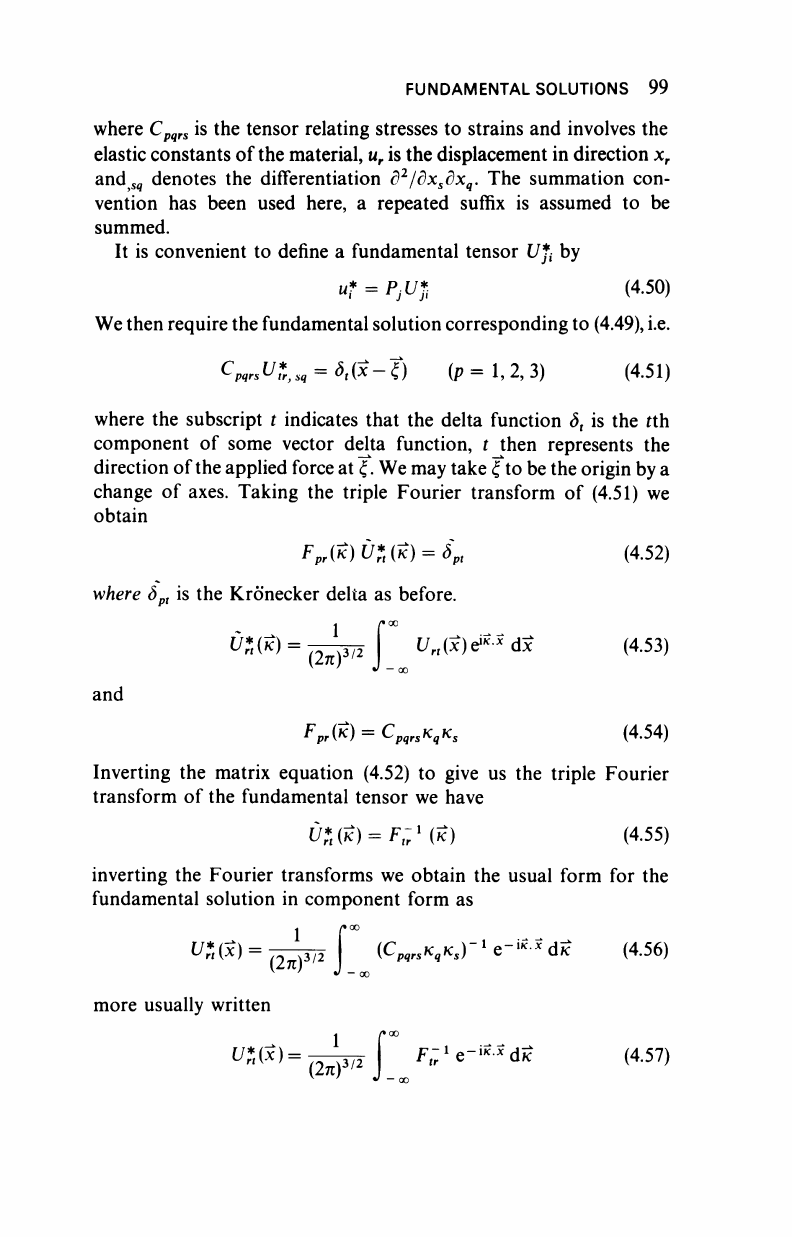
FUNDAMENTAL SOLUTIONS 99
where
C
pqrs
is the tensor relating stresses to strains and involves the
elastic constants of
the
material, u
r
is the displacement in direction x
r
and
s<?
denotes the differentiation d
2
/dx
s
dx
q
. The summation con-
vention has been used here, a repeated suffix is assumed to be
summed.
It is convenient to define a fundamental tensor l/* by
uf = PjUft (4.50)
We then require the fundamental solution corresponding to (4.49), i.e.
CwsU*^
= ö
t
(x-t) (p = 1, 2, 3) (4.51)
where the subscript t indicates that the delta function S
t
is the ith
component of some vector delta function, t then represents the
direction of
the
applied force at ξ. We may take ξ to be the origin by a
change of axes. Taking the triple Fourier transform of (4.51) we
obtain
F
pr
(K)U*(K) = ö
pt
(4.52)
where S
pt
is the Krönecker delta as before.
ύ*Λκ) = ^372 ί
U
rt
(x)e*·*
dx (4.53)
and
F
pr
(K) =
C
pqrs
K
q
K
s
(4.54)
Inverting the matrix equation (4.52) to give us the triple Fourier
transform of the fundamental tensor we have
l/*(ic) = F-
1
(ic) (4.55)
inverting the Fourier transforms we obtain the usual form for the
fundamental solution in component form as
W) = TAT2
(C
pvt
K.K,r
1
e-
iS
-*dK (4.56)
(2π)
3
more usually written
i Γ°°
'die (4.57)
t/*(3c)=
1
(2π)
3/2
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
