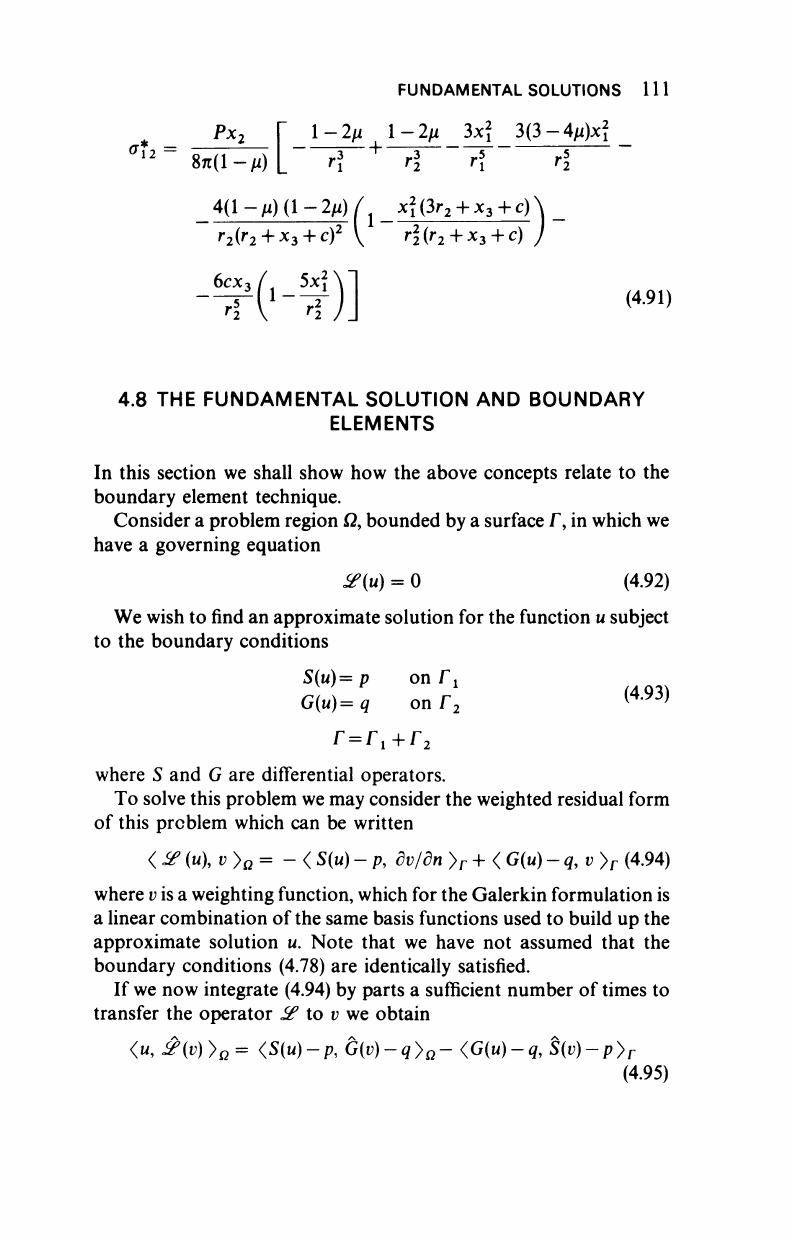
σ*
12
=
FUNDAMENTAL SOLUTIONS 111
Px
2
Γ l-2/i ί-2μ 3xj 3(3-4|t)xf
^L
Ί
+
Ί Ί Ί
8π(1
4(1-μ)(1-2μ)/'
1
_χ?(3Γ
2
+
Χ3
+ ο)
r
2
(r
2
+ X3+c) n(r
2
+ x
3
+ c)
6cx
3
ι 5χ
Τ Γϊ/j
(4.91)
4.8 THE FUNDAMENTAL SOLUTION AND BOUNDARY
ELEMENTS
In this section we shall show how the above concepts relate to the
boundary element technique.
Consider a problem region Ω, bounded by a surface Γ, in which we
have a governing equation
■Sf(«) = 0 (4.92)
We wish to find an approximate solution for the function u subject
to the boundary conditions
S{u)= p on Γ
1
G(u)= q on Γ
2
(493)
Γ
=
Γ
1+
Γ
2
where 5 and G are differential operators.
To solve this problem we may consider the weighted residual form
of this problem which can be written
<
if
(K),
v >
Ω
= -
< S(u)
- p, dv/dn >
r
+
< G(u)
-q,v}
r
(4.94)
where v is a weighting function, which for the Galerkin formulation is
a linear combination of the same basis functions used to build up the
approximate solution u. Note that we have not assumed that the
boundary conditions (4.78) are identically satisfied.
If we now integrate (4.94) by parts a sufficient number of times to
transfer the operator if to v we obtain
<n,j£(t?)>o= <S(u)-p,G(v)-qy
Q
-(G(u)-q,S(v)-p}
r
(4.95)

112 FUNDAMENTAL SOLUTIONS
where J^, G and S are the adjoint operators (corresponding to if, G
and S. (The adjoint operator is defined by forming
υ<£(μ)
- u&(v) and
making this expression a divergence, i.e. of the form du/dx + dv/dy
with
w
and v two functions
5
.)
If if is self adjoint then if = J
7
. If we now choose v to be the
fundamental solution v*(x, ξ) of J
7
defined by
if(t;*) = (5(x-f) (4.96)
then equation (4.95) becomes, by equation (4.35)
«to
c«(ö
0
= <S(H)-p, G(t>*)-q>
r
- <fi(u)-q, S(v*)-p)
r
(4.97)
notice that
v*
is a function of two variables and that (86) is an identity
for all values of the source or reference point ξ. (4.83) is the basic
equation for the direct boundary element method. If we choose ξ to be
outside our problem region Ω then we have a relation between values
of« on the boundary only. When these integrals are discretised in the
usual boundary element way we obtain a number of equations (one
for eachj:hoice of
ξ)
involving our boundary values of
u.
If
we
choose
all our ξ'δ to be outside Ω then we shall have no singular integrals to
evaluate.
Another way of eliminating the integration over our problem
region is to choose our weighting function v to be a homogeneous
solution for the operator &
9
i.e.
&(v) = 0 in Ω (4.98)
Some care is needed here, however, as we need to choose v such that it
involves another point ξ in order that we may obtain enough
equations to solve our problem. In addition if our problem region
extends to infinity then we need to ensure that the integrals along the
part of the boundary at infinity are bounded for this solution. This
condition is automatically satisfied for our choice of the fundamental
solution for this function, as can be seen from its physical
interpretation.
5
Having obtained the values of u on the boundary we can re-use
equation (4.87) to obtain values of the function
u
at any internal point ξ

FUNDAMENTAL SOLUTIONS 113
by now putting ξ ε
Ω.
We must now choose v to be the fundamental
solution in order to exploit the selective property of the delta function.
Note that for these internal points we again have no singular
integrals to evaluate after the boundary element discretisation.
Example 4.8
Let us now consider an example which illustrates how a
non-self-
adjoint problem can be solved. Consider the equation,
d
2
u du
dx
2
dx
2
- — +
w
= 0 (a)
with boundary conditions to be specified soon.
This equation can now be weighted by u* to give,
d
2
u du
dx
2
dx
33--
+
M
)u*dx = 0 (b)
If we integrate this expression by parts twice we obtain,
C/d
2
u*
du*
JV^
r +
^r
+
W
*J
Wdx=
"Ι^-Φ*]Γ
+
Ι>*"]Γ
(C)
We have to find a solution to the adjoint equation, i.e.
d
2
u* du*
dx
2
dx
+
u*
= 0 (d)
Note that this is the same type of equation as for a damped one degree
of freedom system. Hence we can take
u* = e~
x/2
Asin(^A-x j + ßcos(^-|-x j (e)
where A and B are arbitrary constants, to be determined from the
boundary conditions.
Hence we can write,
[(q-u)u*]
r
= [q*ul (f)
..................Content has been hidden....................
You can't read the all page of ebook, please click here login for view all page.
