8.13.  LINEAR ALGEBRAIC ASPECTS OF CONTROL-SYSTEM DESIGN COMPUTATIONS [21–24]

The fundamental problems of linear control-system theory were reconsidered at the end of the 1960s and the beginning of the 1970s. The algebraic characteristics of the control-system problems were reestablished and have resulted in a better understanding of the foundations of linear control-system theory.

This section focuses on the interplay between some recent results and methodologies in numerical linear algebra and their application to problems arising in control systems. Let us reconsider the phase-variable canonical form representation of a control system:

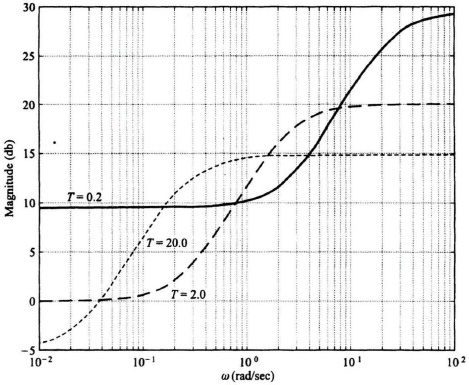
Figure 8.35  Optimal sensitivity frequency characteristic as a function of T.

We can represent this canonical form representation in terms of its frequency response by using Eqs. (8.10) and (8.18):

The problem of computing C(s)/R(s), or C(jω/R(jω), from knowledge of K, P, b, L, and h, efficiently is a practical problem of great interest. Reference 23 presents an efficient and generally applicable algorithm to solve this problem. The approach recommended performs an initial reduction of P to upper Hessenberg form H, rather than solving the linear equation (with dense, unstructured P) (jωI− P)X = b, which would require O(n³) operations for each successive value of ω. The orthogonal matrices used to effect the Hessenberg form of P are incorporated into b and L giving and . Therefore, as ω varies, the coefficient matrix in the linear equation (jωI − H)X = remains in upper Hessenberg form. The advantage is that X can be determined in O(n²) operations rather than O(n³) as before, which is a very significant saving. In addition, this methodology is numerically very stable and has the advantage of being independent of the eigenstructure (possibly ill-conditioned) of P.

This methodology can be extended to state-variable models in implicit form. For example, let us replace Eq. (8.264) by

Therefore, Eq. (8.266) can be replaced with the following:

We can use the initial triangular/Hessenberg reduction to again reduce the problem to one of updating the diagonal of a Hessenberg matrix and, therefore, an O(n²) linear equation problem. [24].